Difference between revisions of "Flow (continuous-time dynamical system)"
From Encyclopedia of Mathematics
(MSC|37-01) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 5: | Line 5: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406506.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406506.png" /></td> </tr></table> | ||
| − | In this case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406507.png" /> is usually called | + | In this case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406507.png" /> is usually called "time" and the dependence of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406508.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f0406509.png" /> (for a fixed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065010.png" />) is said to be the "motion" of the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065011.png" />; the set of all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065012.png" /> for a given <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065013.png" /> is called the trajectory (or orbit) of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065014.png" /> (sometimes this term is used to describe the function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065015.png" />). Just as for traditional dynamical systems the phase space of a flow usually is provided with a certain structure with which the flow is compatible: the transformations <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065016.png" /> preserve this structure and certain conditions are imposed on the manner in which <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065017.png" /> depends on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065018.png" />. |
In applications one usually encounters flows described by autonomous systems (cf. [[Autonomous system|Autonomous system]]) of ordinary differential equations | In applications one usually encounters flows described by autonomous systems (cf. [[Autonomous system|Autonomous system]]) of ordinary differential equations | ||
| Line 13: | Line 13: | ||
or, in vector notation, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065020.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065021.png" />. The immediate generalization of a flow is a flow on a differentiable manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065022.png" /> defined ( "generated" ) by a smooth vector field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065023.png" /> of class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065024.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065025.png" /> (a smooth flow of class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065027.png" />) given on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065028.png" />. In this case the motion of a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065029.png" />, as long as it stays within one chart (local coordinate system), is described by a system of the form (*), in the right-hand side of which one finds the components of the vector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065030.png" /> in the corresponding coordinates. When passing to another chart the description of the motion changes, since in this case both the coordinates of the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065031.png" /> change as well as the expressions for the components of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065032.png" /> as functions of the local coordinates. See also [[Measurable flow|Measurable flow]]; [[Continuous flow|Continuous flow]]; [[Topological dynamical system|Topological dynamical system]]. | or, in vector notation, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065020.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065021.png" />. The immediate generalization of a flow is a flow on a differentiable manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065022.png" /> defined ( "generated" ) by a smooth vector field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065023.png" /> of class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065024.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065025.png" /> (a smooth flow of class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065027.png" />) given on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065028.png" />. In this case the motion of a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065029.png" />, as long as it stays within one chart (local coordinate system), is described by a system of the form (*), in the right-hand side of which one finds the components of the vector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065030.png" /> in the corresponding coordinates. When passing to another chart the description of the motion changes, since in this case both the coordinates of the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065031.png" /> change as well as the expressions for the components of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f040/f040650/f04065032.png" /> as functions of the local coordinates. See also [[Measurable flow|Measurable flow]]; [[Continuous flow|Continuous flow]]; [[Topological dynamical system|Topological dynamical system]]. | ||
| − | Flows form the most important class of dynamical systems and were, moreover, the first to be studied. The term | + | Flows form the most important class of dynamical systems and were, moreover, the first to be studied. The term "dynamical system" is often used in a narrow sense, meaning precisely a flow (or a flow and a [[Cascade|cascade]]). |
| Line 21: | Line 21: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N.P. Bhatia, G.P. Szegö, "Stability theory of dynamical systems" , Springer (1970) {{MR|0289890}} {{ZBL|0213.10904}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.P. [I.P. Kornfel'd] Cornfel'd, S.V. Fomin, Ya.G. Sinai, "Ergodic theory" , Springer (1982) (Translated from Russian) {{MR|832433}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Palis, W. de Melo, "Geometric theory of dynamical systems" , Springer (1982) {{MR|0669541}} {{ZBL|0491.58001}} </TD></TR></table> |
Revision as of 10:30, 27 March 2012
2020 Mathematics Subject Classification: Primary: 37-01 [MSN][ZBL]
A dynamical system determined by an action of the additive group of real numbers 
(or additive semi-group of non-negative real numbers) on a phase space 
. In other words, to each 
(to each 
) corresponds a transformation 
such that
 |
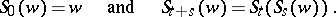In this case 
is usually called "time" and the dependence of 
on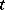 
(for a fixed 
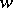) is said to be the "motion" of the point 
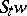; the set of all 
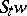for a given 
is called the trajectory (or orbit) of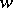 
(sometimes this term is used to describe the function 
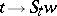). Just as for traditional dynamical systems the phase space of a flow usually is provided with a certain structure with which the flow is compatible: the transformations 
preserve this structure and certain conditions are imposed on the manner in which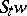 
depends on 
.
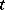In applications one usually encounters flows described by autonomous systems (cf. Autonomous system) of ordinary differential equations
 | (*) |
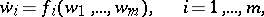or, in vector notation,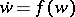 
, 
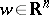. The immediate generalization of a flow is a flow on a differentiable manifold 
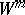defined ( "generated" ) by a smooth vector field 
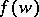of class 
, 
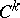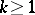(a smooth flow of class 
) given on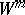 
. In this case the motion of a point 
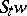, as long as it stays within one chart (local coordinate system), is described by a system of the form (*), in the right-hand side of which one finds the components of the vector 
in the corresponding coordinates. When passing to another chart the description of the motion changes, since in this case both the coordinates of the point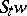 
change as well as the expressions for the components of 
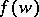as functions of the local coordinates. See also Measurable flow; Continuous flow; Topological dynamical system.
Flows form the most important class of dynamical systems and were, moreover, the first to be studied. The term "dynamical system" is often used in a narrow sense, meaning precisely a flow (or a flow and a cascade).
Comments
For general introductions into the theory of continuous, measurable or smooth flows, consult, respectively, [a1], [a2] and [a3].
References
| [a1] | N.P. Bhatia, G.P. Szegö, "Stability theory of dynamical systems" , Springer (1970) MR0289890 Zbl 0213.10904 |
| [a2] | I.P. [I.P. Kornfel'd] Cornfel'd, S.V. Fomin, Ya.G. Sinai, "Ergodic theory" , Springer (1982) (Translated from Russian) MR832433 |
| [a3] | J. Palis, W. de Melo, "Geometric theory of dynamical systems" , Springer (1982) MR0669541 Zbl 0491.58001 |
How to Cite This Entry:
Flow (continuous-time dynamical system). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Flow_(continuous-time_dynamical_system)&oldid=23609
Flow (continuous-time dynamical system). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Flow_(continuous-time_dynamical_system)&oldid=23609
This article was adapted from an original article by D.V. Anosov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article