Difference between revisions of "Sinusoid"
(TeX encoding is done + graph) |
(picture improved (see talk)) |
||
| Line 3: | Line 3: | ||
The graph of the function $y=\sin x$ (see Fig.). The sinusoid is a continuous curve with period $T=2\pi$. It intersects the $x$-axis at the points $(k\pi, 0)$. These are also points of inflection, meeting the $x$-axis at an angle of $\pm\pi/4$. The extrema are at the points $((k+1/2)\pi, (-1)^k)$. | The graph of the function $y=\sin x$ (see Fig.). The sinusoid is a continuous curve with period $T=2\pi$. It intersects the $x$-axis at the points $(k\pi, 0)$. These are also points of inflection, meeting the $x$-axis at an angle of $\pm\pi/4$. The extrema are at the points $((k+1/2)\pi, (-1)^k)$. | ||
| − | <asy> | + | <center><asy> |
import graph; | import graph; | ||
| − | size( | + | size(450); |
real f(real x) {return sin(x);}; | real f(real x) {return sin(x);}; | ||
real f1(real x) {return cos(x);}; | real f1(real x) {return cos(x);}; | ||
| − | draw(graph(f1,-2*pi,2*pi),blue,"$\cos(x)$"); | + | draw(graph(f1,-2*pi,2*pi),blue+1,"$\cos(x)$"); |
| − | draw(graph(f,-2*pi,2*pi),red,"$\sin(x)$"); | + | draw(graph(f,-2*pi,2*pi),red+1,"$\sin(x)$"); |
xaxis("$x$",Arrow); | xaxis("$x$",Arrow); | ||
yaxis(); | yaxis(); | ||
| Line 26: | Line 26: | ||
xtick("$-2\pi$",-2*pi,N); | xtick("$-2\pi$",-2*pi,N); | ||
| − | ytick("$1/2$",0.5,1); | + | ytick("$1/2$",0.5,1,fontsize(8pt)); |
| − | ytick("$\sqrt{2}/2$",sqrt(2)/2,1); | + | ytick("$\sqrt{2}/2$",sqrt(2)/2,1,fontsize(8pt)); |
| − | ytick("$\sqrt{3}/2$",sqrt(3)/2,1); | + | ytick("$\sqrt{3}/2$",sqrt(3)/2,1,fontsize(8pt)); |
| − | ytick("$1$",1,1); | + | ytick("$1$",1,1,fontsize(8pt)); |
| − | ytick("$-1/2$",-0.5,-1); | + | ytick("$-1/2$",-0.5,-1,fontsize(8pt)); |
| − | ytick("$-\sqrt{2}/2$",-sqrt(2)/2,-1); | + | ytick("$-\sqrt{2}/2$",-sqrt(2)/2,-1,fontsize(8pt)); |
| − | ytick("$-\sqrt{3}/2$",-sqrt(3)/2,-1); | + | ytick("$-\sqrt{3}/2$",-sqrt(3)/2,-1,fontsize(8pt)); |
| − | ytick("$-1$",-1,-1); | + | ytick("$-1$",-1,-1,fontsize(8pt)); |
attach(legend(),truepoint(E),10E,UnFill); | attach(legend(),truepoint(E),10E,UnFill); | ||
| − | </asy> | + | </asy></center> |
The graph of $y=\cos x = \sin(x+\pi/2)$ is the cosinusoid, obtained by shifting the sinusoid a distance of $\pi/2$ to the left. The cosinusoid intersects the $x$-axis at the points $((k+1/2)\pi,0)$, and its extrema are at the points $(k\pi, (-1)^k)$. | The graph of $y=\cos x = \sin(x+\pi/2)$ is the cosinusoid, obtained by shifting the sinusoid a distance of $\pi/2$ to the left. The cosinusoid intersects the $x$-axis at the points $((k+1/2)\pi,0)$, and its extrema are at the points $(k\pi, (-1)^k)$. | ||
Revision as of 16:25, 5 December 2014
The graph of the function $y=\sin x$ (see Fig.). The sinusoid is a continuous curve with period $T=2\pi$. It intersects the $x$-axis at the points $(k\pi, 0)$. These are also points of inflection, meeting the $x$-axis at an angle of $\pm\pi/4$. The extrema are at the points $((k+1/2)\pi, (-1)^k)$.
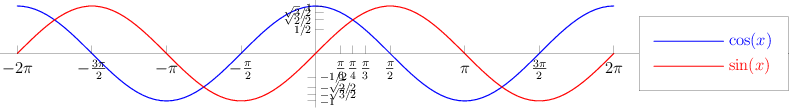
The graph of $y=\cos x = \sin(x+\pi/2)$ is the cosinusoid, obtained by shifting the sinusoid a distance of $\pi/2$ to the left. The cosinusoid intersects the $x$-axis at the points $((k+1/2)\pi,0)$, and its extrema are at the points $(k\pi, (-1)^k)$.
Many oscillatory processes can be described by a periodic function of the form y=a\sin(bx+c), where $a$, $b$ and $c$ are constants and $b>0$. The graph of this function (called a general sinusoid) is obtained from the graph of $y=\sin x$ (the ordinary sinusoid) as follows: expand in the direction of the $y$-axis by a factor $|a|$, contract in the $x$-direction by a factor $b$, translate to the left over a distance $c/b$, and when $a<0$: reflect in the $x$-axis. Its period is $T=2\pi/b$ and it meets the $x$-axis at the points $((k\pi-c)/b,0)$. Its extrema are at the points $(((k+1/2)\pi-c)/b,(-1)^ka)$.
In this article, $k\in \mathbb Z$.
See also Sine; Trigonometric functions.
Sinusoid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Sinusoid&oldid=29097