Difference between revisions of "Discrete series (of representations)"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | d0331201.png | ||
| + | $#A+1 = 37 n = 2 | ||
| + | $#C+1 = 37 : ~/encyclopedia/old_files/data/D033/D.0303120 Discrete series (of representations) | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | + | The family of continuous irreducible unitary representations of a locally compact group $ G $ | |
| + | which are equivalent to the subrepresentations of the regular representation of this group. If the group $ G $ | ||
| + | is unimodular, then a continuous irreducible unitary representation $ \pi $ | ||
| + | of $ G $ | ||
| + | belongs to the discrete series if and only if the matrix entries of $ \pi $ | ||
| + | lie in $ L _ {2} ( G) $. | ||
| + | In such a case there exists a positive number $ d _ \pi $, | ||
| + | known as the formal degree of the representation $ \pi $, | ||
| + | such that the relations | ||
| − | + | $$ \tag{1 } | |
| + | \int\limits _ { G } ( \pi ( g) \xi , \eta ) ( {\pi ( g) \xi ^ \prime , \eta ^ \prime | ||
| + | } bar ) dg = d _ \pi ^ {-} 1 ( \xi , \xi ^ \prime ) ( {\eta , \eta | ||
| + | ^ \prime } bar ); | ||
| + | $$ | ||
| − | + | $$ \tag{2 } | |
| + | ( \pi ( g) \xi , \eta ) * ( \pi ( g) \xi ^ \prime , \eta ^ \prime ) = d _ \pi ^ {-} 1 ( \xi , \eta ^ \prime ) ( \pi ( g) \xi ^ \prime , \eta ) , | ||
| + | $$ | ||
| − | + | are satisfied for all vectors $ \xi , \eta , \xi ^ \prime , \eta ^ \prime $ | |
| + | of the space $ H _ \pi $ | ||
| + | of the representation $ \pi $. | ||
| + | If $ \pi _ {1} $ | ||
| + | and $ \pi _ {2} $ | ||
| + | are two non-equivalent representations of $ G $ | ||
| + | in the spaces $ H _ {1} $ | ||
| + | and $ H _ {2} $, | ||
| + | respectively, which belong to the discrete series, then the relations | ||
| − | + | $$ \tag{3 } | |
| + | \int\limits _ { G } ( \pi _ {1} ( g) \xi _ {1} \eta _ {1} ) ( {\pi _ {2} ( g) | ||
| + | \xi _ {2} \eta _ {2} } bar ) dg = 0 , | ||
| + | $$ | ||
| − | The equivalence class of a representation | + | $$ \tag{4 } |
| + | ( \pi _ {1} ( g) \xi _ {1} , \eta _ {1} ) * ( \pi _ {2} ( g) \xi _ {2} , \eta _ {2} ) = 0 , | ||
| + | $$ | ||
| + | |||
| + | are valid for all $ \xi _ {1} , \eta _ {1} \in H _ {1} $, | ||
| + | $ \xi _ {2} , \eta _ {2} \in H _ {2} $. | ||
| + | The relations (1)–(4) are generalizations of the orthogonality relations for the matrix entries of representations of compact topological groups (cf. [[Representation of a compact group|Representation of a compact group]]); the group $ G $ | ||
| + | is compact if and only if all continuous irreducible unitary representations of $ G $ | ||
| + | belong to the discrete series, and if $ G $ | ||
| + | is compact and the Haar measure $ dg $ | ||
| + | satisfies the condition $ \int _ {G} dg = 1 $, | ||
| + | then the number $ d _ \pi $ | ||
| + | coincides with the dimension of the representation $ \pi $. | ||
| + | Simply-connected nilpotent real Lie groups and complex semi-simple Lie groups have no discrete series. | ||
| + | |||
| + | The equivalence class of a representation $ \pi $ | ||
| + | forming part of the discrete series is a closed point in the dual space $ \widehat{G} $ | ||
| + | of the group $ G $, | ||
| + | and the Plancherel measure of this point coincides with the formal degree $ d _ \pi $; | ||
| + | if, in addition, some non-zero matrix entry of the representation $ \pi $ | ||
| + | is summable, the representation $ \pi $ | ||
| + | is an open point in the support of the regular representation of $ G $, | ||
| + | but open points in $ \widehat{G} $ | ||
| + | need not correspond to representations of the discrete series. The properties of discrete series representations may be partly extended to the case of non-unimodular locally compact groups. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d033/d033120/d03312038.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR><TR><TD valign="top">[2a]</TD> <TD valign="top"> Harish-Chandra, "Discrete series for semisimple Lie groups I" ''Acta Math.'' , '''113''' (1965) pp. 241–318</TD></TR><TR><TD valign="top">[2b]</TD> <TD valign="top"> Harish-Chandra, "Discrete series for semisimple Lie groups II" ''Acta Math.'' , '''116''' (1966) pp. 1–111</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> W. Schmid, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d033/d033120/d03312039.png" />-cohomology and the discrete series" ''Ann. of Math.'' , '''103''' (1976) pp. 375–394</TD></TR><TR><TD valign="top">[4a]</TD> <TD valign="top"> A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions" ''Ann. Sci. Ecole Norm. Sup.'' , '''5''' (1972) pp. 459–516</TD></TR><TR><TD valign="top">[4b]</TD> <TD valign="top"> A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions II" ''Ann. Sci. Ecole Norm. Sup.'' , '''6''' (1973) pp. 103–132</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d033/d033120/d03312038.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR><TR><TD valign="top">[2a]</TD> <TD valign="top"> Harish-Chandra, "Discrete series for semisimple Lie groups I" ''Acta Math.'' , '''113''' (1965) pp. 241–318</TD></TR><TR><TD valign="top">[2b]</TD> <TD valign="top"> Harish-Chandra, "Discrete series for semisimple Lie groups II" ''Acta Math.'' , '''116''' (1966) pp. 1–111</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> W. Schmid, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d033/d033120/d03312039.png" />-cohomology and the discrete series" ''Ann. of Math.'' , '''103''' (1976) pp. 375–394</TD></TR><TR><TD valign="top">[4a]</TD> <TD valign="top"> A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions" ''Ann. Sci. Ecole Norm. Sup.'' , '''5''' (1972) pp. 459–516</TD></TR><TR><TD valign="top">[4b]</TD> <TD valign="top"> A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions II" ''Ann. Sci. Ecole Norm. Sup.'' , '''6''' (1973) pp. 103–132</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
Revision as of 19:36, 5 June 2020
The family of continuous irreducible unitary representations of a locally compact group $ G $
which are equivalent to the subrepresentations of the regular representation of this group. If the group $ G $
is unimodular, then a continuous irreducible unitary representation $ \pi $
of $ G $
belongs to the discrete series if and only if the matrix entries of $ \pi $
lie in $ L _ {2} ( G) $.
In such a case there exists a positive number $ d _ \pi $,
known as the formal degree of the representation $ \pi $,
such that the relations
$$ \tag{1 } \int\limits _ { G } ( \pi ( g) \xi , \eta ) ( {\pi ( g) \xi ^ \prime , \eta ^ \prime } bar ) dg = d _ \pi ^ {-} 1 ( \xi , \xi ^ \prime ) ( {\eta , \eta ^ \prime } bar ); $$
$$ \tag{2 } ( \pi ( g) \xi , \eta ) * ( \pi ( g) \xi ^ \prime , \eta ^ \prime ) = d _ \pi ^ {-} 1 ( \xi , \eta ^ \prime ) ( \pi ( g) \xi ^ \prime , \eta ) , $$
are satisfied for all vectors $ \xi , \eta , \xi ^ \prime , \eta ^ \prime $ of the space $ H _ \pi $ of the representation $ \pi $. If $ \pi _ {1} $ and $ \pi _ {2} $ are two non-equivalent representations of $ G $ in the spaces $ H _ {1} $ and $ H _ {2} $, respectively, which belong to the discrete series, then the relations
$$ \tag{3 } \int\limits _ { G } ( \pi _ {1} ( g) \xi _ {1} \eta _ {1} ) ( {\pi _ {2} ( g) \xi _ {2} \eta _ {2} } bar ) dg = 0 , $$
$$ \tag{4 } ( \pi _ {1} ( g) \xi _ {1} , \eta _ {1} ) * ( \pi _ {2} ( g) \xi _ {2} , \eta _ {2} ) = 0 , $$
are valid for all $ \xi _ {1} , \eta _ {1} \in H _ {1} $, $ \xi _ {2} , \eta _ {2} \in H _ {2} $. The relations (1)–(4) are generalizations of the orthogonality relations for the matrix entries of representations of compact topological groups (cf. Representation of a compact group); the group $ G $ is compact if and only if all continuous irreducible unitary representations of $ G $ belong to the discrete series, and if $ G $ is compact and the Haar measure $ dg $ satisfies the condition $ \int _ {G} dg = 1 $, then the number $ d _ \pi $ coincides with the dimension of the representation $ \pi $. Simply-connected nilpotent real Lie groups and complex semi-simple Lie groups have no discrete series.
The equivalence class of a representation $ \pi $ forming part of the discrete series is a closed point in the dual space $ \widehat{G} $ of the group $ G $, and the Plancherel measure of this point coincides with the formal degree $ d _ \pi $; if, in addition, some non-zero matrix entry of the representation $ \pi $ is summable, the representation $ \pi $ is an open point in the support of the regular representation of $ G $, but open points in $ \widehat{G} $ need not correspond to representations of the discrete series. The properties of discrete series representations may be partly extended to the case of non-unimodular locally compact groups.
References
| [1] | J. Dixmier, "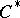 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
| [2a] | Harish-Chandra, "Discrete series for semisimple Lie groups I" Acta Math. , 113 (1965) pp. 241–318 |
| [2b] | Harish-Chandra, "Discrete series for semisimple Lie groups II" Acta Math. , 116 (1966) pp. 1–111 |
| [3] | W. Schmid, "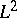 -cohomology and the discrete series" Ann. of Math. , 103 (1976) pp. 375–394 -cohomology and the discrete series" Ann. of Math. , 103 (1976) pp. 375–394 |
| [4a] | A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions" Ann. Sci. Ecole Norm. Sup. , 5 (1972) pp. 459–516 |
| [4b] | A. Kleppner, R. Lipsman, "The Plancherel formula for group extensions II" Ann. Sci. Ecole Norm. Sup. , 6 (1973) pp. 103–132 |
Comments
Especially for a semi-simple Lie group the representations belonging to the discrete series of the group or of some of its subgroups play an essential role in the harmonic analysis on the group.
References
| [a1] | V.S. Varadarajan, "Harmonic analysis on real reductive groups" , Springer (1977) |
Discrete series (of representations). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Discrete_series_(of_representations)&oldid=13646