Difference between revisions of "Brauer first main theorem"
m (Automatically changed introduction) |
m (Automatically changed introduction) |
||
| Line 6: | Line 6: | ||
Out of 125 formulas, 122 were replaced by TEX code.--> | Out of 125 formulas, 122 were replaced by TEX code.--> | ||
| − | {{TEX|semi-auto}}{{TEX| | + | {{TEX|semi-auto}}{{TEX|part}} |
The $p$-local structure of a [[Finite group|finite group]] $G$, where $p$ is a [[Prime number|prime number]], is the collection of non-trivial $p$-subgroups of $G$ (cf. also [[P-group|$p$-group]]), together with their normalizers and centralizers (cf. also [[Centralizer|Centralizer]]; [[Normalizer of a subset|Normalizer of a subset]]). One of the principal goals of representation theory (cf. also [[Representation of a group|Representation of a group]]) is to find rules relating representations and characters to the various $p$-local structures of $G$. If a prime number $p$ is fixed, representations over fields (cf. also [[Field|Field]]) of characteristic $p$, and values of irreducible complex characters on elements of order divisible by $p$, are controlled to a considerable extent by the $p$-local structure. Brauer's three main theorems on blocks are the oldest and most important tools for investigating this phenomenon. (See also [[Brauer second main theorem|Brauer second main theorem]]; [[Brauer third main theorem|Brauer third main theorem]].) | The $p$-local structure of a [[Finite group|finite group]] $G$, where $p$ is a [[Prime number|prime number]], is the collection of non-trivial $p$-subgroups of $G$ (cf. also [[P-group|$p$-group]]), together with their normalizers and centralizers (cf. also [[Centralizer|Centralizer]]; [[Normalizer of a subset|Normalizer of a subset]]). One of the principal goals of representation theory (cf. also [[Representation of a group|Representation of a group]]) is to find rules relating representations and characters to the various $p$-local structures of $G$. If a prime number $p$ is fixed, representations over fields (cf. also [[Field|Field]]) of characteristic $p$, and values of irreducible complex characters on elements of order divisible by $p$, are controlled to a considerable extent by the $p$-local structure. Brauer's three main theorems on blocks are the oldest and most important tools for investigating this phenomenon. (See also [[Brauer second main theorem|Brauer second main theorem]]; [[Brauer third main theorem|Brauer third main theorem]].) | ||
Latest revision as of 17:46, 1 July 2020
The $p$-local structure of a finite group $G$, where $p$ is a prime number, is the collection of non-trivial $p$-subgroups of $G$ (cf. also $p$-group), together with their normalizers and centralizers (cf. also Centralizer; Normalizer of a subset). One of the principal goals of representation theory (cf. also Representation of a group) is to find rules relating representations and characters to the various $p$-local structures of $G$. If a prime number $p$ is fixed, representations over fields (cf. also Field) of characteristic $p$, and values of irreducible complex characters on elements of order divisible by $p$, are controlled to a considerable extent by the $p$-local structure. Brauer's three main theorems on blocks are the oldest and most important tools for investigating this phenomenon. (See also Brauer second main theorem; Brauer third main theorem.)
The first step is the partition of indecomposable representations and irreducible characters into blocks. Fix a prime number $p$. Let $R$ be a complete discrete valuation ring of characteristic $0$ with $p$ in its radical $J ( R )$ (cf. also Radical of rings and algebras), let $K$ be its field of fractions, and let $k$ be the residue field $R / J ( R )$. Assume, in addition, that $K$ contains an $e$th root of $1$, where $e$ is the least common multiple of the orders of the elements of $G$. The group algebra $R G$ has a unique decomposition as a direct sum of indecomposable two-sided ideals,
\begin{equation*} R G = B _ { 1 } \bigoplus \ldots \bigoplus B _ { n }. \end{equation*}
The ideals $B _ { i }$ are called the blocks of $R G$. Applying to this decomposition the natural mapping $R G \rightarrow k G$ (i.e. reducing coefficients modulo $J ( R )$), one obtains the unique decomposition of $k G$ as a direct sum of indecomposable two-sided ideals. Any indecomposable $R$-free $R G$-module, or any indecomposable $k G$-module, is annihilated by all blocks except one, and if 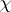 is an irreducible $K$-character of $G$, then $\chi ( B _ { i } ) = 0$ for all but one $i$. Thus, to each block belong a category of $R$-free $R G$-modules, a category of $k G$-modules, and a set of irreducible $K$-characters. The word "block" is sometimes used to refer to the corresponding categories or the corresponding set of characters, rather than to the ideal.
is an irreducible $K$-character of $G$, then $\chi ( B _ { i } ) = 0$ for all but one $i$. Thus, to each block belong a category of $R$-free $R G$-modules, a category of $k G$-modules, and a set of irreducible $K$-characters. The word "block" is sometimes used to refer to the corresponding categories or the corresponding set of characters, rather than to the ideal.
Let $L$ be the subfield of $K$ consisting of all elements that are algebraic over the prime subfield (cf. also Algebraic number). The values on elements of $G$ of any irreducible $K$-character are in $L$. If an imbedding of $L$ in the complex numbers is chosen, the irreducible $K$-characters of $G$ can be identified with the irreducible complex characters of $G$. Thus, the irreducible complex characters are partitioned into blocks. This partition is independent of the choice of the imbedding. When it is not clear from the context which prime number $p$ is intended, one refers to a partition of the irreducible complex characters into $p$-blocks.
To each block is associated a $G$-conjugacy class of $p$-subgroups of $G$, called its defect groups (cf. also Defect group of a block), which substantially control its representation theory. They are defined as follows. Fix a block $B$. For any subgroup $H$ of $G$, let
\begin{equation*} B ^ { H } = \{ a \in B : h ^ { - 1 } a h =a \ \text {for all } h \in H \}. \end{equation*}
Let $T _ { H } ^ { G } : B ^ { H } \rightarrow B ^ { G }$ be given by $T _ { H } ^ { G } ( a ) = \sum _ { j } g _ { j } ^ { - 1 } a g_j$, where $\{ g_j\}$ is a set of representatives for the right cosets of $H$ in $G$. The set of subgroups $H$ minimal such that $B ^ { G } = T _ { H } ^ { G } ( B ^ { H } )$ is a $G$-conjugacy class of $p$-subgroups; they are called the defect groups of $B$. If a block has defect group $1$, then it is isomorphic as an $R$-algebra to a full matrix algebra with entries in $R$, and it is associated to just a single irreducible character, a single indecomposable $R$-free $R G$-module, and a single indecomposable $k G$-module. Blocks with cyclic defect group are very well understood, due to work of E.C. Dade (see [a5], Chap. VII). The process of understanding blocks with more complicated defect groups is still far from complete (as of 1998). For example, R. Brauer has conjectured that the number of irreducible characters belonging to a block with defect group $D$ is less than or equal to the order of $D$. See also Brauer height-zero conjecture.
The Brauer correspondence is a tool for relating blocks of $G$ to blocks of subgroups of $G$; it is defined as follows. The algebra $R G$ is a right module over 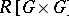 , with $a ( g h ) = g ^ { - 1 } a h$ for all $g \in G$, $h \in G$, and $a \in R G$. The decomposition of $R G$ into blocks is also a decomposition of $R G$ as a direct sum of indecomposable
, with $a ( g h ) = g ^ { - 1 } a h$ for all $g \in G$, $h \in G$, and $a \in R G$. The decomposition of $R G$ into blocks is also a decomposition of $R G$ as a direct sum of indecomposable 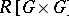 -modules. Let $H$ be a subgroup of $G$ and let $b$ be a block of $R H$. If there is a unique block $B$ of $G$ with $b$ isomorphic as an $R [ H \times H]$-module to a direct summand of the restriction $B _ { R [ H \times H ]}$, then one says that $b ^ { G }$ is defined and $B = b ^ { G }$. The mapping $b \mapsto b ^ { G }$ is called the Brauer correspondence. Let $D$ be a defect group of $b$. Assume that $C _ { G } ( D ) \subseteq H$; then in any decomposition of $R G$ as a direct sum of indecomposable $R [ H \times H]$-modules there is a unique module isomorphic to $b$; so certainly $b ^ { G }$ is defined. Most of the important applications of the Brauer correspondence arise in this way.
-modules. Let $H$ be a subgroup of $G$ and let $b$ be a block of $R H$. If there is a unique block $B$ of $G$ with $b$ isomorphic as an $R [ H \times H]$-module to a direct summand of the restriction $B _ { R [ H \times H ]}$, then one says that $b ^ { G }$ is defined and $B = b ^ { G }$. The mapping $b \mapsto b ^ { G }$ is called the Brauer correspondence. Let $D$ be a defect group of $b$. Assume that $C _ { G } ( D ) \subseteq H$; then in any decomposition of $R G$ as a direct sum of indecomposable $R [ H \times H]$-modules there is a unique module isomorphic to $b$; so certainly $b ^ { G }$ is defined. Most of the important applications of the Brauer correspondence arise in this way.
Brauer's original definition of this correspondence in terms of central characters (see [a5], Chap. III) yields a mapping that has a slightly different domain of definition from the mapping described here. The mappings agree on the intersection of their domains, and both are defined in the important case when $C _ { G } ( D ) \subseteq H$.
Brauer's first main theorem says that if $D$ is a $p$-subgroup of $G$ and $H$ is a subgroup of $G$ with $N _ { G } ( D ) \subseteq H$, then the Brauer correspondence gives a bijection between the blocks of $R G$ with defect group $D$ and the blocks of $R H$ with defect group $D$. Thus, the number of blocks with non-trivial defect group is $p$-locally determined.
There is a close relationship between a block of $R G$ with defect group $D$ and the corresponding block of $RN_G(D)$; however, the investigation of this relationship is far from complete. See, for example, McKay–Alperin conjecture, the Broué conjecture in [a3], or the many consequences of the Alperin weight conjecture in [a2].
General references in this subject are [a1], [a4], [a5], and [a6].
References
| [a1] | J.L. Alperin, "Local representation theory" , Cambridge Univ. Press (1986) |
| [a2] | J.L. Alperin, "Weights for finite groups" P. Fong (ed.) , Representations of Finite Groups , Proc. Symp. Pure Math. , 47 , Amer. Math. Soc. (1987) pp. 369–379 |
| [a3] | M. Broué, "Isométries parfaites, types de blocs, catégories dérivées" Astérisque , 181–182 (1990) pp. 61–92 |
| [a4] | C. Curtis, I. Reiner, "Methods of representation theory" , II , Wiley (1987) |
| [a5] | W. Feit, "The representation theory of finite groups" , North-Holland (1982) |
| [a6] | H. Nagao, Y. Tsushima, "Representation of finite groups" , Acad. Press (1987) |
Brauer first main theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Brauer_first_main_theorem&oldid=50548