Difference between revisions of "Weyl quantization"
m (AUTOMATIC EDIT (latexlist): Replaced 257 formulas out of 284 by TEX code with an average confidence of 2.0 and a minimal confidence of 2.0.) |
(latex details) |
||
| (2 intermediate revisions by one other user not shown) | |||
| Line 2: | Line 2: | ||
the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist | ||
was used. | was used. | ||
| − | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. | + | If the TeX and formula formatting is correct and if all png images have been replaced by TeX code, please remove this message and the {{TEX|semi-auto}} category. |
Out of 284 formulas, 257 were replaced by TEX code.--> | Out of 284 formulas, 257 were replaced by TEX code.--> | ||
| − | {{TEX|semi-auto}}{{TEX| | + | {{TEX|semi-auto}}{{TEX|part}} |
Let be a classical Hamiltonian (cf. also [[Hamilton operator|Hamilton operator]]) defined on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. The Weyl quantization rule associates to this function the operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w1201103.png"/> defined on functions u ( x ) as | Let a ( x , \xi ) be a classical Hamiltonian (cf. also [[Hamilton operator|Hamilton operator]]) defined on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. The Weyl quantization rule associates to this function the operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w1201103.png"/> defined on functions u ( x ) as | ||
| Line 68: | Line 68: | ||
\begin{equation*} \alpha ^ { w } = \int _ { \mathbf{R} ^ { 2 n } } a ( X ) 2 ^ { n } \sigma _ { X } d X = \end{equation*} | \begin{equation*} \alpha ^ { w } = \int _ { \mathbf{R} ^ { 2 n } } a ( X ) 2 ^ { n } \sigma _ { X } d X = \end{equation*} | ||
| − | \begin{equation*} = \int _ { \mathbf{R} ^ { 2 n } } \hat { a } ( \Xi ) \operatorname { exp } ( 2 i \pi \Xi . M ) d \Xi | + | \begin{equation*} = \int _ { \mathbf{R} ^ { 2 n } } \hat { a } ( \Xi ) \operatorname { exp } ( 2 i \pi \Xi . M ) d \Xi \end{equation*} |
where \Xi \cdot M = \hat{x} \cdot x + \widehat { \xi } \cdot D _{x} (here \Xi = ( \hat { x } , \hat { \xi } )). These formulas give, in particular, | where \Xi \cdot M = \hat{x} \cdot x + \widehat { \xi } \cdot D _{x} (here \Xi = ( \hat { x } , \hat { \xi } )). These formulas give, in particular, | ||
| Line 76: | Line 76: | ||
where \mathcal{L} ( L ^ { 2 } ) stands for the space of bounded linear mappings from L ^ { 2 } ( \mathbf{R} ^ { n } ) into itself. The operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w12011051.png"/> is in the Hilbert–Schmidt class (cf. also [[Hilbert–Schmidt operator|Hilbert–Schmidt operator]]) if and only if a belongs to L ^ { 2 } ( \mathbf{R} ^ { 2 n } ) and \| a\| _{\text{HS}} = \| a \| _ { L } 2 _ { ( \mathbf{R} ^ { 2 n }) } . To get this, it suffices to notice the relationship between the symbol a of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w12011056.png"/> and its distribution kernel k: | where \mathcal{L} ( L ^ { 2 } ) stands for the space of bounded linear mappings from L ^ { 2 } ( \mathbf{R} ^ { n } ) into itself. The operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w12011051.png"/> is in the Hilbert–Schmidt class (cf. also [[Hilbert–Schmidt operator|Hilbert–Schmidt operator]]) if and only if a belongs to L ^ { 2 } ( \mathbf{R} ^ { 2 n } ) and \| a\| _{\text{HS}} = \| a \| _ { L } 2 _ { ( \mathbf{R} ^ { 2 n }) } . To get this, it suffices to notice the relationship between the symbol a of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w12011056.png"/> and its distribution kernel k: | ||
| − | \begin{equation*} | + | \begin{equation*} a ( x , \xi ) = \int k \left( x + \frac { t } { 2 } , x - \frac { t } { 2 } \right) e ^ { - 2 i \pi t \xi } d t. \end{equation*} |
The Fourier transform of the Wigner function is the so-called ambiguity function | The Fourier transform of the Wigner function is the so-called ambiguity function | ||
| Line 149: | Line 149: | ||
with an integral on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. It is convenient to give an asymptotic version of these compositions formulas, e.g. in the semi-classical case. Let m be a real number. A smooth function a ( x , \xi , h ) defined on \mathbf{R} _ { x } ^ { n } \times \mathbf{R} _ { \xi } ^ { n } \times ( 0,1 ] is in the symbol class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110133.png"/> if | with an integral on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. It is convenient to give an asymptotic version of these compositions formulas, e.g. in the semi-classical case. Let m be a real number. A smooth function a ( x , \xi , h ) defined on \mathbf{R} _ { x } ^ { n } \times \mathbf{R} _ { \xi } ^ { n } \times ( 0,1 ] is in the symbol class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110133.png"/> if | ||
| − | \begin{equation*} \operatorname { sup } _ {\substack{ ( x , \xi ) \in {\bf R} ^ { 2 n }}, \\{0 | + | \begin{equation*} \operatorname { sup } _ {\substack{ ( x , \xi ) \in {\bf R} ^ { 2 n }}, \\{0<h\leq 1} } \left| D _ { x } ^ { \alpha } D _ { \xi } ^ { \beta } a ( x , \xi , h ) \right| h ^ { m - | \beta | } < \infty . \end{equation*} |
Then one has for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110135.png"/> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110136.png"/> the expansion | Then one has for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110135.png"/> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110136.png"/> the expansion | ||
| Line 155: | Line 155: | ||
\begin{equation} \tag{a11} ( a \sharp b ) ( x , \xi ) = r _ { N } ( a , b ) + \end{equation} | \begin{equation} \tag{a11} ( a \sharp b ) ( x , \xi ) = r _ { N } ( a , b ) + \end{equation} | ||
| − | \begin{equation*} + \sum _ { 0 \leq k | + | \begin{equation*} + \sum _ { 0 \leq k < N } 2 ^ { - k } \sum _ { | \alpha | + | \beta | = k } \frac { ( - 1 ) ^ { | \beta | } } { \alpha ! \beta ! } D _ { \xi } ^ { \alpha } \partial _ { x } ^ { \beta } a D _ { \xi } ^ { \beta } \partial _ { x } ^ { \alpha } b, \end{equation*} |
with r _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N}. The beginning of this expansion is thus | with r _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N}. The beginning of this expansion is thus | ||
| Line 163: | Line 163: | ||
where \{ a , b \} denotes the [[Poisson brackets|Poisson brackets]] and \iota = 2 \pi {i} . The sums inside (a11) with k even are symmetric in a , b and skew-symmetric for k odd. This can be compared to the classical expansion formula | where \{ a , b \} denotes the [[Poisson brackets|Poisson brackets]] and \iota = 2 \pi {i} . The sums inside (a11) with k even are symmetric in a , b and skew-symmetric for k odd. This can be compared to the classical expansion formula | ||
| − | \begin{equation*} ( a \circ b ) ( x , \xi ) = \sum _ { | \alpha | | + | \begin{equation*} ( a \circ b ) ( x , \xi ) = \sum _ { | \alpha | < N } \frac { 1 } { \alpha ! } D _ { \xi } ^ { \alpha } a \partial _ { x } ^ { \alpha } b + t _ { N } ( a , b ), \end{equation*} |
with t _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N }. Moreover, for a _ { 1 } , \dots , a _ { 2k } in L ^ { 1 } ( \Phi = \mathbf{R} ^ { 2 n } ), the multiple composition formula gives | with t _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N }. Moreover, for a _ { 1 } , \dots , a _ { 2k } in L ^ { 1 } ( \Phi = \mathbf{R} ^ { 2 n } ), the multiple composition formula gives | ||
| Line 177: | Line 177: | ||
<table class="eq" style="width:100%;"> <tr><td style="width:94%;text-align:center;" valign="top"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110154.png"/></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td style="width:94%;text-align:center;" valign="top"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/w/w120/w120110/w120110154.png"/></td> </tr></table> | ||
| − | \begin{equation*} = 2 ^ { 2 n k } \int _ { \Phi ^ { 2 k } } a _ { 1 } ( Y _ { 1 } ) \ldots a _ { 2 k } ( Y _ { 2 k } ) \text{..} a _ { 2 k + 1 } \left( X + \sum _ { 1 \leq j | + | \begin{equation*} = 2 ^ { 2 n k } \int _ { \Phi ^ { 2 k } } a _ { 1 } ( Y _ { 1 } ) \ldots a _ { 2 k } ( Y _ { 2 k } ) \text{..} a _ { 2 k + 1 } \left( X + \sum _ { 1 \leq j < l \leq 2 k } ( - 1 ) ^ { j + l } ( Y _ { j } - Y _ { l } ) \right). \end{equation*} |
| − | \begin{equation*} .\operatorname { exp } 4 i \pi \sum _ { 1 \leq j | + | \begin{equation*} .\operatorname { exp } 4 i \pi \sum _ { 1 \leq j < l \leq 2 k } ( - 1 ) ^ { j + l } [ X - Y _ { j } , X - Y _ { l } ] .. d Y _ { 1 } \ldots d Y _ { 2 k }. \end{equation*} |
Consider the standard sum of homogeneous symbols defined on \Omega \times \mathbf{R} ^ { n }, where \Omega is an open subset of {\bf R} ^ { n }, | Consider the standard sum of homogeneous symbols defined on \Omega \times \mathbf{R} ^ { n }, where \Omega is an open subset of {\bf R} ^ { n }, | ||
| Line 191: | Line 191: | ||
and r _ { m - 2} \in S _ { \text{loc} } ^ { m - 2 } ( \Omega ), i.e. for all compact subsets K of \Omega, | and r _ { m - 2} \in S _ { \text{loc} } ^ { m - 2 } ( \Omega ), i.e. for all compact subsets K of \Omega, | ||
| − | \begin{equation*} \operatorname { sup } _ { x \in K, \atop \xi \in {\bf R} ^ { n } } \left| ( D _ { x } ^ { \alpha } D _ { \xi } ^ { \beta } r _ { m - 2 } ) ( x , \xi ) \right| ( 1 + | \xi | ) ^ { 2 - m + | \beta | } | + | \begin{equation*} \operatorname { sup } _ { x \in K, \atop \xi \in {\bf R} ^ { n } } \left| ( D _ { x } ^ { \alpha } D _ { \xi } ^ { \beta } r _ { m - 2 } ) ( x , \xi ) \right| ( 1 + | \xi | ) ^ { 2 - m + | \beta | } < \infty. \end{equation*} |
This class of pseudo-differential operators (cf. also [[Pseudo-differential operator|Pseudo-differential operator]]) is invariant under diffeomorphisms, and using the Weyl quantization one gets that the principal symbol a _ { m } is invariantly defined on the cotangent bundle T ^ { * } ( \Omega ) whereas the subprincipal symbol a_{m - 1} is invariantly defined on the double characteristic set | This class of pseudo-differential operators (cf. also [[Pseudo-differential operator|Pseudo-differential operator]]) is invariant under diffeomorphisms, and using the Weyl quantization one gets that the principal symbol a _ { m } is invariantly defined on the cotangent bundle T ^ { * } ( \Omega ) whereas the subprincipal symbol a_{m - 1} is invariantly defined on the double characteristic set | ||
| Line 248: | Line 248: | ||
Eventually, one defines the class of symbols S ( m , G ) as the C ^ { \infty }-functions a on the phase space such that | Eventually, one defines the class of symbols S ( m , G ) as the C ^ { \infty }-functions a on the phase space such that | ||
| − | \begin{equation} \tag{a18} \operatorname { sup } _ { X \in \Phi } \| a ^ { ( k ) } ( X ) \| _ { G _ { X } } m ( X ) ^ { - 1 } | + | \begin{equation} \tag{a18} \operatorname { sup } _ { X \in \Phi } \| a ^ { ( k ) } ( X ) \| _ { G _ { X } } m ( X ) ^ { - 1 } < \infty. \end{equation} |
It is, for instance, easily checked that | It is, for instance, easily checked that | ||
| Line 254: | Line 254: | ||
\begin{equation*} S _ { \rho , \delta } ^ { \mu } = S \left( \langle \xi \rangle ^ { \mu } , \langle \xi \rangle ^ { 2 \delta } | d x | ^ { 2 } + \langle \xi \rangle ^ { - 2 \rho } | d \xi | ^ { 2 } \right), \end{equation*} | \begin{equation*} S _ { \rho , \delta } ^ { \mu } = S \left( \langle \xi \rangle ^ { \mu } , \langle \xi \rangle ^ { 2 \delta } | d x | ^ { 2 } + \langle \xi \rangle ^ { - 2 \rho } | d \xi | ^ { 2 } \right), \end{equation*} | ||
| − | with \langle \xi \rangle = 1 + | \xi | and that this metric is an admissible metric when 0 \leq \delta \leq \rho \leq 1, $\delta | + | with \langle \xi \rangle = 1 + | \xi | and that this metric is an admissible metric when 0 \leq \delta \leq \rho \leq 1, $\delta < 1. The metric defining S _ { 1,1 } ^ { 0 } satisfies (a13)–(a14) but fails to satisfy (a15). Indeed, there are counterexamples showing that for the classical and the Weyl quantization [[#References|[a4]]] there are symbols in S _ { 1,1 } ^ { 0 } whose quantization is not L^{2}-bounded. In fact, one of the building block for the calculus of pseudo-differential operators is the L^{2}-boundedness of the Weyl quantization of symbols in S ( 1 , G ), where G$ is an admissible metric. One defines the Planck function of the calculus as |
\begin{equation} \tag{a19} H ( X ) = \operatorname { sup } _ { T \neq 0 } \sqrt { \frac { G _{X} ( T ) } { G _ { X } ^ { \sigma } ( T ) } } \end{equation} | \begin{equation} \tag{a19} H ( X ) = \operatorname { sup } _ { T \neq 0 } \sqrt { \frac { G _{X} ( T ) } { G _ { X } ^ { \sigma } ( T ) } } \end{equation} | ||
| Line 292: | Line 292: | ||
\begin{equation*} g _ { 1 } = | d x | ^ { 2 } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } } \leq g_2 = \frac { | d x | ^ { 2 } } { | x | ^ { 2 } } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } }, \end{equation*} | \begin{equation*} g _ { 1 } = | d x | ^ { 2 } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } } \leq g_2 = \frac { | d x | ^ { 2 } } { | x | ^ { 2 } } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } }, \end{equation*} | ||
| − | where g_1 is defined on $| \xi | | + | where g_1 is defined on $| \xi | > 1, and g_{2}$ on |
| − | \begin{equation*} \{ | x | | + | \begin{equation*} \{ | x | < 1 , | x | | \xi | > 1 \} \end{equation*} |
It is then possible to quantize functions a ( x , \xi ) homogeneous of degree \mu in the variable x, and \nu in the variable \xi , so as to get composition formulas, Sobolev spaces, and the standard pseudo-differential apparatus allowing a commutator argument to work for propagation results. | It is then possible to quantize functions a ( x , \xi ) homogeneous of degree \mu in the variable x, and \nu in the variable \xi , so as to get composition formulas, Sobolev spaces, and the standard pseudo-differential apparatus allowing a commutator argument to work for propagation results. | ||
Latest revision as of 19:54, 6 February 2024
Let a ( x , \xi ) be a classical Hamiltonian (cf. also Hamilton operator) defined on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. The Weyl quantization rule associates to this function the operator  defined on functions u ( x ) as
defined on functions u ( x ) as
\begin{equation} \tag{a1} ( a ^ { w } u ) ( x ) = \end{equation}
\begin{equation*} = \int \int e ^ { 2 i \pi ( x - y ) . \xi } a \left( \frac { x + y } { 2 } , \xi \right) u ( y ) d y d \xi. \end{equation*}
For instance, ( x . \xi ) ^ { w } = ( x . D _ { x } + D _ { x } .x ) / 2, with
\begin{equation*} D _ { x } = \frac { 1 } { 2 i \pi } \frac { \partial } { \partial x }, \end{equation*}
whereas the classical quantization rule would map the Hamiltonian x \cdot \xi to the operator x . D _ { x }. A nice feature of the Weyl quantization rule, introduced in 1928 by H. Weyl [a12], is the fact that real Hamiltonians get quantized by (formally) self-adjoint operators. Recall that the classical quantization of the Hamiltonian a ( x , \xi ) is given by the operator \operatorname{Op} ( a ) acting on functions u ( x ) by
\begin{equation} \tag{a2} ( \operatorname{Op} ( a ) u ) ( x ) = \int e ^ { 2 i \pi x \cdot \xi } a ( x , \xi ) \hat { u } ( \xi ) d \xi, \end{equation}
where the Fourier transform \widehat{u} is defined by
\begin{equation} \tag{a3} \hat { u } ( \xi ) = \int e ^ { - 2 i \pi x . \xi } u ( x ) d x, \end{equation}
so that \check{\widehat { u }} = u, with \check{v} ( x ) = v ( - x ). In fact, introducing the one-parameter group J ^ { t } = \operatorname { exp } 2 i \pi t D _ { x } . D _ { \xi }, given by the integral formula
\begin{equation} \tag{a4} ( J ^ { t } a ) ( x , \xi ) = \end{equation}
\begin{equation*} = | t | ^ { - n } \int \int e ^ { - 2 i \pi t ^ { - 1 } y . \eta } { a ( x + y , \xi + \eta ) d y d \eta }, \end{equation*}
one sees that
\begin{equation*} ( \operatorname{Op} ( J ^ { t } a ) u ) ( x ) = \end{equation*}
\begin{equation*} = \int \int e ^ { 2 i \pi ( x - y ) . \xi } a ( ( 1 - t ) x + t y , \xi ) u ( y ) d y d \xi. \end{equation*}
In particular, one gets a ^ { w } = \text{Op} ( J ^ { 1 / 2 } a ). Moreover, since ( \operatorname{Op} ( a ) ) ^ { * } = \operatorname{Op} ( J \overline { a } ) one obtains
\begin{equation*} ( a ^ { w } ) ^ { * } = \operatorname { Op } \left( J ( \overline { ( J ^ { 1 / 2 } a ) } \right) = \operatorname { Op } ( J ^ { 1 / 2 } \overline { a } ) = ( \overline { a } ) ^ { w }, \end{equation*}
yielding formal self-adjointness for real a (cf. also Self-adjoint operator).
Wigner functions.
Formula (a1) can be written as
\begin{equation} \tag{a5} ( a ^ { w } u , v ) = \int \int a ( x , \xi ) {\cal H} ( u , v ) ( x , \xi ) d x d \xi, \end{equation}
where the Wigner function \mathcal{H} is defined as
\begin{equation} \tag{a6} \mathcal{H} ( u , v ) ( x , \xi ) = \end{equation}
\begin{equation*} = \int u \left( x + \frac { y } { 2 } \right) \overline{v} \left( x - \frac { y } { 2 } \right) e ^ { - 2 i \pi y \cdot \xi } d y. \end{equation*}
The mapping ( u , v ) \mapsto \mathcal{H} ( u , v ) is sesquilinear continuous from \mathcal{S} ( \mathbf{R} ^ { n } ) \times \mathcal{S} ( \mathbf{R} ^ { n } ) to \mathcal{S} ( \mathbf{R} ^ { 2 n } ), so that 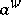 makes sense for a \in {\cal S} ^ { \prime } ( {\bf R} ^ { 2 n } ) (here, u , v \in \mathcal{S} ( \mathbf{R} ^ { n } ) and \mathcal{S} ^ { * } stands for the anti-dual):
makes sense for a \in {\cal S} ^ { \prime } ( {\bf R} ^ { 2 n } ) (here, u , v \in \mathcal{S} ( \mathbf{R} ^ { n } ) and \mathcal{S} ^ { * } stands for the anti-dual):
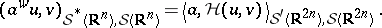 |
The Wigner function also satisfies
\begin{equation*} \| \mathcal{H} ( u , v ) \| _ { L ^ { 2 } ( \mathbf{R} ^{n}) } = \| u \|_ { L ^ { 2 } ( \mathbf{R} ^{n}) } \| v \| _ { L ^ { 2 } ( \mathbf{R} ^{n}) } , \end{equation*}
\begin{equation*} \mathcal{H} ( u , v ) ( x , \xi ) = 2 ^ { n } \langle \sigma _ { x , \xi }u , v \rangle _ { L^2 ( \mathbf{R} ^ { n } )} , ( \sigma _ { x , \xi} u ) ( y ) = u ( 2 x - y ) \operatorname { exp } ( - 4 i \pi ( x - y ) . \xi). \end{equation*}
and the phase symmetries \sigma _{X} are unitary and self-adjoint operators on L ^ { 2 } ( \mathbf{R} ^ { n } ). Also ([a10], [a12]),
\begin{equation*} \alpha ^ { w } = \int _ { \mathbf{R} ^ { 2 n } } a ( X ) 2 ^ { n } \sigma _ { X } d X = \end{equation*}
\begin{equation*} = \int _ { \mathbf{R} ^ { 2 n } } \hat { a } ( \Xi ) \operatorname { exp } ( 2 i \pi \Xi . M ) d \Xi \end{equation*}
where \Xi \cdot M = \hat{x} \cdot x + \widehat { \xi } \cdot D _{x} (here \Xi = ( \hat { x } , \hat { \xi } )). These formulas give, in particular,
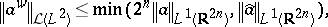 |
where \mathcal{L} ( L ^ { 2 } ) stands for the space of bounded linear mappings from L ^ { 2 } ( \mathbf{R} ^ { n } ) into itself. The operator 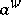 is in the Hilbert–Schmidt class (cf. also Hilbert–Schmidt operator) if and only if a belongs to L ^ { 2 } ( \mathbf{R} ^ { 2 n } ) and \| a\| _{\text{HS}} = \| a \| _ { L } 2 _ { ( \mathbf{R} ^ { 2 n }) } . To get this, it suffices to notice the relationship between the symbol a of
is in the Hilbert–Schmidt class (cf. also Hilbert–Schmidt operator) if and only if a belongs to L ^ { 2 } ( \mathbf{R} ^ { 2 n } ) and \| a\| _{\text{HS}} = \| a \| _ { L } 2 _ { ( \mathbf{R} ^ { 2 n }) } . To get this, it suffices to notice the relationship between the symbol a of 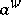 and its distribution kernel k:
and its distribution kernel k:
\begin{equation*} a ( x , \xi ) = \int k \left( x + \frac { t } { 2 } , x - \frac { t } { 2 } \right) e ^ { - 2 i \pi t \xi } d t. \end{equation*}
The Fourier transform of the Wigner function is the so-called ambiguity function
\begin{equation} \tag{a7} {\cal A} ( u , v ) ( \xi , x ) = \int u \left( z - \frac { x } { 2 } \right) \bar{v} \left( z + \frac { x } { 2 } \right) e ^ { - 2 i \pi z . \xi } d z. \end{equation}
For \varphi , \psi \in L ^ { 2 } ( \mathbf{R} ^ { n} ), the Wigner function \mathcal{H} ( \varphi , \psi ) is the Weyl symbol of the operator u \mapsto ( u , \psi ) \varphi (cf. also Symbol of an operator), where ( u , \psi ) is the L^{2} (Hermitian) dot-product, so that from (a5) one finds
\begin{equation*} (u, \psi ) _ { L ^ { 2 } ( {\bf R} ^ { n } ) } ( \varphi , u ) _ { L ^ { 2 } ( {\bf R} ^ { n } ) } = ( {\cal H} ( u , v ) , {\cal H} ( \psi , \varphi ) ) _ { L ^ { 2 } ( {\bf R} ^ { 2 n } ) }. \end{equation*}
As is shown below, the symplectic invariance of the Weyl quantization is actually its most important property.
Symplectic invariance.
Consider a finite-dimensional real vector space E (the configuration space {\bf R} _ { x } ^ { n }) and its dual space E ^ { * } (the momentum space {\bf R} _ { \xi } ^ { n }). The phase space is defined as \Phi = E \oplus E ^ { * }; its running point will be denoted, in general, by a capital letter (X = ( x , \xi ) , Y = ( y , \eta )). The symplectic form (cf. also Symplectic connection) on \Phi is given by
\begin{equation} \tag{a8} [ ( x , \xi ) , ( y , \eta ) ] = \langle \xi , y \rangle _ { E ^{ * } , E } - \langle \eta , x \rangle _ { E ^{ * } , E }, \end{equation}
where \langle \cdot , \cdot \rangle _ { E ^ { * } , E} stands for the bracket of duality. The symplectic group is the subgroup of the linear group of \Phi preserving (a8). With
\begin{equation*} \sigma = \left( \begin{array} { c c } { 0 } & { \operatorname{Id} ( E ^ { * } ) } \\ { - \operatorname{Id} ( E ) } & { 0 } \end{array} \right), \end{equation*}
for X , Y \in \Phi one has
\begin{equation*} [ X , Y ] = \langle \sigma X , Y \rangle _ { \Phi ^ { * } , \Phi }, \end{equation*}
so that the equation of the symplectic group is
\begin{equation*} A ^ { * } \sigma A = \sigma. \end{equation*}
One can describe a set of generators for the symplectic group \operatorname{Sp} ( n ), identifying \Phi with \mathbf{R} _ { x } ^ { n } \times \mathbf{R} _ { \xi } ^ { n }: the mappings
i) ( x , \xi ) \mapsto ( T x , \square ^ { t } T ^ { - 1 } \xi ), where T is an automorphism of E;
ii) ( x _ { k } , \xi _ { k } ) \mapsto ( \xi _ { k } , - x _ { k } ) and the other coordinates fixed;
iii) ( x , \xi ) \mapsto ( x , \xi + S x ), where S is symmetric from E to E ^ { * }. One then describes the metaplectic group, introduced by A. Weil [a11]. The metaplectic group \operatorname{ Mp } ( n ) is the subgroup of the group of unitary transformations of L ^ { 2 } ( \mathbf{R} ^ { n } ) generated by
j) ( M _ { T } u ) ( x ) = | \operatorname { det } T \rceil ^ { - 1 / 2 } u ( T ^ { - 1 } x ), where T \in \operatorname{GL} ( n , \mathbf{R} );
jj) partial Fourier transformations;
jjj) multiplication by \operatorname { exp } ( i \pi \langle S x , x \rangle ), where S is a symmetric matrix. There exists a two-fold covering (the \pi_{1} of both \operatorname{ Mp } ( n ) and \operatorname{Sp} ( n ) is \bf Z)
\begin{equation*} \pi : \operatorname { Mp} ( n ) \rightarrow \operatorname { Sp} ( n ) \end{equation*}
such that, if \chi = \pi ( M ) and u, v are in L ^ { 2 } ( \mathbf{R} ^ { n } ), while \mathcal{H} ( u , v ) is their Wigner function, then
\begin{equation*} \mathcal{H} ( M u , M v ) = \mathcal{H} ( u , v ) \circ \chi ^ { - 1 }. \end{equation*}
This is Segal's formula [a9], which can be rephrased as follows. Let a \in {\cal S} ^ { \prime } ( {\bf R} ^ { 2 n } ) and \chi \in \operatorname { Sp } ( n ). There exists an M in the fibre of  such that
such that
\begin{equation} \tag{a9} ( a \circ \chi ) ^ { w } = M ^ { * } a ^ { w } M. \end{equation}
In particular, the images by \pi of the transformations j), jj), jjj) are, respectively, i), ii), iii). Moreover, if 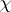 is the phase translation, \chi ( x , \xi ) = ( x + x _ { 0 } , \xi + \xi _ { 0 } ), (a9) is fulfilled with M = \tau _ { x _ { 0 } , \xi _ { 0 }} and phase translation given by
is the phase translation, \chi ( x , \xi ) = ( x + x _ { 0 } , \xi + \xi _ { 0 } ), (a9) is fulfilled with M = \tau _ { x _ { 0 } , \xi _ { 0 }} and phase translation given by
\begin{equation*} ( \tau _ { x _ { 0 } , \xi _ { 0 } } u ) ( y ) = u ( y - x _ { 0 } ) e ^ { 2 i \pi \langle y - x _ { 0 } / 2 , \xi _ { 0 } \rangle }. \end{equation*}
If 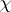 is the symmetry with respect to ( x _ { 0 } , \xi _ { 0 } ), M in (a9) is, up to a unit factor, the phase symmetry \sigma _ { x _ { 0 } , \xi _ { 0 } } defined above. This yields the following composition formula:
is the symmetry with respect to ( x _ { 0 } , \xi _ { 0 } ), M in (a9) is, up to a unit factor, the phase symmetry \sigma _ { x _ { 0 } , \xi _ { 0 } } defined above. This yields the following composition formula: 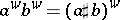 with
with
\begin{equation} \tag{a10} ( a \sharp b ) ( X ) = \end{equation}
\begin{equation*} = 2 ^ { 2 n } \int \int e ^ { - 4 i \pi [ X - Y , X - Z ] } { a } ( Y ) b ( Z ) d Y d Z , \end{equation*}
with an integral on \mathbf{R} ^ { 2 n } \times \mathbf{R} ^ { 2 n }. One can compare this with the classical composition formula,
\begin{equation*} \operatorname {Op} ( a ) \operatorname {Op} ( b ) = \operatorname {Op} ( a \circ b ) \end{equation*}
(cf. (a2)) with
\begin{equation*} ( a \circ b ) ( x , \xi ) = \int \int e ^ { - 2 i \pi y . \eta } a ( x , \xi + \eta ) b ( y + x , \xi ) d y d \eta, \end{equation*}
with an integral on \mathbf{R} ^ { n } \times \mathbf{R} ^ { n }. It is convenient to give an asymptotic version of these compositions formulas, e.g. in the semi-classical case. Let m be a real number. A smooth function a ( x , \xi , h ) defined on \mathbf{R} _ { x } ^ { n } \times \mathbf{R} _ { \xi } ^ { n } \times ( 0,1 ] is in the symbol class  if
if
\begin{equation*} \operatorname { sup } _ {\substack{ ( x , \xi ) \in {\bf R} ^ { 2 n }}, \\{0<h\leq 1} } \left| D _ { x } ^ { \alpha } D _ { \xi } ^ { \beta } a ( x , \xi , h ) \right| h ^ { m - | \beta | } < \infty . \end{equation*}
Then one has for 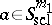 and
and 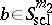 the expansion
the expansion
\begin{equation} \tag{a11} ( a \sharp b ) ( x , \xi ) = r _ { N } ( a , b ) + \end{equation}
\begin{equation*} + \sum _ { 0 \leq k < N } 2 ^ { - k } \sum _ { | \alpha | + | \beta | = k } \frac { ( - 1 ) ^ { | \beta | } } { \alpha ! \beta ! } D _ { \xi } ^ { \alpha } \partial _ { x } ^ { \beta } a D _ { \xi } ^ { \beta } \partial _ { x } ^ { \alpha } b, \end{equation*}
with r _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N}. The beginning of this expansion is thus
\begin{equation*} a b + \frac { 1 } { 2 \iota} \{ a , b \}, \end{equation*}
where \{ a , b \} denotes the Poisson brackets and \iota = 2 \pi {i} . The sums inside (a11) with k even are symmetric in a , b and skew-symmetric for k odd. This can be compared to the classical expansion formula
\begin{equation*} ( a \circ b ) ( x , \xi ) = \sum _ { | \alpha | < N } \frac { 1 } { \alpha ! } D _ { \xi } ^ { \alpha } a \partial _ { x } ^ { \alpha } b + t _ { N } ( a , b ), \end{equation*}
with t _ { N } ( a , b ) \in S _ { \text{scl} } ^ { m _ { 1 } + m _ { 2 } - N }. Moreover, for a _ { 1 } , \dots , a _ { 2k } in L ^ { 1 } ( \Phi = \mathbf{R} ^ { 2 n } ), the multiple composition formula gives
 |
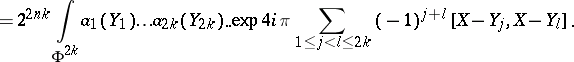 |
\begin{equation*} .d Y _ { 1 } \ldots d Y _ { 2 k }, \end{equation*}
and if a _ { 2 k + 1 } \in L ^ { 1 } ( \Phi ),
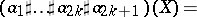 |
\begin{equation*} = 2 ^ { 2 n k } \int _ { \Phi ^ { 2 k } } a _ { 1 } ( Y _ { 1 } ) \ldots a _ { 2 k } ( Y _ { 2 k } ) \text{..} a _ { 2 k + 1 } \left( X + \sum _ { 1 \leq j < l \leq 2 k } ( - 1 ) ^ { j + l } ( Y _ { j } - Y _ { l } ) \right). \end{equation*}
\begin{equation*} .\operatorname { exp } 4 i \pi \sum _ { 1 \leq j < l \leq 2 k } ( - 1 ) ^ { j + l } [ X - Y _ { j } , X - Y _ { l } ] .. d Y _ { 1 } \ldots d Y _ { 2 k }. \end{equation*}
Consider the standard sum of homogeneous symbols defined on \Omega \times \mathbf{R} ^ { n }, where \Omega is an open subset of {\bf R} ^ { n },
\begin{equation*} a = a _ { m } + a _ { m - 1 } + r _ { m - 2 }, \end{equation*}
with a _ { j } smooth on \Omega \times \mathbf{R} ^ { n } and homogeneous in the following sense:
\begin{equation*} a _ { j } ( x , \lambda \xi ) = \lambda ^ { j } a _ { j } ( x , \xi ) , \text { for } | \xi | \geq 1 , \lambda \geq 1, \end{equation*}
and r _ { m - 2} \in S _ { \text{loc} } ^ { m - 2 } ( \Omega ), i.e. for all compact subsets K of \Omega,
\begin{equation*} \operatorname { sup } _ { x \in K, \atop \xi \in {\bf R} ^ { n } } \left| ( D _ { x } ^ { \alpha } D _ { \xi } ^ { \beta } r _ { m - 2 } ) ( x , \xi ) \right| ( 1 + | \xi | ) ^ { 2 - m + | \beta | } < \infty. \end{equation*}
This class of pseudo-differential operators (cf. also Pseudo-differential operator) is invariant under diffeomorphisms, and using the Weyl quantization one gets that the principal symbol a _ { m } is invariantly defined on the cotangent bundle T ^ { * } ( \Omega ) whereas the subprincipal symbol a_{m - 1} is invariantly defined on the double characteristic set
\begin{equation*} \{ a _ { m } = 0 , d a _ { m } = 0 \} \end{equation*}
of the principal symbol. If one writes
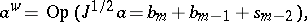 |
one gets a = J ^ { - 1 / 2 } b and a _ { m } = b _ { m }. Moreover,
\begin{equation*} a _ { m - 1 } = b _ { m - 1 } - \frac { 1 } { 2 \iota } \sum _ { 1 \leq j \leq n } \frac { \partial ^ { 2 } b _ { m } } { \partial x _ { j } \partial \xi _ { j } } = b _ { m - 1 } ^ { s }. \end{equation*}
Thus, if one defines the subprincipal symbol as the above analytic expression b ^ { s } _{m - 1} where b = b _ { m } + b _ { m - 1} + \ldots is the classical symbol of a ^ { w } = \operatorname{Op} ( b ), one finds that this invariant b ^ { s } _{m - 1} is simply the second term a_{m - 1} in the expansion of the Weyl symbol a. In the same vein, it is also useful to note that when considering pseudo-differential operators acting on half-densities one gets a refined principal symbol a _ { m } + a _ { m - 1 } invariant by diffeomorphism.
Weyl–Hörmander calculus and admissible metrics.
The developments of the analysis of partial differential operators in the 1970s required refined localizations in the phase space. E.g., the Beals–Fefferman local solvability theorem [a2] yields the geometric condition (P) as an if-and-only-if solvability condition for differential operators of principal type (with possibly complex symbols). These authors removed the analyticity assumption used by L. Nirenberg and F. Treves, and a key point in their method is a Calderón–Zygmund decomposition of the symbol, that is, a micro-localization procedure depending on a particular function, yielding a pseudo-differential calculus tailored to the symbol under investigation. Another example is provided by the Fefferman–Phong inequality [a6], establishing that second-order operators with non-negative symbols are bounded from below on L^{2}; a Calderón–Zygmund decomposition is needed in the proof, as well as an induction on the number of variables. These micro-localizations go much beyond the standard homogeneous calculus and also beyond the classes 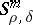 , previously called exotic. In 1979, L.V. Hörmander published [a7], providing simple and general rules for a pseudo-differential calculus to be admissible. Consider a positive-definite quadratic form G defined on \Phi. The dual quadratic form G ^ { \sigma } with respect to the symplectic structure is
, previously called exotic. In 1979, L.V. Hörmander published [a7], providing simple and general rules for a pseudo-differential calculus to be admissible. Consider a positive-definite quadratic form G defined on \Phi. The dual quadratic form G ^ { \sigma } with respect to the symplectic structure is
\begin{equation} \tag{a12} G ^ { \sigma } ( T ) = \operatorname { sup } _ { G ( U ) = 1 } [ T , U ] ^ { 2 } . \end{equation}
Define an admissible metric on the phase space as a mapping from \Phi to the set of positive-definite quadratic forms on \Phi, X \mapsto G _ { X }, such that the following three properties are fulfilled:
(uncertainty principle) For all X \in \Phi,
\begin{equation} \tag{a13} G _ { X } \leq G _ { X } ^ { g }; \end{equation}
there exist some positive constants \rho, C, such that, for all X , Y \in \Phi,
\begin{equation} \tag{a14} G _ { X } ( X - Y) \leq \rho ^ { 2 } \Rightarrow G _ { Y } \leq C G _ { X }; \end{equation}
there exist some positive constants N, C, such that, for all X , Y \in \Phi,
\begin{equation} \tag{a15} G _ { X } \leq C ( 1 + G _ { X } ^ { \sigma } ( X - Y ) ) ^ { N } G _ { Y }. \end{equation}
Property (a13) is clearly related to the uncertainty principle, since for each X one can diagonalize the quadratic form G _ { X } in a symplectic basis so that
\begin{equation*} G _ { X } = \sum _ { 1 \leq j \leq n } h _ { j } ( | d q _ { j } | ^ { 2 } + | d p _ { j } | ^ { 2 } ), \end{equation*}
where ( q_j , p _ { j } ) is a set of symplectic coordinates. One then gets
\begin{equation*} G _ { X } ^ { g } = \sum _ { 1 \leq j \leq n } h _ { j } ^ { - 1 } ( | d q _ { j } | ^ { 2 } + | d p _ { j } | ^ { 2 } ). \end{equation*}
Condition (a13) thus means that \operatorname{max} h_{j} \leq 1, which can be rephrased in the familiarly vague version as
\begin{equation*} \Delta p _ { j } \Delta q_j \sim h _ { j } ^ { - 1 } \geq 1 \end{equation*}
in the G-balls. This condition is relevant to any micro-localization procedure. When G = G ^ { \sigma }, one says that the quadratic form is symplectic. Property (a14) is called slowness of the metric and is usually easy to verify. Property (a15) is the temperance of the metric and is more of a technical character, although very important in handling non-local terms in the composition formula. In particular, this property is useful to verify the assumptions of Cotlar's lemma. Moreover, one defines a weight m as a positive function on \Phi such that there exist positive constants C, N so that for all X , Y \in \Phi,
\begin{equation} \tag{a16} m ( X ) \leq C ( 1 + G _ { X } ^ { \sigma } ( X - Y ) ) ^ { N } m ( Y ), \end{equation}
and
\begin{equation} \tag{a17} G _ { X } ( X - Y ) \leq C ^ { - 1 } \Rightarrow C ^ { - 1 } \leq \frac { m ( X ) } { m ( Y ) } \leq C. \end{equation}
Eventually, one defines the class of symbols S ( m , G ) as the C ^ { \infty }-functions a on the phase space such that
\begin{equation} \tag{a18} \operatorname { sup } _ { X \in \Phi } \| a ^ { ( k ) } ( X ) \| _ { G _ { X } } m ( X ) ^ { - 1 } < \infty. \end{equation}
It is, for instance, easily checked that
\begin{equation*} S _ { \rho , \delta } ^ { \mu } = S \left( \langle \xi \rangle ^ { \mu } , \langle \xi \rangle ^ { 2 \delta } | d x | ^ { 2 } + \langle \xi \rangle ^ { - 2 \rho } | d \xi | ^ { 2 } \right), \end{equation*}
with \langle \xi \rangle = 1 + | \xi | and that this metric is an admissible metric when 0 \leq \delta \leq \rho \leq 1, \delta < 1. The metric defining S _ { 1,1 } ^ { 0 } satisfies (a13)–(a14) but fails to satisfy (a15). Indeed, there are counterexamples showing that for the classical and the Weyl quantization [a4] there are symbols in S _ { 1,1 } ^ { 0 } whose quantization is not L^{2}-bounded. In fact, one of the building block for the calculus of pseudo-differential operators is the L^{2}-boundedness of the Weyl quantization of symbols in S ( 1 , G ), where G is an admissible metric. One defines the Planck function of the calculus as
\begin{equation} \tag{a19} H ( X ) = \operatorname { sup } _ { T \neq 0 } \sqrt { \frac { G _{X} ( T ) } { G _ { X } ^ { \sigma } ( T ) } } \end{equation}
and notes that from (a13), H ( X ) \leq 1. One obtains the composition formula (a11) with \alpha \in S ( m _ { 1 } , G ), b \in S ( m _ { 2 } , G ) and r _ { N } ( a , b ) \in S ( m _ { 1 } m _ { 2 } H ^ { N } , G ). In particular, one obtains, with obvious notations,
\begin{equation*} a \sharp b \in S ( m _ { 1 } m _ { 2 } , G ), \end{equation*}
\begin{equation*} a \sharp b = a b + S ( m _ { 1 } m _ { 2 } H , G ) ,\; a \sharp b = a b + \frac { 1 } { 2 \iota } \{ a , b \} + S ( m _ { 1 } m _ { 2 } H ^ { 2 } , G ), \end{equation*}
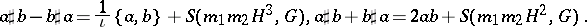 |
The Fefferman–Phong inequality has also a simple expression in this framework: Let a be a non-negative symbol in S ( H ^ { - 2 } , G ), then the operator 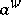 is semi-bounded from below in L ^ { 2 } ( \mathbf{R} ^ { n } ). The proof uses a Calderón–Zygmund decomposition and in fact one shows that a \in S ( h ^ { - 2 } , g ), where h is the Planck function related to the admissible metric g defined by
is semi-bounded from below in L ^ { 2 } ( \mathbf{R} ^ { n } ). The proof uses a Calderón–Zygmund decomposition and in fact one shows that a \in S ( h ^ { - 2 } , g ), where h is the Planck function related to the admissible metric g defined by
\begin{equation*} g_{X} ( T ) = \frac { G _ { X } ( T ) } { H ( X ) [ 1 + a ( X ) + H ( X ) ^ { 2 } \| a ^ { \prime \prime } ( X ) \| ^ { 2 _{ G _ { X }}} ] ^ { 1 / 2 } }. \end{equation*}
On the other hand, if G is an admissible metric and q _ { \alpha } \in S ( H ^ { - 1 } , G ) uniformly with respect to a parameter \alpha, the following metric also satisfies (a13)–(a15): \tilde { g } _ { X } = H ( X ) ^ { - 1 } \tilde { h } ( X ) G _ { X } ( T ), with
\begin{equation*} \widetilde { h } ( X ) ^ { - 1 } = 1 + \operatorname { sup } _ { \alpha } | q _ { \alpha } ( X ) | + H ( X ) \operatorname { sup } _ { \alpha } \| q _ { \alpha } ^ { \prime } ( X ) \| _ { G _ { X } } ^ { 2 }. \end{equation*}
One gets in this case that q _ { \alpha } \in S ( \tilde { h } ^ { - 1 } , \tilde{g} ) uniformly. A key point in the Beals–Fefferman proof of local solvability under condition (P) can be reformulated through the construction of the previous metric. Sobolev spaces related to this type of calculus were studied in [a1] (cf. also Sobolev space). For an admissible metric G and a weight m, the space H ( m , G ) is defined as
\begin{equation*} \{ u \in \mathcal{S} ^ { \prime } ( \mathbf{R} ^ { n } ) : \forall a \in S ( m , G ) , a ^ { w } u \in L ^ { 2 } ( \mathbf{R} ^ { n } ) \}. \end{equation*}
It can be proven that a Hilbertian structure can be set on H ( m , G ), that H ( 1 , G ) = L ^ { 2 } ( \mathbf{R} ^ { n } ) and that for a \in S ( m , G ) and m_1 another weight, the mapping
\begin{equation*} a ^ { w } : H ( m m _ { 1 } , G ) \rightarrow H ( m _ { 1 } , G ) \end{equation*}
is continuous.
Further developments of the Weyl calculus were explored in [a3], with higher-order micro-localizations. Several metrics
\begin{equation*} g _ { 1 } \leq \ldots \leq g _ { k } \end{equation*}
are given on the phase space. All these metrics satisfy (a13)–(a14), but, except for g_1, fail to satisfy globally the temperance condition (a15). Instead, the metric g_{l+ 1} is assumed to be (uniformly) temperate on the g_{l}-balls. It is then possible to produce a satisfactory quantization formula for symbols belonging to a class S ( m , g _ { k } ). A typical example is given in [a5], with applications to propagation of singularities for non-linear hyperbolic equations:
\begin{equation*} g _ { 1 } = | d x | ^ { 2 } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } } \leq g_2 = \frac { | d x | ^ { 2 } } { | x | ^ { 2 } } + \frac { | d \xi | ^ { 2 } } { | \xi | ^ { 2 } }, \end{equation*}
where g_1 is defined on | \xi | > 1, and g_{2} on
\begin{equation*} \{ | x | < 1 , | x | | \xi | > 1 \} \end{equation*}
It is then possible to quantize functions a ( x , \xi ) homogeneous of degree \mu in the variable x, and \nu in the variable \xi , so as to get composition formulas, Sobolev spaces, and the standard pseudo-differential apparatus allowing a commutator argument to work for propagation results.
References
| [a1] | J.-M. Bony, J.-Y. Chemin, "Espaces fonctionnels associés au calcul de Weyl–Hörmander" Bull. Soc. Math. France , 122 (1994) pp. 77–118 Zbl 0798.35172 |
| [a2] | R. Beals, C. Fefferman, "On local solvability of linear partial differential equations" Ann. of Math. , 97 (1973) pp. 482–498 MR0352746 Zbl 0256.35002 |
| [a3] | J.-M. Bony, N. Lerner, "Quantification asymtotique et microlocalisations d'ordre supérieur" Ann. Sci. Ecole Norm. Sup. , 22 (1989) pp. 377–483 |
| [a4] | A. Boulkhemair, "Remarque sur la quantification de Weyl pour la classe de symboles S _ { 1,1 } ^ { 0 }" C.R. Acad. Sci. Paris , 321 : 8 (1995) pp. 1017–1022 MR1360564 Zbl 0842.35144 |
| [a5] | J.-M. Bony, "Second microlocalization and propagation of singularities for semi-linear hyperbolic equations" K. Mizohata (ed.) , Hyperbolic Equations and Related Topics , Kinokuniya (1986) pp. 11–49 MR925240 |
| [a6] | C. Fefferman, D.H. Phong, "On positivity of pseudo-differential operators" Proc. Nat. Acad. Sci. USA , 75 (1978) pp. 4673–4674 MR0507931 Zbl 0391.35062 |
| [a7] | L. Hörmander, "The Weyl calculus of pseudo-differential operators" Commun. Pure Appl. Math. , 32 (1979) pp. 359–443 MR517939 Zbl 0388.47032 |
| [a8] | L. Hörmander, "The analysis of linear partial differential operators III-IV" , Springer (1985) |
| [a9] | I. Segal, "Transforms for operators and asymptotic automorphisms over a locally compact abelian group" Math. Scand. , 13 (1963) pp. 31–43 |
| [a10] | A. Unterberger, "Oscillateur harmonique et opérateurs pseudo-différentiels" Ann. Inst. Fourier , 29 : 3 (1979) pp. 201–221 MR0552965 Zbl 0396.47027 |
| [a11] | A. Weil, "Sur certains groupes d'opérateurs unitaires" Acta Math. , 111 (1964) pp. 143–211 MR0165033 |
| [a12] | H. Weyl, "Gruppentheorie und Quantenmechanik" , S. Hirzel (1928) MR0450450 Zbl 54.0954.03 |
Weyl quantization. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Weyl_quantization&oldid=50110