Difference between revisions of "Cantor ternary function"
(Created page with "{{MSC|26A45|28A15}} Category:Analysis {{TEX|done}} The Cantor ternary function (also called Devil's staircase) is a continuous monotone function $f$ mapping the interva...") |
(asy code to subpage) |
||
| (9 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| + | |||
{{MSC|26A45|28A15}} | {{MSC|26A45|28A15}} | ||
| Line 5: | Line 6: | ||
{{TEX|done}} | {{TEX|done}} | ||
| − | The Cantor ternary function (also called Devil's staircase) is a continuous monotone function $f$ mapping the interval $[0,1]$ onto itself, with the remarkable property that its derivative vanishes almost everywhere (recall that any monotone function is differentiable almost everywhere, see for instance [[Function of bounded variation]]). | + | The Cantor ternary function (also called Devil's staircase and, rarely, Lebesgue's singular function) is a continuous monotone function $f$ mapping the interval $[0,1]$ onto itself, with the remarkable property that its derivative vanishes almost everywhere (recall that any monotone function is differentiable almost everywhere, see for instance [[Function of bounded variation]]). |
| − | The function can be defined in the following way ( | + | {{:Cantor ternary function/Fig1}} |
| + | |||
| + | The function can be defined in the following way (see, for example, Exercise 46 in Chapter 2 of {{Cite|Ro}}). Given $x\in [0,1]$ consider its ternary expansion $\{a_i\}$, i.e. a choice of coefficients $a_i\in \{0,1,2\}$ such that | ||
\[ | \[ | ||
| − | x = \sum_{i= | + | x = \sum_{i=1}^\infty \frac{a_i}{3^i}\, . |
\] | \] | ||
Define $n(x)$ to be | Define $n(x)$ to be | ||
| Line 16: | Line 19: | ||
Then | Then | ||
\[ | \[ | ||
| − | f(x) = \sum_{i= | + | f(x) = \sum_{i=1}^{n(x)-1} \frac{a_i}{2^{i+1}} + \frac{1}{2^{n(x)}}. |
\] | \] | ||
For alternative definitions see Example 1.67 of {{Cite|AFP}} and page 55 of {{Cite|Co}}. | For alternative definitions see Example 1.67 of {{Cite|AFP}} and page 55 of {{Cite|Co}}. | ||
| Line 22: | Line 25: | ||
It follows trivially from the definition that $f$ is locally constant on the complement of the [[Cantor set]] $C$: since the Cantor set is a set of Lebesgue measure zero, the derivative of $f$ vanishes almost everywhere. For the same reason, the [[Generalized derivative|distributional derivative]] of $f$ is a [[Singular measures|singular measure]] $\mu$ supported on $C$. | It follows trivially from the definition that $f$ is locally constant on the complement of the [[Cantor set]] $C$: since the Cantor set is a set of Lebesgue measure zero, the derivative of $f$ vanishes almost everywhere. For the same reason, the [[Generalized derivative|distributional derivative]] of $f$ is a [[Singular measures|singular measure]] $\mu$ supported on $C$. | ||
| − | The Cantor function is a prototype of [[Singular function]] in the sense of Lebesgue, | + | The Cantor function is a prototype of a [[Singular function|singular function]] in the sense of Lebesgue, cf. [[Lebesgue decomposition]]. The observation that $f$ is a function of bounded variation for which |
| + | \[ | ||
f (1)-f(0) \neq \int_0^a f'(t)\, dt | f (1)-f(0) \neq \int_0^a f'(t)\, dt | ||
\] | \] | ||
| Line 36: | Line 40: | ||
|valign="top"|{{Ref|Ro}}|| H.L. Royden, "Real analysis" , Macmillan (1969). {{MR|0151555}} {{ZBL|0197.03501}} | |valign="top"|{{Ref|Ro}}|| H.L. Royden, "Real analysis" , Macmillan (1969). {{MR|0151555}} {{ZBL|0197.03501}} | ||
|- | |- | ||
| − | |valign="top"|{{Ref|Vi}}|| A. Vitali,"Sulle funzioni integrali", Atti Accad. Sci. Torino Cl. Sci. Fis. Mat. Natur., '''40''' 1905 pp. 1021-1034. | + | |valign="top"|{{Ref|Vi}}|| A. Vitali, "Sulle funzioni integrali", Atti Accad. Sci. Torino Cl. Sci. Fis. Mat. Natur., '''40''' 1905 pp. 1021-1034. |
|- | |- | ||
|} | |} | ||
Latest revision as of 11:26, 13 December 2014
2020 Mathematics Subject Classification: Primary: 26A45 Secondary: 28A15 [MSN][ZBL]
The Cantor ternary function (also called Devil's staircase and, rarely, Lebesgue's singular function) is a continuous monotone function $f$ mapping the interval $[0,1]$ onto itself, with the remarkable property that its derivative vanishes almost everywhere (recall that any monotone function is differentiable almost everywhere, see for instance Function of bounded variation).
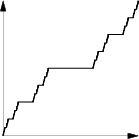
The function can be defined in the following way (see, for example, Exercise 46 in Chapter 2 of [Ro]). Given $x\in [0,1]$ consider its ternary expansion $\{a_i\}$, i.e. a choice of coefficients $a_i\in \{0,1,2\}$ such that \[ x = \sum_{i=1}^\infty \frac{a_i}{3^i}\, . \] Define $n(x)$ to be
- $\infty$ if none of the coefficients $a_i$ takes the value $1$
- the smallest integer $n$ such that $a_n=1$ otherwise.
Then \[ f(x) = \sum_{i=1}^{n(x)-1} \frac{a_i}{2^{i+1}} + \frac{1}{2^{n(x)}}. \] For alternative definitions see Example 1.67 of [AFP] and page 55 of [Co].
It follows trivially from the definition that $f$ is locally constant on the complement of the Cantor set $C$: since the Cantor set is a set of Lebesgue measure zero, the derivative of $f$ vanishes almost everywhere. For the same reason, the distributional derivative of $f$ is a singular measure $\mu$ supported on $C$.
The Cantor function is a prototype of a singular function in the sense of Lebesgue, cf. Lebesgue decomposition. The observation that $f$ is a function of bounded variation for which \[ f (1)-f(0) \neq \int_0^a f'(t)\, dt \] (where $f'$ denotes the classical pointwise derivative) was first made by Vitali in [Vi]. For this reason some authors use the terminology Cantor-Vitali function (see [AFP]).
References
| [AFP] | L. Ambrosio, N. Fusco, D. Pallara, "Functions of bounded variations and free discontinuity problems". Oxford Mathematical Monographs. The Clarendon Press, Oxford University Press, New York, 2000. MR1857292Zbl 0957.49001 |
| [Co] | D. L. Cohn, "Measure theory". Birkhäuser, Boston 1993. |
| [Ro] | H.L. Royden, "Real analysis" , Macmillan (1969). MR0151555 Zbl 0197.03501 |
| [Vi] | A. Vitali, "Sulle funzioni integrali", Atti Accad. Sci. Torino Cl. Sci. Fis. Mat. Natur., 40 1905 pp. 1021-1034. |
Cantor ternary function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cantor_ternary_function&oldid=27834