Difference between revisions of "Stable rank"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | s0871301.png | ||
| + | $#A+1 = 101 n = 2 | ||
| + | $#C+1 = 101 : ~/encyclopedia/old_files/data/S087/S.0807130 Stable rank | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | + | Let $ R $ | |
| + | be an associative ring with unit element. A sequence of elements $ ( a _ {1} \dots a _ {n} ) $ | ||
| + | is called left unimodular if the left ideal generated by the $ a _ {i} $, | ||
| + | $ i= 1 \dots n $, | ||
| + | is all of $ R $. | ||
| − | + | The left stable rank of $ R $ | |
| + | is the least integer $ n $ | ||
| + | such that for each $ m> n $ | ||
| + | and left unimodular sequence $ ( a _ {1} \dots a _ {m} ) $ | ||
| + | there are $ r _ {1} \dots r _ {m-} 1 $ | ||
| + | such that $ ( a _ {1} ^ \prime \dots a _ {m-} 1 ^ \prime ) $ | ||
| + | with $ a _ {i} ^ \prime = a _ {i} + r _ {i} a _ {m} $, | ||
| + | $ i= 1 \dots m- 1 $, | ||
| + | is also left unimodular. | ||
| − | + | The right stable rank of $ R $ | |
| + | is defined analogously by replacing left with right everywhere. The left and right stable ranks are equal [[#References|[a1]]], cf. also, e.g., [[#References|[a2]]], §11.3, and both are therefore called the stable rank of $ R $, | ||
| + | denoted by $ \textrm{ st.r. } ( R) $. | ||
| − | + | By writing a left unimodular sequence $ ( a _ {1} \dots a _ {n} ) $ | |
| + | as a column, there is a natural left action of $ \mathop{\rm GL} _ {n} ( R ) $ | ||
| + | on $ U _ {c} ( n, R ) $, | ||
| + | the set of all left unimodular sequences of length $ n $. | ||
| + | The general linear rank, $ \mathop{\rm glr} ( R) $, | ||
| + | of $ R $ | ||
| + | is the least integer $ n $ | ||
| + | such that $ \mathop{\rm GL} _ {m} ( R) $ | ||
| + | acts transitively on $ U _ {c} ( m, R) $ | ||
| + | for all $ m> n $. | ||
| + | This is equivalent to the property that all right stably-free modules of rank $ \geq n $ | ||
| + | are free, [[#References|[a2]]]. | ||
| − | + | Recall that $ P $ | |
| + | is [[Stably free module|stably free]] if $ P\oplus R ^ {n} \simeq R ^ {m} $ | ||
| + | for some $ n, m $; | ||
| + | the rank of $ P $ | ||
| + | is then defined as $ m- n $. | ||
| + | This is well-defined if $ R $ | ||
| + | has the invariant basis property (i.e. $ R ^ {n} \simeq R ^ {m} $ | ||
| + | if and only if $ n= m $). | ||
| + | This property holds, e.g., if $ R $ | ||
| + | is commutative or right Noetherian. | ||
| − | + | One has $ \mathop{\rm glr} ( R) \leq \textrm{ st.r. } ( R) $, | |
| + | so that any stably-free module of rank $ \geq \textrm{ st.r. } ( R) $ | ||
| + | is free. | ||
| − | + | For a field $ k $ | |
| + | one has $ \mathop{\rm glr} ( k[ X _ {1} \dots X _ {n} ])= 1 $ | ||
| + | for all $ n $. | ||
| − | Let | + | Let $ k $ |
| + | be a field of transcendence degree $ t $ | ||
| + | over its prime subfield $ k _ {0} $. | ||
| + | The Kronecker dimension of $ k $ | ||
| + | is then defined as $ t+ 1 $ | ||
| + | if $ \mathop{\rm char} k= 0 $ | ||
| + | and as $ t $ | ||
| + | otherwise. For $ n\leq $ | ||
| + | Kronecker dimension of $ k $, | ||
| + | $ \textrm{ st.r. } ( k[ X _ {1} \dots X _ {n} ]) = n+ 1 $. | ||
| + | If $ R $ | ||
| + | is commutative of Krull dimension $ m< \infty $( | ||
| + | cf. also [[Dimension|Dimension]] of an associative ring), then $ \textrm{ st.r. } ( R[ X _ {1} \dots X _ {n} ]) \leq m+ n+ 1 $( | ||
| + | Bass' theorem). | ||
| − | + | Let $ X $ | |
| + | be a topological space, $ Y $ | ||
| + | a metric space and $ f: X \rightarrow Y $ | ||
| + | a continuous mapping. A point $ y \in Y $ | ||
| + | is a stable value of $ f $ | ||
| + | if it is in $ f( X) $ | ||
| + | and if there is an $ \epsilon $ | ||
| + | such that for every continuous mapping $ g: X \rightarrow Y $ | ||
| + | with $ \| f( x)- g( x) \| < \epsilon $ | ||
| + | for all $ x \in X $ | ||
| + | it is still true that $ y \in g( X) $. | ||
| + | The mapping dimension of a topological space $ X $, | ||
| + | $ d( X) $, | ||
| + | is the largest integer $ d $ | ||
| + | for which there exists a mapping $ X \rightarrow \mathbf R ^ {d} $ | ||
| + | for which the origin is a stable value. (If no such $ d $ | ||
| + | exists, $ d( X) $ | ||
| + | is set equal to $ \infty $.) | ||
| + | For nice spaces, e.g., metrizable, separable, $ X $, | ||
| + | this concept of dimension coincides with other notions of dimension, such as inductive dimension, [[#References|[a5]]], Chapt. VI, §1 (cf. [[Dimension theory|Dimension theory]]). It always coincides with the notion of dimension defined by essential mappings (cf. [[Dimension theory|Dimension theory]]), [[#References|[a5]]], Chapt. VI, §3. | ||
| − | + | Let $ C( X) $ | |
| + | be the ring of real-valued continuous functions on a topological space $ X $ | ||
| + | and $ C _ {b} ( X) \subset C( X) $ | ||
| + | the subring of bounded functions. Then $ \textrm{ st.r. } ( C( X)) = \textrm{ st.r. } ( C _ {b} ( X))= d( X)+ 1 $( | ||
| + | Vaserstein's theorem). | ||
| − | + | Both Bass' and Vaserstein's theorem indicate that $ \textrm{ st.r. } ( R) - 1 $ | |
| + | is a good dimension concept for rings. | ||
| + | |||
| + | More generally, the stable rank is defined for subrings and ideals of an associative ring $ R $ | ||
| + | with unit. | ||
| + | |||
| + | Let $ R $ | ||
| + | be an associative ring with unit, and let $ \mathfrak q $ | ||
| + | be a subring (possibly without unit) of $ R $. | ||
| + | A sequence of elements $ ( a _ {1} \dots a _ {n} ) $ | ||
| + | is left $ \mathfrak q $- | ||
| + | unimodular if it is left unimodular (in $ R $) | ||
| + | and, moreover, $ a _ {1} - 1 \in \mathfrak q $, | ||
| + | $ a _ {i} \in \mathfrak q $, | ||
| + | $ i= 2 \dots n $. | ||
| + | The stable rank of the subring $ \mathfrak q $ | ||
| + | is the least number $ n $ | ||
| + | such that for each left $ \mathfrak q $- | ||
| + | unimodular sequence $ ( a _ {1} \dots a _ {m} ) $ | ||
| + | of length $ m> n $ | ||
| + | there are $ q _ {i} \in \mathfrak q $, | ||
| + | $ i= 1 \dots m- 1 $, | ||
| + | such that $ ( a _ {1} ^ \prime \dots a _ {m-} 1 ^ \prime ) $, | ||
| + | with $ a _ {i} ^ \prime = a _ {i} + q _ {i} a _ {m} $, | ||
| + | is a left $ \mathfrak q $- | ||
| + | unimodular sequence of length $ m- 1 $. | ||
| + | (Such a property is referred to as a stable range condition, cf., e.g., [[#References|[a4]]]). The stable rank of $ \mathfrak q $ | ||
| + | does not depend on the ambient ring $ R $. | ||
| + | Again it is true that the notion of stable rank is left/right symmetric, [[#References|[a1]]]. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> L.N. Vaserstein, "Stable ranks of rings and dimensionality of topological spaces" ''Funct. Anal. Appl.'' , '''5''' (1971) pp. 102–110 ''Funkts. Anal. i Prilozhen.'' , '''5''' : 2 (1970) pp. 17–27</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.C. McConnell, J.C. Robson, "Noncommutative Noetherian rings" , Wiley (1987) {{MR|934572}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> A.J. Hahn, O.T. O'Meara, "The classical groups and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s087/s087130/s087130103.png" />-theory" , Springer (1981) pp. §4.1 {{MR|1007302}} {{MR|0842441}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H. Bass, "Algebraic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s087/s087130/s087130104.png" />-theory" , Benjamin (1968) pp. Chapt. V, §3 {{MR|249491}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948)</TD></TR></table> |
Latest revision as of 08:22, 6 June 2020
Let $ R $
be an associative ring with unit element. A sequence of elements $ ( a _ {1} \dots a _ {n} ) $
is called left unimodular if the left ideal generated by the $ a _ {i} $,
$ i= 1 \dots n $,
is all of $ R $.
The left stable rank of $ R $ is the least integer $ n $ such that for each $ m> n $ and left unimodular sequence $ ( a _ {1} \dots a _ {m} ) $ there are $ r _ {1} \dots r _ {m-} 1 $ such that $ ( a _ {1} ^ \prime \dots a _ {m-} 1 ^ \prime ) $ with $ a _ {i} ^ \prime = a _ {i} + r _ {i} a _ {m} $, $ i= 1 \dots m- 1 $, is also left unimodular.
The right stable rank of $ R $ is defined analogously by replacing left with right everywhere. The left and right stable ranks are equal [a1], cf. also, e.g., [a2], §11.3, and both are therefore called the stable rank of $ R $, denoted by $ \textrm{ st.r. } ( R) $.
By writing a left unimodular sequence $ ( a _ {1} \dots a _ {n} ) $ as a column, there is a natural left action of $ \mathop{\rm GL} _ {n} ( R ) $ on $ U _ {c} ( n, R ) $, the set of all left unimodular sequences of length $ n $. The general linear rank, $ \mathop{\rm glr} ( R) $, of $ R $ is the least integer $ n $ such that $ \mathop{\rm GL} _ {m} ( R) $ acts transitively on $ U _ {c} ( m, R) $ for all $ m> n $. This is equivalent to the property that all right stably-free modules of rank $ \geq n $ are free, [a2].
Recall that $ P $ is stably free if $ P\oplus R ^ {n} \simeq R ^ {m} $ for some $ n, m $; the rank of $ P $ is then defined as $ m- n $. This is well-defined if $ R $ has the invariant basis property (i.e. $ R ^ {n} \simeq R ^ {m} $ if and only if $ n= m $). This property holds, e.g., if $ R $ is commutative or right Noetherian.
One has $ \mathop{\rm glr} ( R) \leq \textrm{ st.r. } ( R) $, so that any stably-free module of rank $ \geq \textrm{ st.r. } ( R) $ is free.
For a field $ k $ one has $ \mathop{\rm glr} ( k[ X _ {1} \dots X _ {n} ])= 1 $ for all $ n $.
Let $ k $ be a field of transcendence degree $ t $ over its prime subfield $ k _ {0} $. The Kronecker dimension of $ k $ is then defined as $ t+ 1 $ if $ \mathop{\rm char} k= 0 $ and as $ t $ otherwise. For $ n\leq $ Kronecker dimension of $ k $, $ \textrm{ st.r. } ( k[ X _ {1} \dots X _ {n} ]) = n+ 1 $. If $ R $ is commutative of Krull dimension $ m< \infty $( cf. also Dimension of an associative ring), then $ \textrm{ st.r. } ( R[ X _ {1} \dots X _ {n} ]) \leq m+ n+ 1 $( Bass' theorem).
Let $ X $ be a topological space, $ Y $ a metric space and $ f: X \rightarrow Y $ a continuous mapping. A point $ y \in Y $ is a stable value of $ f $ if it is in $ f( X) $ and if there is an $ \epsilon $ such that for every continuous mapping $ g: X \rightarrow Y $ with $ \| f( x)- g( x) \| < \epsilon $ for all $ x \in X $ it is still true that $ y \in g( X) $. The mapping dimension of a topological space $ X $, $ d( X) $, is the largest integer $ d $ for which there exists a mapping $ X \rightarrow \mathbf R ^ {d} $ for which the origin is a stable value. (If no such $ d $ exists, $ d( X) $ is set equal to $ \infty $.) For nice spaces, e.g., metrizable, separable, $ X $, this concept of dimension coincides with other notions of dimension, such as inductive dimension, [a5], Chapt. VI, §1 (cf. Dimension theory). It always coincides with the notion of dimension defined by essential mappings (cf. Dimension theory), [a5], Chapt. VI, §3.
Let $ C( X) $ be the ring of real-valued continuous functions on a topological space $ X $ and $ C _ {b} ( X) \subset C( X) $ the subring of bounded functions. Then $ \textrm{ st.r. } ( C( X)) = \textrm{ st.r. } ( C _ {b} ( X))= d( X)+ 1 $( Vaserstein's theorem).
Both Bass' and Vaserstein's theorem indicate that $ \textrm{ st.r. } ( R) - 1 $ is a good dimension concept for rings.
More generally, the stable rank is defined for subrings and ideals of an associative ring $ R $ with unit.
Let $ R $ be an associative ring with unit, and let $ \mathfrak q $ be a subring (possibly without unit) of $ R $. A sequence of elements $ ( a _ {1} \dots a _ {n} ) $ is left $ \mathfrak q $- unimodular if it is left unimodular (in $ R $) and, moreover, $ a _ {1} - 1 \in \mathfrak q $, $ a _ {i} \in \mathfrak q $, $ i= 2 \dots n $. The stable rank of the subring $ \mathfrak q $ is the least number $ n $ such that for each left $ \mathfrak q $- unimodular sequence $ ( a _ {1} \dots a _ {m} ) $ of length $ m> n $ there are $ q _ {i} \in \mathfrak q $, $ i= 1 \dots m- 1 $, such that $ ( a _ {1} ^ \prime \dots a _ {m-} 1 ^ \prime ) $, with $ a _ {i} ^ \prime = a _ {i} + q _ {i} a _ {m} $, is a left $ \mathfrak q $- unimodular sequence of length $ m- 1 $. (Such a property is referred to as a stable range condition, cf., e.g., [a4]). The stable rank of $ \mathfrak q $ does not depend on the ambient ring $ R $. Again it is true that the notion of stable rank is left/right symmetric, [a1].
References
| [a1] | L.N. Vaserstein, "Stable ranks of rings and dimensionality of topological spaces" Funct. Anal. Appl. , 5 (1971) pp. 102–110 Funkts. Anal. i Prilozhen. , 5 : 2 (1970) pp. 17–27 |
| [a2] | J.C. McConnell, J.C. Robson, "Noncommutative Noetherian rings" , Wiley (1987) MR934572 |
| [a3] | A.J. Hahn, O.T. O'Meara, "The classical groups and 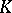 -theory" , Springer (1981) pp. §4.1 MR1007302 MR0842441 -theory" , Springer (1981) pp. §4.1 MR1007302 MR0842441 |
| [a4] | H. Bass, "Algebraic 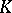 -theory" , Benjamin (1968) pp. Chapt. V, §3 MR249491 -theory" , Benjamin (1968) pp. Chapt. V, §3 MR249491 |
| [a5] | W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) |
Stable rank. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Stable_rank&oldid=12780