Difference between revisions of "Lieb-Thirring inequalities"
Ulf Rehmann (talk | contribs) m (moved Lieb–Thirring inequalities to Lieb-Thirring inequalities: ascii title) |
m (AUTOMATIC EDIT (latexlist): Replaced 148 formulas out of 153 by TEX code with an average confidence of 2.0 and a minimal confidence of 2.0.) |
||
| Line 1: | Line 1: | ||
| + | <!--This article has been texified automatically. Since there was no Nroff source code for this article, | ||
| + | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist | ||
| + | was used. | ||
| + | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. | ||
| + | |||
| + | Out of 153 formulas, 148 were replaced by TEX code.--> | ||
| + | |||
| + | {{TEX|semi-auto}}{{TEX|partial}} | ||
Inequalities concerning the negative | Inequalities concerning the negative | ||
| Line 9: | Line 17: | ||
[[Schrödinger equation|Schrödinger equation]]) | [[Schrödinger equation|Schrödinger equation]]) | ||
| − | + | \begin{equation*} H = - \Delta + V ( x ) \end{equation*} | |
on | on | ||
| − | + | $L ^ { 2 } ( \mathbf{R} ^ { n } )$, | |
| − | + | $n \geq 1$. | |
With | With | ||
| − | + | $e _ { 1 } \leq e _ { 2 } \leq \ldots < 0$ | |
denoting the negative eigenvalue(s) of | denoting the negative eigenvalue(s) of | ||
| − | + | $H$ | |
(if any), the Lieb–Thirring | (if any), the Lieb–Thirring | ||
| Line 29: | Line 37: | ||
inequalities state that for suitable | inequalities state that for suitable | ||
| − | + | $\gamma \geq 0$ | |
and constants | and constants | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l1201007.png" />, | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l1201007.png"/>, |
| − | + | \begin{equation} \tag{a1} \sum _ { j \geq 1 } | e _ { j } | ^ { \gamma } \leq L _ { \gamma , n } \int _ { \mathbf{R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x \end{equation} | |
with | with | ||
| − | + | $V _ { - } ( x ) : = \operatorname { max } \{ - V ( x ) , 0 \}$. | |
When | When | ||
| − | + | $\gamma = 0$, | |
the left-hand side is just the number of negative eigenvalues. Such an | the left-hand side is just the number of negative eigenvalues. Such an | ||
| Line 53: | Line 61: | ||
can hold if and only if | can hold if and only if | ||
| − | + | \begin{equation} \tag{a2} \left\{ \begin{array} { l l } { \gamma \geq \frac { 1 } { 2 } } & { \text { for } n= 1, } \\ { \gamma > 0 } & { \text { for }n = 2, } \\ { \gamma \geq 0 } & { \text { for } n\geq 3. } \end{array} \right. \end{equation} | |
The cases | The cases | ||
| − | + | $\gamma > 1 / 2$, | |
| − | + | $n = 1$, | |
| − | + | $\gamma > 0$, | |
| − | + | $n \geq 2$, | |
were established by | were established by | ||
| Line 81: | Line 89: | ||
The case | The case | ||
| − | + | $\gamma = 1 / 2$, | |
| − | + | $n = 1$, | |
was established by | was established by | ||
| Line 93: | Line 101: | ||
The case | The case | ||
| − | + | $\gamma = 0$, | |
| − | + | $n \geq 3$, | |
was established independently by | was established independently by | ||
| Line 125: | Line 133: | ||
for | for | ||
| − | + | $L _ { 0 , n }$ | |
is in | is in | ||
| Line 143: | Line 151: | ||
for | for | ||
| − | + | $\sum | e | ^ { \gamma }$, | |
which serves as a heuristic motivation for | which serves as a heuristic motivation for | ||
| Line 155: | Line 163: | ||
[[#References|[a14]]]): | [[#References|[a14]]]): | ||
| − | + | \begin{equation*} \sum _ { j \geq 1 } | e | ^ { \gamma } \approx \end{equation*} | |
| − | + | \begin{equation*} \approx ( 2 \pi ) ^ { - n } \int _ { {\bf R} ^ { n } \times {\bf R} ^ { n } } [ p ^ { 2 } + V ( x ) ] _ { - } ^ { \gamma } d p d x = \end{equation*} | |
| − | + | \begin{equation*} = L _ { \gamma , n } ^ { c } \int _ { {\bf R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x, \end{equation*} | |
with | with | ||
| − | + | \begin{equation*} L _ { \gamma , n } ^ { c } = 2 ^ { - n } \pi ^ { - n / 2 } \frac { \Gamma ( \gamma + 1 ) } { \Gamma ( \gamma + 1 + n / 2 ) }. \end{equation*} | |
Indeed, | Indeed, | ||
| − | + | $L _ { \gamma , n } ^ { c } < \infty$ | |
for all | for all | ||
| − | + | $\gamma \geq 0$, | |
whereas | whereas | ||
| Line 183: | Line 191: | ||
It is easy to prove (by considering | It is easy to prove (by considering | ||
| − | + | $V ( x ) = \lambda W ( x )$ | |
with | with | ||
| − | + | $W$ | |
smooth and | smooth and | ||
| − | + | $\lambda \rightarrow \infty$) | |
that | that | ||
| − | + | \begin{equation*} L _ { \gamma , n } \geq L _ { \gamma , n } ^ { c }. \end{equation*} | |
An interesting, and mostly open | An interesting, and mostly open | ||
| Line 207: | Line 215: | ||
of the constant | of the constant | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l12010032.png" />, | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l12010032.png"/>, |
especially to find those cases in which | especially to find those cases in which | ||
| − | + | $L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$. | |
M. Aizenman | M. Aizenman | ||
| Line 223: | Line 231: | ||
proved that the ratio | proved that the ratio | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l12010034.png" /> | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l12010034.png"/> |
is a monotonically non-increasing function of | is a monotonically non-increasing function of | ||
| − | + | $\gamma$. | |
Thus, if | Thus, if | ||
| − | + | $R _ { \Gamma , n } = 1$ | |
for some | for some | ||
| − | + | $\Gamma$, | |
then | then | ||
| − | + | $L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$ | |
for all | for all | ||
| − | + | $\gamma \geq \Gamma$. | |
The equality | The equality | ||
| − | + | $L _ { \frac { 3 } { 2 } ,\, n } = L _ { \frac { 3 } { 2 } ,\, n } ^ { c }$ | |
was proved for | was proved for | ||
| − | + | $n = 1$ | |
in | in | ||
| Line 259: | Line 267: | ||
and for | and for | ||
| − | + | $n > 1$ | |
in | in | ||
| Line 279: | Line 287: | ||
The following sharp constants are known: | The following sharp constants are known: | ||
| − | + | $L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$, | |
all | all | ||
| − | + | $\gamma \geq 3 / 2$, | |
[[#References|[a14]]], | [[#References|[a14]]], | ||
| Line 291: | Line 299: | ||
[[#References|[a2]]]; | [[#References|[a2]]]; | ||
| − | + | $L _ { 1 / 2,1 } = 1 / 2$, | |
[[#References|[a11]]]. | [[#References|[a11]]]. | ||
| Line 303: | Line 311: | ||
that | that | ||
| − | + | \begin{equation} \tag{a3} L _ { \gamma , 1 } = \frac { 1 } { \sqrt { \pi } ( \gamma - \frac { 1 } { 2 } ) } \frac { \Gamma ( \gamma + 1 ) } { \Gamma ( \gamma + 1 / 2 ) } \left( \frac { \gamma - \frac { 1 } { 2 } } { \gamma + \frac { 1 } { 2 } } \right) ^ { \gamma + 1 / 2 } \end{equation} | |
for | for | ||
| − | + | $1 / 2 < \gamma < 3 / 2$. | |
Instead of considering all the negative eigenvalues as in | Instead of considering all the negative eigenvalues as in | ||
| Line 315: | Line 323: | ||
one can consider just | one can consider just | ||
| − | + | $e_1$. | |
Then for | Then for | ||
| − | + | $\gamma$ | |
as in | as in | ||
| Line 325: | Line 333: | ||
(a2), | (a2), | ||
| − | + | \begin{equation*} | e _ { 1 } | ^ { \gamma } \leq L _ { \gamma , n } ^ { 1 } \int _ { \mathbf{R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x. \end{equation*} | |
Clearly, | Clearly, | ||
| − | + | $L _ { \gamma , n } ^ { 1 } \leq L _ { \gamma ,n }$, | |
but equality can hold, as in the cases | but equality can hold, as in the cases | ||
| − | + | $\gamma = 1 / 2$ | |
and | and | ||
| − | + | $3 / 2$ | |
for | for | ||
| − | + | $n = 1$. | |
Indeed, the conjecture in | Indeed, the conjecture in | ||
| Line 349: | Line 357: | ||
amounts to | amounts to | ||
| − | + | $L _ { \gamma , 1 } ^ { 1 } = L _ { \gamma , 1 }$ | |
for | for | ||
| − | + | $1 / 2 < \gamma < 3 / 2$. | |
The sharp value | The sharp value | ||
| Line 361: | Line 369: | ||
of | of | ||
| − | + | $L _ { \gamma , n} ^ { 1 }$ | |
is obtained by solving a differential equation | is obtained by solving a differential equation | ||
| Line 369: | Line 377: | ||
It has been conjectured that for | It has been conjectured that for | ||
| − | + | $n \geq 3$, | |
| − | + | $L _ { 0 ,\, n } = L _ { 0 ,\, n } ^ { 1 }$. | |
In any case, | In any case, | ||
| Line 385: | Line 393: | ||
showed that for all | showed that for all | ||
| − | + | $n$ | |
and all | and all | ||
| − | + | $\gamma < 1$, | |
| − | + | $L _ { \gamma , n } > L _ { \gamma , n } ^ { c }$. | |
The sharp constant | The sharp constant | ||
| − | + | $L _ { 0 , n } ^ { 1 }$, | |
| − | + | $n \geq 3$, | |
is related to the sharp constant | is related to the sharp constant | ||
| − | + | $S _ { n }$ | |
in the | in the | ||
| Line 407: | Line 415: | ||
Sobolev inequality | Sobolev inequality | ||
| − | + | \begin{equation} \tag{a4} \| \nabla f \| _ {{ L } ^ 2 ( \mathbf{R} ^ { n } ) } \geq S _ { n } \| f \| _ { L ^{ 2 n / ( n - 2 ) } ( \mathbf{R} ^ { n } ) } \end{equation} | |
by | by | ||
| − | + | $L _ { 0 , n } ^ { 1 } = ( S _ { n } ) ^ { - n }$. | |
By a | By a | ||
| Line 421: | Line 429: | ||
the case | the case | ||
| − | + | $\gamma = 1$ | |
in | in | ||
| Line 431: | Line 439: | ||
[[Laplace operator|Laplace operator]], | [[Laplace operator|Laplace operator]], | ||
| − | + | $\Delta$. | |
This bound is | This bound is | ||
| Line 449: | Line 457: | ||
Let | Let | ||
| − | + | $f _ { 1 } , f _ { 2 } , \ldots$ | |
be | be | ||
| Line 461: | Line 469: | ||
in | in | ||
| − | + | $L ^ { 2 } ( \mathbf{R} ^ { n } )$ | |
such that | such that | ||
| − | + | $\nabla f _ { j } \in L ^ { 2 } ( \mathbf{R} ^ { n } )$ | |
for all | for all | ||
| − | + | $j \geq 1$. | |
Associated with this sequence is a | Associated with this sequence is a | ||
| Line 475: | Line 483: | ||
"density" | "density" | ||
| − | + | \begin{equation} \tag{a5} \rho ( x ) = \sum _ { j \geq 1 } | f _ { j } ( x ) | ^ { 2 }. \end{equation} | |
Then, with | Then, with | ||
| − | + | $K _ { n } : = n ( 2 / L _ { 1 , n } ) ^ { 2 / n } ( n + 2 ) ^ { - 1 - 2 / n }$, | |
| − | + | \begin{equation} \tag{a6} \sum _ { j \geq 1 } \int _ { \mathbf{R} ^ { n } } | \nabla f _ { j } ( x ) | ^ { 2 } d x \geq K _ { n } \int _ { \mathbf{R} ^ { n } } \rho ( x ) ^ { 1 + 2 / n } d x. \end{equation} | |
This can be extended to | This can be extended to | ||
| Line 489: | Line 497: | ||
in | in | ||
| − | + | $L ^ { 2 } ( \mathbf{R} ^ { n N } )$. | |
If | If | ||
| − | + | $\Phi = \Phi ( x _ { 1 } , \dots , x _ { N } )$ | |
is such a function, one defines, for | is such a function, one defines, for | ||
| − | + | $x \in \mathbf{R} ^ { n }$, | |
| − | + | \begin{equation*} \rho ( x ) = N \int _ { \mathbf{R} ^ { n ( N - 1 ) } } | \Phi ( x , x _ { 2 } , \ldots , x _ { N } ) | ^ { 2 } d x _ { 2 } \ldots d x _ { N }. \end{equation*} | |
Then, if | Then, if | ||
| − | + | $\int _ { \mathbf{R} ^ { n N } } | \Phi | ^ { 2 } = 1$, | |
| − | + | \begin{equation} \tag{a7} \int _ { R ^ { n N } } | \nabla \Phi | ^ { 2 } \geq K _ { n } \int _ { {\bf R} ^ { n } } \rho ( x ) ^ { 1 + 2 / n } d x. \end{equation} | |
Note that the choice | Note that the choice | ||
| − | + | $\Phi = ( N ! ) ^ { - 1 / 2 } \operatorname { det } f _ { j } ( x _ { k } ) | _ { j , k = 1 } ^ { N }$ | |
with | with | ||
| − | + | $f_j$ | |
orthonormal reduces the general case | orthonormal reduces the general case | ||
| Line 525: | Line 533: | ||
If the conjecture | If the conjecture | ||
| − | + | $L _ { 1,3 } = L _ { 1,3 } ^ { c }$ | |
is correct, then the bound in | is correct, then the bound in | ||
| Line 553: | Line 561: | ||
Of course, | Of course, | ||
| − | + | $\int ( \nabla f ) ^ { 2 } = \int f ( - \Delta f )$. | |
Inequalities of the type | Inequalities of the type | ||
| Line 561: | Line 569: | ||
can be found for other powers of | can be found for other powers of | ||
| − | + | $- \Delta$ | |
than the first power. The first example of this kind, due to | than the first power. The first example of this kind, due to | ||
| Line 571: | Line 579: | ||
and one of the most important physically, is to replace | and one of the most important physically, is to replace | ||
| − | + | $- \Delta$ | |
by | by | ||
| − | + | $\sqrt { - \Delta }$ | |
in | in | ||
| − | + | $H$. | |
Then an inequality similar to | Then an inequality similar to | ||
| Line 587: | Line 595: | ||
holds with | holds with | ||
| − | + | $\gamma + n / 2$ | |
replaced by | replaced by | ||
| − | + | $\gamma + n$ | |
(and with a different | (and with a different | ||
| − | + | $L _ { \gamma , n _ { 1 }}$, | |
of course). Likewise there is an analogue of | of course). Likewise there is an analogue of | ||
| Line 603: | Line 611: | ||
with | with | ||
| − | + | $1 + 2 / n$ | |
replaced by | replaced by | ||
| − | + | $1 + 1 / n$. | |
All proofs of | All proofs of | ||
| Line 625: | Line 633: | ||
proceed by finding an upper bound to | proceed by finding an upper bound to | ||
| − | + | $N _ { E } ( V )$, | |
the number of eigenvalues of | the number of eigenvalues of | ||
| − | + | $H = - \Delta + V ( x )$ | |
that are below | that are below | ||
| − | + | $- E$. | |
Then, for | Then, for | ||
| − | + | $\gamma > 0$, | |
| − | + | \begin{equation*} \sum | e | ^ { \gamma } = \gamma \int _ { 0 } ^ { \infty } N _ { E } ( V ) E ^ { \gamma - 1 } d E. \end{equation*} | |
Assuming | Assuming | ||
| − | + | $V = - V _ { - }$ | |
(since | (since | ||
| − | + | $V _ { + }$ | |
only raises the eigenvalues), | only raises the eigenvalues), | ||
| − | + | $N _ { E } ( V )$ | |
is most accessible via the positive semi-definite | is most accessible via the positive semi-definite | ||
| Line 661: | Line 669: | ||
[[#References|[a4]]]) | [[#References|[a4]]]) | ||
| − | + | \begin{equation*} K _ { E } ( V ) = \sqrt { V _ { - } } ( - \Delta + E ) ^ { - 1 } \sqrt { V _ { - } }. \end{equation*} | |
| − | + | $e < 0$ | |
is an eigenvalue of | is an eigenvalue of | ||
| − | + | $H$ | |
if and only if | if and only if | ||
| − | + | $1$ | |
is an eigenvalue of | is an eigenvalue of | ||
| − | + | $K _ { |e| } ( V )$. | |
Furthermore, | Furthermore, | ||
| − | + | $K _ { E } ( V )$ | |
is | is | ||
| Line 687: | Line 695: | ||
that is monotone decreasing in | that is monotone decreasing in | ||
| − | + | $E$, | |
and hence | and hence | ||
| − | + | $N _ { E } ( V )$ | |
equals the number of eigenvalues of | equals the number of eigenvalues of | ||
| − | + | $K _ { E } ( V )$ | |
that are greater than | that are greater than | ||
| − | + | $1$. | |
An important generalization of | An important generalization of | ||
| Line 707: | Line 715: | ||
is to replace | is to replace | ||
| − | + | $- \Delta$ | |
in | in | ||
| − | + | $H$ | |
by | by | ||
| − | + | $| i \nabla + A ( x ) | ^ { 2 }$, | |
where | where | ||
| − | + | $A ( x )$ | |
is some arbitrary vector field in | is some arbitrary vector field in | ||
| − | + | ${\bf R} ^ { n }$ | |
(called a | (called a | ||
| Line 735: | Line 743: | ||
still holds, but it is not known if the sharp value of | still holds, but it is not known if the sharp value of | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l120100119.png" /> | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l120100119.png"/> |
changes. What is known is that all | changes. What is known is that all | ||
| Line 745: | Line 753: | ||
known values of | known values of | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l120100120.png" /> | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l120/l120100/l120100120.png"/> |
are unchanged. It is also known that | are unchanged. It is also known that | ||
| − | + | $( - \Delta + E ) ^ { - 1 }$, | |
as a kernel in | as a kernel in | ||
| − | + | $\mathbf{R} ^ { n } \times \mathbf{R} ^ { n }$, | |
is pointwise greater than the absolute value of the kernel | is pointwise greater than the absolute value of the kernel | ||
| − | + | $( | i \nabla + A | ^ { 2 } + E ) ^ { - 1 }$. | |
There is another family of inequalities for orthonormal functions, | There is another family of inequalities for orthonormal functions, | ||
| Line 773: | Line 781: | ||
As before, let | As before, let | ||
| − | + | $f _ { 1 } , \dots , f _ { N }$ | |
be | be | ||
| − | + | $N$ | |
orthonormal functions in | orthonormal functions in | ||
| − | + | $L ^ { 2 } ( \mathbf{R} ^ { n } )$ | |
and set | and set | ||
| − | + | \begin{equation*} u _ { j } = ( - \Delta + m ^ { 2 } ) ^ { - 1 / 2 } f _ { j }, \end{equation*} | |
| − | + | \begin{equation*} \rho ( x ) = \sum _ { j = 1 } ^ { N } | u _ { j } ( x ) | ^ { 2 }. \end{equation*} | |
| − | + | $u _ { j }$ | |
is a | is a | ||
| Line 795: | Line 803: | ||
[[Riesz potential|Riesz potential]] | [[Riesz potential|Riesz potential]] | ||
| − | ( | + | ($m = 0$) |
or a | or a | ||
| Line 801: | Line 809: | ||
[[Bessel potential|Bessel potential]] | [[Bessel potential|Bessel potential]] | ||
| − | ( | + | ($m > 0$) |
of | of | ||
| − | + | $f_j$. | |
If | If | ||
| − | + | $n = 1$ | |
and | and | ||
| − | + | $m > 0$, | |
then | then | ||
| − | + | $\rho \in C ^ { 0,1 / 2 } ( \mathbf{R} ^ { n } )$ | |
and | and | ||
| − | + | $\| \rho \| _ { L^\infty ( {\bf R} )} \leq L / m$. | |
If | If | ||
| − | + | $n = 2$ | |
and | and | ||
| − | + | $m > 0$, | |
then for all | then for all | ||
| − | + | $1 \leq p < \infty$, | |
| − | + | $\| \rho \| _ { L ^ { p } ( R ^ { 2 } ) } \leq B _ { p } m ^ { - 2 / p } N ^ { 1 / p }$. | |
If | If | ||
| − | + | $n \geq 3$, | |
| − | + | $p = n / ( n - 2 )$ | |
and | and | ||
| − | + | $m \geq 0$ | |
(including | (including | ||
| − | + | $m = 0$), | |
then | then | ||
| − | + | $\| \rho \| _ { L ^ { p } ( \mathbf{R} ^ { n } ) } \leq A _ { n } N ^ { 1 / p }$. | |
Here, | Here, | ||
| − | + | $L$, | |
| − | + | $B _ { p }$, | |
| − | + | $A _ { n }$ | |
are universal constants. Without the orthogonality, | are universal constants. Without the orthogonality, | ||
| − | + | $N ^ { 1 / p }$ | |
would have to be replaced by | would have to be replaced by | ||
| − | + | $N$. | |
Further generalizations are | Further generalizations are | ||
| Line 878: | Line 886: | ||
====References==== | ====References==== | ||
| − | <table>< | + | <table><tr><td valign="top">[a1]</td> <td valign="top"> |
R. Benguria, | R. Benguria, | ||
| Line 888: | Line 896: | ||
''Preprint'' | ''Preprint'' | ||
| − | (1999)</ | + | (1999)</td></tr><tr><td valign="top">[a2]</td> <td valign="top"> |
A. Laptev, | A. Laptev, | ||
| Line 898: | Line 906: | ||
''Acta Math.'' | ''Acta Math.'' | ||
| − | (in press 1999)</ | + | (in press 1999)</td></tr><tr><td valign="top">[a3]</td> <td valign="top"> |
M.A. Aizenman, | M.A. Aizenman, | ||
| Line 912: | Line 920: | ||
(1978) | (1978) | ||
| − | pp. 427–429</ | + | pp. 427–429</td></tr><tr><td valign="top">[a4]</td> <td valign="top"> |
B. Simon, | B. Simon, | ||
| Line 924: | Line 932: | ||
, Acad. Press | , Acad. Press | ||
| − | (1979)</ | + | (1979)</td></tr><tr><td valign="top">[a5]</td> <td valign="top"> |
Ph. Blanchard, | Ph. Blanchard, | ||
| Line 938: | Line 946: | ||
(1996) | (1996) | ||
| − | pp. 503–547</ | + | pp. 503–547</td></tr><tr><td valign="top">[a6]</td> <td valign="top"> |
E.H. Lieb, | E.H. Lieb, | ||
| Line 954: | Line 962: | ||
(1980) | (1980) | ||
| − | pp. 241–251</ | + | pp. 241–251</td></tr><tr><td valign="top">[a7]</td> <td valign="top"> |
E.H. Lieb, | E.H. Lieb, | ||
| Line 966: | Line 974: | ||
(1984) | (1984) | ||
| − | pp. 473–480</ | + | pp. 473–480</td></tr><tr><td valign="top">[a8]</td> <td valign="top"> |
E.H. Lieb, | E.H. Lieb, | ||
| Line 986: | Line 994: | ||
(1989) | (1989) | ||
| − | pp. 371–382</ | + | pp. 371–382</td></tr><tr><td valign="top">[a9]</td> <td valign="top"> |
E.H. Lieb, | E.H. Lieb, | ||
| Line 992: | Line 1,000: | ||
"An | "An | ||
| − | + | $L ^ { p }$ bound for the Riesz and Bessel potentials of orthonormal functions" | |
''J. Funct. Anal.'' | ''J. Funct. Anal.'' | ||
| Line 1,000: | Line 1,008: | ||
(1983) | (1983) | ||
| − | pp. 159–165</ | + | pp. 159–165</td></tr><tr><td valign="top">[a10]</td> <td valign="top"> |
G.V. Rosenbljum, | G.V. Rosenbljum, | ||
| Line 1,014: | Line 1,022: | ||
pp. 1012–1015 | pp. 1012–1015 | ||
| − | ((The details are given in: Izv. Vyss. Uchebn. Zaved. Mat. 164 (1976), 75-86 (English transl.: Soviet Math. (Izv. VUZ) 20 (1976), 63-71)))</ | + | ((The details are given in: Izv. Vyss. Uchebn. Zaved. Mat. 164 (1976), 75-86 (English transl.: Soviet Math. (Izv. VUZ) 20 (1976), 63-71)))</td></tr><tr><td valign="top">[a11]</td> <td valign="top"> |
D. Hundertmark, | D. Hundertmark, | ||
| Line 1,030: | Line 1,038: | ||
(1998) | (1998) | ||
| − | pp. 719–731</ | + | pp. 719–731</td></tr><tr><td valign="top">[a12]</td> <td valign="top"> |
B. Helffer, | B. Helffer, | ||
| Line 1,044: | Line 1,052: | ||
(1990) | (1990) | ||
| − | pp. 139–147</ | + | pp. 139–147</td></tr><tr><td valign="top">[a13]</td> <td valign="top"> |
I. Daubechies, | I. Daubechies, | ||
| Line 1,056: | Line 1,064: | ||
(1983) | (1983) | ||
| − | pp. 511–520</ | + | pp. 511–520</td></tr><tr><td valign="top">[a14]</td> <td valign="top"> |
E.H. Lieb, | E.H. Lieb, | ||
| Line 1,078: | Line 1,086: | ||
pp. 269–303 | pp. 269–303 | ||
| − | ((See also: W. Thirring (ed.), The stability of matter: from the atoms to stars, Selecta of E.H. Lieb, Springer, 1977))</ | + | ((See also: W. Thirring (ed.), The stability of matter: from the atoms to stars, Selecta of E.H. Lieb, Springer, 1977))</td></tr><tr><td valign="top">[a15]</td> <td valign="top"> |
M. Cwikel, | M. Cwikel, | ||
| Line 1,090: | Line 1,098: | ||
(1977) | (1977) | ||
| − | pp. 93–100</ | + | pp. 93–100</td></tr><tr><td valign="top">[a16]</td> <td valign="top"> |
T. Weidl, | T. Weidl, | ||
| Line 1,096: | Line 1,104: | ||
"On the Lieb–Thirring constants | "On the Lieb–Thirring constants | ||
| − | + | $L_{ \gamma , 1}$ for | |
| − | + | $\gamma \geq 1 / 2$" | |
''Comm. Math. Phys.'' | ''Comm. Math. Phys.'' | ||
| Line 1,108: | Line 1,116: | ||
(1996) | (1996) | ||
| − | pp. 135–146</ | + | pp. 135–146</td></tr></table> |
''Elliott H. Lieb'' | ''Elliott H. Lieb'' | ||
Copyright to this article is held by Elliott Lieb. | Copyright to this article is held by Elliott Lieb. | ||
Revision as of 17:01, 1 July 2020
Inequalities concerning the negative
eigenvalues of the
Schrödinger operator
(cf. also
\begin{equation*} H = - \Delta + V ( x ) \end{equation*}
on
$L ^ { 2 } ( \mathbf{R} ^ { n } )$,
$n \geq 1$.
With
$e _ { 1 } \leq e _ { 2 } \leq \ldots < 0$
denoting the negative eigenvalue(s) of
$H$
(if any), the Lieb–Thirring
inequalities state that for suitable
$\gamma \geq 0$
and constants
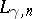 ,
,
\begin{equation} \tag{a1} \sum _ { j \geq 1 } | e _ { j } | ^ { \gamma } \leq L _ { \gamma , n } \int _ { \mathbf{R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x \end{equation}
with
$V _ { - } ( x ) : = \operatorname { max } \{ - V ( x ) , 0 \}$.
When
$\gamma = 0$,
the left-hand side is just the number of negative eigenvalues. Such an
inequality
(a1)
can hold if and only if
\begin{equation} \tag{a2} \left\{ \begin{array} { l l } { \gamma \geq \frac { 1 } { 2 } } & { \text { for } n= 1, } \\ { \gamma > 0 } & { \text { for }n = 2, } \\ { \gamma \geq 0 } & { \text { for } n\geq 3. } \end{array} \right. \end{equation}
The cases
$\gamma > 1 / 2$,
$n = 1$,
$\gamma > 0$,
$n \geq 2$,
were established by
E.H. Lieb
and
W.E. Thirring
in connection with their proof of
stability of matter.
The case
$\gamma = 1 / 2$,
$n = 1$,
was established by
T. Weidl
The case
$\gamma = 0$,
$n \geq 3$,
was established independently by
M. Cwikel
Lieb
and
G.V. Rosenbljum
by different methods and is known as the
CLR bound;
the smallest known
value (as of
1998)
for
$L _ { 0 , n }$
is in
[a6],
[a7].
Closely associated with the inequality
(a1)
is the
semi-classical approximation
for
$\sum | e | ^ { \gamma }$,
which serves as a heuristic motivation for
(a1).
It is
(cf.
[a14]):
\begin{equation*} \sum _ { j \geq 1 } | e | ^ { \gamma } \approx \end{equation*}
\begin{equation*} \approx ( 2 \pi ) ^ { - n } \int _ { {\bf R} ^ { n } \times {\bf R} ^ { n } } [ p ^ { 2 } + V ( x ) ] _ { - } ^ { \gamma } d p d x = \end{equation*}
\begin{equation*} = L _ { \gamma , n } ^ { c } \int _ { {\bf R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x, \end{equation*}
with
\begin{equation*} L _ { \gamma , n } ^ { c } = 2 ^ { - n } \pi ^ { - n / 2 } \frac { \Gamma ( \gamma + 1 ) } { \Gamma ( \gamma + 1 + n / 2 ) }. \end{equation*}
Indeed,
$L _ { \gamma , n } ^ { c } < \infty$
for all
$\gamma \geq 0$,
whereas
(a1)
holds only for the range given in
(a2).
It is easy to prove (by considering
$V ( x ) = \lambda W ( x )$
with
$W$
smooth and
$\lambda \rightarrow \infty$)
that
\begin{equation*} L _ { \gamma , n } \geq L _ { \gamma , n } ^ { c }. \end{equation*}
An interesting, and mostly open
(1998)
problem is to determine the sharp
value
of the constant
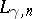 ,
,
especially to find those cases in which
$L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$.
M. Aizenman
and
Lieb
proved that the ratio
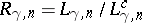
is a monotonically non-increasing function of
$\gamma$.
Thus, if
$R _ { \Gamma , n } = 1$
for some
$\Gamma$,
then
$L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$
for all
$\gamma \geq \Gamma$.
The equality
$L _ { \frac { 3 } { 2 } ,\, n } = L _ { \frac { 3 } { 2 } ,\, n } ^ { c }$
was proved for
$n = 1$
in
and for
$n > 1$
in
by
A. Laptev
and
Weidl.
(See also
[a1].)
The following sharp constants are known:
$L _ { \gamma , n } = L _ { \gamma , n } ^ { c }$,
all
$\gamma \geq 3 / 2$,
[a3],
[a2];
$L _ { 1 / 2,1 } = 1 / 2$,
There is strong support for the
conjecture
that
\begin{equation} \tag{a3} L _ { \gamma , 1 } = \frac { 1 } { \sqrt { \pi } ( \gamma - \frac { 1 } { 2 } ) } \frac { \Gamma ( \gamma + 1 ) } { \Gamma ( \gamma + 1 / 2 ) } \left( \frac { \gamma - \frac { 1 } { 2 } } { \gamma + \frac { 1 } { 2 } } \right) ^ { \gamma + 1 / 2 } \end{equation}
for
$1 / 2 < \gamma < 3 / 2$.
Instead of considering all the negative eigenvalues as in
(a1),
one can consider just
$e_1$.
Then for
$\gamma$
as in
(a2),
\begin{equation*} | e _ { 1 } | ^ { \gamma } \leq L _ { \gamma , n } ^ { 1 } \int _ { \mathbf{R} ^ { n } } V _ { - } ( x ) ^ { \gamma + n / 2 } d x. \end{equation*}
Clearly,
$L _ { \gamma , n } ^ { 1 } \leq L _ { \gamma ,n }$,
but equality can hold, as in the cases
$\gamma = 1 / 2$
and
$3 / 2$
for
$n = 1$.
Indeed, the conjecture in
(a3)
amounts to
$L _ { \gamma , 1 } ^ { 1 } = L _ { \gamma , 1 }$
for
$1 / 2 < \gamma < 3 / 2$.
The sharp value
(a3)
of
$L _ { \gamma , n} ^ { 1 }$
is obtained by solving a differential equation
It has been conjectured that for
$n \geq 3$,
$L _ { 0 ,\, n } = L _ { 0 ,\, n } ^ { 1 }$.
In any case,
B. Helffer
and
D. Robert
showed that for all
$n$
and all
$\gamma < 1$,
$L _ { \gamma , n } > L _ { \gamma , n } ^ { c }$.
The sharp constant
$L _ { 0 , n } ^ { 1 }$,
$n \geq 3$,
is related to the sharp constant
$S _ { n }$
in the
Sobolev inequality
\begin{equation} \tag{a4} \| \nabla f \| _ {{ L } ^ 2 ( \mathbf{R} ^ { n } ) } \geq S _ { n } \| f \| _ { L ^{ 2 n / ( n - 2 ) } ( \mathbf{R} ^ { n } ) } \end{equation}
by
$L _ { 0 , n } ^ { 1 } = ( S _ { n } ) ^ { - n }$.
By a
"duality argument"
the case
$\gamma = 1$
in
(a1)
can be converted into the following bound for the
$\Delta$.
This bound is
referred to as a
Lieb–Thirring kinetic energy inequality
and its most important application is to the
stability of matter
[a8],
Let
$f _ { 1 } , f _ { 2 } , \ldots$
be
any
orthonormal sequence (finite or infinite, cf. also
in
$L ^ { 2 } ( \mathbf{R} ^ { n } )$
such that
$\nabla f _ { j } \in L ^ { 2 } ( \mathbf{R} ^ { n } )$
for all
$j \geq 1$.
Associated with this sequence is a
"density"
\begin{equation} \tag{a5} \rho ( x ) = \sum _ { j \geq 1 } | f _ { j } ( x ) | ^ { 2 }. \end{equation}
Then, with
$K _ { n } : = n ( 2 / L _ { 1 , n } ) ^ { 2 / n } ( n + 2 ) ^ { - 1 - 2 / n }$,
\begin{equation} \tag{a6} \sum _ { j \geq 1 } \int _ { \mathbf{R} ^ { n } } | \nabla f _ { j } ( x ) | ^ { 2 } d x \geq K _ { n } \int _ { \mathbf{R} ^ { n } } \rho ( x ) ^ { 1 + 2 / n } d x. \end{equation}
This can be extended to
anti-symmetric functions
in
$L ^ { 2 } ( \mathbf{R} ^ { n N } )$.
If
$\Phi = \Phi ( x _ { 1 } , \dots , x _ { N } )$
is such a function, one defines, for
$x \in \mathbf{R} ^ { n }$,
\begin{equation*} \rho ( x ) = N \int _ { \mathbf{R} ^ { n ( N - 1 ) } } | \Phi ( x , x _ { 2 } , \ldots , x _ { N } ) | ^ { 2 } d x _ { 2 } \ldots d x _ { N }. \end{equation*}
Then, if
$\int _ { \mathbf{R} ^ { n N } } | \Phi | ^ { 2 } = 1$,
\begin{equation} \tag{a7} \int _ { R ^ { n N } } | \nabla \Phi | ^ { 2 } \geq K _ { n } \int _ { {\bf R} ^ { n } } \rho ( x ) ^ { 1 + 2 / n } d x. \end{equation}
Note that the choice
$\Phi = ( N ! ) ^ { - 1 / 2 } \operatorname { det } f _ { j } ( x _ { k } ) | _ { j , k = 1 } ^ { N }$
with
$f_j$
orthonormal reduces the general case
(a7)
to
(a6).
If the conjecture
$L _ { 1,3 } = L _ { 1,3 } ^ { c }$
is correct, then the bound in
(a7)
equals the
Thomas–Fermi kinetic energy Ansatz
(cf.
and hence it is a challenge to prove this conjecture. In the meantime,
see
[a7],
for the best available constants to date
(1998).
Of course,
$\int ( \nabla f ) ^ { 2 } = \int f ( - \Delta f )$.
Inequalities of the type
(a7)
can be found for other powers of
$- \Delta$
than the first power. The first example of this kind, due to
I. Daubechies
and one of the most important physically, is to replace
$- \Delta$
by
$\sqrt { - \Delta }$
in
$H$.
Then an inequality similar to
(a1)
holds with
$\gamma + n / 2$
replaced by
$\gamma + n$
(and with a different
$L _ { \gamma , n _ { 1 }}$,
of course). Likewise there is an analogue of
(a7)
with
$1 + 2 / n$
replaced by
$1 + 1 / n$.
All proofs of
(a1)
(except
and
actually
proceed by finding an upper bound to
$N _ { E } ( V )$,
the number of eigenvalues of
$H = - \Delta + V ( x )$
that are below
$- E$.
Then, for
$\gamma > 0$,
\begin{equation*} \sum | e | ^ { \gamma } = \gamma \int _ { 0 } ^ { \infty } N _ { E } ( V ) E ^ { \gamma - 1 } d E. \end{equation*}
Assuming
$V = - V _ { - }$
(since
$V _ { + }$
only raises the eigenvalues),
$N _ { E } ( V )$
is most accessible via the positive semi-definite
Birman–Schwinger kernel
(cf.
[a4])
\begin{equation*} K _ { E } ( V ) = \sqrt { V _ { - } } ( - \Delta + E ) ^ { - 1 } \sqrt { V _ { - } }. \end{equation*}
$e < 0$
is an eigenvalue of
$H$
if and only if
$1$
is an eigenvalue of
$K _ { |e| } ( V )$.
Furthermore,
$K _ { E } ( V )$
is
operator
that is monotone decreasing in
$E$,
and hence
$N _ { E } ( V )$
equals the number of eigenvalues of
$K _ { E } ( V )$
that are greater than
$1$.
An important generalization of
(a1)
is to replace
$- \Delta$
in
$H$
by
$| i \nabla + A ( x ) | ^ { 2 }$,
where
$A ( x )$
is some arbitrary vector field in
${\bf R} ^ { n }$
(called a
magnetic vector potential).
Then
(a1)
still holds, but it is not known if the sharp value of

changes. What is known is that all
presently
(1998)
known values of

are unchanged. It is also known that
$( - \Delta + E ) ^ { - 1 }$,
as a kernel in
$\mathbf{R} ^ { n } \times \mathbf{R} ^ { n }$,
is pointwise greater than the absolute value of the kernel
$( | i \nabla + A | ^ { 2 } + E ) ^ { - 1 }$.
There is another family of inequalities for orthonormal functions,
which is closely related to
(a1)
and to the CLR
bound
[a9].
As before, let
$f _ { 1 } , \dots , f _ { N }$
be
$N$
orthonormal functions in
$L ^ { 2 } ( \mathbf{R} ^ { n } )$
and set
\begin{equation*} u _ { j } = ( - \Delta + m ^ { 2 } ) ^ { - 1 / 2 } f _ { j }, \end{equation*}
\begin{equation*} \rho ( x ) = \sum _ { j = 1 } ^ { N } | u _ { j } ( x ) | ^ { 2 }. \end{equation*}
$u _ { j }$
is a
($m = 0$)
or a
($m > 0$)
of
$f_j$.
If
$n = 1$
and
$m > 0$,
then
$\rho \in C ^ { 0,1 / 2 } ( \mathbf{R} ^ { n } )$
and
$\| \rho \| _ { L^\infty ( {\bf R} )} \leq L / m$.
If
$n = 2$
and
$m > 0$,
then for all
$1 \leq p < \infty$,
$\| \rho \| _ { L ^ { p } ( R ^ { 2 } ) } \leq B _ { p } m ^ { - 2 / p } N ^ { 1 / p }$.
If
$n \geq 3$,
$p = n / ( n - 2 )$
and
$m \geq 0$
(including
$m = 0$),
then
$\| \rho \| _ { L ^ { p } ( \mathbf{R} ^ { n } ) } \leq A _ { n } N ^ { 1 / p }$.
Here,
$L$,
$B _ { p }$,
$A _ { n }$
are universal constants. Without the orthogonality,
$N ^ { 1 / p }$
would have to be replaced by
$N$.
Further generalizations are
possible
[a9].
References
| [a1] |
R. Benguria, M. Loss, "A simple proof of a theorem of Laptev and Weidl" Preprint (1999) |
| [a2] |
A. Laptev, T. Weidl, "Sharp Lieb–Thirring inequalities in high dimensions" Acta Math. (in press 1999) |
| [a3] |
M.A. Aizenman, E.H. Lieb, "On semiclassical bounds for eigenvalues of Schrödinger operators" Phys. Lett. , 66A (1978) pp. 427–429 |
| [a4] |
B. Simon, "Functional integration and quantum physics" , Pure Appl. Math. , 86 , Acad. Press (1979) |
| [a5] |
Ph. Blanchard, J. Stubbe, "Bound states for Schrödinger Hamiltonians: phase space methods and applications" Rev. Math. Phys. , 8 (1996) pp. 503–547 |
| [a6] |
E.H. Lieb, "The numbers of bound states of one-body Schrödinger operators and the Weyl problem" , Geometry of the Laplace Operator (Honolulu, 1979) , Proc. Symp. Pure Math. , 36 , Amer. Math. Soc. (1980) pp. 241–251 |
| [a7] |
E.H. Lieb, "On characteristic exponents in turbulence" Comm. Math. Phys. , 92 (1984) pp. 473–480 |
| [a8] |
E.H. Lieb, "Kinetic energy bounds and their applications to the stability of matter" H. Holden (ed.) A. Jensen (ed.) , Schrödinger Operators (Proc. Nordic Summer School, 1988) , Lecture Notes Physics , 345 , Springer (1989) pp. 371–382 |
| [a9] |
E.H. Lieb, "An $L ^ { p }$ bound for the Riesz and Bessel potentials of orthonormal functions" J. Funct. Anal. , 51 (1983) pp. 159–165 |
| [a10] |
G.V. Rosenbljum, "Distribution of the discrete spectrum of singular differential operators" Dokl. Akad. Nauk SSSR , 202 (1972) pp. 1012–1015 ((The details are given in: Izv. Vyss. Uchebn. Zaved. Mat. 164 (1976), 75-86 (English transl.: Soviet Math. (Izv. VUZ) 20 (1976), 63-71))) |
| [a11] |
D. Hundertmark, E.H. Lieb, L.E. Thomas, "A sharp bound for an eigenvalue moment of the one-dimensional Schrödinger operator" Adv. Theor. Math. Phys. , 2 (1998) pp. 719–731 |
| [a12] |
B. Helffer, D. Robert, "Riesz means of bound states and semi-classical limit connected with a Lieb–Thirring conjecture, II" Ann. Inst. H. Poincaré Phys. Th. , 53 (1990) pp. 139–147 |
| [a13] |
I. Daubechies, "An uncertainty principle for fermions with generalized kinetic energy" Comm. Math. Phys. , 90 (1983) pp. 511–520 |
| [a14] |
E.H. Lieb, W. Thirring, "Inequalities for the moments of the eigenvalues of the Schrödinger Hamiltonian and their relation to Sobolev inequalities" E. Lieb (ed.) B. Simon (ed.) A. Wightman (ed.) , Studies in Mathematical Physics: Essays in Honor of Valentine Bargmann , Princeton Univ. Press (1976) pp. 269–303 ((See also: W. Thirring (ed.), The stability of matter: from the atoms to stars, Selecta of E.H. Lieb, Springer, 1977)) |
| [a15] |
M. Cwikel, "Weak type estimates for singular values and the number of bound states of Schrödinger operators" Ann. Math. , 106 (1977) pp. 93–100 |
| [a16] |
T. Weidl, "On the Lieb–Thirring constants $L_{ \gamma , 1}$ for $\gamma \geq 1 / 2$" Comm. Math. Phys. , 178
(1996) pp. 135–146 |
Elliott H. Lieb
Copyright to this article is held by Elliott Lieb.
Lieb-Thirring inequalities. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lieb-Thirring_inequalities&oldid=22739