Difference between revisions of "Trace on a C*-algebra"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | t0935602.png | ||
| + | $#A+1 = 55 n = 1 | ||
| + | $#C+1 = 55 : ~/encyclopedia/old_files/data/T093/T.0903560 Trace on a \BMI C sup \ast\EMI\AAhalgebra | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | An additive functional | + | {{TEX|auto}} |
| + | {{TEX|done}} | ||
| + | |||
| + | '' $ A $'' | ||
| + | |||
| + | An additive functional $ f $ | ||
| + | on the set $ A ^ {+} $ | ||
| + | of positive elements of $ A $ | ||
| + | that takes values in $ [ 0, + \infty ] $, | ||
| + | is homogeneous with respect to multiplication by positive numbers and satisfies the condition $ f ( xx ^ {*} ) = f ( x ^ {*} x) $ | ||
| + | for all $ x \in A $. | ||
| + | A trace $ f $ | ||
| + | is said to be finite if $ f ( x) < + \infty $ | ||
| + | for all $ x \in A ^ {+} $, | ||
| + | and semi-finite if $ f ( x) = \sup \{ {f ( y) } : {y \in A, y \leq x, f( y) < + \infty } \} $ | ||
| + | for all $ x \in A ^ {+} $. | ||
| + | The finite traces on $ A $ | ||
| + | are the restrictions to $ A ^ {+} $ | ||
| + | of those positive linear functionals $ \phi $ | ||
| + | on $ A $ | ||
| + | such that $ \phi ( xy) = \phi ( yx) $ | ||
| + | for all $ x, y \in A $. | ||
| + | Let $ f $ | ||
| + | be a trace on $ A $, | ||
| + | let $ \mathfrak N _ {f} $ | ||
| + | be the set of elements $ x \in A $ | ||
| + | such that $ f ( xx ^ {*} ) < + \infty $, | ||
| + | and let $ \mathfrak M _ {f} $ | ||
| + | be the set of linear combinations of products of pairs of elements of $ \mathfrak N _ {f} $. | ||
| + | Then $ \mathfrak N _ {f} $ | ||
| + | and $ \mathfrak M _ {f} $ | ||
| + | are self-adjoint two-sided ideals of $ A $, | ||
| + | and there is a unique linear functional $ \phi $ | ||
| + | on $ \mathfrak M _ {f} $ | ||
| + | that coincides with $ f $ | ||
| + | on $ \mathfrak M _ {f} \cap A ^ {+} $. | ||
| + | Let $ f $ | ||
| + | be a lower semi-continuous semi-finite trace on a $ C ^ {*} $- | ||
| + | algebra $ A $. | ||
| + | Then the formula $ s ( x, y) = \phi ( y ^ {*} x) $ | ||
| + | defines a Hermitian form on $ \mathfrak N _ {f} $, | ||
| + | with respect to which the mapping $ \lambda _ {f} ( x): x \mapsto xy $ | ||
| + | of $ \mathfrak N _ {f} $ | ||
| + | into itself is continuous for any $ x \in A $. | ||
| + | Put $ N _ {f} = \{ {x \in \mathfrak N _ {f} } : {s ( x, x) = 0 } \} $, | ||
| + | and let $ H _ {f} $ | ||
| + | be the completion of the quotient space $ \mathfrak N _ {f} /N _ {f} $ | ||
| + | with respect to the scalar product defined by the form $ s $. | ||
| + | By passing to the quotient space and subsequent completion, the operators $ \lambda _ {f} ( x) $ | ||
| + | determine certain operators $ \pi _ {f} ( x) $ | ||
| + | on the Hilbert space $ H _ {f} $, | ||
| + | and the mapping $ x \mapsto \pi _ {f} ( x) $ | ||
| + | is a representation of the $ C ^ {*} $- | ||
| + | algebra $ A $ | ||
| + | in $ H _ {f} $. | ||
| + | The mapping $ f \mapsto \pi _ {f} $ | ||
| + | establishes a one-to-one correspondence between the set of lower semi-continuous semi-finite traces on $ A $ | ||
| + | and the set of representations of $ A $ | ||
| + | with a trace, defined up to quasi-equivalence. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093560/t09356058.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093560/t09356058.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | Cf. also [[C*-algebra| | + | Cf. also [[C*-algebra| $ C ^ {*} $- |
| + | algebra]]; [[Trace|Trace]]; [[Quasi-equivalent representations|Quasi-equivalent representations]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> O. Bratteli, D.W. Robinson, "Operator algebras and quantum statistical mechanics" , '''1''' , Springer (1979)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> O. Bratteli, D.W. Robinson, "Operator algebras and quantum statistical mechanics" , '''1''' , Springer (1979)</TD></TR></table> | ||
Revision as of 08:26, 6 June 2020
$ A $
An additive functional $ f $ on the set $ A ^ {+} $ of positive elements of $ A $ that takes values in $ [ 0, + \infty ] $, is homogeneous with respect to multiplication by positive numbers and satisfies the condition $ f ( xx ^ {*} ) = f ( x ^ {*} x) $ for all $ x \in A $. A trace $ f $ is said to be finite if $ f ( x) < + \infty $ for all $ x \in A ^ {+} $, and semi-finite if $ f ( x) = \sup \{ {f ( y) } : {y \in A, y \leq x, f( y) < + \infty } \} $ for all $ x \in A ^ {+} $. The finite traces on $ A $ are the restrictions to $ A ^ {+} $ of those positive linear functionals $ \phi $ on $ A $ such that $ \phi ( xy) = \phi ( yx) $ for all $ x, y \in A $. Let $ f $ be a trace on $ A $, let $ \mathfrak N _ {f} $ be the set of elements $ x \in A $ such that $ f ( xx ^ {*} ) < + \infty $, and let $ \mathfrak M _ {f} $ be the set of linear combinations of products of pairs of elements of $ \mathfrak N _ {f} $. Then $ \mathfrak N _ {f} $ and $ \mathfrak M _ {f} $ are self-adjoint two-sided ideals of $ A $, and there is a unique linear functional $ \phi $ on $ \mathfrak M _ {f} $ that coincides with $ f $ on $ \mathfrak M _ {f} \cap A ^ {+} $. Let $ f $ be a lower semi-continuous semi-finite trace on a $ C ^ {*} $- algebra $ A $. Then the formula $ s ( x, y) = \phi ( y ^ {*} x) $ defines a Hermitian form on $ \mathfrak N _ {f} $, with respect to which the mapping $ \lambda _ {f} ( x): x \mapsto xy $ of $ \mathfrak N _ {f} $ into itself is continuous for any $ x \in A $. Put $ N _ {f} = \{ {x \in \mathfrak N _ {f} } : {s ( x, x) = 0 } \} $, and let $ H _ {f} $ be the completion of the quotient space $ \mathfrak N _ {f} /N _ {f} $ with respect to the scalar product defined by the form $ s $. By passing to the quotient space and subsequent completion, the operators $ \lambda _ {f} ( x) $ determine certain operators $ \pi _ {f} ( x) $ on the Hilbert space $ H _ {f} $, and the mapping $ x \mapsto \pi _ {f} ( x) $ is a representation of the $ C ^ {*} $- algebra $ A $ in $ H _ {f} $. The mapping $ f \mapsto \pi _ {f} $ establishes a one-to-one correspondence between the set of lower semi-continuous semi-finite traces on $ A $ and the set of representations of $ A $ with a trace, defined up to quasi-equivalence.
References
| [1] | J. Dixmier, "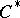 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
Comments
Cf. also $ C ^ {*} $- algebra; Trace; Quasi-equivalent representations.
References
| [a1] | O. Bratteli, D.W. Robinson, "Operator algebras and quantum statistical mechanics" , 1 , Springer (1979) |
Trace on a C*-algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Trace_on_a_C*-algebra&oldid=16757