Difference between revisions of "Stein space"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | s0876401.png | ||
| + | $#A+1 = 111 n = 1 | ||
| + | $#C+1 = 111 : ~/encyclopedia/old_files/data/S087/S.0807640 Stein space, | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
''holomorphically-complete space'' | ''holomorphically-complete space'' | ||
| − | A paracompact complex [[Analytic space|analytic space]] | + | A paracompact complex [[Analytic space|analytic space]] $ ( X, {\mathcal O}) $ |
| + | with the following properties: | ||
| − | 1) any compact analytic subset in | + | 1) any compact analytic subset in $ X $ |
| + | is finite (cf. [[Analytic set|Analytic set]] 6)); | ||
| − | 2) any compact set | + | 2) any compact set $ K \subset X $ |
| + | has an open neighbourhood $ W $ | ||
| + | in $ X $ | ||
| + | such that | ||
| − | + | $$ | |
| + | \left \{ { | ||
| + | x \in W } : {| f( x) | \leq \sup _ {z \in K } | f( z) | \textrm{ for } \textrm{ all } f \in | ||
| + | {\mathcal O}( X) } \right \} | ||
| + | $$ | ||
is compact (weak holomorphic convexity). | is compact (weak holomorphic convexity). | ||
| − | A complex manifold | + | A complex manifold $ M $ |
| + | is a Stein space if and only if $ M $ | ||
| + | is a [[Stein manifold|Stein manifold]]. A [[Complex space|complex space]] is a Stein space if and only if its reduction has this property. Any holomorphically-convex open subspace in a Stein space is a Stein space. A reduced complex space is a Stein space if and only if its normalization is a Stein space. Any closed analytic subspace in a Stein space, for instance in $ \mathbf C ^ {n} $, | ||
| + | is a Stein space. Any finite-dimensional Stein space has a proper injective holomorphic mapping (cf. [[Proper morphism|Proper morphism]]) into some $ \mathbf C ^ {n} $ | ||
| + | which is regular at every non-singular point. Any unramified covering of a Stein space is a Stein space. The direct product of two Stein spaces is a Stein space. In many cases a holomorphic fibre space whose base and fibres are Stein spaces is a Stein space (e.g. if the structure group is a complex Lie group with a finite number of connected components). However, there are holomorphic fibre spaces with fibre $ \mathbf C ^ {2} $ | ||
| + | and base $ \mathbf C $ | ||
| + | that are not Stein manifolds [[#References|[2]]]. | ||
| − | Let | + | Let $ {\mathcal F} $ |
| + | be a [[Coherent analytic sheaf|coherent analytic sheaf]] on a Stein space $ ( X, {\mathcal O}) $. | ||
| + | Then the following theorems $ A $ | ||
| + | and $ B $ | ||
| + | of H. Cartan (cf. [[Cartan theorem|Cartan theorem]]) hold: | ||
| − | A) The space | + | A) The space $ H ^ {0} ( X, {\mathcal F}) $ |
| + | generates the stalk $ {\mathcal F} _ {x} $ | ||
| + | of the sheaf $ {\mathcal F} $ | ||
| + | at any point $ x \in X $; | ||
| − | B) | + | B) $ H ^ {q} ( X, {\mathcal F}) = 0 $ |
| + | for all $ q > 0 $. | ||
| − | Conversely, if | + | Conversely, if $ H ^ {1} ( X, {\mathcal I} ) = 0 $ |
| + | for any coherent sheaf of ideals $ {\mathcal I} \subseteq {\mathcal O} $, | ||
| + | then $ X $ | ||
| + | is a Stein space. A domain $ D \subset \mathbf C ^ {n} $ | ||
| + | is a Stein manifold if and only if $ H ^ {1} ( D, {\mathcal O}) = \dots = H ^ {n-} 1 ( D, {\mathcal O}) = 0 $. | ||
| − | From the Cartan theorems it follows that on a Stein space the first Cousin problem is always solvable, and if | + | From the Cartan theorems it follows that on a Stein space the first Cousin problem is always solvable, and if $ H ^ {2} ( X, \mathbf Z ) = 0 $, |
| + | then the second Cousin problem is solvable as well (see [[Cousin problems|Cousin problems]]). On any Stein manifold $ X $ | ||
| + | the Poincaré problem, i.e. can any meromorphic function be represented in the form $ f/g $, | ||
| + | where $ f, g \in {\mathcal O} ( X) $, | ||
| + | $ g \neq 0 $, | ||
| + | is solvable. Furthermore, if $ H ^ {2} ( X, \mathbf Z ) = 0 $, | ||
| + | then $ f $ | ||
| + | and $ g $ | ||
| + | can be chosen in such a way that the germs $ f _ {x} , g _ {x} $ | ||
| + | at any point $ x \in X $ | ||
| + | are relatively prime. The group of divisor classes of an irreducible reduced Stein space $ X $ | ||
| + | is isomorphic to $ H ^ {2} ( X, \mathbf Z ) $. | ||
| + | For any $ n $- | ||
| + | dimensional Stein space $ X $, | ||
| + | the homology groups $ H _ {q} ( X, \mathbf Z ) = 0 $ | ||
| + | for $ q > n $, | ||
| + | and the group $ H _ {n} ( X, \mathbf Z ) $ | ||
| + | is torsion-free. If $ X $ | ||
| + | is a manifold, then $ X $ | ||
| + | is homotopy equivalent to an $ n $- | ||
| + | dimensional cell complex. On the other hand, for any countable Abelian group $ G $ | ||
| + | and any $ q \geq 1 $ | ||
| + | there is a [[Domain of holomorphy|domain of holomorphy]] $ D \subset \mathbf C ^ {2q+} 3 $ | ||
| + | such that $ H _ {q} ( D, \mathbf Z ) \cong G $. | ||
| − | An important trend in the theory of Stein spaces is connected with studies of the plurisubharmonic functions on them (see [[Levi problem|Levi problem]]; [[Pseudo-convex and pseudo-concave|Pseudo-convex and pseudo-concave]]). The basic result here is that a Stein space is characterized as a space on which there exists a strongly | + | An important trend in the theory of Stein spaces is connected with studies of the plurisubharmonic functions on them (see [[Levi problem|Levi problem]]; [[Pseudo-convex and pseudo-concave|Pseudo-convex and pseudo-concave]]). The basic result here is that a Stein space is characterized as a space on which there exists a strongly $ 1 $- |
| + | pseudoconvex function exhausting it. | ||
| − | Algebras of holomorphic functions | + | Algebras of holomorphic functions $ {\mathcal O} ( X) $ |
| + | on a Stein space $ X $( | ||
| + | so-called Stein algebras) have the following properties. For a maximal ideal $ I \subset {\mathcal O} ( X) $ | ||
| + | the following conditions are equivalent: $ I $ | ||
| + | is closed in $ {\mathcal O} ( X) $ | ||
| + | with respect to the topology of compact convergence; $ I = \{ {f \in {\mathcal O} ( X) } : {f( x) = 0 } \} $ | ||
| + | for some point $ x \in X $; | ||
| + | and $ I $ | ||
| + | is finitely generated. If $ X $ | ||
| + | is finite-dimensional, then each character $ \chi : {\mathcal O} ( X) \rightarrow \mathbf C $ | ||
| + | is of the form $ \chi ( f ) = f( x) $ | ||
| + | for some $ x \in X $. | ||
| + | If $ ( X, {\mathcal O} _ {X} ) $, | ||
| + | $ ( Y, {\mathcal O} _ {Y} ) $ | ||
| + | are two finite-dimensional Stein spaces with isomorphic algebras $ {\mathcal O} _ {X} ( X) \cong {\mathcal O} _ {Y} ( Y) $, | ||
| + | then $ ( X, {\mathcal O} _ {X} ) \cong ( Y, {\mathcal O} _ {Y} ) $; | ||
| + | moreover, any isomorphism $ {\mathcal O} _ {X} ( X) \rightarrow {\mathcal O} _ {Y} ( Y) $ | ||
| + | is continuous and is induced by some isomorphism $ Y \rightarrow X $ | ||
| + | of complex spaces. | ||
| − | A significant role in the theory of Stein spaces is played by the so-called Oka principle, which states that a problem in the class of analytic functions on a Stein space is solvable if and only if it is solvable in the class of continuous functions. The second Cousin problem satisfies this principle. The following statement is still more general: The classification of the principal analytic fibrations (cf. [[Principal analytic fibration|Principal analytic fibration]]) with as basis a given reduced Stein space | + | A significant role in the theory of Stein spaces is played by the so-called Oka principle, which states that a problem in the class of analytic functions on a Stein space is solvable if and only if it is solvable in the class of continuous functions. The second Cousin problem satisfies this principle. The following statement is still more general: The classification of the principal analytic fibrations (cf. [[Principal analytic fibration|Principal analytic fibration]]) with as basis a given reduced Stein space $ X $ |
| + | and as structure group a given complex Lie group $ G $, | ||
| + | coincides with the classification of the topological fibrations with the same basis and the same structure group. The groups of connected components in the groups of analytic and continuous functions $ X \rightarrow G $ | ||
| + | also coincide. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Grauert, R. Remmert, "Theory of Stein spaces" , Springer (1977) (Translated from German)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J.-P. Demailly, "Un example de fibré holomorphe non de Stein à fibre <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s087/s087640/s08764076.png" /> ayant pour base le disque ou le plan" ''Invent. Math.'' , '''48''' : 3 (1978) pp. 293–302</TD></TR><TR><TD valign="top">[3a]</TD> <TD valign="top"> A.L. Onishchik, "Stein spaces" ''J. Soviet Math.'' , '''4''' : 5 (1974) pp. 540–554 ''Itogi Nauk. i Tekhn. Algebra.Topol. Geom.'' , '''11''' (1974) pp. 125–151</TD></TR><TR><TD valign="top">[3b]</TD> <TD valign="top"> A.L. Onishchik, "Pseudoconvexity in the theory of complex spaces" ''J. Soviet Math.'' , '''14''' : 4 (1977) pp. 1363–1407 ''Itogi Nauk. i Tekhn. Algebra. Topol. Geom.'' , '''15''' (1977) pp. 93–171</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Grauert, R. Remmert, "Theory of Stein spaces" , Springer (1977) (Translated from German)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J.-P. Demailly, "Un example de fibré holomorphe non de Stein à fibre <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s087/s087640/s08764076.png" /> ayant pour base le disque ou le plan" ''Invent. Math.'' , '''48''' : 3 (1978) pp. 293–302</TD></TR><TR><TD valign="top">[3a]</TD> <TD valign="top"> A.L. Onishchik, "Stein spaces" ''J. Soviet Math.'' , '''4''' : 5 (1974) pp. 540–554 ''Itogi Nauk. i Tekhn. Algebra.Topol. Geom.'' , '''11''' (1974) pp. 125–151</TD></TR><TR><TD valign="top">[3b]</TD> <TD valign="top"> A.L. Onishchik, "Pseudoconvexity in the theory of complex spaces" ''J. Soviet Math.'' , '''14''' : 4 (1977) pp. 1363–1407 ''Itogi Nauk. i Tekhn. Algebra. Topol. Geom.'' , '''15''' (1977) pp. 93–171</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | Let | + | Let $ X = ( X, {\mathcal O} _ {X} ) $ |
| + | be a [[Complex space|complex space]]. Let $ {\mathcal n} ( {\mathcal O} _ {X} ) = \cup {\mathcal n} ( {\mathcal O} _ {x} ) $ | ||
| + | be the so-called nil radical of $ {\mathcal O} _ {X} $, | ||
| + | i.e. the union of the nil radicals of the stalks $ {\mathcal O} _ {x} $. | ||
| + | It is a coherent sheaf (of ideals). The space $ X _ { \mathop{\rm red} } = ( X, {\mathcal O} _ {X} / {\mathcal n} ( {\mathcal O} _ {X} )) $ | ||
| + | is called the reduction of $ ( X, {\mathcal O} _ {X} ) $, | ||
| + | as is the associated mapping $ X _ { \mathop{\rm red} } \rightarrow X $. | ||
| + | A complex space $ X $ | ||
| + | is called reduced at a point $ x \in X $ | ||
| + | if $ {\mathcal n} ( {\mathcal O} _ {x} ) = 0 $. | ||
| + | The space $ X $ | ||
| + | is called reduced if it is reduced at all its points (i.e. if $ X = X _ { \mathop{\rm red} } $). | ||
| − | The set | + | The set $ N \subset {\mathcal O} _ {X} $ |
| + | of elements not dividing zero is multiplicative (i.e. $ N $ | ||
| + | is open in $ {\mathcal O} _ {x} $; | ||
| + | $ 1 \in N $; | ||
| + | and $ a, b \in N $ | ||
| + | implies $ ab \in N $). | ||
| + | Hence $ {\mathcal M} = {\mathcal O} _ {N} $( | ||
| + | with $ {\mathcal M} _ {x} = ( {\mathcal O} _ {x} ) _ {N _ {x} } $, | ||
| + | $ x \in X $) | ||
| + | is a well-defined $ {\mathcal O} _ {X} $- | ||
| + | module. $ {\mathcal M} $ | ||
| + | is called the sheaf of germs of meromorphic functions on $ X $. | ||
| + | The complex space $ X $ | ||
| + | is called normal at $ x \in X $ | ||
| + | if $ X $ | ||
| + | is reduced at $ x $ | ||
| + | and $ {\mathcal O} _ {x} $ | ||
| + | is integrally closed in $ {\mathcal M} _ {x} $. | ||
| + | A complex space is called normal if it is normal at every point. The normalization theorem says that for each reduced complex space $ X $ | ||
| + | there are a normal complex space $ \widetilde{X} $ | ||
| + | and a finite surjective holomorphic mapping $ \zeta : \widetilde{X} \rightarrow X $. | ||
| + | The pair $ ( \widetilde{X} , \zeta ) $ | ||
| + | is called the normalization of $ X $. | ||
| + | It is uniquely defined up to analytic isomorphisms. | ||
| − | Finally, | + | Finally, $ ( X , {\mathcal O} _ {X} ) $ |
| + | is called irreducible at $ x $ | ||
| + | if $ {\mathcal O} _ {x} $ | ||
| + | is an integral domain, and irreducible if it is irreducible at all points. See [[#References|[1]]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> L. Kaup, B. Kaup, "Holomorphic functions of several variables" , de Gruyter (1983) (Translated from German)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Sect. C</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> L. Kaup, B. Kaup, "Holomorphic functions of several variables" , de Gruyter (1983) (Translated from German)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Sect. C</TD></TR></table> | ||
Latest revision as of 08:23, 6 June 2020
holomorphically-complete space
A paracompact complex analytic space $ ( X, {\mathcal O}) $ with the following properties:
1) any compact analytic subset in $ X $ is finite (cf. Analytic set 6));
2) any compact set $ K \subset X $ has an open neighbourhood $ W $ in $ X $ such that
$$ \left \{ { x \in W } : {| f( x) | \leq \sup _ {z \in K } | f( z) | \textrm{ for } \textrm{ all } f \in {\mathcal O}( X) } \right \} $$
is compact (weak holomorphic convexity).
A complex manifold $ M $ is a Stein space if and only if $ M $ is a Stein manifold. A complex space is a Stein space if and only if its reduction has this property. Any holomorphically-convex open subspace in a Stein space is a Stein space. A reduced complex space is a Stein space if and only if its normalization is a Stein space. Any closed analytic subspace in a Stein space, for instance in $ \mathbf C ^ {n} $, is a Stein space. Any finite-dimensional Stein space has a proper injective holomorphic mapping (cf. Proper morphism) into some $ \mathbf C ^ {n} $ which is regular at every non-singular point. Any unramified covering of a Stein space is a Stein space. The direct product of two Stein spaces is a Stein space. In many cases a holomorphic fibre space whose base and fibres are Stein spaces is a Stein space (e.g. if the structure group is a complex Lie group with a finite number of connected components). However, there are holomorphic fibre spaces with fibre $ \mathbf C ^ {2} $ and base $ \mathbf C $ that are not Stein manifolds [2].
Let $ {\mathcal F} $ be a coherent analytic sheaf on a Stein space $ ( X, {\mathcal O}) $. Then the following theorems $ A $ and $ B $ of H. Cartan (cf. Cartan theorem) hold:
A) The space $ H ^ {0} ( X, {\mathcal F}) $ generates the stalk $ {\mathcal F} _ {x} $ of the sheaf $ {\mathcal F} $ at any point $ x \in X $;
B) $ H ^ {q} ( X, {\mathcal F}) = 0 $ for all $ q > 0 $.
Conversely, if $ H ^ {1} ( X, {\mathcal I} ) = 0 $ for any coherent sheaf of ideals $ {\mathcal I} \subseteq {\mathcal O} $, then $ X $ is a Stein space. A domain $ D \subset \mathbf C ^ {n} $ is a Stein manifold if and only if $ H ^ {1} ( D, {\mathcal O}) = \dots = H ^ {n-} 1 ( D, {\mathcal O}) = 0 $.
From the Cartan theorems it follows that on a Stein space the first Cousin problem is always solvable, and if $ H ^ {2} ( X, \mathbf Z ) = 0 $, then the second Cousin problem is solvable as well (see Cousin problems). On any Stein manifold $ X $ the Poincaré problem, i.e. can any meromorphic function be represented in the form $ f/g $, where $ f, g \in {\mathcal O} ( X) $, $ g \neq 0 $, is solvable. Furthermore, if $ H ^ {2} ( X, \mathbf Z ) = 0 $, then $ f $ and $ g $ can be chosen in such a way that the germs $ f _ {x} , g _ {x} $ at any point $ x \in X $ are relatively prime. The group of divisor classes of an irreducible reduced Stein space $ X $ is isomorphic to $ H ^ {2} ( X, \mathbf Z ) $. For any $ n $- dimensional Stein space $ X $, the homology groups $ H _ {q} ( X, \mathbf Z ) = 0 $ for $ q > n $, and the group $ H _ {n} ( X, \mathbf Z ) $ is torsion-free. If $ X $ is a manifold, then $ X $ is homotopy equivalent to an $ n $- dimensional cell complex. On the other hand, for any countable Abelian group $ G $ and any $ q \geq 1 $ there is a domain of holomorphy $ D \subset \mathbf C ^ {2q+} 3 $ such that $ H _ {q} ( D, \mathbf Z ) \cong G $.
An important trend in the theory of Stein spaces is connected with studies of the plurisubharmonic functions on them (see Levi problem; Pseudo-convex and pseudo-concave). The basic result here is that a Stein space is characterized as a space on which there exists a strongly $ 1 $- pseudoconvex function exhausting it.
Algebras of holomorphic functions $ {\mathcal O} ( X) $ on a Stein space $ X $( so-called Stein algebras) have the following properties. For a maximal ideal $ I \subset {\mathcal O} ( X) $ the following conditions are equivalent: $ I $ is closed in $ {\mathcal O} ( X) $ with respect to the topology of compact convergence; $ I = \{ {f \in {\mathcal O} ( X) } : {f( x) = 0 } \} $ for some point $ x \in X $; and $ I $ is finitely generated. If $ X $ is finite-dimensional, then each character $ \chi : {\mathcal O} ( X) \rightarrow \mathbf C $ is of the form $ \chi ( f ) = f( x) $ for some $ x \in X $. If $ ( X, {\mathcal O} _ {X} ) $, $ ( Y, {\mathcal O} _ {Y} ) $ are two finite-dimensional Stein spaces with isomorphic algebras $ {\mathcal O} _ {X} ( X) \cong {\mathcal O} _ {Y} ( Y) $, then $ ( X, {\mathcal O} _ {X} ) \cong ( Y, {\mathcal O} _ {Y} ) $; moreover, any isomorphism $ {\mathcal O} _ {X} ( X) \rightarrow {\mathcal O} _ {Y} ( Y) $ is continuous and is induced by some isomorphism $ Y \rightarrow X $ of complex spaces.
A significant role in the theory of Stein spaces is played by the so-called Oka principle, which states that a problem in the class of analytic functions on a Stein space is solvable if and only if it is solvable in the class of continuous functions. The second Cousin problem satisfies this principle. The following statement is still more general: The classification of the principal analytic fibrations (cf. Principal analytic fibration) with as basis a given reduced Stein space $ X $ and as structure group a given complex Lie group $ G $, coincides with the classification of the topological fibrations with the same basis and the same structure group. The groups of connected components in the groups of analytic and continuous functions $ X \rightarrow G $ also coincide.
References
| [1] | H. Grauert, R. Remmert, "Theory of Stein spaces" , Springer (1977) (Translated from German) |
| [2] | J.-P. Demailly, "Un example de fibré holomorphe non de Stein à fibre 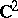 ayant pour base le disque ou le plan" Invent. Math. , 48 : 3 (1978) pp. 293–302 ayant pour base le disque ou le plan" Invent. Math. , 48 : 3 (1978) pp. 293–302 |
| [3a] | A.L. Onishchik, "Stein spaces" J. Soviet Math. , 4 : 5 (1974) pp. 540–554 Itogi Nauk. i Tekhn. Algebra.Topol. Geom. , 11 (1974) pp. 125–151 |
| [3b] | A.L. Onishchik, "Pseudoconvexity in the theory of complex spaces" J. Soviet Math. , 14 : 4 (1977) pp. 1363–1407 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 15 (1977) pp. 93–171 |
Comments
Let $ X = ( X, {\mathcal O} _ {X} ) $ be a complex space. Let $ {\mathcal n} ( {\mathcal O} _ {X} ) = \cup {\mathcal n} ( {\mathcal O} _ {x} ) $ be the so-called nil radical of $ {\mathcal O} _ {X} $, i.e. the union of the nil radicals of the stalks $ {\mathcal O} _ {x} $. It is a coherent sheaf (of ideals). The space $ X _ { \mathop{\rm red} } = ( X, {\mathcal O} _ {X} / {\mathcal n} ( {\mathcal O} _ {X} )) $ is called the reduction of $ ( X, {\mathcal O} _ {X} ) $, as is the associated mapping $ X _ { \mathop{\rm red} } \rightarrow X $. A complex space $ X $ is called reduced at a point $ x \in X $ if $ {\mathcal n} ( {\mathcal O} _ {x} ) = 0 $. The space $ X $ is called reduced if it is reduced at all its points (i.e. if $ X = X _ { \mathop{\rm red} } $).
The set $ N \subset {\mathcal O} _ {X} $ of elements not dividing zero is multiplicative (i.e. $ N $ is open in $ {\mathcal O} _ {x} $; $ 1 \in N $; and $ a, b \in N $ implies $ ab \in N $). Hence $ {\mathcal M} = {\mathcal O} _ {N} $( with $ {\mathcal M} _ {x} = ( {\mathcal O} _ {x} ) _ {N _ {x} } $, $ x \in X $) is a well-defined $ {\mathcal O} _ {X} $- module. $ {\mathcal M} $ is called the sheaf of germs of meromorphic functions on $ X $. The complex space $ X $ is called normal at $ x \in X $ if $ X $ is reduced at $ x $ and $ {\mathcal O} _ {x} $ is integrally closed in $ {\mathcal M} _ {x} $. A complex space is called normal if it is normal at every point. The normalization theorem says that for each reduced complex space $ X $ there are a normal complex space $ \widetilde{X} $ and a finite surjective holomorphic mapping $ \zeta : \widetilde{X} \rightarrow X $. The pair $ ( \widetilde{X} , \zeta ) $ is called the normalization of $ X $. It is uniquely defined up to analytic isomorphisms.
Finally, $ ( X , {\mathcal O} _ {X} ) $ is called irreducible at $ x $ if $ {\mathcal O} _ {x} $ is an integral domain, and irreducible if it is irreducible at all points. See [1].
References
| [a1] | L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973) |
| [a2] | L. Kaup, B. Kaup, "Holomorphic functions of several variables" , de Gruyter (1983) (Translated from German) |
| [a3] | R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Sect. C |
Stein space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Stein_space&oldid=17413