Difference between revisions of "Laplace transform"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | l0575401.png | ||
| + | $#A+1 = 178 n = 1 | ||
| + | $#C+1 = 178 : ~/encyclopedia/old_files/data/L057/L.0507540 Laplace transform | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
In the wide sense it is a Laplace integral of the form | In the wide sense it is a Laplace integral of the form | ||
| − | + | $$ \tag{1 } | |
| + | F ( p) = \int\limits _ { L } | ||
| + | f ( z) e ^ {- p z } d z , | ||
| + | $$ | ||
| − | where the integration is carried out over some contour | + | where the integration is carried out over some contour $ L $ |
| + | in the complex $ z $- | ||
| + | plane, which sets up a correspondence between a function $ f ( z) $, | ||
| + | defined on $ L $, | ||
| + | and an analytic function $ F ( p) $ | ||
| + | of the complex variable $ p = \sigma + i \tau $. | ||
| + | Many integrals of the form (1) were considered by P. Laplace (see [[#References|[1]]]). | ||
In the narrow sense the Laplace transform is understood to be the one-sided Laplace transform | In the narrow sense the Laplace transform is understood to be the one-sided Laplace transform | ||
| − | + | $$ \tag{2 } | |
| + | F ( p) = L [ f] ( p) = \int\limits _ { 0 } ^ \infty | ||
| + | f ( t) e ^ {- p t } d t , | ||
| + | $$ | ||
so called to distinguish it from the two-sided Laplace transform | so called to distinguish it from the two-sided Laplace transform | ||
| − | + | $$ \tag{3 } | |
| + | F ( p) = L [ f] ( p) = \int\limits _ {- \infty } ^ \infty | ||
| + | f ( t) e ^ {- p t } d t . | ||
| + | $$ | ||
| − | The Laplace transform is a special kind of [[Integral transform|integral transform]]; transforms of the form (2) or (3) are closely connected with the [[Fourier transform|Fourier transform]]. The two-sided Laplace transform (3) can be regarded as the Fourier transform of the function | + | The Laplace transform is a special kind of [[Integral transform|integral transform]]; transforms of the form (2) or (3) are closely connected with the [[Fourier transform|Fourier transform]]. The two-sided Laplace transform (3) can be regarded as the Fourier transform of the function $ f ( t) e ^ {- \sigma t } $, |
| + | and the one-sided Laplace transform (2) can be regarded as the Fourier transform of the function $ \phi ( t) $ | ||
| + | equal to $ f ( t) e ^ {- \sigma t } $ | ||
| + | for $ 0 < t < \infty $ | ||
| + | and equal to zero for $ - \infty < t < 0 $. | ||
| − | The complex locally summable integrand | + | The complex locally summable integrand $ f ( t) $ |
| + | is called the original function, or simply the original; in applications it is often convenient to treat the variable $ t $ | ||
| + | as time. The function $ F ( p) = L [ f] ( p) $ | ||
| + | is called the Laplace transform of the original $ f ( t) $. | ||
| + | Generally speaking, the integral (2) is understood to be conditionally convergent at infinity. A priori, three cases are possible: 1) there is a real number $ \sigma _ {c} $ | ||
| + | such that the integral (2) converges for $ \mathop{\rm Re} p = \sigma > \sigma _ {c} $ | ||
| + | and diverges for $ \mathop{\rm Re} p = \sigma < \sigma _ {c} $; | ||
| + | this number $ \sigma _ {c} $ | ||
| + | is called the abscissa of (conditional) convergence; 2) the integral (2) converges for all $ p $, | ||
| + | in which case one puts $ \sigma _ {c} = - \infty $; | ||
| + | and 3) the integral (2) diverges for all $ p $, | ||
| + | in which case one puts $ \sigma _ {c} = + \infty $. | ||
| + | If $ \sigma _ {c} < + \infty $, | ||
| + | then the integral (2) represents a single-valued analytic function $ F ( p) $ | ||
| + | in the half-plane of convergence $ \mathop{\rm Re} p > \sigma _ {c} $. | ||
| + | One usually restricts oneself to the consideration of absolutely convergent integrals (2). The greatest lower bound of those $ \sigma $ | ||
| + | for which the integral | ||
| − | + | $$ | |
| + | \int\limits _ { 0 } ^ \infty | f ( t) | e ^ {- \sigma t } d t | ||
| + | $$ | ||
| − | exists, is called the abscissa of absolute convergence | + | exists, is called the abscissa of absolute convergence $ \sigma _ {a} $, |
| + | $ \sigma _ {c} \leq \sigma _ {a} $. | ||
| + | If $ a $ | ||
| + | is the lower bound of those $ \sigma $ | ||
| + | for which $ | f ( t) | = O ( e ^ {\sigma t } ) $, | ||
| + | $ t \rightarrow \infty $, | ||
| + | then $ \sigma _ {a} = a $; | ||
| + | the number $ a $ | ||
| + | is sometimes called the index of growth of $ f ( t) $. | ||
| − | Under certain additional conditions | + | Under certain additional conditions $ f ( t) $ |
| + | can be uniquely restored from its Laplace transform $ F ( p) $. | ||
| + | For example, if $ f ( t) $ | ||
| + | has bounded variation in a neighbourhood of $ t _ {0} $ | ||
| + | or if $ f ( t) $ | ||
| + | is piecewise smooth, then the inversion formula for the Laplace transform holds: | ||
| − | + | $$ \tag{4 } | |
| + | f ^ \circ ( t _ {0} ) = \ | ||
| − | + | \frac{f ( t _ {0} + 0 ) + f ( t _ {0} - 0 ) }{2\ } | |
| + | = | ||
| + | $$ | ||
| + | |||
| + | $$ | ||
| + | = \ | ||
| + | |||
| + | \frac{1}{2 \pi i } | ||
| + | \lim\limits _ {R \rightarrow \infty } | ||
| + | \int\limits _ {\sigma - i R } ^ { \sigma + i R } F ( p) | ||
| + | e ^ {pt _ {0} } d p ,\ \sigma > \sigma _ {a} . | ||
| + | $$ | ||
Formulas (2) and (4) make it possible to obtain a number of relations between operations carried out over originals and transforms, and also to obtain a table of transforms for frequently occurring originals. All this constitutes an elementary part of [[Operational calculus|operational calculus]]. | Formulas (2) and (4) make it possible to obtain a number of relations between operations carried out over originals and transforms, and also to obtain a table of transforms for frequently occurring originals. All this constitutes an elementary part of [[Operational calculus|operational calculus]]. | ||
| Line 31: | Line 105: | ||
In mathematical physics there are important applications of the multi-dimensional Laplace transform | In mathematical physics there are important applications of the multi-dimensional Laplace transform | ||
| − | + | $$ \tag{5 } | |
| + | F ( p) = \int\limits _ {C _ {+} } f ( t) e ^ {- ( p , t ) } d t , | ||
| + | $$ | ||
| − | where | + | where $ t = ( t _ {1} \dots t _ {n} ) $ |
| + | is a point of the $ n $- | ||
| + | dimensional Euclidean space $ \mathbf R ^ {n} $, | ||
| + | $ p = ( p _ {1} \dots p _ {n} ) = \sigma + i \tau = ( \sigma _ {1} \dots \sigma _ {n} ) + i ( \tau _ {1} \dots \tau _ {n} ) $ | ||
| + | is a point of the complex space $ \mathbf C ^ {n} $, | ||
| + | $ n \geq 1 $, | ||
| − | + | $$ | |
| + | ( p , t ) = ( \sigma , t ) + i ( \tau , t) = p _ {1} t _ {1} + \dots | ||
| + | + p _ {n} t _ {n} $$ | ||
| − | is the scalar product and | + | is the scalar product and $ d t = d t _ {1} \dots d t _ {n} $ |
| + | is the volume element in $ \mathbf R ^ {n} $. | ||
| + | The complex function $ f ( t) $ | ||
| + | in (5) is defined and locally summable in the domain of integration $ C _ {+} = \{ {t \in \mathbf R ^ {n} } : {t _ {j} > 0, j = 1 \dots n } \} $, | ||
| + | the positive octant of $ \mathbf R ^ {n} $. | ||
| + | If $ f ( t) $ | ||
| + | is bounded in $ C _ {+} $, | ||
| + | then the integral (5) exists at all points $ p \in \mathbf C ^ {n} $ | ||
| + | that satisfy the condition $ \mathop{\rm Re} ( p , t ) > 0 $, | ||
| + | $ t \in C _ {+} $, | ||
| + | which again determines the positive octant $ S = \{ {\sigma \in \mathbf R ^ {n} } : {\sigma _ {j} > 0, j = 1 \dots n } \} $. | ||
| + | The integral (5) determines a holomorphic function of the complex variable $ p = ( p _ {1} \dots p _ {n} ) $ | ||
| + | in the tube domain $ T ^ {S} = S + i \mathbf R ^ {n} = \{ {p = \sigma + i \tau \in \mathbf C ^ {n} } : {\sigma \in S, \tau \in \mathbf R ^ {n} } \} $ | ||
| + | in $ \mathbf C ^ {n} $ | ||
| + | with base $ S $. | ||
| + | In the more general case, for the domain of integration $ C _ {+} $ | ||
| + | in (5) and the base $ S $ | ||
| + | of the tube domain one can take any pair of conjugate closed convex acute-angled cones in $ \mathbf R ^ {n} $ | ||
| + | with vertex at the origin. For $ n = 1 $ | ||
| + | formula (5) becomes (2), $ C _ {+} = \{ {t \in \mathbf R } : {t > 0 } \} $ | ||
| + | becomes the positive semi-axis and $ T ^ {S} = \{ {p = \sigma + i \tau \in \mathbf C } : {\sigma > 0 } \} $ | ||
| + | becomes the right half-plane. The Laplace transform (5) is defined and holomorphic for functions $ f ( t) $ | ||
| + | of much wider classes, for example, for all rapidly-decreasing functions that constitute the class $ {\mathcal S} = {\mathcal S} ( \mathbf R ^ {n} ) $, | ||
| + | that is, for infinitely differentiable functions $ f ( t) $ | ||
| + | in $ \mathbf R ^ {n} $ | ||
| + | that decrease as $ | t | \rightarrow \infty $ | ||
| + | together with all their derivatives faster than any power of $ | t | ^ {-} 1 $. | ||
| + | Elementary properties of the Laplace transform, with corresponding changes, remain true for the multi-dimensional case. | ||
| − | A generalization of the Laplace transform is the Laplace transform of measures and, in general, of generalized functions. The theory of the Laplace transform of generalized functions has been most completely developed for the class | + | A generalization of the Laplace transform is the Laplace transform of measures and, in general, of generalized functions. The theory of the Laplace transform of generalized functions has been most completely developed for the class $ {\mathcal S} ^ \prime = {\mathcal S} ^ \prime ( \mathbf R ^ {n} ) $, |
| + | which is important in mathematical physics, of generalized functions of slow growth, defined as linear continuous functionals on the space of rapidly-decreasing test functions $ {\mathcal S} = {\mathcal S} ( \mathbf R ^ {n} ) $. | ||
| + | The Laplace transform $ L [ g] $ | ||
| + | of a generalized function of slow growth $ g \in {\mathcal S} ^ \prime $ | ||
| + | is again a generalized function of slow growth, $ L [ g] \in {\mathcal S} ^ \prime $. | ||
==Numerical Laplace transformation.== | ==Numerical Laplace transformation.== | ||
| − | This is a numerical realization of the transform (2) that takes the original < | + | This is a numerical realization of the transform (2) that takes the original $ f ( t) $, |
| + | $ 0 < t < \infty $, | ||
| + | into the transform $ F ( p) $, | ||
| + | $ p = \sigma + i \tau $, | ||
| + | and also the numerical inversion of the Laplace transform, that is, the numerical determination of $ f ( t) $ | ||
| + | from the integral equation (2) or from the inversion formula (4). | ||
The need to apply the numerical Laplace transform arises as a consequence of the fact that the tables of originals and transforms cover by no means all cases occurring in practice, and also as a consequence of the fact that the original or the transform is frequently expressed by formulas that are too complicated and inconvenient for applications. | The need to apply the numerical Laplace transform arises as a consequence of the fact that the tables of originals and transforms cover by no means all cases occurring in practice, and also as a consequence of the fact that the original or the transform is frequently expressed by formulas that are too complicated and inconvenient for applications. | ||
| − | In the case of real values of | + | In the case of real values of $ p $, |
| + | formula (2) can be reduced, under certain additional assumptions, to an integral with Laguerre weight: | ||
| − | + | $$ \tag{6 } | |
| + | F ( p) = | ||
| + | \frac{1}{p} | ||
| + | \int\limits _ { 0 } ^ \infty | ||
| + | x ^ {s} e ^ {-} x \phi ( x) d x | ||
| + | $$ | ||
| − | for some | + | for some $ s \geq 0 $. |
| + | Under certain conditions a Laplace transform reduces to the integral (6) for complex $ p $( | ||
| + | see [[#References|[9]]]). | ||
To calculate the integral in (6) one can use the quadrature formula | To calculate the integral in (6) one can use the quadrature formula | ||
| − | + | $$ \tag{7 } | |
| + | \int\limits _ { 0 } ^ \infty x ^ {s} e ^ {-} x | ||
| + | \phi ( x) d x \approx \sum _ { k= } 1 ^ { n } | ||
| + | A _ {k} ^ {(} s) \phi ( x _ {k} ^ {(} s) ) , | ||
| + | $$ | ||
| + | |||
| + | where the coefficients $ A _ {k} ^ {(} s) $ | ||
| + | and the points $ x _ {k} ^ {(} s) $ | ||
| + | are chosen in such a way that the equality (7) for fixed $ n $ | ||
| + | is exact either for all polynomials of degree $ \leq 2 n - 1 $ | ||
| + | or for some system of rational functions, depending on the properties of $ \phi ( x) $. | ||
| + | The coefficients $ A _ {k} ^ {(} s) $ | ||
| + | and the points $ x _ {k} ^ {(} s) $ | ||
| + | for such quadrature formulas have been calculated for many values of $ s $( | ||
| + | see [[#References|[9]]]–[[#References|[11]]]). | ||
| + | |||
| + | The problem of inverting the Laplace transform, as a problem of finding a solution $ f ( x) $ | ||
| + | of the integral equation of the first kind (2), concerns a class of [[Ill-posed problems|ill-posed problems]] and can be solved, in particular, by means of a regularizing algorithm. | ||
| − | + | The problem of numerical inversion of a Laplace transform can also be solved by methods based on the expansion of the original in a series of functions. Here, in the first place, one can carry out an expansion in a power series, a generalized power series, a series of exponential functions, and also series of orthogonal functions, in particular, in Chebyshev, Legendre, Jacobi, or Laguerre polynomials. The problem of expanding the original in a series of Chebyshev, Legendre or Jacobi polynomials in its final form reduces to the problem of moments on a finite interval. Suppose one knows the Laplace transform $ F ( p) $ | |
| + | of the function $ \beta ( t) f ( t) $: | ||
| − | + | $$ | |
| + | F ( p) = \int\limits _ { 0 } ^ \infty | ||
| + | e ^ {-} pt \beta ( t) f ( t) d t , | ||
| + | $$ | ||
| − | + | where $ f ( t) $ | |
| + | is the unknown function and $ \beta ( t) $ | ||
| + | is a non-negative integrable function on $ [ 0 , \infty ] $. | ||
| + | Assume that $ f ( t) $ | ||
| + | is integrable on any finite interval $ [ 0 , T ] $ | ||
| + | and belongs to the class $ L _ {2} ( \beta ( t) , [ 0 , \infty )) $. | ||
| + | From the transform $ F ( p) $ | ||
| + | of $ \beta ( t) f ( t) $ | ||
| + | the function $ f ( t) $ | ||
| + | can be constructed as a series in shifted Jacobi polynomials, in particular, in shifted Legendre polynomials and Chebyshev polynomials of the first and second kind, the coefficients $ a _ {k} $ | ||
| + | of which are calculated from the formula | ||
| − | + | $$ | |
| + | a _ {k} = \sum _ { i= } 0 ^ { k } | ||
| + | \alpha _ {i} ^ {(} k) F ( i) , | ||
| + | $$ | ||
| − | where | + | where the $ \alpha _ {i} ^ {(} k) $ |
| + | are the coefficients of the shifted Legendre polynomials or Chebyshev polynomials of the first and second kind, respectively, written in the form $ \sum _ {i=} 1 ^ {k} \alpha _ {i} ^ {(} k) x ^ {i} $( | ||
| + | see [[#References|[4]]]). | ||
| − | + | Suppose one is given the Laplace transform $ F ( p) $ | |
| + | of the function $ f ( t) $ | ||
| + | and that $ f ( t) $ | ||
| + | satisfies the condition | ||
| − | + | $$ | |
| + | \int\limits _ { 0 } ^ \infty e ^ {-} t t ^ \lambda | ||
| + | | f ( t) | ^ {2} d t < \infty ,\ \ | ||
| + | \lambda > - 1 . | ||
| + | $$ | ||
| − | + | Then $ f ( t) $ | |
| + | can be expanded in a series of generalized Laguerre polynomials, | ||
| − | + | $$ | |
| + | f ( t) = t ^ \lambda \sum _ { k= } 0 ^ \infty | ||
| + | a _ {k} | ||
| + | \frac{k ! }{\Gamma ( k + \lambda + 1 ) } | ||
| − | + | L _ {k} ^ {( \lambda ) } ( t) , | |
| + | $$ | ||
| − | + | which converges to $ f ( t) $ | |
| + | in the mean. The coefficients $ a _ {k} $ | ||
| + | of this series are calculated from the formula | ||
| − | + | $$ | |
| + | a _ {k} = \ | ||
| − | + | \frac{( - 1 ) ^ {k} }{k ! } | |
| + | |||
| + | \frac{d ^ {k} }{d z ^ {k} } | ||
| + | |||
| + | \left . \left \{ | ||
| + | |||
| + | \frac{1}{z ^ {\lambda + 1 } } | ||
| + | F | ||
| + | \left ( | ||
| + | \frac{1}{z} | ||
| + | \right ) | ||
| + | \right \} \right | _ {z = 1 } . | ||
| + | $$ | ||
Another method of inverting the Laplace transform is to construct quadrature formulas for the inversion integral (4). | Another method of inverting the Laplace transform is to construct quadrature formulas for the inversion integral (4). | ||
| − | The transform | + | The transform $ F ( p) $ |
| + | tends to zero if the point $ p $ | ||
| + | tends to infinity in such a way that $ \mathop{\rm Re} p $ | ||
| + | tends to infinity. Assume that $ F ( p) $ | ||
| + | decreases polynomially, that is, $ F ( p) $ | ||
| + | can be expressed in the form | ||
| − | + | $$ | |
| + | F ( p) = | ||
| + | \frac{1}{p ^ {s} } | ||
| + | \phi ( p) ,\ \ | ||
| + | s > 0 , | ||
| + | $$ | ||
| − | with | + | with $ \phi ( p) $ |
| + | regular in the half-plane $ \mathop{\rm Re} p > \sigma _ {a} $ | ||
| + | and continuous for $ \mathop{\rm Re} p \geq \sigma _ {a} $. | ||
| + | The integral (4) has the form | ||
| − | + | $$ \tag{8 } | |
| + | f ( t) = | ||
| + | \frac{1}{2 \pi i } | ||
| − | + | \int\limits _ {\sigma - i \infty } ^ { \sigma + i \infty } | |
| + | e ^ {pt} p ^ {-} s \phi ( p) d p . | ||
| + | $$ | ||
| − | + | For the integral (8) an interpolation quadrature formula has been constructed, based on the interpolation of $ \phi ( p) $ | |
| + | by polynomials in $ 1 / p $: | ||
| − | + | $$ \tag{9 } | |
| + | f ( t) = \sum _ { k= } 0 ^ { n } | ||
| + | A _ {k} ^ {(} s) ( t) \phi ( p _ {k} ) + R _ {n} , | ||
| + | $$ | ||
| − | + | where the $ p _ {k} $ | |
| + | are the interpolation points, which are arbitrary and situated to the right of the line $ \mathop{\rm Re} p = \sigma _ {a} $, | ||
| + | $ R _ {n} $ | ||
| + | is the remainder term of the formula, and | ||
| − | + | $$ | |
| + | A _ {k} ^ {(} s) ( t) = \ | ||
| + | \sum _ { j= } 0 ^ { n } | ||
| − | + | \frac{a _ {kj} t ^ {s+} j- 1 }{\Gamma ( s + j ) } | |
| + | . | ||
| + | $$ | ||
| − | + | The coefficients $ a _ {kj} $ | |
| + | depend only on the chosen points $ p _ {k} $ | ||
| + | and for some methods of choosing them (in particular, for equidistant points) they have been calculated (see [[#References|[12]]]). The problem of investigating the convergence of interpolation quadrature formulas consists in finding relations between the properties of $ \phi ( p) $ | ||
| + | and the points $ p _ {k} $ | ||
| + | for which one can check that the remainder term $ R _ {n} $ | ||
| + | in (9) tends to zero. This problem has been solved for certain concrete points $ p _ {k} $ | ||
| + | and for certain special classes of functions $ \phi ( p) $( | ||
| + | see [[#References|[13]]]). | ||
| − | + | For the integral (4) one can construct quadrature formulas of the highest degree of accuracy in the class of rational functions of special form. In order that the parameters of the formula do not depend on $ \sigma _ {a} $ | |
| + | and $ t $, | ||
| + | one makes the change of variable $ p = \sigma _ {a} + z / t $. | ||
| + | The integral (4) then takes the form | ||
| − | + | $$ | |
| + | f ( t) = | ||
| + | \frac{1}{2 \pi i } | ||
| − | + | \frac{e ^ {\sigma _ {a} t } }{t} | |
| − | + | \int\limits _ {\epsilon - i \infty } ^ { \epsilon + i \infty } | |
| + | e ^ {z} F ^ { * } ( z) d z = \ | ||
| − | + | \frac{e ^ {\sigma _ {a} t } }{t} | |
| − | + | J ( s) , | |
| + | $$ | ||
| + | |||
| + | $$ | ||
| + | \epsilon > 0 ,\ F ^ { * } ( z) = F \left ( | ||
| + | \frac{z}{t} | ||
| + | + \sigma _ {a} \right ) = F ( p) . | ||
| + | $$ | ||
| + | |||
| + | As before, assume that $ F ^ { * } ( z) = z ^ {-} s \phi ( z) $. | ||
| + | In order to calculate the integral $ J ( s) $ | ||
| + | one constructs the quadrature formula | ||
| + | |||
| + | $$ \tag{10 } | ||
| + | J ( s) \approx \sum _ { k= } 1 ^ { n } | ||
| + | A _ {k} ^ {(} s) \phi ( z _ {k} ^ {(} s) ) , | ||
| + | $$ | ||
| + | |||
| + | which should be exact for any polynomial of degree $ \leq 2 n - 1 $ | ||
| + | in $ 1 / z $. | ||
| + | For this it is necessary and sufficient that (10) is an interpolation formula and that the points $ z _ {k} ^ {(} s) $ | ||
| + | are the roots of some system of orthogonal polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $. | ||
| + | Finally, this condition leads to the formula | ||
| + | |||
| + | $$ \tag{11 } | ||
| + | f ( t) \approx | ||
| + | \frac{e ^ {\sigma _ {a} t } }{t} | ||
| + | |||
| + | \sum _ { k= } 1 ^ { n } A _ {k} ^ {(} s) | ||
| + | ( z _ {k} ^ {(} s) ) ^ {s} F | ||
| + | \left ( | ||
| + | \frac{z _ {k} ^ {(} s) }{t} | ||
| + | + \sigma _ {a} \right ) , | ||
| + | $$ | ||
| + | |||
| + | where the $ z _ {k} ^ {(} s) $ | ||
| + | are the roots of the orthogonal polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $. | ||
| + | For the polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $ | ||
| + | an explicit expression is known, as well as a recurrence relation, a differential equation of which they are the solutions, and a generating function. For certain special values of $ s $ | ||
| + | it has been shown that the roots of the polynomials $ \omega _ {n} ( 1 / z ) $ | ||
| + | lie in the right half-plane (see [[#References|[13]]]). Values of the points and the coefficients $ A _ {k} ^ {(} s) $ | ||
| + | in (11) were given in [[#References|[12]]] for $ s = 1, 2, 3, 4, 5 $; | ||
| + | $ n = 1( 1) 15 $ | ||
| + | with 20 correct decimal places and for $ s = 0.01 ( 0.01 ) 3 $; | ||
| + | $ n = 1 ( 1) 10 $ | ||
| + | with 7–8 correct decimal places. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> P.S. Laplace, "Théorie analytique des probabilités" , Paris (1812)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> B. van der Pol, H. Bremmer, "Operational calculus based on the two-sided Laplace integral" , Cambridge Univ. Press (1955)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> S. Bochner, "Lectures on Fourier integrals" , Princeton Univ. Press (1959) (Translated from German)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> V.A. Ditkin, A.P. Prudnikov, "Operational calculus" , Moscow (1966) (In Russian)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> V.A. Ditkin, A.P. Prudnikov, "Transformations intégrales et calcul opérationnel" , MIR (1978) (Translated from Russian)</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> G. Doetsch, "Handbuch der Laplace-Transformation" , '''1–3''' , Birkhäuser (1950–1956)</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> V.S. Vladimirov, "Generalized functions in mathematical physics" , MIR (1977) (Translated from Russian)</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> A.H. Zemanian, "Generalized integral transformations" , Wiley (1968)</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> V.S. Aizenshtat, V.I. Krylov, A.S. Metel'skii, "Tables for the numerical Laplace transform and the evaluation of integrals of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057540/l057540179.png" />" , Minsk (1962) (In Russian)</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> H.E. Salzer, R. Zucker, "Tables of the zeros and weight factors of the first fifteen Laguerre polynomials" ''Bull. Amer. Math. Soc.'' , '''55''' (1949) pp. 1004–1012</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> A.A. Pal'tsev, N.S. Skoblya, "The integration of bounded functions with a Laguerre weight" ''Izv. Akad. Nauk BSSR Ser. Fiz. Mat. Nauk'' , '''3''' (1965) pp. 15–23 (In Russian)</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> V.I. Krylov, N.S. Skoblya, "Handbook of numerical inversion of Laplace transforms" , Israel Program Sci. Transl. (1969) (Translated from Russian)</TD></TR><TR><TD valign="top">[13]</TD> <TD valign="top"> V.I. Krylov, N.S. Skoblya, "Handbook of methods of approximate Fourier transformation and inversion of Laplace transformation" , MIR (1977) (Translated from Russian)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> P.S. Laplace, "Théorie analytique des probabilités" , Paris (1812)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> B. van der Pol, H. Bremmer, "Operational calculus based on the two-sided Laplace integral" , Cambridge Univ. Press (1955)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> S. Bochner, "Lectures on Fourier integrals" , Princeton Univ. Press (1959) (Translated from German)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> V.A. Ditkin, A.P. Prudnikov, "Operational calculus" , Moscow (1966) (In Russian)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> V.A. Ditkin, A.P. Prudnikov, "Transformations intégrales et calcul opérationnel" , MIR (1978) (Translated from Russian)</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> G. Doetsch, "Handbuch der Laplace-Transformation" , '''1–3''' , Birkhäuser (1950–1956)</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> V.S. Vladimirov, "Generalized functions in mathematical physics" , MIR (1977) (Translated from Russian)</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> A.H. Zemanian, "Generalized integral transformations" , Wiley (1968)</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> V.S. Aizenshtat, V.I. Krylov, A.S. Metel'skii, "Tables for the numerical Laplace transform and the evaluation of integrals of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057540/l057540179.png" />" , Minsk (1962) (In Russian)</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> H.E. Salzer, R. Zucker, "Tables of the zeros and weight factors of the first fifteen Laguerre polynomials" ''Bull. Amer. Math. Soc.'' , '''55''' (1949) pp. 1004–1012</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> A.A. Pal'tsev, N.S. Skoblya, "The integration of bounded functions with a Laguerre weight" ''Izv. Akad. Nauk BSSR Ser. Fiz. Mat. Nauk'' , '''3''' (1965) pp. 15–23 (In Russian)</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> V.I. Krylov, N.S. Skoblya, "Handbook of numerical inversion of Laplace transforms" , Israel Program Sci. Transl. (1969) (Translated from Russian)</TD></TR><TR><TD valign="top">[13]</TD> <TD valign="top"> V.I. Krylov, N.S. Skoblya, "Handbook of methods of approximate Fourier transformation and inversion of Laplace transformation" , MIR (1977) (Translated from Russian)</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | |||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> F. Oberhettinger, L. Badii, "Tables of Laplace transforms" , Springer (1973)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.N. Sneddon, "The use of integral transforms" , McGraw-Hill (1972) pp. Chapt. 6</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> D.V. Widder, "The Laplace transform" , Princeton Univ. Press (1972)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> G. Doetsch, "Introduction to the theory and application of the Laplace transformation" , Springer (1974) (Translated from German)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> K.B. Wolff, "Integral transforms in science and engineering" , Plenum (1979)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> F. Oberhettinger, L. Badii, "Tables of Laplace transforms" , Springer (1973)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.N. Sneddon, "The use of integral transforms" , McGraw-Hill (1972) pp. Chapt. 6</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> D.V. Widder, "The Laplace transform" , Princeton Univ. Press (1972)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> G. Doetsch, "Introduction to the theory and application of the Laplace transformation" , Springer (1974) (Translated from German)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> K.B. Wolff, "Integral transforms in science and engineering" , Plenum (1979)</TD></TR></table> | ||
Revision as of 22:15, 5 June 2020
In the wide sense it is a Laplace integral of the form
$$ \tag{1 } F ( p) = \int\limits _ { L } f ( z) e ^ {- p z } d z , $$
where the integration is carried out over some contour $ L $ in the complex $ z $- plane, which sets up a correspondence between a function $ f ( z) $, defined on $ L $, and an analytic function $ F ( p) $ of the complex variable $ p = \sigma + i \tau $. Many integrals of the form (1) were considered by P. Laplace (see [1]).
In the narrow sense the Laplace transform is understood to be the one-sided Laplace transform
$$ \tag{2 } F ( p) = L [ f] ( p) = \int\limits _ { 0 } ^ \infty f ( t) e ^ {- p t } d t , $$
so called to distinguish it from the two-sided Laplace transform
$$ \tag{3 } F ( p) = L [ f] ( p) = \int\limits _ {- \infty } ^ \infty f ( t) e ^ {- p t } d t . $$
The Laplace transform is a special kind of integral transform; transforms of the form (2) or (3) are closely connected with the Fourier transform. The two-sided Laplace transform (3) can be regarded as the Fourier transform of the function $ f ( t) e ^ {- \sigma t } $, and the one-sided Laplace transform (2) can be regarded as the Fourier transform of the function $ \phi ( t) $ equal to $ f ( t) e ^ {- \sigma t } $ for $ 0 < t < \infty $ and equal to zero for $ - \infty < t < 0 $.
The complex locally summable integrand $ f ( t) $ is called the original function, or simply the original; in applications it is often convenient to treat the variable $ t $ as time. The function $ F ( p) = L [ f] ( p) $ is called the Laplace transform of the original $ f ( t) $. Generally speaking, the integral (2) is understood to be conditionally convergent at infinity. A priori, three cases are possible: 1) there is a real number $ \sigma _ {c} $ such that the integral (2) converges for $ \mathop{\rm Re} p = \sigma > \sigma _ {c} $ and diverges for $ \mathop{\rm Re} p = \sigma < \sigma _ {c} $; this number $ \sigma _ {c} $ is called the abscissa of (conditional) convergence; 2) the integral (2) converges for all $ p $, in which case one puts $ \sigma _ {c} = - \infty $; and 3) the integral (2) diverges for all $ p $, in which case one puts $ \sigma _ {c} = + \infty $. If $ \sigma _ {c} < + \infty $, then the integral (2) represents a single-valued analytic function $ F ( p) $ in the half-plane of convergence $ \mathop{\rm Re} p > \sigma _ {c} $. One usually restricts oneself to the consideration of absolutely convergent integrals (2). The greatest lower bound of those $ \sigma $ for which the integral
$$ \int\limits _ { 0 } ^ \infty | f ( t) | e ^ {- \sigma t } d t $$
exists, is called the abscissa of absolute convergence $ \sigma _ {a} $, $ \sigma _ {c} \leq \sigma _ {a} $. If $ a $ is the lower bound of those $ \sigma $ for which $ | f ( t) | = O ( e ^ {\sigma t } ) $, $ t \rightarrow \infty $, then $ \sigma _ {a} = a $; the number $ a $ is sometimes called the index of growth of $ f ( t) $.
Under certain additional conditions $ f ( t) $ can be uniquely restored from its Laplace transform $ F ( p) $. For example, if $ f ( t) $ has bounded variation in a neighbourhood of $ t _ {0} $ or if $ f ( t) $ is piecewise smooth, then the inversion formula for the Laplace transform holds:
$$ \tag{4 } f ^ \circ ( t _ {0} ) = \ \frac{f ( t _ {0} + 0 ) + f ( t _ {0} - 0 ) }{2\ } = $$
$$ = \ \frac{1}{2 \pi i } \lim\limits _ {R \rightarrow \infty } \int\limits _ {\sigma - i R } ^ { \sigma + i R } F ( p) e ^ {pt _ {0} } d p ,\ \sigma > \sigma _ {a} . $$
Formulas (2) and (4) make it possible to obtain a number of relations between operations carried out over originals and transforms, and also to obtain a table of transforms for frequently occurring originals. All this constitutes an elementary part of operational calculus.
In mathematical physics there are important applications of the multi-dimensional Laplace transform
$$ \tag{5 } F ( p) = \int\limits _ {C _ {+} } f ( t) e ^ {- ( p , t ) } d t , $$
where $ t = ( t _ {1} \dots t _ {n} ) $ is a point of the $ n $- dimensional Euclidean space $ \mathbf R ^ {n} $, $ p = ( p _ {1} \dots p _ {n} ) = \sigma + i \tau = ( \sigma _ {1} \dots \sigma _ {n} ) + i ( \tau _ {1} \dots \tau _ {n} ) $ is a point of the complex space $ \mathbf C ^ {n} $, $ n \geq 1 $,
$$ ( p , t ) = ( \sigma , t ) + i ( \tau , t) = p _ {1} t _ {1} + \dots + p _ {n} t _ {n} $$
is the scalar product and $ d t = d t _ {1} \dots d t _ {n} $ is the volume element in $ \mathbf R ^ {n} $. The complex function $ f ( t) $ in (5) is defined and locally summable in the domain of integration $ C _ {+} = \{ {t \in \mathbf R ^ {n} } : {t _ {j} > 0, j = 1 \dots n } \} $, the positive octant of $ \mathbf R ^ {n} $. If $ f ( t) $ is bounded in $ C _ {+} $, then the integral (5) exists at all points $ p \in \mathbf C ^ {n} $ that satisfy the condition $ \mathop{\rm Re} ( p , t ) > 0 $, $ t \in C _ {+} $, which again determines the positive octant $ S = \{ {\sigma \in \mathbf R ^ {n} } : {\sigma _ {j} > 0, j = 1 \dots n } \} $. The integral (5) determines a holomorphic function of the complex variable $ p = ( p _ {1} \dots p _ {n} ) $ in the tube domain $ T ^ {S} = S + i \mathbf R ^ {n} = \{ {p = \sigma + i \tau \in \mathbf C ^ {n} } : {\sigma \in S, \tau \in \mathbf R ^ {n} } \} $ in $ \mathbf C ^ {n} $ with base $ S $. In the more general case, for the domain of integration $ C _ {+} $ in (5) and the base $ S $ of the tube domain one can take any pair of conjugate closed convex acute-angled cones in $ \mathbf R ^ {n} $ with vertex at the origin. For $ n = 1 $ formula (5) becomes (2), $ C _ {+} = \{ {t \in \mathbf R } : {t > 0 } \} $ becomes the positive semi-axis and $ T ^ {S} = \{ {p = \sigma + i \tau \in \mathbf C } : {\sigma > 0 } \} $ becomes the right half-plane. The Laplace transform (5) is defined and holomorphic for functions $ f ( t) $ of much wider classes, for example, for all rapidly-decreasing functions that constitute the class $ {\mathcal S} = {\mathcal S} ( \mathbf R ^ {n} ) $, that is, for infinitely differentiable functions $ f ( t) $ in $ \mathbf R ^ {n} $ that decrease as $ | t | \rightarrow \infty $ together with all their derivatives faster than any power of $ | t | ^ {-} 1 $. Elementary properties of the Laplace transform, with corresponding changes, remain true for the multi-dimensional case.
A generalization of the Laplace transform is the Laplace transform of measures and, in general, of generalized functions. The theory of the Laplace transform of generalized functions has been most completely developed for the class $ {\mathcal S} ^ \prime = {\mathcal S} ^ \prime ( \mathbf R ^ {n} ) $, which is important in mathematical physics, of generalized functions of slow growth, defined as linear continuous functionals on the space of rapidly-decreasing test functions $ {\mathcal S} = {\mathcal S} ( \mathbf R ^ {n} ) $. The Laplace transform $ L [ g] $ of a generalized function of slow growth $ g \in {\mathcal S} ^ \prime $ is again a generalized function of slow growth, $ L [ g] \in {\mathcal S} ^ \prime $.
Numerical Laplace transformation.
This is a numerical realization of the transform (2) that takes the original $ f ( t) $, $ 0 < t < \infty $, into the transform $ F ( p) $, $ p = \sigma + i \tau $, and also the numerical inversion of the Laplace transform, that is, the numerical determination of $ f ( t) $ from the integral equation (2) or from the inversion formula (4).
The need to apply the numerical Laplace transform arises as a consequence of the fact that the tables of originals and transforms cover by no means all cases occurring in practice, and also as a consequence of the fact that the original or the transform is frequently expressed by formulas that are too complicated and inconvenient for applications.
In the case of real values of $ p $, formula (2) can be reduced, under certain additional assumptions, to an integral with Laguerre weight:
$$ \tag{6 } F ( p) = \frac{1}{p} \int\limits _ { 0 } ^ \infty x ^ {s} e ^ {-} x \phi ( x) d x $$
for some $ s \geq 0 $. Under certain conditions a Laplace transform reduces to the integral (6) for complex $ p $( see [9]).
To calculate the integral in (6) one can use the quadrature formula
$$ \tag{7 } \int\limits _ { 0 } ^ \infty x ^ {s} e ^ {-} x \phi ( x) d x \approx \sum _ { k= } 1 ^ { n } A _ {k} ^ {(} s) \phi ( x _ {k} ^ {(} s) ) , $$
where the coefficients $ A _ {k} ^ {(} s) $ and the points $ x _ {k} ^ {(} s) $ are chosen in such a way that the equality (7) for fixed $ n $ is exact either for all polynomials of degree $ \leq 2 n - 1 $ or for some system of rational functions, depending on the properties of $ \phi ( x) $. The coefficients $ A _ {k} ^ {(} s) $ and the points $ x _ {k} ^ {(} s) $ for such quadrature formulas have been calculated for many values of $ s $( see [9]–[11]).
The problem of inverting the Laplace transform, as a problem of finding a solution $ f ( x) $ of the integral equation of the first kind (2), concerns a class of ill-posed problems and can be solved, in particular, by means of a regularizing algorithm.
The problem of numerical inversion of a Laplace transform can also be solved by methods based on the expansion of the original in a series of functions. Here, in the first place, one can carry out an expansion in a power series, a generalized power series, a series of exponential functions, and also series of orthogonal functions, in particular, in Chebyshev, Legendre, Jacobi, or Laguerre polynomials. The problem of expanding the original in a series of Chebyshev, Legendre or Jacobi polynomials in its final form reduces to the problem of moments on a finite interval. Suppose one knows the Laplace transform $ F ( p) $ of the function $ \beta ( t) f ( t) $:
$$ F ( p) = \int\limits _ { 0 } ^ \infty e ^ {-} pt \beta ( t) f ( t) d t , $$
where $ f ( t) $ is the unknown function and $ \beta ( t) $ is a non-negative integrable function on $ [ 0 , \infty ] $. Assume that $ f ( t) $ is integrable on any finite interval $ [ 0 , T ] $ and belongs to the class $ L _ {2} ( \beta ( t) , [ 0 , \infty )) $. From the transform $ F ( p) $ of $ \beta ( t) f ( t) $ the function $ f ( t) $ can be constructed as a series in shifted Jacobi polynomials, in particular, in shifted Legendre polynomials and Chebyshev polynomials of the first and second kind, the coefficients $ a _ {k} $ of which are calculated from the formula
$$ a _ {k} = \sum _ { i= } 0 ^ { k } \alpha _ {i} ^ {(} k) F ( i) , $$
where the $ \alpha _ {i} ^ {(} k) $ are the coefficients of the shifted Legendre polynomials or Chebyshev polynomials of the first and second kind, respectively, written in the form $ \sum _ {i=} 1 ^ {k} \alpha _ {i} ^ {(} k) x ^ {i} $( see [4]).
Suppose one is given the Laplace transform $ F ( p) $ of the function $ f ( t) $ and that $ f ( t) $ satisfies the condition
$$ \int\limits _ { 0 } ^ \infty e ^ {-} t t ^ \lambda | f ( t) | ^ {2} d t < \infty ,\ \ \lambda > - 1 . $$
Then $ f ( t) $ can be expanded in a series of generalized Laguerre polynomials,
$$ f ( t) = t ^ \lambda \sum _ { k= } 0 ^ \infty a _ {k} \frac{k ! }{\Gamma ( k + \lambda + 1 ) } L _ {k} ^ {( \lambda ) } ( t) , $$
which converges to $ f ( t) $ in the mean. The coefficients $ a _ {k} $ of this series are calculated from the formula
$$ a _ {k} = \ \frac{( - 1 ) ^ {k} }{k ! } \frac{d ^ {k} }{d z ^ {k} } \left . \left \{ \frac{1}{z ^ {\lambda + 1 } } F \left ( \frac{1}{z} \right ) \right \} \right | _ {z = 1 } . $$
Another method of inverting the Laplace transform is to construct quadrature formulas for the inversion integral (4).
The transform $ F ( p) $ tends to zero if the point $ p $ tends to infinity in such a way that $ \mathop{\rm Re} p $ tends to infinity. Assume that $ F ( p) $ decreases polynomially, that is, $ F ( p) $ can be expressed in the form
$$ F ( p) = \frac{1}{p ^ {s} } \phi ( p) ,\ \ s > 0 , $$
with $ \phi ( p) $ regular in the half-plane $ \mathop{\rm Re} p > \sigma _ {a} $ and continuous for $ \mathop{\rm Re} p \geq \sigma _ {a} $. The integral (4) has the form
$$ \tag{8 } f ( t) = \frac{1}{2 \pi i } \int\limits _ {\sigma - i \infty } ^ { \sigma + i \infty } e ^ {pt} p ^ {-} s \phi ( p) d p . $$
For the integral (8) an interpolation quadrature formula has been constructed, based on the interpolation of $ \phi ( p) $ by polynomials in $ 1 / p $:
$$ \tag{9 } f ( t) = \sum _ { k= } 0 ^ { n } A _ {k} ^ {(} s) ( t) \phi ( p _ {k} ) + R _ {n} , $$
where the $ p _ {k} $ are the interpolation points, which are arbitrary and situated to the right of the line $ \mathop{\rm Re} p = \sigma _ {a} $, $ R _ {n} $ is the remainder term of the formula, and
$$ A _ {k} ^ {(} s) ( t) = \ \sum _ { j= } 0 ^ { n } \frac{a _ {kj} t ^ {s+} j- 1 }{\Gamma ( s + j ) } . $$
The coefficients $ a _ {kj} $ depend only on the chosen points $ p _ {k} $ and for some methods of choosing them (in particular, for equidistant points) they have been calculated (see [12]). The problem of investigating the convergence of interpolation quadrature formulas consists in finding relations between the properties of $ \phi ( p) $ and the points $ p _ {k} $ for which one can check that the remainder term $ R _ {n} $ in (9) tends to zero. This problem has been solved for certain concrete points $ p _ {k} $ and for certain special classes of functions $ \phi ( p) $( see [13]).
For the integral (4) one can construct quadrature formulas of the highest degree of accuracy in the class of rational functions of special form. In order that the parameters of the formula do not depend on $ \sigma _ {a} $ and $ t $, one makes the change of variable $ p = \sigma _ {a} + z / t $. The integral (4) then takes the form
$$ f ( t) = \frac{1}{2 \pi i } \frac{e ^ {\sigma _ {a} t } }{t} \int\limits _ {\epsilon - i \infty } ^ { \epsilon + i \infty } e ^ {z} F ^ { * } ( z) d z = \ \frac{e ^ {\sigma _ {a} t } }{t} J ( s) , $$
$$ \epsilon > 0 ,\ F ^ { * } ( z) = F \left ( \frac{z}{t} + \sigma _ {a} \right ) = F ( p) . $$
As before, assume that $ F ^ { * } ( z) = z ^ {-} s \phi ( z) $. In order to calculate the integral $ J ( s) $ one constructs the quadrature formula
$$ \tag{10 } J ( s) \approx \sum _ { k= } 1 ^ { n } A _ {k} ^ {(} s) \phi ( z _ {k} ^ {(} s) ) , $$
which should be exact for any polynomial of degree $ \leq 2 n - 1 $ in $ 1 / z $. For this it is necessary and sufficient that (10) is an interpolation formula and that the points $ z _ {k} ^ {(} s) $ are the roots of some system of orthogonal polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $. Finally, this condition leads to the formula
$$ \tag{11 } f ( t) \approx \frac{e ^ {\sigma _ {a} t } }{t} \sum _ { k= } 1 ^ { n } A _ {k} ^ {(} s) ( z _ {k} ^ {(} s) ) ^ {s} F \left ( \frac{z _ {k} ^ {(} s) }{t} + \sigma _ {a} \right ) , $$
where the $ z _ {k} ^ {(} s) $ are the roots of the orthogonal polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $. For the polynomials $ \omega _ {n} ^ {(} s) ( 1 / z ) $ an explicit expression is known, as well as a recurrence relation, a differential equation of which they are the solutions, and a generating function. For certain special values of $ s $ it has been shown that the roots of the polynomials $ \omega _ {n} ( 1 / z ) $ lie in the right half-plane (see [13]). Values of the points and the coefficients $ A _ {k} ^ {(} s) $ in (11) were given in [12] for $ s = 1, 2, 3, 4, 5 $; $ n = 1( 1) 15 $ with 20 correct decimal places and for $ s = 0.01 ( 0.01 ) 3 $; $ n = 1 ( 1) 10 $ with 7–8 correct decimal places.
References
| [1] | P.S. Laplace, "Théorie analytique des probabilités" , Paris (1812) |
| [2] | B. van der Pol, H. Bremmer, "Operational calculus based on the two-sided Laplace integral" , Cambridge Univ. Press (1955) |
| [3] | S. Bochner, "Lectures on Fourier integrals" , Princeton Univ. Press (1959) (Translated from German) |
| [4] | V.A. Ditkin, A.P. Prudnikov, "Operational calculus" , Moscow (1966) (In Russian) |
| [5] | V.A. Ditkin, A.P. Prudnikov, "Transformations intégrales et calcul opérationnel" , MIR (1978) (Translated from Russian) |
| [6] | G. Doetsch, "Handbuch der Laplace-Transformation" , 1–3 , Birkhäuser (1950–1956) |
| [7] | V.S. Vladimirov, "Generalized functions in mathematical physics" , MIR (1977) (Translated from Russian) |
| [8] | A.H. Zemanian, "Generalized integral transformations" , Wiley (1968) |
| [9] | V.S. Aizenshtat, V.I. Krylov, A.S. Metel'skii, "Tables for the numerical Laplace transform and the evaluation of integrals of the form 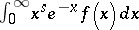 " , Minsk (1962) (In Russian) " , Minsk (1962) (In Russian) |
| [10] | H.E. Salzer, R. Zucker, "Tables of the zeros and weight factors of the first fifteen Laguerre polynomials" Bull. Amer. Math. Soc. , 55 (1949) pp. 1004–1012 |
| [11] | A.A. Pal'tsev, N.S. Skoblya, "The integration of bounded functions with a Laguerre weight" Izv. Akad. Nauk BSSR Ser. Fiz. Mat. Nauk , 3 (1965) pp. 15–23 (In Russian) |
| [12] | V.I. Krylov, N.S. Skoblya, "Handbook of numerical inversion of Laplace transforms" , Israel Program Sci. Transl. (1969) (Translated from Russian) |
| [13] | V.I. Krylov, N.S. Skoblya, "Handbook of methods of approximate Fourier transformation and inversion of Laplace transformation" , MIR (1977) (Translated from Russian) |
Comments
References
| [a1] | F. Oberhettinger, L. Badii, "Tables of Laplace transforms" , Springer (1973) |
| [a2] | I.N. Sneddon, "The use of integral transforms" , McGraw-Hill (1972) pp. Chapt. 6 |
| [a3] | D.V. Widder, "The Laplace transform" , Princeton Univ. Press (1972) |
| [a4] | G. Doetsch, "Introduction to the theory and application of the Laplace transformation" , Springer (1974) (Translated from German) |
| [a5] | K.B. Wolff, "Integral transforms in science and engineering" , Plenum (1979) |
Laplace transform. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laplace_transform&oldid=13300