Difference between revisions of "Fano variety"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | f0382201.png | ||
| + | $#A+1 = 54 n = 2 | ||
| + | $#C+1 = 54 : ~/encyclopedia/old_files/data/F038/F.0308220 Fano variety | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | A Fano variety of dimension 2 is called a del Pezzo surface and is a [[Rational surface|rational surface]]. The multi-dimensional analogues of del Pezzo surfaces — Fano varieties of dimension | + | {{TEX|auto}} |
| + | {{TEX|done}} | ||
| + | |||
| + | A smooth complete irreducible [[Algebraic variety|algebraic variety]] $ X $ | ||
| + | over a field $ k $ | ||
| + | whose anti-canonical sheaf $ K _ {X} ^ {-} 1 $ | ||
| + | is ample (cf. [[Ample sheaf|Ample sheaf]]). The basic research into such varieties was done by G. Fano ([[#References|[1]]], [[#References|[2]]]). | ||
| + | |||
| + | A Fano variety of dimension 2 is called a del Pezzo surface and is a [[Rational surface|rational surface]]. The multi-dimensional analogues of del Pezzo surfaces — Fano varieties of dimension $ > 2 $— | ||
| + | are not all rational varieties, for example the general cubic in the projective space $ P ^ {4} $. | ||
| + | It is not known (1984) whether all Fano varieties are unirational. | ||
Three-dimensional Fano varieties have been thoroughly investigated (see [[#References|[3]]], ). Only isolated particular results are known about Fano varieties of dimension greater than 3. | Three-dimensional Fano varieties have been thoroughly investigated (see [[#References|[3]]], ). Only isolated particular results are known about Fano varieties of dimension greater than 3. | ||
| − | The [[Picard group|Picard group]] | + | The [[Picard group|Picard group]] $ \mathop{\rm Pic} X $ |
| + | of a three-dimensional Fano variety is finitely generated and torsion-free. In case the ground field $ k $ | ||
| + | is $ \mathbf C $, | ||
| + | the rank of $ \mathop{\rm Pic} X $, | ||
| + | which is equal to the second [[Betti number|Betti number]] $ b _ {2} ( X) $, | ||
| + | does not exceed 10 (see [[#References|[4]]]). If $ b _ {2} ( X) \geq 6 $, | ||
| + | then the Fano variety is isomorphic to $ P ^ {1} \times S _ {11 - b _ {2} ( X) } $, | ||
| + | where $ S _ {d} $ | ||
| + | is the del Pezzo surface of order $ d $. | ||
| + | A Fano variety $ X $ | ||
| + | is called primitive if there is no [[Monoidal transformation|monoidal transformation]] $ \sigma : X \rightarrow X _ {1} $ | ||
| + | to a smooth variety $ X _ {1} $ | ||
| + | with centre at a non-singular irreducible curve. If $ X $ | ||
| + | is a primitive Fano variety, then $ b _ {2} ( X) \leq 3 $. | ||
| + | If $ b _ {2} ( X) = 3 $, | ||
| + | then $ X $ | ||
| + | is a conic fibre space over $ S = P ^ {1} \times P ^ {1} $, | ||
| + | in other words, then there is a morphism $ \pi : X \rightarrow S $ | ||
| + | each fibre of which is isomorphic to a conic, that is, an algebraic scheme given by a homogeneous equation of degree 2 in $ P ^ {2} $. | ||
| + | A Fano variety $ X $ | ||
| + | with $ b _ {2} ( X) = 2 $ | ||
| + | is a conic fibre space over the projective plane $ P ^ {2} $( | ||
| + | see [[#References|[3]]]). In the case $ b _ {2} ( X) = 1 $ | ||
| + | there are 18 types of Fano varieties, which have been classified (see [[#References|[6]]]). | ||
| − | For three-dimensional Fano varieties | + | For three-dimensional Fano varieties $ X $ |
| + | the self-intersection index of the anti-canonical divisor $ (- K _ {X} ^ {3} ) \leq 64 $. | ||
| + | The largest integer $ r \geq 1 $ | ||
| + | such that $ H ^ {\otimes r } $ | ||
| + | is isomorphic to $ K _ {X} ^ {-} 1 $ | ||
| + | for some divisor $ H \in \mathop{\rm Pic} X $ | ||
| + | is called the index of the Fano variety. The index of a three-dimensional Fano variety can take the values 1, 2, 3, or 4. A Fano variety of index 4 is isomorphic to the projective space $ P ^ {3} $, | ||
| + | and a Fano variety of index 3 is isomorphic to a smooth quadric $ Q \subset P ^ {4} $. | ||
| + | If $ r = 2 $, | ||
| + | then the self-intersection index $ d = H ^ {3} $ | ||
| + | can take the values $ 1 \leq d \leq 7 $, | ||
| + | with each of them being realized for some Fano variety. For a Fano variety of index 1 the mapping $ \phi _ {K _ {X} ^ {-} 1 } : X \rightarrow P ^ { \mathop{\rm dim} | K _ {X} ^ {-} 1 | } $ | ||
| + | defined by the linear system $ | K _ {X} ^ {-} 1 | $ | ||
| + | has degree $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 1 $ | ||
| + | or 2. The Fano varieties of index 1 for which $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 2 $ | ||
| + | have been classified. If $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 1 $, | ||
| + | then $ X $ | ||
| + | can be realized as a subvariety $ V _ {2g - 2 } $ | ||
| + | of degree $ 2g - 2 $ | ||
| + | in the projective space $ P ^ {g + 1 } $. | ||
| + | The number $ g $ | ||
| + | is called the genus of the Fano variety $ V _ {2g - 2 } $ | ||
| + | and is the same as the genus of the canonical curve — the section of $ X $ | ||
| + | under the anti-canonical imbedding into $ P ^ {g + 1 } $. | ||
| + | The Fano varieties $ V _ {2g - 2 } \subset P ^ {g + 1 } $ | ||
| + | the class of a hyperplane section of which is the same as the anti-canonical class and generates $ \mathop{\rm Pic} V _ {2g - 2 } $ | ||
| + | have been classified (see [[#References|[4]]], ). | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> G. Fano, "Sulle varietà algebriche a tre dimensioni aventi tutti i generi nulu" , ''Proc. Internat. Congress Mathematicians (Bologna)'' , '''4''' , Zanichelli (1934) pp. 115–119</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> G. Fano, "Si alcune varietà algebriche a tre dimensioni razionali, e aventi curve-sezioni canoniche" ''Comment. Math. Helv.'' , '''14''' (1942) pp. 202–211</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> S. Mori, S. Mukai, "Classification of Fano 3-folds with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f038/f038220/f03822055.png" />" ''Manuscripta Math.'' , '''36''' : 2 (1981) pp. 147–162</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> L. Roth, "Sulle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f038/f038220/f03822056.png" /> algebriche su cui l'aggiunzione si estingue" ''Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat.'' , '''9''' (1950) pp. 246–250</TD></TR><TR><TD valign="top">[5a]</TD> <TD valign="top"> V.A. Iskovskikh, "Fano 3-folds. I" ''Math. USSR. Izv.'' , '''11''' : 3 (1977) pp. 485–527 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''41''' : 3 (1977) pp. 516–562</TD></TR><TR><TD valign="top">[5b]</TD> <TD valign="top"> V.A. Iskovskikh, "Fano 3-folds. II" ''Math. USSR. Izv.'' , '''12''' : 3 (1978) pp. 469–506 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''42''' : 3 (1978) pp. 506–549</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> V.A. Iskovskikh, "Anticanonical models of three-dimensional algebraic varieties" ''J. Soviet Math.'' , '''13''' : 6 (1980) pp. 745–850 ''Itogi Nauk. i Tekhn. Sovr. Probl. Mat.'' , '''12''' (1979) pp. 59–157</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> G. Fano, "Sulle varietà algebriche a tre dimensioni aventi tutti i generi nulu" , ''Proc. Internat. Congress Mathematicians (Bologna)'' , '''4''' , Zanichelli (1934) pp. 115–119</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> G. Fano, "Si alcune varietà algebriche a tre dimensioni razionali, e aventi curve-sezioni canoniche" ''Comment. Math. Helv.'' , '''14''' (1942) pp. 202–211</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> S. Mori, S. Mukai, "Classification of Fano 3-folds with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f038/f038220/f03822055.png" />" ''Manuscripta Math.'' , '''36''' : 2 (1981) pp. 147–162</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> L. Roth, "Sulle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f038/f038220/f03822056.png" /> algebriche su cui l'aggiunzione si estingue" ''Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat.'' , '''9''' (1950) pp. 246–250</TD></TR><TR><TD valign="top">[5a]</TD> <TD valign="top"> V.A. Iskovskikh, "Fano 3-folds. I" ''Math. USSR. Izv.'' , '''11''' : 3 (1977) pp. 485–527 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''41''' : 3 (1977) pp. 516–562</TD></TR><TR><TD valign="top">[5b]</TD> <TD valign="top"> V.A. Iskovskikh, "Fano 3-folds. II" ''Math. USSR. Izv.'' , '''12''' : 3 (1978) pp. 469–506 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''42''' : 3 (1978) pp. 506–549</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> V.A. Iskovskikh, "Anticanonical models of three-dimensional algebraic varieties" ''J. Soviet Math.'' , '''13''' : 6 (1980) pp. 745–850 ''Itogi Nauk. i Tekhn. Sovr. Probl. Mat.'' , '''12''' (1979) pp. 59–157</TD></TR></table> | ||
Revision as of 19:38, 5 June 2020
A smooth complete irreducible algebraic variety $ X $
over a field $ k $
whose anti-canonical sheaf $ K _ {X} ^ {-} 1 $
is ample (cf. Ample sheaf). The basic research into such varieties was done by G. Fano ([1], [2]).
A Fano variety of dimension 2 is called a del Pezzo surface and is a rational surface. The multi-dimensional analogues of del Pezzo surfaces — Fano varieties of dimension $ > 2 $— are not all rational varieties, for example the general cubic in the projective space $ P ^ {4} $. It is not known (1984) whether all Fano varieties are unirational.
Three-dimensional Fano varieties have been thoroughly investigated (see [3], ). Only isolated particular results are known about Fano varieties of dimension greater than 3.
The Picard group $ \mathop{\rm Pic} X $ of a three-dimensional Fano variety is finitely generated and torsion-free. In case the ground field $ k $ is $ \mathbf C $, the rank of $ \mathop{\rm Pic} X $, which is equal to the second Betti number $ b _ {2} ( X) $, does not exceed 10 (see [4]). If $ b _ {2} ( X) \geq 6 $, then the Fano variety is isomorphic to $ P ^ {1} \times S _ {11 - b _ {2} ( X) } $, where $ S _ {d} $ is the del Pezzo surface of order $ d $. A Fano variety $ X $ is called primitive if there is no monoidal transformation $ \sigma : X \rightarrow X _ {1} $ to a smooth variety $ X _ {1} $ with centre at a non-singular irreducible curve. If $ X $ is a primitive Fano variety, then $ b _ {2} ( X) \leq 3 $. If $ b _ {2} ( X) = 3 $, then $ X $ is a conic fibre space over $ S = P ^ {1} \times P ^ {1} $, in other words, then there is a morphism $ \pi : X \rightarrow S $ each fibre of which is isomorphic to a conic, that is, an algebraic scheme given by a homogeneous equation of degree 2 in $ P ^ {2} $. A Fano variety $ X $ with $ b _ {2} ( X) = 2 $ is a conic fibre space over the projective plane $ P ^ {2} $( see [3]). In the case $ b _ {2} ( X) = 1 $ there are 18 types of Fano varieties, which have been classified (see [6]).
For three-dimensional Fano varieties $ X $ the self-intersection index of the anti-canonical divisor $ (- K _ {X} ^ {3} ) \leq 64 $. The largest integer $ r \geq 1 $ such that $ H ^ {\otimes r } $ is isomorphic to $ K _ {X} ^ {-} 1 $ for some divisor $ H \in \mathop{\rm Pic} X $ is called the index of the Fano variety. The index of a three-dimensional Fano variety can take the values 1, 2, 3, or 4. A Fano variety of index 4 is isomorphic to the projective space $ P ^ {3} $, and a Fano variety of index 3 is isomorphic to a smooth quadric $ Q \subset P ^ {4} $. If $ r = 2 $, then the self-intersection index $ d = H ^ {3} $ can take the values $ 1 \leq d \leq 7 $, with each of them being realized for some Fano variety. For a Fano variety of index 1 the mapping $ \phi _ {K _ {X} ^ {-} 1 } : X \rightarrow P ^ { \mathop{\rm dim} | K _ {X} ^ {-} 1 | } $ defined by the linear system $ | K _ {X} ^ {-} 1 | $ has degree $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 1 $ or 2. The Fano varieties of index 1 for which $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 2 $ have been classified. If $ \mathop{\rm deg} \phi _ {K _ {X} ^ {-} 1 } = 1 $, then $ X $ can be realized as a subvariety $ V _ {2g - 2 } $ of degree $ 2g - 2 $ in the projective space $ P ^ {g + 1 } $. The number $ g $ is called the genus of the Fano variety $ V _ {2g - 2 } $ and is the same as the genus of the canonical curve — the section of $ X $ under the anti-canonical imbedding into $ P ^ {g + 1 } $. The Fano varieties $ V _ {2g - 2 } \subset P ^ {g + 1 } $ the class of a hyperplane section of which is the same as the anti-canonical class and generates $ \mathop{\rm Pic} V _ {2g - 2 } $ have been classified (see [4], ).
References
| [1] | G. Fano, "Sulle varietà algebriche a tre dimensioni aventi tutti i generi nulu" , Proc. Internat. Congress Mathematicians (Bologna) , 4 , Zanichelli (1934) pp. 115–119 |
| [2] | G. Fano, "Si alcune varietà algebriche a tre dimensioni razionali, e aventi curve-sezioni canoniche" Comment. Math. Helv. , 14 (1942) pp. 202–211 |
| [3] | S. Mori, S. Mukai, "Classification of Fano 3-folds with 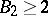 " Manuscripta Math. , 36 : 2 (1981) pp. 147–162 " Manuscripta Math. , 36 : 2 (1981) pp. 147–162 |
| [4] | L. Roth, "Sulle 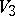 algebriche su cui l'aggiunzione si estingue" Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat. , 9 (1950) pp. 246–250 algebriche su cui l'aggiunzione si estingue" Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat. , 9 (1950) pp. 246–250 |
| [5a] | V.A. Iskovskikh, "Fano 3-folds. I" Math. USSR. Izv. , 11 : 3 (1977) pp. 485–527 Izv. Akad. Nauk SSSR Ser. Mat. , 41 : 3 (1977) pp. 516–562 |
| [5b] | V.A. Iskovskikh, "Fano 3-folds. II" Math. USSR. Izv. , 12 : 3 (1978) pp. 469–506 Izv. Akad. Nauk SSSR Ser. Mat. , 42 : 3 (1978) pp. 506–549 |
| [6] | V.A. Iskovskikh, "Anticanonical models of three-dimensional algebraic varieties" J. Soviet Math. , 13 : 6 (1980) pp. 745–850 Itogi Nauk. i Tekhn. Sovr. Probl. Mat. , 12 (1979) pp. 59–157 |
Fano variety. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fano_variety&oldid=14961