Zariski-Lipman conjecture

Let be a field of characteristic zero and let be a finitely-generated -algebra, that is, a homomorphic image of a ring of polynomials .

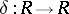A -derivation of is a -linear mapping that satisfies the Leibniz rule

for all pairs of elements of .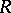

The set of all such mappings is a Lie algebra (often non-commutative; cf. also Commutative algebra) that is a finitely-generated 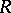-module 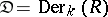. The algebra and module structures of 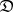often code aspects of the singularities of .

A more primitive object attached to 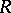is its module of Kähler differentials, 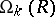, of which 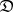is its 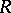-dual, .

More directly, the structure of reflects many properties of 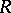. Thus, the classical Jacobian criterion asserts that 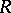is a smooth algebra over exactly when 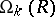is a projective 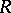-module (cf. also Projective module).

For an algebra 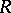without non-trivial nilpotent elements, local complete intersections are also characterized by saying that the projective dimension of 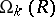(cf. also Dimension) is at most one.

The technical issues linking these properties are the comparison between the set of polynomials that define 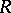, represented by the ideal 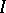, and the syzygies of eithe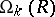r or (cf. also Syzygy).

The Zariski–Lipman conjecture makes predictions about 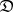, similar to those properties of .

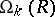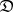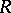The most important of these questions is as follows. If is -projective, then 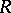is a regular ring (in commutative algebra). More precisely, it predicts that if 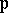is a prime ideal for which is a free 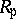-module, then 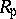is a regular ring.

In [a3], the question is settled affirmatively for rings of Krull dimension 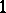(cf. also Dimension), and in all dimensions the rings are shown to be normal (cf. also Normal ring). Subsequently, G. Scheja and U. Storch [a4] established the conjecture for hypersurface rings, that is, when is defined by a single equation, .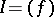

As of 2000, the last major progress on the question was the proof by M. Hochster [a2] of the graded case.

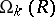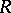A related set of questions is collected in [a5]: whether the finite projective dimension of either or necessarily forces to be a local complete intersection. It is not known (as of 2000) whether this is true if is projective, a fact which would be a consequence of the Zariski–Lipman conjecture. Several lower dimension cases are known, but the most significant progress was made by L. Avramov and J. Herzog when they solved the graded case [a1].

References

[a1]	L. Avramov, J. Herzog, "Jacobian criteria for complete intersections. The graded case" Invent. Math. (1994) pp. 75–88
[a2]	M. Hochster, "The Zariski–Lipman conjecture in the graded case" J. Algebra , 47 (1977) pp. 411–424
[a3]	J. Lipman, "Free derivation modules" Amer. J. Math. , 87 (1965) pp. 874–898
[a4]	G. Scheja, U. Storch, "Differentielle Eigenschaften der Lokalisierungen analytischer Algebren" Math. Ann. , 197 (1972) pp. 137–170
[a5]	W.V. Vasconcelos, "On the homology of 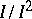" Commun. Algebra , 6 (1978) pp. 1801–1809