Difference between revisions of "Deformation"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 16: | Line 16: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> I.F. Donin, "Construction of a versal family of deformations for holomorphic bundles over a compact complex space" ''Math. USSR Sb.'' , '''23''' : 3 (1974) pp. 405–416 ''Mat. Sb.'' , '''94''' : 3 (1974) pp. 430–443</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Douady, "Le problème des modules pour les sous-espaces analytiques compacts d'un espace analytique donné" ''Ann. Inst. Fourier'' , '''16''' (1966) pp. 1–95</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Douady, "Le problème des modules locaux pour les espaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700100.png" />-analytiques compacts" ''Ann. Sci. Ecole Norm. Sup.'' , '''7''' : 4 (1974) pp. 569–602</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> H. Grauert, "Ueber die Deformation isolierter Singularitäten analytischer Menge" ''Invent. Math.'' , '''15''' : 3 (1972) pp. 171–198</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> H. Grauert, "Der Satz von Kuranishi für kompakte komplexe Räume" ''Invent. Math.'' , '''25''' : 2 (1974) pp. 107–142</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures I" ''Ann. of Math.'' , '''67''' : 2 (1958) pp. 328–401</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures II" ''Ann. of Math.'' , '''67''' : 3 (1958) pp. 403–466</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures III" ''Ann. of Math.'' , '''71''' : 1 (1960) pp. 43–76</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> M. Kuranishi, "New proof for the existence of locally complete families of complex structures" , ''Proc. Conf. Complex Analysis (Minneapolis, 1964)'' , Springer (1965) pp. 142–154</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> S.H. Moolgavkar, "On the existence of a universal germ of deformations for elliptic pseudogroup structures on compact manifolds" ''Trans. Amer. Math. Soc.'' , '''212''' : 485 (1975) pp. 173–197</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> V.P. Palamodov, "Deformations of complex spaces" ''Russian Math. Surveys'' , '''31''' : 3 (1976) pp. 129–197 ''Usphekhi Mat. Nauk'' , '''31''' (1976) pp. 129–194</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> M. Schlessinger, "Functors of Artin rings" ''Trans. Amer. Math. Soc.'' , '''130''' (1968) pp. 208–222</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> I.F. Donin, "Construction of a versal family of deformations for holomorphic bundles over a compact complex space" ''Math. USSR Sb.'' , '''23''' : 3 (1974) pp. 405–416 ''Mat. Sb.'' , '''94''' : 3 (1974) pp. 430–443 {{MR|393581}} {{ZBL|0325.32008}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Douady, "Le problème des modules pour les sous-espaces analytiques compacts d'un espace analytique donné" ''Ann. Inst. Fourier'' , '''16''' (1966) pp. 1–95 {{MR|0210935}} {{MR|0203082}} {{ZBL|}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Douady, "Le problème des modules locaux pour les espaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700100.png" />-analytiques compacts" ''Ann. Sci. Ecole Norm. Sup.'' , '''7''' : 4 (1974) pp. 569–602 {{MR|382729}} {{ZBL|0282.32014}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> H. Grauert, "Ueber die Deformation isolierter Singularitäten analytischer Menge" ''Invent. Math.'' , '''15''' : 3 (1972) pp. 171–198 {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> H. Grauert, "Der Satz von Kuranishi für kompakte komplexe Räume" ''Invent. Math.'' , '''25''' : 2 (1974) pp. 107–142 {{MR|0346194}} {{ZBL|0286.32015}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures I" ''Ann. of Math.'' , '''67''' : 2 (1958) pp. 328–401 {{MR|0112154}} {{ZBL|0128.16901}} </TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures II" ''Ann. of Math.'' , '''67''' : 3 (1958) pp. 403–466 {{MR|112154}} {{ZBL|0128.16901}} </TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures III" ''Ann. of Math.'' , '''71''' : 1 (1960) pp. 43–76 {{MR|0115189}} {{ZBL|0128.16902}} </TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> M. Kuranishi, "New proof for the existence of locally complete families of complex structures" , ''Proc. Conf. Complex Analysis (Minneapolis, 1964)'' , Springer (1965) pp. 142–154 {{MR|0176496}} {{ZBL|0144.21102}} </TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> S.H. Moolgavkar, "On the existence of a universal germ of deformations for elliptic pseudogroup structures on compact manifolds" ''Trans. Amer. Math. Soc.'' , '''212''' : 485 (1975) pp. 173–197 {{MR|0458515}} {{ZBL|0308.58014}} </TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> V.P. Palamodov, "Deformations of complex spaces" ''Russian Math. Surveys'' , '''31''' : 3 (1976) pp. 129–197 ''Usphekhi Mat. Nauk'' , '''31''' (1976) pp. 129–194 {{MR|0508121}} {{MR|0435451}} {{ZBL|0355.32020}} {{ZBL|0347.32009}} </TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> M. Schlessinger, "Functors of Artin rings" ''Trans. Amer. Math. Soc.'' , '''130''' (1968) pp. 208–222 {{MR|0217093}} {{ZBL|0167.49503}} </TD></TR></table> |
| Line 24: | Line 24: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Complexes of differential operators and symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 797–828</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Some rigidity results in the deformation theory of symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 839–851</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Deformations infinitesimals des structures conformes plates" , Birkhäuser (1984)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R. Hermann, "Geometric and Lie-theoretic principles in pure and applied deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 701–796</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> J.F. Pommaret, "Deformation theory of geometric and algebraic structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 829–838</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> K. Kodaira, "Complex manifolds and deformations of complex structures" , Springer (1986)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Complexes of differential operators and symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 797–828 {{MR|0981631}} {{ZBL|0666.58041}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Some rigidity results in the deformation theory of symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 839–851 {{MR|0981633}} {{ZBL|0698.53034}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Gasqui, H. Goldschmidt, "Deformations infinitesimals des structures conformes plates" , Birkhäuser (1984) {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R. Hermann, "Geometric and Lie-theoretic principles in pure and applied deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 701–796 {{MR|0981630}} {{ZBL|0685.58038}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> J.F. Pommaret, "Deformation theory of geometric and algebraic structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 829–838 {{MR|0981632}} {{ZBL|0684.58047}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> K. Kodaira, "Complex manifolds and deformations of complex structures" , Springer (1986) {{MR|0815922}} {{ZBL|}} </TD></TR></table> |
A deformation of an algebraic variety is the inclusion of the algebraic variety into a family of algebraic varieties. The theory of deformation of algebraic varieties and schemes is the algebraic analogue of the theory of deformation of analytic structures. Its principal problems are listed below. | A deformation of an algebraic variety is the inclusion of the algebraic variety into a family of algebraic varieties. The theory of deformation of algebraic varieties and schemes is the algebraic analogue of the theory of deformation of analytic structures. Its principal problems are listed below. | ||
| Line 57: | Line 57: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M. Artin, "Algebraic approximation of structures over complete local rings" ''Publ. Math. IHES'' , '''36''' (1969) pp. 23–58</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> I.V. Dolgachev, V.A. Iskovskikh, "Geometry of algebraic varieties" ''Russian Math. Surveys'' , '''5''' : 6 (1976) pp. 803–864 ''Itogi Nauk. i Tekhn. Algebra Topol. Geom.'' , '''12''' (1974) pp. 77–170</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M. Schlessinger, "Functors of Artin rings" ''Trans. Amer. Math. Soc.'' , '''130''' (1968) pp. 208–222</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> A. Grothendieck (ed.) et al. (ed.) , ''Révètement étales et groupe fondamental, SGA 1'' , ''Lect. notes in math.'' , '''224''' , Springer (1971)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> D.S. Rim, "Formal deformation theory" A. Grothendieck (ed.) et al. (ed.) , ''Groupes de monodromie en géométrie algébrique. SGA 7'' , ''Lect. notes in math.'' , '''288''' , Springer (1972) pp. 32–132</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> D. Mumford, O. Zariski (ed.) , ''Algebraic surfaces'' , Springer (1971) pp. 118–128</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M. Artin, "Algebraic approximation of structures over complete local rings" ''Publ. Math. IHES'' , '''36''' (1969) pp. 23–58 {{MR|0268188}} {{ZBL|0181.48802}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> I.V. Dolgachev, V.A. Iskovskikh, "Geometry of algebraic varieties" ''Russian Math. Surveys'' , '''5''' : 6 (1976) pp. 803–864 ''Itogi Nauk. i Tekhn. Algebra Topol. Geom.'' , '''12''' (1974) pp. 77–170 {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M. Schlessinger, "Functors of Artin rings" ''Trans. Amer. Math. Soc.'' , '''130''' (1968) pp. 208–222 {{MR|0217093}} {{ZBL|0167.49503}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> A. Grothendieck (ed.) et al. (ed.) , ''Révètement étales et groupe fondamental, SGA 1'' , ''Lect. notes in math.'' , '''224''' , Springer (1971) {{MR|2017446}} {{ZBL|}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> D.S. Rim, "Formal deformation theory" A. Grothendieck (ed.) et al. (ed.) , ''Groupes de monodromie en géométrie algébrique. SGA 7'' , ''Lect. notes in math.'' , '''288''' , Springer (1972) pp. 32–132 {{MR|}} {{ZBL|0246.14001}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> D. Mumford, O. Zariski (ed.) , ''Algebraic surfaces'' , Springer (1971) pp. 118–128 {{MR|0469915}} {{ZBL|0138.42002}} </TD></TR></table> |
''I.V. Dolgachev'' | ''I.V. Dolgachev'' | ||
| Line 88: | Line 88: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1a]</TD> <TD valign="top"> A. Nijenhuis, R.W. Richardson Jr., "Cohomology and deformations in graded Lie algebras" ''Bull. Amer. Math. Soc.'' , '''72''' : 1 (1966) pp. 1–29</TD></TR><TR><TD valign="top">[1b]</TD> <TD valign="top"> A. Nijenhuis, R.W. Richardson Jr., "Deformations of homomorphisms of Lie groups and Lie algebras" ''Bull. Amer. Math. Soc.'' , '''73''' : 1 (1967) pp. 175–179</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> M. Gerstenhaber, "On the deformation of rings and algebras" ''Ann. of Math.'' , '''79''' (1964) pp. 59–103</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> D. Knudson, "On the deformation of commutative algebras" ''Trans. Amer. Math. Soc.'' , '''140''' (1969) pp. 55–70</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> E. Inönü, E.P. Wigner, "On the contraction of groups and their representations" ''Proc. Nat. Acad. Sci. USA'' , '''39''' : 6 (1953) pp. 510–524</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> R. Hermann, "Lie groups for physicists" , Benjamin (1966)</TD></TR></table> | + | <table><TR><TD valign="top">[1a]</TD> <TD valign="top"> A. Nijenhuis, R.W. Richardson Jr., "Cohomology and deformations in graded Lie algebras" ''Bull. Amer. Math. Soc.'' , '''72''' : 1 (1966) pp. 1–29 {{MR|0195995}} {{ZBL|0136.30502}} </TD></TR><TR><TD valign="top">[1b]</TD> <TD valign="top"> A. Nijenhuis, R.W. Richardson Jr., "Deformations of homomorphisms of Lie groups and Lie algebras" ''Bull. Amer. Math. Soc.'' , '''73''' : 1 (1967) pp. 175–179 {{MR|0204575}} {{ZBL|0153.04402}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> M. Gerstenhaber, "On the deformation of rings and algebras" ''Ann. of Math.'' , '''79''' (1964) pp. 59–103 {{MR|0171807}} {{ZBL|0123.03101}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> D. Knudson, "On the deformation of commutative algebras" ''Trans. Amer. Math. Soc.'' , '''140''' (1969) pp. 55–70 {{MR|0240091}} {{ZBL|0199.36102}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> E. Inönü, E.P. Wigner, "On the contraction of groups and their representations" ''Proc. Nat. Acad. Sci. USA'' , '''39''' : 6 (1953) pp. 510–524 {{MR|0055352}} {{ZBL|0050.02601}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> R. Hermann, "Lie groups for physicists" , Benjamin (1966) {{MR|0213463}} {{ZBL|0135.06901}} </TD></TR></table> |
''A.A. BoyarkinA.V. MikhalevA.L. Onishchik'' | ''A.A. BoyarkinA.V. MikhalevA.L. Onishchik'' | ||
| Line 108: | Line 108: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M. Gerstenhaber, D. Shack, "Algebraic cohomology and deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 11–264</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> R. Rochberg, "Deformation theory for algebras of analytic functions" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 501–536</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E. Christensen, "Close operator algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 537–556</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> K. Jarosz, "Perturbations of Banach algebras" , Springer (1985)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> B.E. Johnson, "Perturbations of multiplication and homomorphisms" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 565–579</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> A. Lichnerowicz, "Applications of the deformations of algebraic structures to geometry and mathematical physics" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 855–896</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> F. Bayen, M. Flato, C. Fronsdal, A. Lichnerowicz, D. Sternheimer, "Deformation theory and quantization" ''Ann. of Physics'' , '''111''' (1978) pp. 61–110; 111–151</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> M. de Wilde, P. Lecomte, "Invariant deformations of the Poisson Lie algebra of a symplectic manifold and star-products" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 897–960</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> M. Goze, "Perturbations of Lie algebra structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 265–356</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> J.M. Ancochea Bermudez, "On the rigidity of solvable Lie algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 403–446</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> D.G. Babbitt, V.S. Varadarajan, "Local isoformal deformation theory for meromorphic differential equations near an irregular singularity" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 583–700</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988)</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> D. Sternheimer, "Star products: their ubiquity and unicity" J. Bertrand (ed.) et al. (ed.) , ''Modern Group Theoretical Methods in Physics: Proc. Conf. in Honour of Guy Rideau'' , Kluwer (1995) pp. 255–265</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> A. Weinstein, "Deformation quantization" ''Sem. Bourbaki'' , '''789''' (1994)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> A. Connes, "Noncommutative geometry" , Acad. Press (1994)</TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> P. Deligne, "Déformations de l'algèbre des fonctions d'une variété symplectique: comparaison entre Fedosov et De Wilde, Lecomte" ''Selecta Math.'' , '''1''' (1995) pp. 667–697</TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> B. Fedosov, "Deformation quantization and index theory" , Akademie Verlag (1996) (Translated from Russian)</TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> M. Flato, D. Sternheimer, "Star-products, quantum groups, cyclic cohomology and pseudo-differential calculus" P. Sally (ed.) et al. (ed.) , ''Amer. Math. Soc. Contemp. Math.'' , '''175''' (1994) pp. 53–72</TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> M. Flato, "Deformation view of physical theories" ''Czechoslovak J. Phys.'' , '''B32''' (1982) pp. 472–475</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M. Gerstenhaber, D. Shack, "Algebraic cohomology and deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 11–264 {{MR|0981619}} {{ZBL|0676.16022}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> R. Rochberg, "Deformation theory for algebras of analytic functions" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 501–536 {{MR|0981625}} {{ZBL|0701.46042}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E. Christensen, "Close operator algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 537–556 {{MR|0981626}} {{ZBL|0672.46031}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> K. Jarosz, "Perturbations of Banach algebras" , Springer (1985) {{MR|0788884}} {{ZBL|0557.46029}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> B.E. Johnson, "Perturbations of multiplication and homomorphisms" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 565–579 {{MR|0981628}} {{ZBL|0676.46051}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> A. Lichnerowicz, "Applications of the deformations of algebraic structures to geometry and mathematical physics" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 855–896 {{MR|981634}} {{ZBL|0674.58021}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> F. Bayen, M. Flato, C. Fronsdal, A. Lichnerowicz, D. Sternheimer, "Deformation theory and quantization" ''Ann. of Physics'' , '''111''' (1978) pp. 61–110; 111–151 {{MR|0496158}} {{MR|0496157}} {{ZBL|0377.53025}} {{ZBL|0377.53024}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> M. de Wilde, P. Lecomte, "Invariant deformations of the Poisson Lie algebra of a symplectic manifold and star-products" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 897–960 {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> M. Goze, "Perturbations of Lie algebra structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 265–356 {{MR|0981620}} {{ZBL|0715.17009}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> J.M. Ancochea Bermudez, "On the rigidity of solvable Lie algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 403–446 {{MR|}} {{ZBL|0672.17006}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> D.G. Babbitt, V.S. Varadarajan, "Local isoformal deformation theory for meromorphic differential equations near an irregular singularity" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) pp. 583–700 {{MR|0981629}} {{ZBL|0668.34010}} </TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , ''Deformation theory of algebras and structures and applications'' , Kluwer (1988) {{MR|0981617}} {{ZBL|0654.00006}} </TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> D. Sternheimer, "Star products: their ubiquity and unicity" J. Bertrand (ed.) et al. (ed.) , ''Modern Group Theoretical Methods in Physics: Proc. Conf. in Honour of Guy Rideau'' , Kluwer (1995) pp. 255–265 {{MR|1361453}} {{ZBL|0905.17026}} </TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> A. Weinstein, "Deformation quantization" ''Sem. Bourbaki'' , '''789''' (1994) {{MR|2341828}} {{MR|1480730}} {{MR|1321655}} {{ZBL|1146.53072}} {{ZBL|1166.53324}} {{ZBL|0854.58026}} </TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> A. Connes, "Noncommutative geometry" , Acad. Press (1994) {{MR|1303779}} {{ZBL|0818.46076}} </TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> P. Deligne, "Déformations de l'algèbre des fonctions d'une variété symplectique: comparaison entre Fedosov et De Wilde, Lecomte" ''Selecta Math.'' , '''1''' (1995) pp. 667–697 {{MR|1383583}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> B. Fedosov, "Deformation quantization and index theory" , Akademie Verlag (1996) (Translated from Russian) {{MR|1376365}} {{ZBL|0867.58061}} </TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> M. Flato, D. Sternheimer, "Star-products, quantum groups, cyclic cohomology and pseudo-differential calculus" P. Sally (ed.) et al. (ed.) , ''Amer. Math. Soc. Contemp. Math.'' , '''175''' (1994) pp. 53–72 {{MR|1302012}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> M. Flato, "Deformation view of physical theories" ''Czechoslovak J. Phys.'' , '''B32''' (1982) pp. 472–475 {{MR|}} {{ZBL|}} </TD></TR></table> |
A deformation of a subset <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700292.png" /> of a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700293.png" /> is a [[Homotopy|homotopy]] | A deformation of a subset <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700292.png" /> of a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d030/d030700/d030700293.png" /> is a [[Homotopy|homotopy]] | ||
Revision as of 10:03, 24 March 2012
A deformation of an analytic structure is a family of analytic spaces (or analytic objects connected with these spaces) depending on parameters (cf. Analytic space). The theory of deformations originated with the problem of classification of all possible pairwise non-isomorphic complex structures on a given differentiable real manifold. The fundamental idea (which must be credited to B. Riemann) was to introduce an analytic structure on the set of all such structures. The idea was made precise by the following concepts. An analytic family 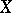 of complex manifolds parametrized by a complex space
of complex manifolds parametrized by a complex space 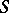 is defined as any smooth (i.e. locally structured as a projection of a direct product with smooth factors) analytic mapping
is defined as any smooth (i.e. locally structured as a projection of a direct product with smooth factors) analytic mapping  . If
. If 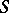 is connected, then all fibres
is connected, then all fibres 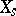 ,
, 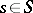 , of
, of 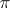 are diffeomorphic to a fixed fibre
are diffeomorphic to a fixed fibre 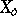 where
where 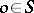 , and may be considered as a family of complex structures on
, and may be considered as a family of complex structures on 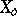 , analytically dependent on the parameter
, analytically dependent on the parameter 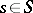 . If the fibres of the family
. If the fibres of the family 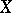 consist all of complex manifolds diffeomorphic to
consist all of complex manifolds diffeomorphic to  and if all fibres are pairwise non-isomorphic,
and if all fibres are pairwise non-isomorphic, 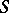 is said to be the moduli space of the real manifold
is said to be the moduli space of the real manifold 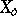 . A moduli space can also be defined for manifolds belonging to a specified class. The problem of constructing a moduli space (or the moduli problem) was first solved for compact Riemann surfaces (cf. Riemann surfaces, conformal classes of). Similar, though incomplete, results were also obtained for compact manifolds of complex dimension 2 (cf. Analytic surface).
. A moduli space can also be defined for manifolds belonging to a specified class. The problem of constructing a moduli space (or the moduli problem) was first solved for compact Riemann surfaces (cf. Riemann surfaces, conformal classes of). Similar, though incomplete, results were also obtained for compact manifolds of complex dimension 2 (cf. Analytic surface).
Considerable difficulties are encountered in the study of moduli problems for higher-dimensional manifolds. In this context K. Kodaira and D.C. Spencer [6], [7], [8] made a local study of moduli problems, thus laying the foundations of the theory of deformation of complex manifolds and analytic bundles. An analytic deformation of a complex manifold 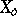 is an analytic family
is an analytic family 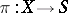 , where
, where 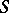 is a complex space with a marked point
is a complex space with a marked point  , with the fibre over it coinciding with
, with the fibre over it coinciding with 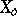 . The deformation
. The deformation 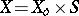 is said to be trivial. A deformation
is said to be trivial. A deformation  of the manifold
of the manifold 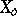 is said to be isomorphic to the deformation
is said to be isomorphic to the deformation 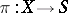 if there exists an analytic isomorphism
if there exists an analytic isomorphism 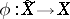 which is the identity on
which is the identity on 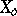 and which is such that
and which is such that 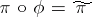 . If
. If 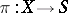 is an analytic deformation, then any analytic mapping
is an analytic deformation, then any analytic mapping 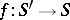 , where
, where 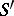 is a space with a marked point
is a space with a marked point 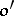 and
and 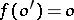 , defines by a base change a deformation
, defines by a base change a deformation 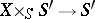 ; the inverse image of this deformation under the mapping
; the inverse image of this deformation under the mapping 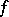 . The deformation
. The deformation 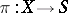 is said to be locally complete (at the point
is said to be locally complete (at the point  ) if any analytic deformation
) if any analytic deformation 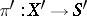 of the manifold
of the manifold 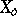 is isomorphic in some neighbourhood of the marked point to its inverse image for some local analytic mapping
is isomorphic in some neighbourhood of the marked point to its inverse image for some local analytic mapping 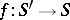 . If
. If 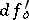 is unambiguously defined, the deformation is said to be versal at
is unambiguously defined, the deformation is said to be versal at  , and if the germ of the mapping
, and if the germ of the mapping 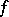 is uniquely defined, the deformation is said to be universal. An important role in the theory is played by the linear mapping
is uniquely defined, the deformation is said to be universal. An important role in the theory is played by the linear mapping 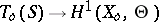 , where
, where 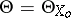 is the sheaf of germs of holomorphic vector fields on
is the sheaf of germs of holomorphic vector fields on 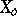 , which is associated an analytic deformation and is named the corresponding infinitesimal deformation.
, which is associated an analytic deformation and is named the corresponding infinitesimal deformation.
The principal theorem of the local theory of deformations, proved by M. Kuranishi [9], states that for each compact complex manifold 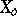 there exists a deformation versal at the point
there exists a deformation versal at the point  , which is parametrized by a (not necessarily smooth) analytic subspace
, which is parametrized by a (not necessarily smooth) analytic subspace 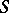 in a neighbourhood of zero of the space
in a neighbourhood of zero of the space 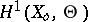 . Here
. Here 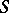 is the fibre at the point
is the fibre at the point  of some local analytic mapping
of some local analytic mapping 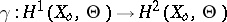 of the form
of the form 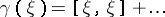 , where
, where 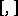 is the operation in the graded Lie algebra
is the operation in the graded Lie algebra 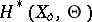 induced by the Lie bracket in the sheaf
induced by the Lie bracket in the sheaf  , the dots denoting terms of order 3 or higher. If
, the dots denoting terms of order 3 or higher. If 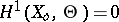 , the manifold
, the manifold 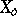 is rigid, i.e. any deformation of it is locally trivial (the Fröhlicher–Nijenhuis rigidity theorem). If
is rigid, i.e. any deformation of it is locally trivial (the Fröhlicher–Nijenhuis rigidity theorem). If 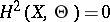 ,
, 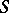 is a neighbourhood of zero in
is a neighbourhood of zero in 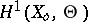 . The tangent space
. The tangent space  always coincides with
always coincides with 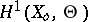 . A deformation is complete at the point
. A deformation is complete at the point  if and only if the corresponding infinitesimal deformation is surjective, and versality is equivalent to bijectivity of the infinitesimal deformation. If
if and only if the corresponding infinitesimal deformation is surjective, and versality is equivalent to bijectivity of the infinitesimal deformation. If 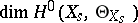 ,
, 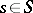 , is constant in a neighbourhood of
, is constant in a neighbourhood of  , the Kuranishi deformation is universal.
, the Kuranishi deformation is universal.
The local theory of deformations of compact complex manifolds may be generalized to include the case of compact complex spaces. The requirements that the mapping 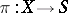 be smooth and that the fibres be compact are then replaced by the requirements that
be smooth and that the fibres be compact are then replaced by the requirements that  be a proper flat mapping. Here, too, it is possible to prove the existence of a deformation versal at the point
be a proper flat mapping. Here, too, it is possible to prove the existence of a deformation versal at the point  [3], [5], [11].
[3], [5], [11].
Studies are also conducted on the deformation of germs of analytic spaces (or, which amounts to the same thing, of analytic algebras). The theorem on the existence of a versal deformation for an isolated singular point of a complex space is valid [4].
In addition to the deformation theories of complex spaces, there also exist deformation theories of various "analytic objects" : analytic bundles, subspaces, mappings, cohomology classes, analytic spaces with additional structures (e.g. with polarization), etc. The principal deformations and the problems involved in these theories are similar to the ones described above. The results obtained for principal analytic bundles are also analogous to the ones above. In particular, for any principal analytic fibration (bundle)  with compact base
with compact base 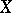 and complex Lie group
and complex Lie group 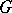 as structure group there exists a deformation of
as structure group there exists a deformation of 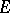 , versal at a point
, versal at a point  , parametrized by an analytic subspace in a neighbourhood of zero of the space
, parametrized by an analytic subspace in a neighbourhood of zero of the space 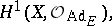 where
where 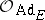 is the sheaf of germs of holomorphic sections of the vector bundle over
is the sheaf of germs of holomorphic sections of the vector bundle over 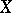 associated with
associated with 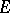 by the adjoint representation [1]. If
by the adjoint representation [1]. If  is a compact Riemann surface and
is a compact Riemann surface and 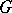 is a reductive algebraic group, it is possible to construct moduli spaces for stable principal analytic bundles. In the theory of deformation of subspaces, on the contrary, one obtains quite general results of a global nature. Thus, if
is a reductive algebraic group, it is possible to construct moduli spaces for stable principal analytic bundles. In the theory of deformation of subspaces, on the contrary, one obtains quite general results of a global nature. Thus, if 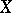 is an arbitrary complex space of finite dimension, a flat analytic family of compact analytic subspaces of
is an arbitrary complex space of finite dimension, a flat analytic family of compact analytic subspaces of 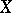 (i.e. an analytic subspace
(i.e. an analytic subspace 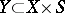 , where
, where 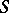 is a complex space and the projection
is a complex space and the projection 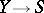 is a proper flat mapping) has been constructed [2], and is a universal (in the global sense) deformation for any compact analytic subspace of
is a proper flat mapping) has been constructed [2], and is a universal (in the global sense) deformation for any compact analytic subspace of 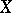 . In particular,
. In particular, 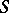 is the moduli space for this problem. A similar moduli problem has also been solved in a related case, and also for compact analytic cycles of a given complex space. The solution of the moduli problem for compact subspaces also entails the solution of the moduli problem for analytic mappings of a given compact complex space into another given complex space.
is the moduli space for this problem. A similar moduli problem has also been solved in a related case, and also for compact analytic cycles of a given complex space. The solution of the moduli problem for compact subspaces also entails the solution of the moduli problem for analytic mappings of a given compact complex space into another given complex space.
Attempts have been made at a unification of the above deformation theories. Each one of these theories may be related to a contravariant functor 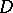 from the category of analytic spaces (or germs of analytic spaces) into the category of sets. For instance, in the theory of local deformations of a complex space
from the category of analytic spaces (or germs of analytic spaces) into the category of sets. For instance, in the theory of local deformations of a complex space 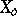 the set
the set 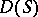 consists of classes of locally isomorphic deformations of the space
consists of classes of locally isomorphic deformations of the space 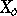 parametrized by an analytic space germ
parametrized by an analytic space germ 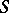 . If
. If 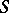 and an element
and an element 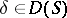 are fixed, there results a morphism of functors
are fixed, there results a morphism of functors 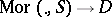 . The surjectivity of this morphism (the pair
. The surjectivity of this morphism (the pair 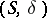 is said to be complete in such a case) corresponds to the property of completeness of the deformation
is said to be complete in such a case) corresponds to the property of completeness of the deformation  , while the bijectivity corresponds to the property of its universality. In this way the moduli problem is connected with the problem of representability of the functor
, while the bijectivity corresponds to the property of its universality. In this way the moduli problem is connected with the problem of representability of the functor 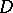 . This stimulated the study of covariant functors from the category of Artinian rings into the category of sets satisfying certain natural conditions [12]. The existence of a complete pair can be proved, but only in the category of formal algebras, which corresponds to the existence of a formally complete deformation (cf. Deformation of an algebraic variety below).
. This stimulated the study of covariant functors from the category of Artinian rings into the category of sets satisfying certain natural conditions [12]. The existence of a complete pair can be proved, but only in the category of formal algebras, which corresponds to the existence of a formally complete deformation (cf. Deformation of an algebraic variety below).
A generalization of the theory of deformations of complex structures on manifolds is the theory of deformations of a pseudo-group structure, the subject of which are families of pseudo-group structures smoothly depending on a parameter which assumes values in a real-analytic space. In particular, the existence of a versal deformation germ has been proved for a pseudo-group structure on a compact smooth manifold, corresponding to an elliptic transitive pseudo-group of transformations [10].
References
| [1] | I.F. Donin, "Construction of a versal family of deformations for holomorphic bundles over a compact complex space" Math. USSR Sb. , 23 : 3 (1974) pp. 405–416 Mat. Sb. , 94 : 3 (1974) pp. 430–443 MR393581 Zbl 0325.32008 |
| [2] | A. Douady, "Le problème des modules pour les sous-espaces analytiques compacts d'un espace analytique donné" Ann. Inst. Fourier , 16 (1966) pp. 1–95 MR0210935 MR0203082 |
| [3] | A. Douady, "Le problème des modules locaux pour les espaces  -analytiques compacts" Ann. Sci. Ecole Norm. Sup. , 7 : 4 (1974) pp. 569–602 MR382729 Zbl 0282.32014 -analytiques compacts" Ann. Sci. Ecole Norm. Sup. , 7 : 4 (1974) pp. 569–602 MR382729 Zbl 0282.32014 |
| [4] | H. Grauert, "Ueber die Deformation isolierter Singularitäten analytischer Menge" Invent. Math. , 15 : 3 (1972) pp. 171–198 |
| [5] | H. Grauert, "Der Satz von Kuranishi für kompakte komplexe Räume" Invent. Math. , 25 : 2 (1974) pp. 107–142 MR0346194 Zbl 0286.32015 |
| [6] | K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures I" Ann. of Math. , 67 : 2 (1958) pp. 328–401 MR0112154 Zbl 0128.16901 |
| [7] | K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures II" Ann. of Math. , 67 : 3 (1958) pp. 403–466 MR112154 Zbl 0128.16901 |
| [8] | K. Kodaira, D.C. Spencer, "On deformations of complex-analytic structures III" Ann. of Math. , 71 : 1 (1960) pp. 43–76 MR0115189 Zbl 0128.16902 |
| [9] | M. Kuranishi, "New proof for the existence of locally complete families of complex structures" , Proc. Conf. Complex Analysis (Minneapolis, 1964) , Springer (1965) pp. 142–154 MR0176496 Zbl 0144.21102 |
| [10] | S.H. Moolgavkar, "On the existence of a universal germ of deformations for elliptic pseudogroup structures on compact manifolds" Trans. Amer. Math. Soc. , 212 : 485 (1975) pp. 173–197 MR0458515 Zbl 0308.58014 |
| [11] | V.P. Palamodov, "Deformations of complex spaces" Russian Math. Surveys , 31 : 3 (1976) pp. 129–197 Usphekhi Mat. Nauk , 31 (1976) pp. 129–194 MR0508121 MR0435451 Zbl 0355.32020 Zbl 0347.32009 |
| [12] | M. Schlessinger, "Functors of Artin rings" Trans. Amer. Math. Soc. , 130 (1968) pp. 208–222 MR0217093 Zbl 0167.49503 |
Comments
Instead of "infinitesimal deformation" it is usual to speak of "Kodaira–Spencer mappingKodaira–Spencer mapping" . For some results and concepts cf. [a1]–[a5]. A comprehensive treatment of the Kodaira–Spencer theory of deformation is [a6].
References
| [a1] | J. Gasqui, H. Goldschmidt, "Complexes of differential operators and symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 797–828 MR0981631 Zbl 0666.58041 |
| [a2] | J. Gasqui, H. Goldschmidt, "Some rigidity results in the deformation theory of symmetric spaces" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 839–851 MR0981633 Zbl 0698.53034 |
| [a3] | J. Gasqui, H. Goldschmidt, "Deformations infinitesimals des structures conformes plates" , Birkhäuser (1984) |
| [a4] | R. Hermann, "Geometric and Lie-theoretic principles in pure and applied deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 701–796 MR0981630 Zbl 0685.58038 |
| [a5] | J.F. Pommaret, "Deformation theory of geometric and algebraic structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 829–838 MR0981632 Zbl 0684.58047 |
| [a6] | K. Kodaira, "Complex manifolds and deformations of complex structures" , Springer (1986) MR0815922 |
A deformation of an algebraic variety is the inclusion of the algebraic variety into a family of algebraic varieties. The theory of deformation of algebraic varieties and schemes is the algebraic analogue of the theory of deformation of analytic structures. Its principal problems are listed below.
Existence of a lift. Let 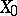 be a scheme over a field
be a scheme over a field 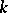 , let
, let 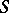 be a scheme and let
be a scheme and let 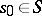 be a point with the residue field
be a point with the residue field 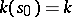 . Does a flat
. Does a flat 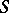 -scheme
-scheme 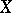 for which the fibre
for which the fibre 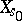 over the point
over the point 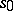 is isomorphic to
is isomorphic to 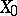 exist? (The
exist? (The 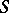 -scheme
-scheme 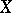 is known as the deformation or lift of the scheme
is known as the deformation or lift of the scheme 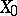 over
over  .)
.)
The universality problem. Does a versal (respectively, universal) deformation of a scheme 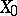 (i.e. a deformation
(i.e. a deformation 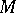 over the scheme
over the scheme 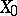 such that for any other deformation
such that for any other deformation 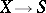 it is possible to find a (respectively, a unique) morphism
it is possible to find a (respectively, a unique) morphism  for which
for which 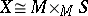 ) exist?
) exist?
Any deformation 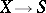 of the scheme
of the scheme 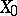 by the operation of formal completion along the fibre
by the operation of formal completion along the fibre 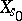 defines a formal deformation
defines a formal deformation 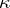 over the completion of the local ring
over the completion of the local ring 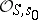 of the scheme
of the scheme 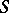 at the point
at the point 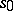 , i.e. a dense formal scheme over
, i.e. a dense formal scheme over 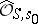 with topological space
with topological space 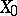 . The formal analogues of the problems listed above are formulated as follows:
. The formal analogues of the problems listed above are formulated as follows:
Existence of a formal deformation. Let a complete local ring 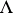 with the residue field
with the residue field 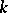 be given. Does a flat formal scheme over
be given. Does a flat formal scheme over 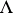 with topological space
with topological space 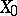 exist?
exist?
Existence of a formal moduli scheme. Does a formal versal (respectively, universal) deformation, i.e. a flat formal scheme 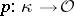 over a complete local ring
over a complete local ring 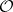 with the residue field
with the residue field 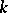 such that for any formal deformation
such that for any formal deformation 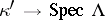 there is a (respectively, a unique) ring homomorphism
there is a (respectively, a unique) ring homomorphism 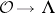 for which
for which 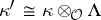 , exist?
, exist?
A universal formal deformation of a smooth variety represents the algebraic analogue of a local moduli space in the theory of deformation of analytic structures.
If 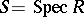 , where
, where 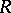 is a local Artinian (respectively, complete) ring with residue field
is a local Artinian (respectively, complete) ring with residue field  , the deformation of
, the deformation of  over
over 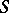 is said to be infinitesimal (respectively, effective formal). If
is said to be infinitesimal (respectively, effective formal). If 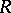 is a complete local ring of characteristic zero (e.g. the ring of Witt vectors, cf. Witt vector), the effective formal deformation of
is a complete local ring of characteristic zero (e.g. the ring of Witt vectors, cf. Witt vector), the effective formal deformation of 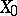 is said to be the lift of
is said to be the lift of 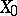 into characteristic zero.
into characteristic zero.
If 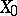 is a smooth
is a smooth 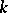 -scheme and
-scheme and 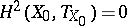 , where
, where 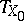 is the tangent bundle on
is the tangent bundle on 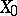 , then for any Artinian (respectively, complete) local ring there exists an infinitesimal (respectively, formal) deformation of
, then for any Artinian (respectively, complete) local ring there exists an infinitesimal (respectively, formal) deformation of 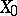 . Moreover, if
. Moreover, if 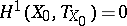 , such a deformation is unique up to isomorphism [4]. Similar statements for not necessarily smooth schemes are given in terms of the cotangent complex [5], [6]. The problem of the existence of an effective formal deformation is studied by means of the functor
, such a deformation is unique up to isomorphism [4]. Similar statements for not necessarily smooth schemes are given in terms of the cotangent complex [5], [6]. The problem of the existence of an effective formal deformation is studied by means of the functor 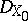 from the category
from the category  of local Artinian rings with residue field
of local Artinian rings with residue field 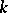 into the category of sets which associates to each object
into the category of sets which associates to each object 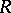 from
from 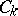 the set of all infinitesimal deformations of
the set of all infinitesimal deformations of 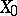 over
over 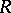 . A universal formal deformation of
. A universal formal deformation of 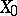 exists if and only if the functor is a pro-representable functor. Here, a pro-representing object — a complete local ring
exists if and only if the functor is a pro-representable functor. Here, a pro-representing object — a complete local ring  with residue field
with residue field 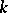 — is said to be a formal scheme of moduli of the
— is said to be a formal scheme of moduli of the 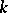 -scheme
-scheme 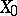 . A formal versal deformation
. A formal versal deformation 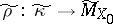 exists if
exists if 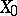 is proper over
is proper over  or if
or if 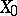 is an affine scheme of finite type over
is an affine scheme of finite type over 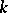 with isolated singular points [2], [6]. A versal formal deformation is universal if for any surjective homomorphisms
with isolated singular points [2], [6]. A versal formal deformation is universal if for any surjective homomorphisms  of Artinian local rings, and any deformation
of Artinian local rings, and any deformation 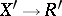 from
from  the natural mapping of automorphism groups
the natural mapping of automorphism groups
 |
is surjective. This condition is satisfied, for example, if 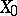 is a smooth scheme and
is a smooth scheme and 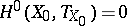 . Here, if
. Here, if 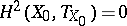 , the formal scheme of moduli
, the formal scheme of moduli  is a complete regular local ring which is isomorphic to the ring
is a complete regular local ring which is isomorphic to the ring 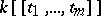 of formal power series in
of formal power series in 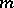 variables. In this case the number
variables. In this case the number 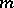 is equal to
is equal to 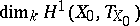 and is called the number of local moduli of the scheme
and is called the number of local moduli of the scheme  . In the general case
. In the general case 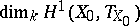 is equal to the dimension of the space tangent to
is equal to the dimension of the space tangent to 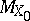 and
and  , i.e. to the dimension
, i.e. to the dimension 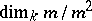 , where
, where 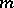 is the maximal ideal of the corresponding local ring, and
is the maximal ideal of the corresponding local ring, and
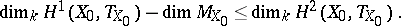 |
The presence of nilpotent elements in a formal scheme of moduli is a fairly frequent phenomenon.
If the versal (respectively, universal) formal deformation 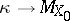 is algebraizable, i.e. if there exists a flat scheme over
is algebraizable, i.e. if there exists a flat scheme over 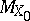 the formal completion of which along a closed fibre is isomorphic to
the formal completion of which along a closed fibre is isomorphic to 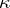 , the corresponding algebraization is said to be a local versal (respectively, universal) deformation of the
, the corresponding algebraization is said to be a local versal (respectively, universal) deformation of the 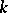 -scheme
-scheme 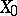 . If
. If  is projective and
is projective and  , the algebraization exists. Thus, for a smooth curve of genus
, the algebraization exists. Thus, for a smooth curve of genus 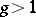 there exists a local universal deformation over the ring
there exists a local universal deformation over the ring 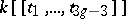 . In the general case, for each polarization
. In the general case, for each polarization  of the variety
of the variety 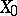 there exists a maximal closed subscheme of moduli
there exists a maximal closed subscheme of moduli 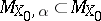 such that
such that 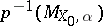 is algebraizable. The codimension of
is algebraizable. The codimension of 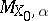 in
in  does not exceed
does not exceed 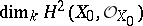 . Thus, if
. Thus, if 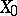 is an algebraic
is an algebraic  -surface,
-surface, 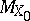 is regular of dimension 20, and for any polarization
is regular of dimension 20, and for any polarization 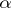 the subscheme
the subscheme 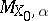 is regular of dimension 19.
is regular of dimension 19.
Artin's approximation theorem is used in the algebraization of formal schemes of moduli. There exists a scheme 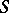 of finite type over
of finite type over 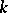 and a point
and a point  with residue field
with residue field 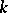 such that the completion
such that the completion 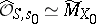 , and there exists a deformation of
, and there exists a deformation of 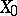 over
over  inducing the versal local deformation
inducing the versal local deformation 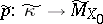 . The scheme
. The scheme 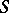 is defined uniquely up to a local isomorphism in the étale topology [1]. For the deformation of singular varieties and singular points see Singular point of an algebraic variety. For the deformation of group schemes see Group scheme.
is defined uniquely up to a local isomorphism in the étale topology [1]. For the deformation of singular varieties and singular points see Singular point of an algebraic variety. For the deformation of group schemes see Group scheme.
References
| [1] | M. Artin, "Algebraic approximation of structures over complete local rings" Publ. Math. IHES , 36 (1969) pp. 23–58 MR0268188 Zbl 0181.48802 |
| [2] | I.V. Dolgachev, V.A. Iskovskikh, "Geometry of algebraic varieties" Russian Math. Surveys , 5 : 6 (1976) pp. 803–864 Itogi Nauk. i Tekhn. Algebra Topol. Geom. , 12 (1974) pp. 77–170 |
| [3] | M. Schlessinger, "Functors of Artin rings" Trans. Amer. Math. Soc. , 130 (1968) pp. 208–222 MR0217093 Zbl 0167.49503 |
| [4] | A. Grothendieck (ed.) et al. (ed.) , Révètement étales et groupe fondamental, SGA 1 , Lect. notes in math. , 224 , Springer (1971) MR2017446 |
| [5] | D.S. Rim, "Formal deformation theory" A. Grothendieck (ed.) et al. (ed.) , Groupes de monodromie en géométrie algébrique. SGA 7 , Lect. notes in math. , 288 , Springer (1972) pp. 32–132 Zbl 0246.14001 |
| [6] | D. Mumford, O. Zariski (ed.) , Algebraic surfaces , Springer (1971) pp. 118–128 MR0469915 Zbl 0138.42002 |
I.V. Dolgachev
A deformation of algebras is a family of algebras depending or parameters. All possible bilinear operations or algebras 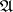 in a space
in a space 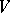 over a field
over a field 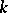 form a vector space,
form a vector space, 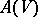 . Two elements of this space represent isomorphic algebras if and only if they lie on the same orbit of the linear group
. Two elements of this space represent isomorphic algebras if and only if they lie on the same orbit of the linear group 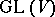 , acting naturally on
, acting naturally on 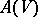 . The theory of deformation of algebras makes it possible to study the local structure of the quotient set
. The theory of deformation of algebras makes it possible to study the local structure of the quotient set 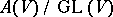 , i.e. the set of classes of isomorphic algebras in the space
, i.e. the set of classes of isomorphic algebras in the space  , a direct description of which involves considerable difficulties. If a certain class of algebras
, a direct description of which involves considerable difficulties. If a certain class of algebras 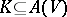 is isolated, one may consider the deformation of the algebras in
is isolated, one may consider the deformation of the algebras in 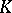 that stay within that class. In particular, one may consider deformations of associative and associative-commutative algebras and Lie algebras, which form the classes
that stay within that class. In particular, one may consider deformations of associative and associative-commutative algebras and Lie algebras, which form the classes 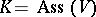 ,
,  ,
, 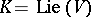 , respectively, which are invariant with respect to the action of the group
, respectively, which are invariant with respect to the action of the group  . If
. If  , these classes are algebraic varieties in the
, these classes are algebraic varieties in the  -dimensional space
-dimensional space 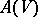 .
.
The theory of deformation of algebras in a finite-dimensional space 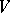 over the field
over the field 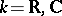 of real or complex numbers in many respects resembles the theory of deformations of analytic structures. Each finite-dimensional algebra
of real or complex numbers in many respects resembles the theory of deformations of analytic structures. Each finite-dimensional algebra 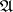 over
over  or
or 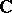 has a complete deformation parametrized by an analytic subspace germ in the zero of the space
has a complete deformation parametrized by an analytic subspace germ in the zero of the space  (if
(if 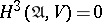 this subspace coincides with
this subspace coincides with 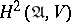 ). The rigidity theorem immediately follows from this fundamental result: If
). The rigidity theorem immediately follows from this fundamental result: If 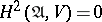 , the algebra
, the algebra 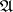 is rigid in
is rigid in 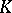 , i.e. the orbit of an element of
, i.e. the orbit of an element of 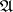 with respect to
with respect to 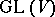 is open in
is open in  . Thus, semi-simple Lie algebras, as well as their Borel subalgebras, are rigid in the class of Lie algebras. The converse proposition is not true. Analogous theorems are valid for finite-dimensional algebras over an arbitrary algebraically closed field
. Thus, semi-simple Lie algebras, as well as their Borel subalgebras, are rigid in the class of Lie algebras. The converse proposition is not true. Analogous theorems are valid for finite-dimensional algebras over an arbitrary algebraically closed field  . For instance, if
. For instance, if  , the orbit of the algebra
, the orbit of the algebra 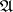 in
in 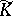 is Zariski open.
is Zariski open.
The theory of deformation of homomorphisms of one finite-dimensional algebra into another may be developed in an analogous manner. In fact, the theories described above form part of a general scheme which involves the use of graded Lie algebras. Similar results have also been obtained for the deformation of subalgebras.
In addition to the above theory there also exists the theory of formal deformations of algebras and their homomorphisms over an arbitrary field 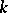 . A formal deformation of an algebra defined in a vector space
. A formal deformation of an algebra defined in a vector space 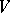 over
over 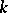 is an algebra over the space
is an algebra over the space 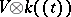 over the field
over the field 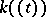 of formal power series over
of formal power series over 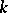 with the operation
with the operation  which is fully determined by the condition
which is fully determined by the condition
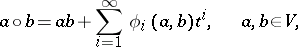 |
where 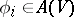 . In requiring the deformation to belong to a given class of algebras, one may speak of formal deformations of associative, associative-commutative, Lie, and other algebras.
. In requiring the deformation to belong to a given class of algebras, one may speak of formal deformations of associative, associative-commutative, Lie, and other algebras.
Two formal deformations of an algebra 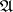 with the multiplications
with the multiplications  and
and  , respectively, are said to be equivalent if there exists a linear automorphism
, respectively, are said to be equivalent if there exists a linear automorphism 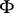 of the space
of the space 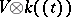 with the property
with the property
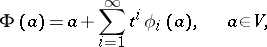 |
where 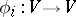 is a linear mapping, such that
is a linear mapping, such that
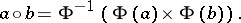 |
A deformation which is equivalent to the algebra 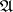 with the initial multiplication is known as trivial. An algebra without non-trivial formal deformations in a given class of algebras is known as formally rigid in that class. For instance, the free algebra in the class of all algebras will be formally rigid. In the classes
with the initial multiplication is known as trivial. An algebra without non-trivial formal deformations in a given class of algebras is known as formally rigid in that class. For instance, the free algebra in the class of all algebras will be formally rigid. In the classes 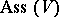 ,
, 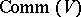 and
and 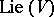 the equation
the equation 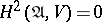 is a sufficient condition of formal rigidity of the algebra
is a sufficient condition of formal rigidity of the algebra 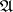 ; in the class
; in the class 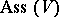 it is also a necessary condition [3].
it is also a necessary condition [3].
If 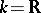 or
or 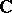 , formal deformations of algebras are a tool in the study of analytic deformations.
, formal deformations of algebras are a tool in the study of analytic deformations.
An important field of applications and a source of examples of deformations of algebras is theoretical physics, in which, in particular, the following class of deformations of an algebra arose [4], [5]. A contraction of a finite-dimensional algebra 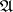 over
over 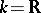 or
or 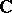 is a continuous curve
is a continuous curve 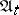 ,
, 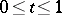 . The algebra
. The algebra 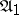 obtained from
obtained from 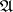 by a contraction is known as the limit algebra and need not be isomorphic to the algebra
by a contraction is known as the limit algebra and need not be isomorphic to the algebra 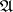 . For instance, any algebra may be contracted into an algebra with zero multiplication; any semi-simple Lie algebra may be contracted into a non-Abelian non-semi-simple algebra.
. For instance, any algebra may be contracted into an algebra with zero multiplication; any semi-simple Lie algebra may be contracted into a non-Abelian non-semi-simple algebra.
References
| [1a] | A. Nijenhuis, R.W. Richardson Jr., "Cohomology and deformations in graded Lie algebras" Bull. Amer. Math. Soc. , 72 : 1 (1966) pp. 1–29 MR0195995 Zbl 0136.30502 |
| [1b] | A. Nijenhuis, R.W. Richardson Jr., "Deformations of homomorphisms of Lie groups and Lie algebras" Bull. Amer. Math. Soc. , 73 : 1 (1967) pp. 175–179 MR0204575 Zbl 0153.04402 |
| [2] | M. Gerstenhaber, "On the deformation of rings and algebras" Ann. of Math. , 79 (1964) pp. 59–103 MR0171807 Zbl 0123.03101 |
| [3] | D. Knudson, "On the deformation of commutative algebras" Trans. Amer. Math. Soc. , 140 (1969) pp. 55–70 MR0240091 Zbl 0199.36102 |
| [4] | E. Inönü, E.P. Wigner, "On the contraction of groups and their representations" Proc. Nat. Acad. Sci. USA , 39 : 6 (1953) pp. 510–524 MR0055352 Zbl 0050.02601 |
| [5] | R. Hermann, "Lie groups for physicists" , Benjamin (1966) MR0213463 Zbl 0135.06901 |
A.A. BoyarkinA.V. MikhalevA.L. Onishchik
Comments
In recent years ideas from non-standard analysis have been applied to deformation problems in algebra. In particular, by this approach rigidity results for Lie algebras have been obtained also in cases with non-vanishing cohomology. For the use of techniques from non-standard analysis in the theory of deformation of algebras, cf. [a9], [a10].
It has now become clear that the deformation theory of algebras is not merely analogous to that of analytic structures but that there are in fact fundamental relations between the two subjects. In particular, the formal deformation theory of a smooth compact complex algebraic variety 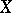 can be reduced to that of a single ring built from
can be reduced to that of a single ring built from 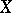 . There is also a relationship between the classical Hodge decomposition of the cohomology of an analytic manifold and the recent Hodge decomposition of the cohomology of a commutative algebra, [a1].
. There is also a relationship between the classical Hodge decomposition of the cohomology of an analytic manifold and the recent Hodge decomposition of the cohomology of a commutative algebra, [a1].
For the relation between deformations of singular and non-singular Riemann surfaces and the deformation theory of the corresponding algebra of analytic functions, cf. [a2].
In the theory of operator and Banach algebras one studies whether algebras close to the given one (in the metric sense) are isomorphic, cf. [a3]–[a5].
There is a deformation-theoretic approach to quantization based on the so-called star-products, which are non-commutative deformations of the commutative product of the ring of functions on a manifold, cf. [a6]–[a8].
For deformations of meromorphic differential equations cf. [a11].
Reference [a12] provides an up-to-date (1988) survey of many aspects of deformation theory, with the notable omission of deformations (bendings) of convex surfaces, resolution of singularities, deformations of vector fields and such in the setting of structural stability of dynamical systems, isospectral and isomonodromy deformations in the setting of integrable systems, and deformations of mechanical structures and solid bodies. Cf. also Deformation, isometric; Deformation over a principal base; Deformation tensor; Integrable system; Resolution of singularities; Stability of an elastic system; Stability in the presence of persistently-acting perturbations; and Perturbation theory, which contain information on other aspects of deformation theory than the ones dealt with above and in [a12].
References
| [a1] | M. Gerstenhaber, D. Shack, "Algebraic cohomology and deformation theory" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 11–264 MR0981619 Zbl 0676.16022 |
| [a2] | R. Rochberg, "Deformation theory for algebras of analytic functions" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 501–536 MR0981625 Zbl 0701.46042 |
| [a3] | E. Christensen, "Close operator algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 537–556 MR0981626 Zbl 0672.46031 |
| [a4] | K. Jarosz, "Perturbations of Banach algebras" , Springer (1985) MR0788884 Zbl 0557.46029 |
| [a5] | B.E. Johnson, "Perturbations of multiplication and homomorphisms" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 565–579 MR0981628 Zbl 0676.46051 |
| [a6] | A. Lichnerowicz, "Applications of the deformations of algebraic structures to geometry and mathematical physics" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 855–896 MR981634 Zbl 0674.58021 |
| [a7] | F. Bayen, M. Flato, C. Fronsdal, A. Lichnerowicz, D. Sternheimer, "Deformation theory and quantization" Ann. of Physics , 111 (1978) pp. 61–110; 111–151 MR0496158 MR0496157 Zbl 0377.53025 Zbl 0377.53024 |
| [a8] | M. de Wilde, P. Lecomte, "Invariant deformations of the Poisson Lie algebra of a symplectic manifold and star-products" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 897–960 |
| [a9] | M. Goze, "Perturbations of Lie algebra structures" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 265–356 MR0981620 Zbl 0715.17009 |
| [a10] | J.M. Ancochea Bermudez, "On the rigidity of solvable Lie algebras" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 403–446 Zbl 0672.17006 |
| [a11] | D.G. Babbitt, V.S. Varadarajan, "Local isoformal deformation theory for meromorphic differential equations near an irregular singularity" M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) pp. 583–700 MR0981629 Zbl 0668.34010 |
| [a12] | M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) MR0981617 Zbl 0654.00006 |
| [a13] | D. Sternheimer, "Star products: their ubiquity and unicity" J. Bertrand (ed.) et al. (ed.) , Modern Group Theoretical Methods in Physics: Proc. Conf. in Honour of Guy Rideau , Kluwer (1995) pp. 255–265 MR1361453 Zbl 0905.17026 |
| [a14] | A. Weinstein, "Deformation quantization" Sem. Bourbaki , 789 (1994) MR2341828 MR1480730 MR1321655 Zbl 1146.53072 Zbl 1166.53324 Zbl 0854.58026 |
| [a15] | A. Connes, "Noncommutative geometry" , Acad. Press (1994) MR1303779 Zbl 0818.46076 |
| [a16] | P. Deligne, "Déformations de l'algèbre des fonctions d'une variété symplectique: comparaison entre Fedosov et De Wilde, Lecomte" Selecta Math. , 1 (1995) pp. 667–697 MR1383583 |
| [a17] | B. Fedosov, "Deformation quantization and index theory" , Akademie Verlag (1996) (Translated from Russian) MR1376365 Zbl 0867.58061 |
| [a18] | M. Flato, D. Sternheimer, "Star-products, quantum groups, cyclic cohomology and pseudo-differential calculus" P. Sally (ed.) et al. (ed.) , Amer. Math. Soc. Contemp. Math. , 175 (1994) pp. 53–72 MR1302012 |
| [a19] | M. Flato, "Deformation view of physical theories" Czechoslovak J. Phys. , B32 (1982) pp. 472–475 |
A deformation of a subset 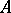 of a space
of a space 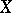 is a homotopy
is a homotopy
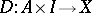 |
for which 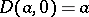 if
if 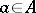 . If, in addition, the set
. If, in addition, the set 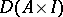 belongs to some subspace
belongs to some subspace 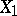 of
of 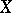 ,
, 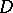 is said to be a deformation of
is said to be a deformation of 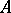 in
in 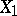 , and
, and 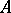 is said to be deformable in
is said to be deformable in 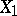 in
in 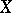 . A space
. A space 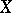 is said to be deformable into a subspace
is said to be deformable into a subspace 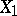 if it is deformable in itself in
if it is deformable in itself in 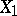 . In particular,
. In particular, 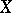 is contractible if and only if it is deformable in one of its points. A space
is contractible if and only if it is deformable in one of its points. A space  is deformable into a subspace
is deformable into a subspace 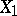 if and only if for the imbedding
if and only if for the imbedding 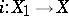 the right homotopy inverse mapping
the right homotopy inverse mapping  exists, i.e.
exists, i.e. 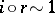 . The concept of deformation of any space in itself in a subspace is related to the concept of a weak retraction.
. The concept of deformation of any space in itself in a subspace is related to the concept of a weak retraction.
M.I. Voitsekhovskii
Deformation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Deformation&oldid=17708