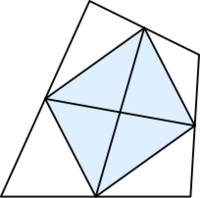
Varignon parallelogram

Take an arbitrary quadrangle and take the midpoints of each of the four sides. Join adjoining midpoints. The result is a parallelogram, called the Varignon parallelogram. This theorem is due to P. Varignon (around 1700). The assertion that the bimedians (i.e. the lines joining opposite midpoints) bisect each other is equivalent to it.

Figure: v130020a

The centre of mass of the Varignon parallelogram is the centroid of the original quadrangle (the centre of mass of four equal masses placed at the four vertices).

A different Varignon theorem deals with sliding vectors.

References

[a1]	H.S.M. Coxeter, "Introduction to geometry" (2nd ed.), Wiley (1969) pp. 199 Zbl 0181.48101; (repr.1989) ISBN 0-471-50458-0
[a2]	H.S.M. Coxeter, S.L. Greitzer, "Geometry revisited" , Math. Assoc. America (1967) pp. 51–56 Zbl 0166.16402

🛠️ This page contains images that should be replaced by better images in the SVG file format. 🛠️