User:Maximilian Janisch/latexlist/latex/35

List

1. 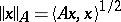 ; $\| x \| _ { A } = \langle A x , x \rangle ^ { 1 / 2 }$ ; confidence 0.059

2.  ; $\alpha ^ { \psi } = Op ( J ^ { 1 / 2 } \alpha )$ ; confidence 0.058

3. 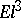 ; $E P$ ; confidence 0.057

4. 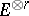 ; $E _ { s } \otimes r$ ; confidence 0.057

5.  ; $\delta _ { \lambda } ( t ) \psi ^ { ( x , y ) _ { \nu } } ( t )$ ; confidence 0.057

6. 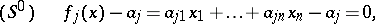 ; $\quad f j ( x ) - \alpha j = \alpha _ { j 1 } x _ { 1 } + \ldots + \alpha _ { j n } x _ { n } - \alpha _ { j } = 0$ ; confidence 0.057

7. 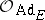 ; $O _ { Ad } _ { E }$ ; confidence 0.056

8. 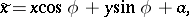 ; $x = x \operatorname { cos } \phi + y \operatorname { sin } \phi + \alpha$ ; confidence 0.056

9. 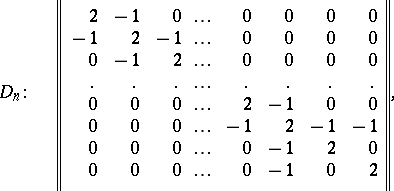 ; $\| \left. \begin{array} { r r r r r r r } { 2 } & { - 1 } & { 0 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 1 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 2 } & { - 1 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 2 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 0 } & { 2 } \end{array} \right. |$ ; confidence 0.055

10. 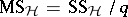 ; $= \operatorname { sin } \gamma q$ ; confidence 0.055

11.  ; $A = \underbrace { \operatorname { lim } _ { n } \frac { \operatorname { lim } } { x \nmid x _ { 0 } } } s _ { n } ( x )$ ; confidence 0.055

12. 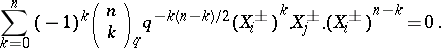 ; $\sum _ { k = 0 } ^ { n } ( - 1 ) ^ { k } \left( \begin{array} { l } { n } \\ { k } \end{array} \right) q ^ { - k ( n - k ) / 2 } ( X _ { i } ^ { \pm } ) ^ { k } X _ { j } ^ { \pm } \cdot ( X _ { i } ^ { \pm } ) ^ { n - k } = 0$ ; confidence 0.055

13. 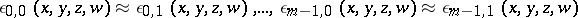 ; $\epsilon 0,0 ( x , y , z , w ) \approx \epsilon 0,1 ( x , y , z , w ) , \ldots , \epsilon _ { m - 1,0 } ( x , y , z , w ) \approx \epsilon _ { m - 1 } , 1 ( x , y , z , w )$ ; confidence 0.055

14. 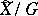 ; $X _ { 1 } G$ ; confidence 0.055

15.  ; $( e ^ { z } 1 ) ^ { z } = e ^ { z } 1 ^ { z _ { 2 } }$ ; confidence 0.053

16.  ; $\alpha _ { 1 } , \ldots , \alpha _ { n } \in F$ ; confidence 0.053

17. 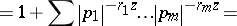 ; $= 1 + \sum | p _ { 1 } | ^ { - r _ { 1 } z } \ldots | p _ { x _ { 2 } } | ^ { - r _ { m } z } =$ ; confidence 0.052

18.  ; $K _ { S } \otimes L , K _ { S } ^ { 2 } \otimes L _ { , }$ ; confidence 0.052

19. 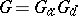 ; $G = G _ { \mathscr { L } } G _ { \mathscr { G } }$ ; confidence 0.052

20. 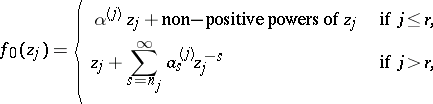 ; $f _ { 0 } ( z _ { j } ) = \left\{ \begin{array} { l l } { \alpha ^ { ( j ) } z _ { j } + \text { non-positive powers of } z _ { j } } & { \text { if } j \leq r } \\ { z _ { j } + \sum _ { s = x _ { j } } ^ { \infty } a _ { s } ^ { ( j ) } z _ { j } ^ { - s } } & { \text { if } j > r } \end{array} \right.$ ; confidence 0.051

21. 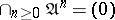 ; $\cap _ { n } \geq 0 \quad 2 ^ { n } = ( 0 )$ ; confidence 0.051

22. 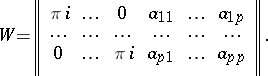 ; $W = \left\| \begin{array} { c c c c c c } { \pi i } & { \ldots } & { 0 } & { a _ { 11 } } & { \ldots } & { a _ { 1 p } } \\ { \cdots } & { \cdots } & { \cdots } & { \cdots } & { \cdots } & { \cdots } \\ { 0 } & { \ldots } & { \pi i } & { a _ { p 1 } } & { \ldots } & { a _ { p p } } \end{array} \right\|$ ; confidence 0.051

23. 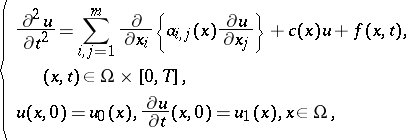 ; $\left. \begin{array}{l}{ \frac { \partial ^ { 2 } u } { \partial t ^ { 2 } } = \sum _ { i , j = 1 } ^ { m } \frac { \partial } { \partial x _ { i } } \{ \alpha _ { j } , ( x ) \frac { \partial u } { \partial x _ { j } } \} + c ( x ) u + f ( x , t ) }\\{ ( x , t ) \in \Omega \times [ 0 , T ] }\\{ u ( x , 0 ) = u _ { 0 } ( x ) , \frac { \partial u } { \partial t } ( x , 0 ) = u _ { 1 } ( x ) , x \in \Omega }\end{array} \right.$ ; confidence 0.050

24. 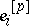 ; $e _ { i } ^ { [ p ] }$ ; confidence 0.050

25.  ; $y _ { L } ( C )$ ; confidence 0.050