Overdetermined system
A system in which the number of equations is greater than the number of unknowns. In the linear case, such a system is given by a rectangular 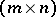 -matrix,
-matrix, 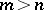 , where
, where 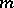 is the number of equations and
is the number of equations and  the number of unknowns. The primary question for an overdetermined system is its solvability, expressed by compatibility conditions.
the number of unknowns. The primary question for an overdetermined system is its solvability, expressed by compatibility conditions.
For example, an overdetermined system of linear algebraic equations
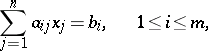 |
is solvable if and only if the ranks of the basic matrix 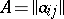 and the expanded matrix obtained by adding the column of free terms to
and the expanded matrix obtained by adding the column of free terms to 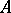 coincide.
coincide.
For an overdetermined system of linear differential equations with constant coefficients,
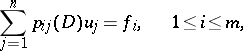 | (1) |
where 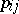 is a polynomial in one variable (an ordinary differential equation) or several variables (a partial differential equation), while
is a polynomial in one variable (an ordinary differential equation) or several variables (a partial differential equation), while  is the symbol of differentiation, the compatibility condition is a homogeneous system of equations with constant coefficients:
is the symbol of differentiation, the compatibility condition is a homogeneous system of equations with constant coefficients:
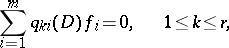 | (2) |
where the matrix 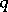 can be algebraically determined from the matrix
can be algebraically determined from the matrix  .
.
If (1) is a system of partial differential equations with variable coefficients 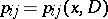 , it is much more difficult to determine compatibility conditions, which take the form (2) with
, it is much more difficult to determine compatibility conditions, which take the form (2) with 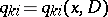 .
.
The simplest example of an overdetermined system is the system of differential equations
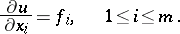 |
The compatibility conditions for this system, necessary and sufficient for it to be solvable, take the form
 |
An analytic function of several complex variables 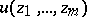 can also be considered as a solution to the overdetermined system of equations
can also be considered as a solution to the overdetermined system of equations
 |
where 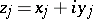 .
.
References
| [1] | A.I. Mal'tsev, "Foundations of linear algebra" , Freeman (1963) (Translated from Russian) |
| [2] | V.V. Palamodov, "Systems of linear differential equations" Progress in Math. , 10 (1971) pp. 1–36 Itogi Nauk. Mat. Anal. (1969) pp. 5–37 |
Comments
The best known overdetermined system of partial differential equations is the Cauchy-Riemann equations for analytic functions in $\mathbb C^n$ with $n>1$. See also Neumann problem.
Overdetermined system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Overdetermined_system&oldid=33902