Cooperative game

A non-strategic game (see Games, theory of), defined by a triple , where is a (usually finite) set whose elements are called players, the subsets are called coalitions, is a real-valued function defined on the set of coalitions, called the characteristic function of the game, and is a subset of vectors (the components correspond to player in ) called the imputations. Cooperative games were first introduced by J. von Neumann, 1928, as a tool in the cooperative theory of (non-cooperative) games.

In the classical theory of cooperative games one takes:

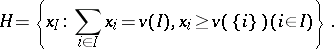On the set one introduces the binary relation 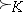of dominance (preference) of the imputations with respect to the coalition :

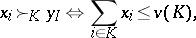If for some , then one writes . Notions of optimality of an imputation are formulated in terms of this relation of dominance.

A significant part of the contents of the theory of cooperative games consists of elaborating the notions of optimality, of proving their realizability for various special classes of cooperative games, and of actually discovering such realizations. Among the principles of optimality that have been developed in connection with cooperative games are the following: double (namely, internal and external) stability, realizable in the form of von Neumann–Morgenstern solutions (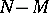-solutions, cf. Solution in game theory); undominated imputations (see Core in the theory of games); stability with respect to threats; stability in the sense of minimization of the greatest insufficiency (see Stability in game theory); fairness (see Shapley vector); etc.

The introduction of algebraic operations in the class of cooperative games leads to the calculus of cooperative games and to the investigation of interrelations between these operations and various principles of optimality. The different special classes of cooperative games described below have been given special attention.

A simple game is a cooperative game in which the characteristic function takes exactly two values (usually 0 and 1); here, coalitions 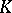on which the maximum value of 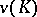is attained are called winning. A special case of simple games is a weighted majority game, in which a coalition 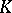is winning if , where () and 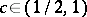are certain constants.

A balanced game is a cooperative game whose characteristic function is such that

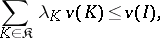if the family of coalitions 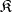and the non-negative numbers 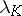() are such that

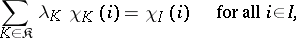where 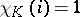if 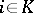and 0 otherwise. Balanced games and only they have a non-empty -core (cf. Core in the theory of games).

A convex game is a cooperative game whose characteristic function is such that for ,

(*)

In a convex game the -core is non-empty and coincides with the -solutions. If a cooperative game is strictly convex (that is, the inequality (*) is strict), then the Shapley vector (value) is the centre of gravity of the -core.

A quota-game is a cooperative game with characteristic function for which there exists a vector 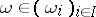such that and for any two players ().

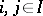A market game is a cooperative game induced by a market, which is taken to be a system

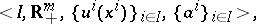where 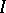is the set of participants in the market (with 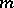commodities), is the initial bundle of commodities of the -th participant and is the utility function of the 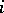-th participant defined on 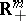. On the basis of such a market a cooperative game is constructed in which

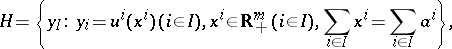while the characteristic function is defined by

The theory of classical cooperative games has undergone generalizations in various directions (see also Non-atomic game).

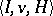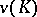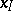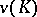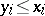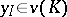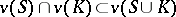Games without side payments are non-strategic games defined by a triple , where (in contrast to classical cooperative games) is a function that associates with each coalition a set of vectors satisfying the following conditions: 1) is closed and convex; 2) if and (), then ; 3) if , then ; 4) 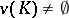for all ; and 5) if and only if 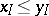for some .

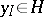Dominance in a game without side payments is defined as follows: if there exists a non-empty coalition such that

A game in partition function form is a non-strategic game defined by a set of players 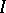and a function that associates with each partition 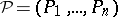of the set a vector . The maximal pay-off that the coalition 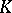itself can guarantee is defined by the formula 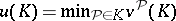. An imputation in a game in partition function form is defined as a vector 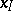satisfying the conditions: 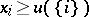(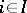); 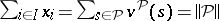for some 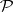. An imputation 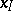dominates an imputation 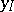with respect to a coalition 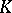if: 1) 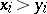(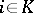); 2) 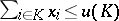; and 3) there exists a 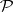such that 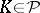and .

References

[1]	J. von Neumann, O. Morgenstern, "Theory of games and economic behavior" , Princeton Univ. Press (1953)
[2]	N.N. Vorob'ev, "The present state of the theory of games" Russian Math. Surveys , 25 : 2 (1970) pp. 81–140 Uspekhi Mat. Nauk , 25 : 2 (1970) pp. 103–107
[3]	I. Rosenmüller, "The theory of games and markets" , North-Holland (1981) (Translated from German)

References

[a1]	J.W. Friedman, "Oligopoly and the theory of games" , North-Holland (1977)
[a2]	J. Szép, F. Forgó, "Introduction to the theory of games" , Reidel (1985)