Barycentric coordinates

Coordinates of a point in an -dimensional vector space , with respect to some fixed system of points that do not lie in an -dimensional subspace. Every point can uniquely be written as

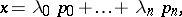where are real numbers satisfying the condition . The point is by definition the centre of gravity of the masses located at the points . The numbers 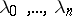are called the barycentric coordinates of the point ; the point with barycentric coordinates is called the barycentre. Barycentric coordinates were introduced by A.F. Möbius in 1827, [1], as an answer to the question about the masses to be placed at the vertices of a triangle so that a given point is the centre of gravity of these masses. Barycentric coordinates are a special case of homogeneous coordinates; they are affine invariants.

Barycentric coordinates of a simplex are used in algebraic topology [2]. Barycentric coordinates of a point of an -dimensional simplex with respect to its vertices 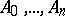is the name given to its (ordinary) Cartesian coordinates in the basis of the vectors 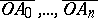, where 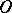is any point that does not lie in the -dimensional subspace carrying 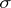(if it is considered that 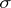lies in some Euclidean space, then the definition does not depend on the point ), or to projective coordinates with respect to in the projective completion of the subspace containing . The barycentric coordinates of the points of a simplex are non-negative and their sum is equal to one. If the 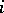-th barycentric coordinate becomes zero, this means that the point lies at the side of the simplex opposite to the vertex . This makes it possible to consider the barycentric coordinates of the points of a geometric complex with respect to all of its vertices. Barycentric coordinates are used to construct the barycentric subdivision of a complex.

Barycentric coordinates of abstract complexes are formally defined in an analogous manner [3].

References

[1]	A.F. Möbius, "Der barycentrische Kalkul" , Gesammelte Werke , 1 , Hirzel , Leipzig (1885)
[2]	L.S. Pontryagin, "Grundzüge der kombinatorischen Topologie" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian)
[3]	E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966)