Zassenhaus algebra
A Lie algebra of special derivations of the divided power algebra
 |
over a field 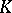 of characteristic
of characteristic 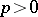 . It is usually denoted by
. It is usually denoted by  , is
, is 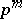 -dimensional and has a basis
-dimensional and has a basis 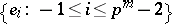 with commutator
with commutator
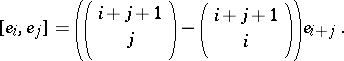 |
It also has another basis,  (if
(if  ), with commutator
), with commutator 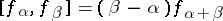 , where
, where 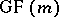 is a finite field of order
is a finite field of order 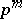 . Zassenhaus algebras appeared first in this form in 1939 [a8] (see also Witt algebra).
. Zassenhaus algebras appeared first in this form in 1939 [a8] (see also Witt algebra). 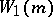 is simple if
is simple if 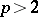 (cf. also Simple algebra), has an ideal of codimension
(cf. also Simple algebra), has an ideal of codimension 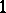 if
if 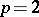 ,
, 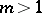 , and is
, and is  -dimensional non-Abelian if
-dimensional non-Abelian if 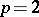 ,
, 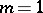 . It is a Lie
. It is a Lie 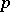 -algebra if and only if
-algebra if and only if 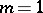 . The
. The 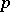 -structure on
-structure on 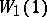 can be given by
can be given by 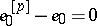 ,
, 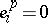 ,
,  . By changing
. By changing 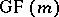 to other additive subgroup of
to other additive subgroup of 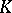 , or by changing the multiplication, one can get different algebras. For example, the multiplication
, or by changing the multiplication, one can get different algebras. For example, the multiplication
 |
where 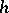 is an additive homomorphism of finite fields, gives rise to the Albert–Zassenhaus algebra.
is an additive homomorphism of finite fields, gives rise to the Albert–Zassenhaus algebra.
Suppose that all algebras and modules are finite-dimensional and that the ground field  is an algebraically closed field of characteristic
is an algebraically closed field of characteristic 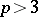 .
.
Let  be the
be the 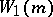 -module defined for
-module defined for 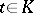 on the vector space
on the vector space 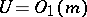 by
by
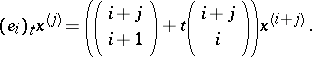 |
For example, 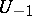 and
and 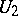 are isomorphic to the adjoint and co-adjoint modules,
are isomorphic to the adjoint and co-adjoint modules, 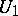 has an irreducible submodule
has an irreducible submodule 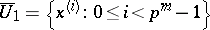 , and
, and 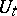 is irreducible if
is irreducible if 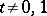 . Any irreducible restricted
. Any irreducible restricted 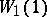 -module is isomorphic to one of the following modules: the
-module is isomorphic to one of the following modules: the 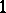 -dimensional trivial module
-dimensional trivial module 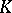 ; the
; the 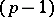 -dimensional module
-dimensional module 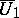 ; or the
; or the  -dimensional module
-dimensional module 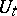 ,
, 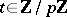 ,
, 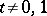 . The maximal dimension of irreducible
. The maximal dimension of irreducible  -modules is
-modules is 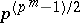 , but there may be infinitely many non-isomorphic irreducible modules of given dimension. The minimal dimension of irreducible non-trivial
, but there may be infinitely many non-isomorphic irreducible modules of given dimension. The minimal dimension of irreducible non-trivial 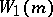 -modules is
-modules is 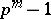 , and any irreducible module of dimension
, and any irreducible module of dimension  is isomorphic to
is isomorphic to 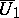 . Any irreducible
. Any irreducible 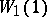 -module with a non-trivial action of
-module with a non-trivial action of 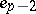 is irreducible as a module over the maximal subalgebra
is irreducible as a module over the maximal subalgebra 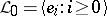 . In any case, any non-restricted irreducible
. In any case, any non-restricted irreducible 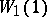 -module is induced by some irreducible submodule of
-module is induced by some irreducible submodule of 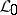 [a3].
[a3].
Any simple Lie algebra of dimension  with a subalgebra of codimension
with a subalgebra of codimension 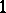 is isomorphic to
is isomorphic to 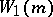 for some
for some 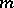 [a4]. Albert–Zassenhaus algebras have subalgebras of codimension
[a4]. Albert–Zassenhaus algebras have subalgebras of codimension  . Any automorphism of
. Any automorphism of 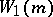 is induced by an admissible automorphism of
is induced by an admissible automorphism of 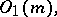 i.e., by an automorphism
i.e., by an automorphism  such that
such that 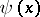 is a linear combination of
is a linear combination of  , where
, where 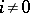 ,
, 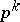 ,
, 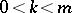 . There are infinitely many non-conjugate Cartan subalgebras (cf. also Cartan subalgebra) of dimension
. There are infinitely many non-conjugate Cartan subalgebras (cf. also Cartan subalgebra) of dimension 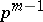 if
if 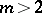 , and exactly two non-conjugate Cartan subalgebras of dimension
, and exactly two non-conjugate Cartan subalgebras of dimension 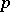 if
if 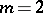 [a2]. The algebra of outer derivations of
[a2]. The algebra of outer derivations of 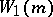 is
is 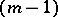 -dimensional and generated by the derivations
-dimensional and generated by the derivations 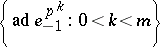 . The algebra
. The algebra 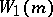 has a
has a 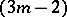 -parametric deformation [a6]. A non-split central extension of
-parametric deformation [a6]. A non-split central extension of  was constructed first by R. Block in 1968 [a1]. The characteristic-
was constructed first by R. Block in 1968 [a1]. The characteristic- infinite-dimensional analogue of this extension is well known as the Virasoro algebra. The list of irreducible
infinite-dimensional analogue of this extension is well known as the Virasoro algebra. The list of irreducible 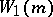 -modules that have non-split extensions is the following:
-modules that have non-split extensions is the following: 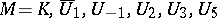 . All bilinear invariant forms of
. All bilinear invariant forms of 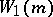 are trivial, but it has a generalized Casimir element
are trivial, but it has a generalized Casimir element 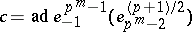 . The centre of the universal enveloping algebra is generated by the
. The centre of the universal enveloping algebra is generated by the 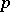 -centre and the generalized Casimir elements [a7].
-centre and the generalized Casimir elements [a7].
References
| [a1] | R.E. Block, "On the extension of Lie algebras" Canad. J. Math. , 20 (1968) pp. 1439–1450 |
| [a2] | G. Brown, "Cartan subalgebras of Zassenhaus algebras" Canad. J. Math. , 27 : 5 (1975) pp. 1011–1021 |
| [a3] | Ho-Jui Chang, "Über Wittsche Lie-Ringe" Abb. Math. Sem. Univ. Hamburg , 14 (1941) pp. 151–184 |
| [a4] | A.S. Dzhumadil'daev, "Simple Lie algebras with a subalgebra of codimension 1" Russian Math. Surveys , 40 : 1 (1985) pp. 215–216 (In Russian) |
| [a5] | A.S. Dzhumadil'daev, "Cohomology and nonsplit extensions of modular Lie algebras" Contemp. Math. , 131:2 (1992) pp. 31–43 |
| [a6] | A.S. Dzhumadil'daev, A.I. Kostrikin, "Deformations of the Lie algebra 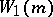 " Proc. Steklov Inst. Math. , 148 (1980) pp. 143–158 (In Russian) " Proc. Steklov Inst. Math. , 148 (1980) pp. 143–158 (In Russian) |
| [a7] | Y.B. Ermolaev, "On structure of the center of the universal enveloping algebra of a Zassenhaus algebra" Soviet Math. (Iz.VUZ) , 20 (1978) Izv. VUZ Mat. , 12(199) (1978) pp. 46–59 |
| [a8] | H. Zassenhaus, "Über Lie'she Ringe mit Primzahlcharacteristik" Abh. Math. Sem. Univ. Hamburg , 13 (1939) pp. 1–100 |
Zassenhaus algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Zassenhaus_algebra&oldid=14470