Unitary operator
From Encyclopedia of Mathematics
A linear operator 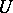 mapping a normed linear space
mapping a normed linear space 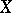 onto a normed linear space
onto a normed linear space 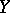 such that
such that 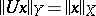 . The most important unitary operators are those mapping a Hilbert space onto itself. Such an operator is unitary if and only if
. The most important unitary operators are those mapping a Hilbert space onto itself. Such an operator is unitary if and only if  for all
for all  . Other characterizations of a unitary operator
. Other characterizations of a unitary operator 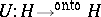 are: 1)
are: 1) 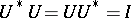 , i.e.
, i.e. 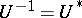 ; and 2) the spectrum of
; and 2) the spectrum of  lies on the unit circle and there is the spectral decomposition
lies on the unit circle and there is the spectral decomposition 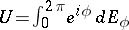 . The set of unitary operators acting on
. The set of unitary operators acting on 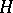 forms a group.
forms a group.
Examples of unitary operators and their inverses on the space  are the Fourier transform and its inverse.
are the Fourier transform and its inverse.
References
| [1] | F. Riesz, B. Szökefalvi-Nagy, "Functional analysis" , F. Ungar (1955) (Translated from French) |
| [2] | N.I. Akhiezer, I.M. Glazman, "Theory of linear operators in Hilbert space" , 1 , Pitman (1980) (Translated from Russian) |
| [3] | A.I. Plessner, "Spectral theory of linear operators" , F. Ungar (1965) (Translated from Russian) |
How to Cite This Entry:
Unitary operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Unitary_operator&oldid=14352
Unitary operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Unitary_operator&oldid=14352
This article was adapted from an original article by V.I. Sobolev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article