Topological dynamical system
A triple  , where
, where  is a topological space,
is a topological space,  is a topological group and
is a topological group and  is a continuous function
is a continuous function  defining a left action of
defining a left action of  on
on  : If
: If  ,
,  is the identity element of
is the identity element of 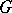 and
and 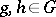 , then (using multiplicative notation for the operation in
, then (using multiplicative notation for the operation in 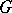 )
) 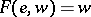 and
and
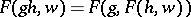 | (1) |
(in other words, if one denotes the transformation 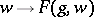 by
by 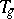 , then
, then 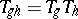 ). Instead of a left action one often considers a right action. In this case the arguments of
). Instead of a left action one often considers a right action. In this case the arguments of 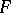 are usually written in the other order (expressing
are usually written in the other order (expressing 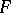 as a mapping
as a mapping 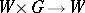 ), and (1) is replaced by the condition
), and (1) is replaced by the condition
 | (2) |
Instead of  or
or 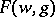 one often writes simply
one often writes simply 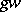 or
or 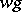 . Then (1) and (2) are written in the form
. Then (1) and (2) are written in the form
 |
If  is commutative, then there is no essential difference between a left and a right action. The most important cases are
is commutative, then there is no essential difference between a left and a right action. The most important cases are  (the additive group of integers with the discrete topology; in this case one speaks of a (topological) cascade) and
(the additive group of integers with the discrete topology; in this case one speaks of a (topological) cascade) and 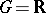 (in this case one speaks of a (topological) flow). In the narrow sense, topological dynamical systems refer to these two cases. Sometimes
(in this case one speaks of a (topological) flow). In the narrow sense, topological dynamical systems refer to these two cases. Sometimes 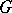 is not a group but a semi-group. Basically, however, one considers only the semi-group of non-negative integers (in other words, one considers iteration of some continuous mapping
is not a group but a semi-group. Basically, however, one considers only the semi-group of non-negative integers (in other words, one considers iteration of some continuous mapping  ) or (more rarely) of non-negative real numbers.
) or (more rarely) of non-negative real numbers.
The term "topological dynamical system" (usually without the first adjective) belongs to topological dynamics, while in topology the same object is called a continuous transformation group. The different terminologies are partly due to the fact that the two disciplines study different properties of the object, and impose different restrictions on it. Thus, a lot of results in topology concern a compact group 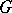 , whereas in topological dynamics
, whereas in topological dynamics  is usually taken to be locally compact, but never compact, and the interest is in the limiting behaviour of the trajectory
is usually taken to be locally compact, but never compact, and the interest is in the limiting behaviour of the trajectory  as
as  (that is, outside arbitrarily large compact parts of
(that is, outside arbitrarily large compact parts of  ), which even in the analytic case can be extremely complicated. In the theory of algebraic transformation groups (cf. Algebraic group of transformations) one does not assume compactness of
), which even in the analytic case can be extremely complicated. In the theory of algebraic transformation groups (cf. Algebraic group of transformations) one does not assume compactness of 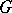 , but on the other hand there is a very strong condition of regularity for
, but on the other hand there is a very strong condition of regularity for 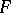 as a mapping between algebraic varieties (where the ground field is usually assumed to be algebraically closed, so that in the classical case one is talking about regularity "in the entire complex domain" ). Combined with connectivity (and usually also reductivity) of
as a mapping between algebraic varieties (where the ground field is usually assumed to be algebraically closed, so that in the classical case one is talking about regularity "in the entire complex domain" ). Combined with connectivity (and usually also reductivity) of 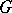 , this enables one, as in the compact case, to obtain significant information about the possible types of mutually adjoining orbits (cf. Orbit), and, in particular, to exclude various phenomena associated with complicated limiting behaviour of trajectories.
, this enables one, as in the compact case, to obtain significant information about the possible types of mutually adjoining orbits (cf. Orbit), and, in particular, to exclude various phenomena associated with complicated limiting behaviour of trajectories.
The term "topological dynamical system" should be preferred to the commonly used "continuous dynamical system (a flow, a cascade)" , because "continuity" can mean also: a) metric continuity, cf. Continuous flow 1); and b)  . (When a topological dynamical system is taken in the narrow sense, one says that a flow is the case of continuous time, and a cascade is the case of discrete time. One sometimes speaks of a continuous and a discrete dynamical system, respectively.)
. (When a topological dynamical system is taken in the narrow sense, one says that a flow is the case of continuous time, and a cascade is the case of discrete time. One sometimes speaks of a continuous and a discrete dynamical system, respectively.)
Comments
For references cf. also Topological dynamics.
References
| [a1] | J. de Vries, "Topological transformation groups" , I , Math. Centre (1975) |
| [a2] | R. Ellis, "Lectures on topological dynamics" , Benjamin (1969) |
| [a3] | I.U. Bronshtein, "Extensions of minimal transformation groups" , Sijthoff & Noordhoff (1979) (Translated from Russian) |
Topological dynamical system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Topological_dynamical_system&oldid=15816