Thom spectrum
A spectrum of spaces, equivalent to the spectrum associated to a certain structure series (cf.  -structure).
-structure).
Let  be a structure series, and let
be a structure series, and let  be the bundle over
be the bundle over  induced by the mapping
induced by the mapping  . Let
. Let  be the Thom space of
be the Thom space of  . The mapping
. The mapping  induces a mapping
induces a mapping  , where
, where 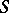 is suspension and
is suspension and 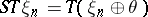 (
( is the one-dimensional trivial bundle). One obtains a spectrum of spaces
is the one-dimensional trivial bundle). One obtains a spectrum of spaces 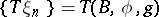 , associated with the structure series
, associated with the structure series  , and a Thom spectrum is any spectrum that is (homotopy) equivalent to a spectrum of the form
, and a Thom spectrum is any spectrum that is (homotopy) equivalent to a spectrum of the form 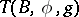 . It represents
. It represents  -cobordism theory. Thus, the series of classical Lie groups
-cobordism theory. Thus, the series of classical Lie groups 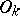 ,
,  ,
,  , and
, and 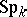 lead to the Thom spectra
lead to the Thom spectra 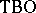 ,
,  ,
,  , and
, and  .
.
Let 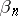 be Artin's braid group on
be Artin's braid group on  strings (cf. Braid theory). The homomorphism
strings (cf. Braid theory). The homomorphism  , where
, where  is the symmetric group, yields a mapping
is the symmetric group, yields a mapping 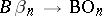 such that a structure series arises (
such that a structure series arises ( is canonically imbedded in
is canonically imbedded in  ). The corresponding Thom spectrum is equivalent to the Eilenberg–MacLane spectrum
). The corresponding Thom spectrum is equivalent to the Eilenberg–MacLane spectrum 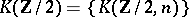 , so that
, so that  is a Thom spectrum (cf. [1], [2]). Analogously,
is a Thom spectrum (cf. [1], [2]). Analogously, 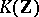 is a Thom spectrum, but using sphere bundles, [3].
is a Thom spectrum, but using sphere bundles, [3].
References
| [1] | M. Mahowold, "A new infinite family in 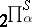 " Topology , 16 (1977) pp. 249–256 " Topology , 16 (1977) pp. 249–256 |
| [2] | S. Priddy, " as a Thom spectrum" Proc. Amer. Math. Soc. , 70 : 2 (1978) pp. 207–208 as a Thom spectrum" Proc. Amer. Math. Soc. , 70 : 2 (1978) pp. 207–208 |
| [3] | M. Mahowold, "Ring spectra which are Thom complexes" Duke Math. J. , 46 : 3 (1979) pp. 549–559 |
Thom spectrum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Thom_spectrum&oldid=18456