Sturm-Liouville theory
Sturm–Liouville problems (cf. Sturm–Liouville problem) have continued to provide new ideas and interesting developments in the spectral theory of operators (cf. also Spectral theory).
Consider the Sturm–Liouville differential equation on the half-line 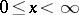 , in its reduced form
, in its reduced form
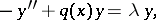 | (a1) |
where 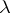 is the complex spectral parameter and the real-valued function
is the complex spectral parameter and the real-valued function 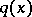 is assumed to be integrable over any finite subinterval of
is assumed to be integrable over any finite subinterval of  . The time-independent Schrödinger equation, at energy
. The time-independent Schrödinger equation, at energy 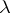 , for a particle having fixed angular momentum quantum numbers moving in a spherically symmetric potential, may be written in the form (a1) — hence there are numerous applications to quantum mechanics ([a1], [a2]).
, for a particle having fixed angular momentum quantum numbers moving in a spherically symmetric potential, may be written in the form (a1) — hence there are numerous applications to quantum mechanics ([a1], [a2]).
Suppose the end-point 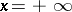 is a limit point. This holds in almost all applications and is valid, for example, if either
is a limit point. This holds in almost all applications and is valid, for example, if either 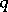 is bounded or if
is bounded or if  satisfies the inequality
satisfies the inequality 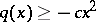 for some positive constant
for some positive constant  . Let
. Let  denote the second-order differential operator
denote the second-order differential operator 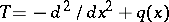 , defined as a self-adjoint operator in
, defined as a self-adjoint operator in 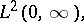 subject to the Dirichlet boundary condition
subject to the Dirichlet boundary condition 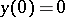 (cf. also Linear ordinary differential equation of the second order). (Other boundary conditions may be considered — in general, there is a one-parameter family of boundary conditions
(cf. also Linear ordinary differential equation of the second order). (Other boundary conditions may be considered — in general, there is a one-parameter family of boundary conditions
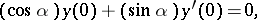 | (a2) |
with the real parameter 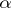 varying over the interval
varying over the interval 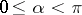 .)
.)
The eigenvalues of the Sturm–Liouville operator 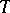 may be characterized as those
may be characterized as those 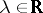 for which the differential equation has a (non-trivial) solution
for which the differential equation has a (non-trivial) solution  satisfying both the boundary condition
satisfying both the boundary condition 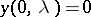 and the
and the 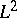 condition
condition  . The solution
. The solution  will always be locally square-integrable, and the
will always be locally square-integrable, and the 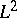 condition is a restriction on the large-
condition is a restriction on the large- asymptotic behaviour of this function. It follows, therefore, that the set of discrete points of the spectrum of
asymptotic behaviour of this function. It follows, therefore, that the set of discrete points of the spectrum of 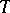 (cf. also Spectrum of an operator) is governed by the asymptotic behaviour of appropriate solutions of (a1). Such considerations, which link asymptotic behaviour of solutions
(cf. also Spectrum of an operator) is governed by the asymptotic behaviour of appropriate solutions of (a1). Such considerations, which link asymptotic behaviour of solutions 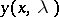 of (a1) to spectral properties of the Sturm–Liouville operator
of (a1) to spectral properties of the Sturm–Liouville operator 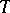 , may be extended to other parts of the spectrum of
, may be extended to other parts of the spectrum of 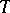 , and provide a powerful tool of spectral analysis.
, and provide a powerful tool of spectral analysis.
As a preliminary straightforward application of this general idea, one may use a Weyl sequence of approximate eigenfunctions of 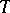 to show that if
to show that if  as
as  ; then the entire positive line
; then the entire positive line 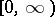 belongs to the essential spectrum of
belongs to the essential spectrum of 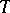 , the essential spectrum consisting of all points of the spectrum of
, the essential spectrum consisting of all points of the spectrum of 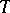 apart from isolated eigenvalues. In contrast, if
apart from isolated eigenvalues. In contrast, if  as
as 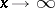 , then no
, then no 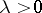 belongs to the essential spectrum.
belongs to the essential spectrum.
In order to carry out a more detailed spectral analysis of the Sturm–Liouville operator 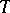 , one has to consider the spectral measure
, one has to consider the spectral measure 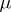 associated with
associated with 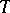 , as well as its spectral decomposition. One of the most convenient ways to do this is through the Weyl
, as well as its spectral decomposition. One of the most convenient ways to do this is through the Weyl 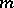 -function for
-function for 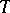 ([a3], [a4], [a5], [a6]), here denoted by
([a3], [a4], [a5], [a6]), here denoted by 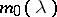 (cf. also Titchmarsh–Weyl
(cf. also Titchmarsh–Weyl 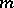 -function). The
-function). The 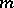 -function
-function 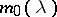 for the Dirichlet Sturm–Liouville operator
for the Dirichlet Sturm–Liouville operator 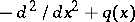 on the half-line is uniquely defined for
on the half-line is uniquely defined for  by the condition that
by the condition that
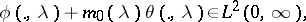 | (a3) |
where  ,
, 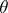 are solutions of (a1), subject, respectively, to the conditions
are solutions of (a1), subject, respectively, to the conditions
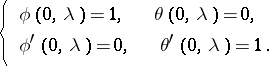 | (a4) |
The function 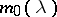 is an analytic function of
is an analytic function of 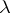 in the upper half-plane, and has strictly positive imaginary part. Such functions are called Herglotz functions, or Nevanlinna functions. (Corresponding to a general boundary condition, as given by (a2), one can define in a similar way an
in the upper half-plane, and has strictly positive imaginary part. Such functions are called Herglotz functions, or Nevanlinna functions. (Corresponding to a general boundary condition, as given by (a2), one can define in a similar way an  -function
-function 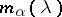 , which is again a Herglotz function, to which the theory outlined below applies with minor modifications.)
, which is again a Herglotz function, to which the theory outlined below applies with minor modifications.)
As a Herglotz function, 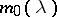 has a representation of the form ([a7]; cf. also Herglotz formula)
has a representation of the form ([a7]; cf. also Herglotz formula)
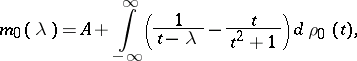 | (a5) |
valid for all 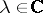 with
with 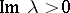 . (Actually, for a general Herglotz function, a term
. (Actually, for a general Herglotz function, a term 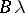 , linear in
, linear in  with
with 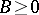 , must be added on the right-hand side, but the asymptotics of
, must be added on the right-hand side, but the asymptotics of  -functions imply that here
-functions imply that here 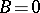 [a7].)
[a7].)
In (a6), 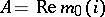 is a positive constant and
is a positive constant and 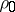 is the spectral function for the problem (a1) with Dirichlet boundary condition at
is the spectral function for the problem (a1) with Dirichlet boundary condition at 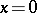 . The spectral function may be taken to be non-decreasing and right continuous, in which case
. The spectral function may be taken to be non-decreasing and right continuous, in which case 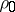 is defined by (a5) up to an additive constant, for a given
is defined by (a5) up to an additive constant, for a given 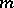 -function
-function 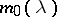 . The measure
. The measure 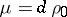 , defined on Borel subsets of
, defined on Borel subsets of 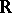 , is called the spectral measure associated with the Dirichlet problem.
, is called the spectral measure associated with the Dirichlet problem.
The Lebesgue decomposition theorem (cf. Lebesgue theorem) leads to a decomposition of the spectral measure into the sum of a part absolutely continuous with respect to Lebesgue measure and a singular part, i.e.
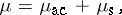 | (a6) |
where 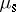 may be further decomposed into its singular continuous and discrete components, thus
may be further decomposed into its singular continuous and discrete components, thus
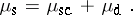 | (a7) |
The Radon–Nikodým theorem implies that the absolutely continuous part 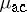 of the spectral measure may be described by means of a density function
of the spectral measure may be described by means of a density function  given at (Lebesgue) almost all
given at (Lebesgue) almost all 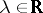 by
by 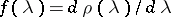 ; thus, for Borel subsets
; thus, for Borel subsets  of
of 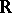 one then has
one then has 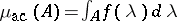 . The support of the singular component
. The support of the singular component 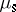 will be a set
will be a set  having Lebesgue measure zero. The discrete part
having Lebesgue measure zero. The discrete part 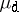 is supported on the set of eigenvalues of the Sturm–Liouville operator
is supported on the set of eigenvalues of the Sturm–Liouville operator 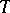 (cf. also Eigen value). These may be characterized as the points
(cf. also Eigen value). These may be characterized as the points 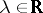 for which
for which 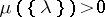 , and alternatively as the points of discontinuity of the spectral function
, and alternatively as the points of discontinuity of the spectral function  .
.
For many physical applications of the Sturm–Liouville problem (a1), the spectrum of the associated differential operator with Dirichlet boundary condition is either purely discrete (e.g. if 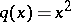 ), or purely absolutely continuous (e.g. if
), or purely absolutely continuous (e.g. if 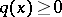 and
and  ), or a combination of discrete and absolutely continuous spectrum (e.g. if
), or a combination of discrete and absolutely continuous spectrum (e.g. if 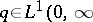 ) and
) and 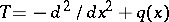 is not a positive operator). However, solution of the inverse Sturm–Liouville problem (cf. also Sturm–Liouville problem, inverse), which leads to the determination of a function
is not a positive operator). However, solution of the inverse Sturm–Liouville problem (cf. also Sturm–Liouville problem, inverse), which leads to the determination of a function 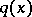 from its spectral measure
from its spectral measure 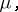 shows that other types of spectra, including for example combinations of absolutely continuous, singular continuous and discrete spectra, are possible. In view of the generality of types of spectral behaviour, mathematicians have sought ways of further characterizing the spectral properties of Sturm–Liouville operators which will apply to a wide range of cases.
shows that other types of spectra, including for example combinations of absolutely continuous, singular continuous and discrete spectra, are possible. In view of the generality of types of spectral behaviour, mathematicians have sought ways of further characterizing the spectral properties of Sturm–Liouville operators which will apply to a wide range of cases.
The supports of the various components of the spectral measure may be characterized in terms of the boundary behaviour of the 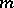 -function
-function 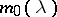 .
.
For (Lebesgue) almost-all 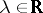 , define the boundary value function
, define the boundary value function  by
by
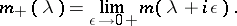 | (a8) |
Here 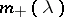 exists as a finite limit for almost-all
exists as a finite limit for almost-all 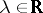 , and one defines
, and one defines 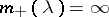 whenever
whenever 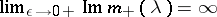 . Then:
. Then:
i) the set of all 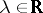 at which
at which 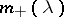 exists and is real and finite, has zero
exists and is real and finite, has zero  -measure;
-measure;
ii) 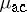 is supported on the set of all
is supported on the set of all  at which
at which 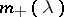 exists finitely, with
exists finitely, with 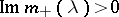 ; the density function for the measure
; the density function for the measure 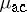 is then
is then 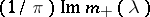 ;
;
iii) 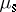 is supported on the set of all
is supported on the set of all 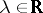 at which
at which 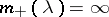 . These supports can also be characterized in terms of large-
. These supports can also be characterized in terms of large- asymptotics of solutions of (a1), by using the notion of subordinacy. A non-trivial solution
asymptotics of solutions of (a1), by using the notion of subordinacy. A non-trivial solution 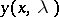 , for given
, for given 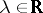 , is said to be subordinate if the norm of
, is said to be subordinate if the norm of 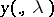 in
in 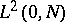 is much smaller, in the limit
is much smaller, in the limit  , than that of any other solution of (a1) that is not a constant multiple of
, than that of any other solution of (a1) that is not a constant multiple of 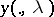 . That is,
. That is, 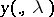 is subordinate if, for any other solution
is subordinate if, for any other solution 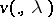 linearly independent of
linearly independent of 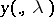 , one has (see [a8], and [a9] for extensions to operators with two singular end-points)
, one has (see [a8], and [a9] for extensions to operators with two singular end-points)
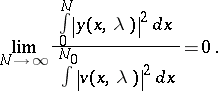 |
Then the following result holds, linking subordinacy with boundary behaviour of the  -function, and thereby to the spectral analysis of Sturm–Liouville operators: A subordinate solution of (a1) exists, at real spectral parameter
-function, and thereby to the spectral analysis of Sturm–Liouville operators: A subordinate solution of (a1) exists, at real spectral parameter 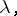 if and only if either
if and only if either
a) 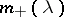 exists and is real and finite (in which case the solution
exists and is real and finite (in which case the solution 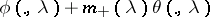 is subordinate); or
is subordinate); or
b) 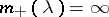 (in which case
(in which case 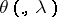 is subordinate). In particular, the singular component
is subordinate). In particular, the singular component 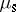 of the spectral measure is concentrated on the set of
of the spectral measure is concentrated on the set of 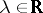 at which the solution
at which the solution 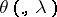 is subordinate, and
is subordinate, and  is concentrated on the set of
is concentrated on the set of 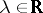 at which there is no subordinate solution.
at which there is no subordinate solution.
Recent developments (as of 2000) of the idea of subordinacy have led [a10] to further refinements of the analysis of singular spectra, in which the Hausdorff dimension of the spectral support plays a significant role.
The use of subordinacy and other techniques of spectral analysis have led to a deeper understanding of spectral properties for Sturm–Liouville operators in terms of the large- behaviour of solutions of the Sturm–Liouville equation (a1). Of course there still remains the problem of analyzing the large-
behaviour of solutions of the Sturm–Liouville equation (a1). Of course there still remains the problem of analyzing the large- asymptotics of solutions of (a1). However, advances in asymptotic analysis have led to the successful treatment of an ever widening class of Sturm–Liouville spectral problems. Examples of some of the most significant classes of function
asymptotics of solutions of (a1). However, advances in asymptotic analysis have led to the successful treatment of an ever widening class of Sturm–Liouville spectral problems. Examples of some of the most significant classes of function  that can be handled in this way are as follows.
that can be handled in this way are as follows.
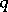 integrable plus function of bounded variation.
integrable plus function of bounded variation.
(For this case plus a more general treatment of asymptotics of solutions of systems of differential equations, see [a11].)
Suppose one can write 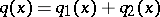 , where
, where 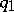 is continuous and of bounded variation, and
is continuous and of bounded variation, and 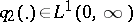 . Suppose also, for simplicity, that
. Suppose also, for simplicity, that 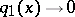 as
as 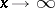 . Then, for
. Then, for 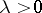 , the WKB method leads to solutions
, the WKB method leads to solutions 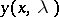 of (a1), having the asymptotic behaviour, as
of (a1), having the asymptotic behaviour, as 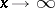 ,
,
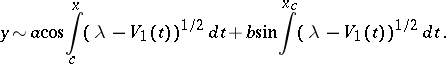 |
Asymptotics for  lead to exponential growth or decay of solutions. The spectrum is purely absolutely continuous for
lead to exponential growth or decay of solutions. The spectrum is purely absolutely continuous for 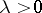 and purely discrete for
and purely discrete for 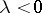 .
.
Example of eigenvalues embedded in continuous spectrum.
([a12]) In (a1), with 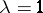 , let
, let
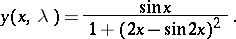 |
A simple calculation then shows that
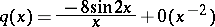 |
as 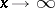 . This solution
. This solution 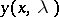 is an eigenfunction of the Dirichlet operator
is an eigenfunction of the Dirichlet operator 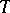 , with eigenvalue
, with eigenvalue 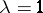 . One may verify that the interval
. One may verify that the interval 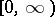 belongs to the absolutely continuous spectrum in this example.
belongs to the absolutely continuous spectrum in this example.
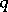 periodic.
periodic.
([a13]) Suppose that 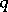 satisfies
satisfies 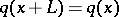 for some
for some 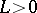 . Then the absolutely continuous spectrum consists of a sequence of disjoint intervals. The detailed location of these intervals is dependent on the particular function
. Then the absolutely continuous spectrum consists of a sequence of disjoint intervals. The detailed location of these intervals is dependent on the particular function 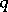 , though general results can be obtained regarding the asymptotic separation of the intervals for large
, though general results can be obtained regarding the asymptotic separation of the intervals for large 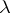 .
.
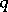 almost periodic or random.
almost periodic or random.
There is an extensive literature (see, for example, [a14]) on the spectral properties of 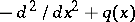 with
with  either almost periodic or
either almost periodic or 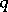 a random function. Such problems can give rise to a singular continuous spectrum, or to a pure point spectrum which is dense in an interval. As an example of the latter phenomenon, on each interval
a random function. Such problems can give rise to a singular continuous spectrum, or to a pure point spectrum which is dense in an interval. As an example of the latter phenomenon, on each interval 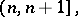 with
with 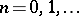 , set
, set 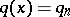 , where the
, where the 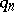 are constant and distributed independently for different
are constant and distributed independently for different  , with (say) uniform probability distribution over the interval
, with (say) uniform probability distribution over the interval 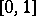 . Then, with probability
. Then, with probability 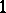 , the Sturm–Liouville operator
, the Sturm–Liouville operator 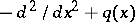 will have eigenvalues dense in the interval
will have eigenvalues dense in the interval 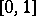 .
.
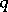 slowly oscillating.
slowly oscillating.
([a15]) A typical function of this type is given by 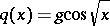 , where
, where 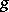 is a constant. The function
is a constant. The function 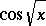 oscillates more and more slowly as
oscillates more and more slowly as  increases. One can show that, for almost all
increases. One can show that, for almost all 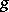 ,
, 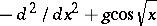 has eigenvalues dense in the interval
has eigenvalues dense in the interval 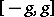 .
.
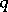 a sparse function.
a sparse function.
([a16]) A typical function of this type may be defined by  , where
, where 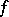 has compact support and the sequence
has compact support and the sequence 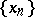 is strongly divergent as
is strongly divergent as  . Such a function
. Such a function 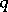 will give rise to a singular continuous spectrum provided
will give rise to a singular continuous spectrum provided 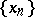 diverges sufficiently rapidly.
diverges sufficiently rapidly.
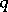 slowly decaying.
slowly decaying.
([a17]) A challenging problem in the spectral theory of Sturm–Liouville equations has been the analysis of the Dirichlet operator 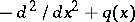 under the hypothesis that
under the hypothesis that 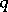 satisfy a bound for sufficiently large
satisfy a bound for sufficiently large  , of the form
, of the form 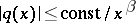 , for some
, for some 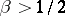 . If additional conditions are imposed, for example appropriate bounds on the derivative of
. If additional conditions are imposed, for example appropriate bounds on the derivative of 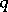 (assuming
(assuming 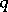 to be differentiable), then such functions
to be differentiable), then such functions  would fall under the category "integrable plus function of bounded variation" considered above, for which a spectral analysis can be carried out. However, in the absence of further conditions on
would fall under the category "integrable plus function of bounded variation" considered above, for which a spectral analysis can be carried out. However, in the absence of further conditions on 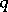 , it is already clear from the example of an eigenvalue in the continuous spectrum above that one cannot prove absolute continuity of the spectrum for
, it is already clear from the example of an eigenvalue in the continuous spectrum above that one cannot prove absolute continuity of the spectrum for 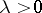 . In fact, for various
. In fact, for various 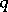 , a dense point spectrum or singular continuous spectrum may be present. A major advance in understanding this problem has been the proof [a17] that, under the hypothesis of
, a dense point spectrum or singular continuous spectrum may be present. A major advance in understanding this problem has been the proof [a17] that, under the hypothesis of  locally integrable and
locally integrable and 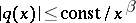 (
(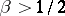 ), the entire semi-interval
), the entire semi-interval 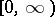 is contained in the absolutely continuous spectrum. Any subinterval
is contained in the absolutely continuous spectrum. Any subinterval  of
of 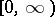 will satisfy
will satisfy 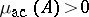 ; this does not exclude the possibility of a subset
; this does not exclude the possibility of a subset 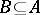 having Lebesgue measure zero with
having Lebesgue measure zero with 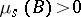 , and results have been obtained which further characterize the support of
, and results have been obtained which further characterize the support of 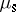 , for given
, for given 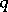 . Further extensions of some of these results to the more general case of
. Further extensions of some of these results to the more general case of  square integrable have been obtained (see [a19]).
square integrable have been obtained (see [a19]).
Numerical approaches.
(See, for example, [a18] and references contained therein.)
Sophisticated software capable of treating an increasingly wide class of spectral problems has been developed. These numerical approaches, often incorporating the use of interval analysis and leading to guaranteed error bounds for eigenvalues, have been used to investigate a variety of limit point and limit circle problems, and to estimate the  -function and spectral density function for a range of values of
-function and spectral density function for a range of values of 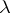 .
.
References
| [a1] | R.G. Newton, "Scattering theory of waves and particles" , Springer (1982) |
| [a2] | E. Prugovečki, "Quantum mechanics in Hilbert space" , Acad. Press (1981) |
| [a3] | E.C. Titchmarsh, "Eigenfunction expansions, Part 1" , Oxford Univ. Press (1962) |
| [a4] | E.A. Coddington, N. Levinson, "Theory of ordinary differential equations" , McGraw-Hill (1955) |
| [a5] | J. Chaudhury, W.N. Everitt, "On the spectrum of ordinary second order differential operators" Proc. Royal Soc. Edinburgh A , 68 (1968) pp. 95–115 |
| [a6] | M.S.P. Eastham, H. Kalf, "Schrödinger-type operators with continuous spectra" , Pitman (1982) |
| [a7] | N.I. Akhiezer, I.M. Glazman, "Theory of linear operators in Hilbert space" , Pitman (1981) |
| [a8] | D.J. Gilbert, D.B. Pearson, "On subordinacy and analysis of the spectrum of one-dimensional Schrödinger operators" J. Math. Anal. Appl. , 128 (1987) pp. 30–56 |
| [a9] | D.J. Gilbert, "On subordinacy and analysis of the spectrum of Schrödinger operators with two singular endpoints" Proc. Royal Soc. Edinburgh A , 112 (1989) pp. 213–229 |
| [a10] | S. Jitomirskaya, Y. Last, "Dimensional Hausdorff properties of singular continuous spectra" Phys. Rev. Lett. , 76 : 11 (1996) pp. 1765–1769 |
| [a11] | M.S.P. Eastham, "The asymptotic solution of linear differential systems" , Oxford Univ. Press (1989) |
| [a12] | M. Reed, B. Simon, "Methods of modern mathematical physics: Analysis of operators" , IV , Acad. Press (1978) |
| [a13] | M.S.P. Eastham, "The spectral theory of periodic differential operators" , Scottish Acad. Press (1973) |
| [a14] | L. Pastur, A. Figotin, "Spectra of random and almost periodic operators" , Springer (1991) |
| [a15] | G. Stolz, "Spectral theory for slowly oscillating potentials: Schrödinger operators" Math. Nachr. , 183 (1997) pp. 275–294 |
| [a16] | B. Simon, G. Stolz, "Operators with singular continuous spectrum: sparse potentials" Proc. Amer. Math. Soc. , 124 : 7 (1996) pp. 2073–2080 |
| [a17] | A. Kiselev, "Absolutely continuous spectrum of one-dimensional Schrödinger operators with slowly decreasing potentials" Comm. Math. Phys. , 179 (1996) pp. 377–400 |
| [a18] | "Spectral theory and computational methods of Sturm–Liouville problems" D. Hinton (ed.) P.W. Schaefer (ed.) , M. Dekker (1997) |
| [a19] | P. Deift, R. Killip, "On the absolutely continuous spectrum of one-dimensional Schrödinger operators with square-summable potentials" Comm. Math. Phys. , 203 (1999) pp. 341–347 |
Sturm-Liouville theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Sturm-Liouville_theory&oldid=18739