Semi-normal operator
A bounded linear operator  , acting on a Hilbert space, with the property that its self-commutator is trace-class, i.e.
, acting on a Hilbert space, with the property that its self-commutator is trace-class, i.e.  . A semi-normal operator can equivalently be defined by a pair
. A semi-normal operator can equivalently be defined by a pair  of self-adjoint operators (cf. Self-adjoint operator) with trace-class commutator (after writing
of self-adjoint operators (cf. Self-adjoint operator) with trace-class commutator (after writing  ). The theory of semi-normal operators is one of the few well-developed spectral theories for a class of non-self-adjoint operators. For the latter, see [a3].
). The theory of semi-normal operators is one of the few well-developed spectral theories for a class of non-self-adjoint operators. For the latter, see [a3].
Most examples of semi-normal operators are obtained via the Berger–Shaw inequality: If  is a hyponormal operator (i.e.
is a hyponormal operator (i.e.  ), of finite rational cyclicity
), of finite rational cyclicity  , then:
, then:
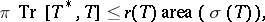 |
where  is the spectrum of
is the spectrum of 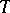 (cf. also Spectrum of an operator).
(cf. also Spectrum of an operator).
Here, the rational cyclicity 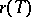 (also called the rational multiplicity) of an operator
(also called the rational multiplicity) of an operator  is defined as follows. Let
is defined as follows. Let  be the spectrum of
be the spectrum of  and let
and let 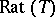 be the algebra of rational functions of a complex variable with poles outside
be the algebra of rational functions of a complex variable with poles outside  . Then
. Then  is the smallest cardinal number such that there is a set of vectors
is the smallest cardinal number such that there is a set of vectors  such that the closure of the span of
such that the closure of the span of
 |
is the whole space.
If 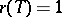 , then
, then 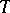 is said to be rationally cyclic. In particular, normal and rationally cyclic subnormal operators are semi-normal, [a2]. Certain singular integral operators with Cauchy-type kernel are also semi-normal, see [a3], [a4], [a6] and Singular integral.
is said to be rationally cyclic. In particular, normal and rationally cyclic subnormal operators are semi-normal, [a2]. Certain singular integral operators with Cauchy-type kernel are also semi-normal, see [a3], [a4], [a6] and Singular integral.
One of the most refined unitary invariants of a pure semi-normal operator 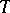 is the principal function
is the principal function  , which was introduced by J.D. Pincus [a6]. Let
, which was introduced by J.D. Pincus [a6]. Let  ,
,  be polynomials in two complex variables, and let
be polynomials in two complex variables, and let  be the Jacobian of the pair
be the Jacobian of the pair 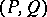 , written in complex coordinates. The Pincus–Helton–Howe trace formula [a4] characterizes
, written in complex coordinates. The Pincus–Helton–Howe trace formula [a4] characterizes 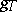 :
:
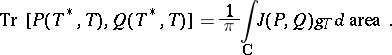 |
Via this formula one can prove the functoriality of the principal function under the holomorphic functional calculus. An observation due to D. Voiculescu shows that 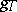 is invariant under Hilbert–Schmidt perturbations of
is invariant under Hilbert–Schmidt perturbations of  . The entire behaviour of the principal function qualifies it as the correct two-dimensional analogue of Krein's spectral shift function, well known in the perturbation theory of self-adjoint operators, see [a5]. The above trace formula can be interpreted as a generalized index theorem; this was one of the origins cyclic cohomology.
. The entire behaviour of the principal function qualifies it as the correct two-dimensional analogue of Krein's spectral shift function, well known in the perturbation theory of self-adjoint operators, see [a5]. The above trace formula can be interpreted as a generalized index theorem; this was one of the origins cyclic cohomology.
Thanks to a deep result of T. Kato and C.R. Putnam, a pure hyponormal operator  with trace-class self-commutator has absolutely continuous real and imaginary parts
with trace-class self-commutator has absolutely continuous real and imaginary parts  and
and  . Consequently, by diagonalizing
. Consequently, by diagonalizing  on a vector-valued
on a vector-valued  -space
-space 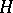 supported on the real line, the operator
supported on the real line, the operator  becomes:
becomes:
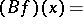 |
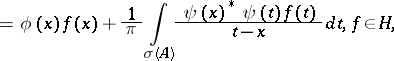 |
where 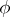 ,
,  are essentially bounded operator-valued functions. This singular integral model extends to all semi-normal operators; it was the source of most results in this area, by putting together methods of scattering theory (cf. also Scattering matrix) and singular integral equations, cf. [a1], [a7].
are essentially bounded operator-valued functions. This singular integral model extends to all semi-normal operators; it was the source of most results in this area, by putting together methods of scattering theory (cf. also Scattering matrix) and singular integral equations, cf. [a1], [a7].
Several invariant-subspace results are known for semi-normal operators. For instance, S.W. Brown has shown that hyponormal operators with thick spectrum (that is, dominant spectrum in an open subset of 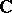 ) have non-trivial invariant subspaces. As an application of the theory of the principal function, C.A. Berger has proved that sufficiently high powers of hyponormal operators have invariant subspaces. For both results see [a5].
) have non-trivial invariant subspaces. As an application of the theory of the principal function, C.A. Berger has proved that sufficiently high powers of hyponormal operators have invariant subspaces. For both results see [a5].
One of the most studied sets of semi-normal operators is the class of operators 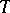 with rank-one self-commutator:
with rank-one self-commutator: 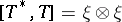 . For irreducible
. For irreducible  , the determinantal function [a6]:
, the determinantal function [a6]:
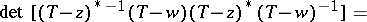 |
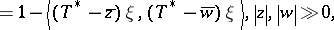 |
is a complete unitary invariant of  . This function can be expressed as the exponential of a double Cauchy transform of the principal function
. This function can be expressed as the exponential of a double Cauchy transform of the principal function  . A variety of applications of the above determinantal function to inverse problems of potential theory in the plane are known, [a1], [a5].
. A variety of applications of the above determinantal function to inverse problems of potential theory in the plane are known, [a1], [a5].
References
| [a1] | K. Clancey, "Seminormal operators" , Lecture Notes Math. , 742 , Springer (1979) |
| [a2] | J.B. Conway, "Theory of subnormal operators" , Math. Surveys Monogr. , 36 , Amer. Math. Soc. (1991) |
| [a3] | I. Gohberg, S. Goldberg, M.A. Kaashoeck, "Classes of linear operators I–II" , Birkhäuser (1990/3) |
| [a4] | J.W. Helton, R. Howe, "Traces of commutators of integral operators" Acta Math. , 135 (1975) pp. 271–305 |
| [a5] | M. Martin, M. Putinar, "Lectures on hyponormal operators" , Birkhäuser (1989) |
| [a6] | J.D. Pincus, "Commutators and systems of singular integral equations I" Acta Math. , 121 (1968) pp. 219–249 |
| [a7] | D. Xia, "Spectral theory of hyponormal operators" , Birkhäuser (1983) |
Semi-normal operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Semi-normal_operator&oldid=19046