Riemannian metric

The metric of a space given by a positive-definite quadratic form. If a local coordinate system is introduced for the space and if at each point functions , , , , are defined which are the components of a covariant symmetric tensor of the second valency, then this tensor is called the fundamental metric tensor of . The length of the covariant vector 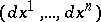is expressed using the fundamental tensor:

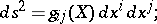the form is a positive-definite quadratic form. The metric of determined using the form is called Riemannian, and a space with a given Riemannian metric introduced into it is called a Riemannian space. The specification of a Riemannian metric on a differentiable manifold means the specification of a Euclidean structure on the tangent spaces of this manifold depending on the points in a differentiable way.

A Riemannian metric is a generalization of the first fundamental form of a surface in three-dimensional Euclidean space — of the internal metric of the surface. The geometry of the space based on a definite Riemannian metric is called a Riemannian geometry.

There are generalizations of the concept of a Riemannian metric. Thus, a pseudo-Riemannian metric is defined with the aid of a non-definite non-degenerate quadratic form (see Pseudo-Riemannian space and Relativity theory). A degenerate Riemannian metric, that is, a metric form defined with the aid of functions for which , defines a semi-Riemannian space.

References

[1]	L.P. Eisenhart, "Riemannian geometry" , Princeton Univ. Press (1949)
[2]	P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)
[3]	B. Riemann, "Ueber die Hypothesen, welche der Geometrie zuGrunde liegen" , Das Kontinuum und andere Monographien , Chelsea, reprint (1973)

Comments

The adjective "semi-Riemannian" is also used for indefinite metrics which are non-degenerate everywhere, cf. [a1]. For additional references see also Riemann tensor.

References

[a1]	B. O'Neill, "Elementary differential geometry" , Acad. Press (1966)