Regular set function
An additive function  defined on a family of sets in a topological space whose total variation
defined on a family of sets in a topological space whose total variation  (cf. Total variation of a function) satisfies the condition
(cf. Total variation of a function) satisfies the condition
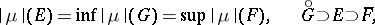 |
where  denotes the interior of a set
denotes the interior of a set 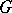 and
and  the closure of a set
the closure of a set  (and
(and  ,
,  ,
, 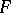 are in the domain of definition of
are in the domain of definition of 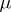 ). Every bounded additive regular set function, defined on a semi-ring of sets in a compact topological space, is countably additive (Aleksandrov's theorem).
). Every bounded additive regular set function, defined on a semi-ring of sets in a compact topological space, is countably additive (Aleksandrov's theorem).
The property of regularity can also be related to a measure, as a special case of a set function, and one speaks of a regular measure, defined on a topological space. For example, the Lebesgue measure is regular.
References
| [1] | N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Wiley (1988) |
| [2] | A.D. Aleksandrov, "Additive set-functions in abstract spaces" Mat. Sb. , 9 (1941) pp. 563–628 (In Russian) |
Comments
Although a set function is called regular if it satisfies a property of approximation from below or above involving "nice" sets, the precise meaning of "regular" usually depends on the context (and on the author). For example, a (Carathéodory) outer measure  is called regular if for every part
is called regular if for every part  of
of  one has
one has  , with
, with  a
a 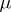 -measurable set containing
-measurable set containing  ; if
; if  is a topological space, the outer measure
is a topological space, the outer measure 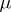 is called Borel regular if Borel sets are
is called Borel regular if Borel sets are  -measurable and if the
-measurable and if the  above can be taken Borel. On the other hand, if
above can be taken Borel. On the other hand, if  is a metrizable space and
is a metrizable space and  is a finite measure on the Borel
is a finite measure on the Borel  -field, then
-field, then  is always regular in the sense of the article above. In this setting
is always regular in the sense of the article above. In this setting  is often called inner regular, or just regular, if for any Borel subset
is often called inner regular, or just regular, if for any Borel subset  one has
one has  , with
, with  a countable union of compact sets included in
a countable union of compact sets included in  , that is, if
, that is, if  is a Radon measure. Instead of calling
is a Radon measure. Instead of calling  Radon, one nowadays most often says that it is tight.
Radon, one nowadays most often says that it is tight.
Regular set function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Regular_set_function&oldid=12357