Quantum probability

Quantum probability theory is a generalization of probability theory in which random variables are not assumed to commute. An alternative name is non-commutative probability theory. It developed in the 1970's from an urge to apply probabilistic concepts, such as "independence" , "noise" and "process" , to quantum mechanics. The mathematical ingredients of quantum probability theory derive from the theory of operator algebras, as founded by J. von Neumann and developed by M.A. Naimark, J. Dixmier, R.V. Kadison [a1], M. Tomita, M. Takesaki [a2], and others.

Classical probability.

The fundamental object of Kolmogorov's probability theory is a triple , where is the set of possible outcomes of some experiment, a -algebra of subsets of called "events" , and a probability measure on the measure space . A random variable is a measurable function on taking values in some measure space 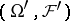, typically the real line with its Borel sets: . An event becomes an event when viewed as a statement "about" , namely . Thus, the random variable corresponds to an imbedding , .

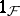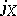One may now shift one's point of view somewhat, and replace the event by its characteristic function . This is a bounded measurable function . The set of bounded measurable functions is the von Neumann algebra . The probability measure extends to a positive linear functional on this algebra, the integral . If is the probability distribution of , then the imbedding mentioned above extends to the imbedding of the pair 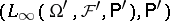into the pair , given by .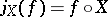

Then one may go one step further, and consider a function 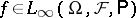as a linear operator on the Hilbert space by the multiplication , . The bounded linear operator comes from an event if and only if it is an orthogonal projection.

Generalization.

By a quantum probability space one means any pair 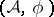, with 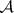a (possibly non-commutative) von Neumann algebra of operators on some Hilbert space and a weak-continuous positive linear functional (a state) on . The events of 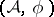are the orthogonal projections 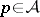. The probability that occurs is 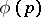. A quantum random variable is an imbedding of one quantum probability space into another. If is of the form , then stands for a random variable 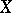taking values in . In particular, the projection stands for the event . 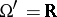In the case , the projection-valued measure determines a self-adjoint operator 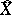(usually identified with the random variable 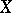) by

Therefore, real-valued quantum random variables correspond to self-adjoint operators affiliated with 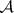, as postulated in quantum mechanics.

A quantum probability space carries a structure which is absent in the classical situation [a2]: If is faithful (i.e. if for , implies ), a one-parameter group 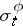, , of automorphisms of is canonically determined by the mismatch between the quadratic forms 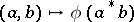and . This group, which is trivial in the commutative case, is called the modular group of 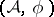and plays a central role in its probabilistic properties; for instance, it decides the existence question of conditional expectations. A random variable is said te be conditioned if there exists a completely positive mapping 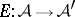such that and 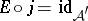. If this is the case, then is a projection of norm 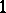(a conditional expectation) . 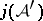Such a mapping exists if and only if is left globally invariant by the modular group of .

Quantum mechanics.

Quantum mechanics provides many examples of the above structure. For instance, the situation of (distinguishable) particles in space corresponds to the algebra consisting of all bounded operators on . The state 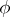is of the form , where 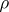ranges over all positive operators on 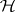with trace 1. Some interesting random variables are the positions and momenta of the particles, and their total energy.

Other examples can be found in quantum field theory and quantum statistical mechanics.

However, application of probabilistic ideas becomes most fruitful when the number of relevant degrees of freedom becomes infinite, and some central limit theorem is active. In particular, if a quantum system is studied which interacts weakly with a large number of objects in the outside world, this influence from the outside can be described by a quantum "noise" . Examples of such noises are: thermal radiation, thermal collisions with heat bath particles, laser fields and atomic beams. The evolution in time of the system under consideration is then described by a quantum stochastic process.

Quantum stochastic processes.

A quantum stochastic process [a3] is a family of quantum random variables indexed by time. (The time 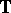may be , , , or .) If the process is conditioned, it determines a family of transition probabilities , i.e. completely positive mappings given by . A quantum Markov process is a quantum stochastic process in which the conditional expectation of the future, given past and present, is entirely determined by the present. In these processes one has an analogue of the classical Chapman–Kolmogorov equation: . The quantum stochastic process is called stationary if 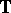is a group and their exists a group of automorphisms of such that . The process is said to be in thermal equilibrium if 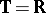and ().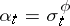

The theory of stationary quantum Markov processes was developed under the name of dilation theory [a4]. It is intimately connected with stochastic differential equations (cf. Langevin equation). A large part of the literature on quantum probability theory is concentrated in a series of proceedings volumes [a5].

References

[a1]	R.V. Kadison, J.R. Ringrose, "Fundamentals of the theory of operator algebras" , I-II , Acad. Press (1983–1986)
[a2]	M. Takesaki, "Tomita's theory of modular Hilbert algebras and its applications" , Lect. notes in math. , 128 , Springer (1970)
[a3]	L. Accardi, A. Frigerio, J.T. Lewis, "Quantum stochastic processes" Publ. RIMS Kyoto , 18 (1982) pp. 97–133
[a4]	B. Kümmerer, "Markov dilations on W*-algebras" J. Funct. Anal. , 63 (1985) pp. 139–177
[a5]	L. Accardi (ed.) W. von Waldenfels (ed.) , Quantum probability and applications I-IV , Lect. notes in math. , 1055, 1136, 1303, 1396 , Springer (1984, 1985, 1988, 1989)