Quadrangle
In elementary geometry a quadrangle is a figure consisting of four segments intersecting in four (corner) points.
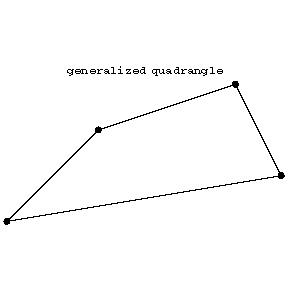
Figure: q076000a
Note that each point is incident with 2 lines, each line is incident with 2 points, that there is at most one line passing through two distinct points, that two lines intersect in at most one point, and that for a point and a line not incident with that point there is a unique line through that point intersecting the given line.
These properties exemplify the simplest case of a generalized quadrangle. This is an incidence system  , i.e. a (symmetric) incidence relation
, i.e. a (symmetric) incidence relation 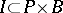 between points (the set
between points (the set 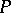 ) and lines (or blocks, the set
) and lines (or blocks, the set 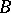 ) such that
) such that
i) for each point 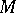 and line
and line 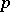 not passing through
not passing through 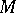 there is precisely one pair
there is precisely one pair 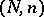 with
with 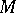 on
on  ,
, 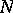 on
on  and
and 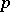 .
.
A generalized quadrangle can be seen as a very special kind of bipartite graph (cf. Graph, bipartite), obtained by taking as its vertex set the disjoint union 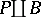 and with
and with 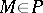 ,
, 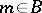 connected if and only if
connected if and only if  is on
is on 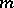 .
.
Interchanging 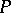 and
and 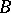 one obtains the dual generalized quadrangle.
one obtains the dual generalized quadrangle.
A generalized quadrangle is non-degenerate if there is no point that is collinear with all others, where two points are collinear if they are on a common line.
A finite generalized quadrangle of order 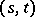 is one that satisfies i) above and also
is one that satisfies i) above and also
ii) each point is incident with precisely 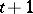 lines and there is at most one line through two distinct points;
lines and there is at most one line through two distinct points;
iii) each line has  points and two lines intersect in at most one point.
points and two lines intersect in at most one point.
A simple example of a finite generalized quadrangle of order 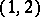 is depicted below
is depicted below
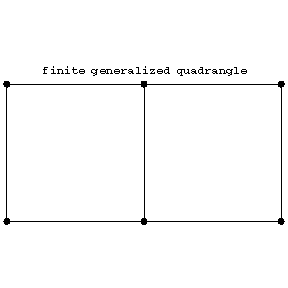
Figure: q076000b
This is also an example of a grid, which is an incidence structure 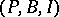 with
with 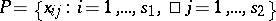 ,
, 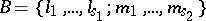 with
with 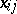 on
on 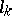 if and only if
if and only if 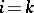 and
and 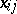 on
on 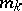 if and only if
if and only if 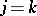 .
.
There are three known families of generalized quadrangles associated with the classical groups; these are known as classical generalized quadrangles. There are also others, for instance coming from ovoids (cf. Ovoid).
Generalized quadrangles were introduced by J. Tits [a1] and he also described the classical ones and the first non-classical ones. More generally one considers generalized  -gons, [a4].
-gons, [a4].
References
| [a1] | J. Tits, "Sur la trialité et certain groupes qui s'en déduisent" Publ. Math. IHES , 2 (1959) pp. 14–60 |
| [a2] | R. Dembowski, "Finite geometries" , Springer (1968) pp. 254 |
| [a3] | S.E. Payne, J.A. Thas, "Finite generalized quadrangles" , Pitman (1984) |
| [a4] | E.E. Shult, "Characterizations of the Lie incidence geometries" K. Lloyd (ed.) , Surveys in Combinatorics , Cambridge Univ. Press (1983) pp. 157–186 |
Quadrangle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quadrangle&oldid=13574