Positive vector bundle
A generalization of the concept of a divisor of positive degree on a Riemann surface. A holomorphic vector bundle 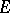 over a complex space
over a complex space  is called positive (denoted by
is called positive (denoted by  ) if on
) if on  there exists a Hermitian metric
there exists a Hermitian metric  such that the function
such that the function
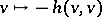 |
on  is strictly pseudo-convex outside the zero section. If
is strictly pseudo-convex outside the zero section. If  is a manifold, the condition for positivity is expressed in terms of the curvature of the metric
is a manifold, the condition for positivity is expressed in terms of the curvature of the metric  . That is, the curvature form of the metric
. That is, the curvature form of the metric  in the bundle
in the bundle  corresponds to a Hermitian quadratic form
corresponds to a Hermitian quadratic form  on
on 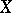 with values in the bundle
with values in the bundle 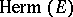 of Hermitian endomorphisms of the bundle
of Hermitian endomorphisms of the bundle  . The positivity condition is equivalent to
. The positivity condition is equivalent to 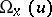 being a positive-definite operator on
being a positive-definite operator on  for any
for any 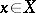 and any non-zero
and any non-zero  .
.
If 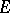 is a complex line bundle over a manifold
is a complex line bundle over a manifold 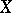 , the condition for positivity is equivalent to that of positive definiteness of the matrix
, the condition for positivity is equivalent to that of positive definiteness of the matrix
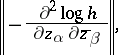 |
where  are local coordinates in
are local coordinates in  and
and  is a function that defines the Hermitian metric for the local trivialization of the bundle. If
is a function that defines the Hermitian metric for the local trivialization of the bundle. If  is compact, then the complex line bundle
is compact, then the complex line bundle  over
over 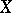 is positive if and only if the Chern class
is positive if and only if the Chern class 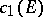 contains a closed form of the type
contains a closed form of the type
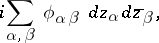 |
where  is a positive-definite Hermitian matrix. In particular, if
is a positive-definite Hermitian matrix. In particular, if 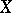 is a Riemann surface, then the bundle over
is a Riemann surface, then the bundle over 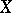 defined by a divisor of degree
defined by a divisor of degree  is positive if and only if
is positive if and only if 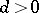 . If
. If  is a bundle of rank
is a bundle of rank 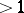 over a manifold
over a manifold  of dimension
of dimension  , one can consider also the following narrower class of positive bundles: A bundle
, one can consider also the following narrower class of positive bundles: A bundle 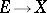 is called positive in the sense of Nakano if there exists on
is called positive in the sense of Nakano if there exists on 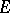 a Hermitian metric
a Hermitian metric  such that the Hermitian quadratic form
such that the Hermitian quadratic form  on the bundle
on the bundle  as defined by the formula
as defined by the formula
 |
where 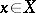 ,
, 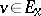 and
and 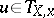 , is positive definite. Examples: the tangent bundle
, is positive definite. Examples: the tangent bundle  of the projective space
of the projective space 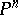 is positive, but for
is positive, but for 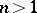 it is not positive in the sense of Nakano; the complex line bundle over
it is not positive in the sense of Nakano; the complex line bundle over 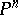 defined by a hyperplane is positive.
defined by a hyperplane is positive.
Any quotient bundle of a positive vector bundle is positive. If  and
and  are positive bundles (in the sense of Nakano), then
are positive bundles (in the sense of Nakano), then  and
and  are positive (in the sense of Nakano).
are positive (in the sense of Nakano).
The concept of a positive bundle was introduced in connection with Kodaira's vanishing theorem (cf. Kodaira theorem) for complex line bundles, and it was then extended to any bundle. Somewhat later, in relation to the existence of an imbedding in a projective space, the concepts of weakly-positive and weakly-negative bundles were introduced.
A holomorphic vector bundle 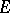 over a compact complex space
over a compact complex space 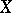 is called weakly negative if its zero section has a strictly pseudo-convex neighbourhood in
is called weakly negative if its zero section has a strictly pseudo-convex neighbourhood in 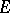 , i.e. if it is an exceptional analytic set. A bundle
, i.e. if it is an exceptional analytic set. A bundle  is called weakly positive if the dual bundle
is called weakly positive if the dual bundle 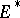 is weakly negative. If
is weakly negative. If  is a Riemann surface, the concepts of a weakly-positive bundle and a positive bundle coincide [5]. In the general case, positivity implies weak positivity; no examples are known at present (1983) of weakly positive but non-positive bundles.
is a Riemann surface, the concepts of a weakly-positive bundle and a positive bundle coincide [5]. In the general case, positivity implies weak positivity; no examples are known at present (1983) of weakly positive but non-positive bundles.
Weak positivity of a bundle  is equivalent to each of the following properties: For any coherent analytic sheaf
is equivalent to each of the following properties: For any coherent analytic sheaf  on
on 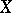 there exists an
there exists an 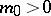 such that
such that 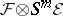 for
for  is generated by global sections; for any analytic sheaf
is generated by global sections; for any analytic sheaf 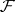 on
on  there exists an
there exists an  such that
such that
 |
for all 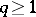 [3], [4]. By
[3], [4]. By 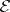 is meant the sheaf of germs of holomorphic sections of the bundle
is meant the sheaf of germs of holomorphic sections of the bundle  . Weakly-positive bundles are therefore analogous to the ample sheafs (cf. Ample sheaf) from algebraic geometry and are sometimes called ample analytic bundles. A weakly-positive bundle over a space
. Weakly-positive bundles are therefore analogous to the ample sheafs (cf. Ample sheaf) from algebraic geometry and are sometimes called ample analytic bundles. A weakly-positive bundle over a space  naturally defines an imbedding of
naturally defines an imbedding of 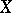 into a Grassmann manifold and thus into a projective space.
into a Grassmann manifold and thus into a projective space.
The concepts of a positive, a negative, a weakly-positive, and a weakly-negative bundle are naturally extended also to the case of a linear space over a complex space 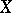 (see Vector bundle, analytic).
(see Vector bundle, analytic).
See also Negative vector bundle.
References
| [1] | R.O. Wells jr., "Differential analysis on complex manifolds" , Springer (1980) |
| [2] | S.S. Chern, "Complex manifolds without potential theory" , Springer (1979) |
| [3] | A.L. Onischik, "Pseudoconvexity in the theory of complex spaces" J. Soviet Math. , 14 : 4 (1980) pp. 1363–1406 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 15 (1977) pp. 93–171 |
| [4] | M. Schneider, "Familien negativer Vektorraumbündel und 1-konvexe Abbildungen" Abh. Math. Sem. Univ. Hamburg , 47 (1978) pp. 150–170 |
| [5] | H. Umemura, "Some results in the theory of vector bundles" Nagoya Math. J. , 52 (1973) pp. 97–128 |
Positive vector bundle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Positive_vector_bundle&oldid=12720