Osculating plane
From Encyclopedia of Mathematics
at a point 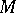 of a curve
of a curve 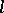
The plane having contact of order 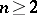 with
with 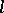 at
at 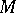 (see Osculation). The osculating plane can also be defined as the limit of a variable plane passing through three points of
(see Osculation). The osculating plane can also be defined as the limit of a variable plane passing through three points of  as these points approach
as these points approach 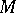 . Usually, a curve intersects the osculating plane at the point of contact (see Fig.).
. Usually, a curve intersects the osculating plane at the point of contact (see Fig.).
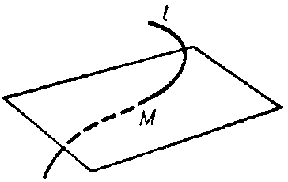
Figure: o070560a
If  is given by equations
is given by equations
 |
then the equation of the osculating plane has the form
 |
where 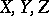 are moving coordinates and
are moving coordinates and 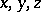 ,
, 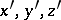 ,
, 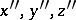 are calculated at the point of contact. If all three coefficients of
are calculated at the point of contact. If all three coefficients of 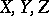 in the equation of the osculating plane vanish, then the osculating plane becomes indefinite (and can coincide with any plane through the tangent line).
in the equation of the osculating plane vanish, then the osculating plane becomes indefinite (and can coincide with any plane through the tangent line).
Comments
References
| [a1] | R.S. Millman, G.D. Parker, "Elements of differential geometry" , Prentice-Hall (1977) pp. 31–35 |
| [a2] | D.J. Struik, "Lectures on classical differential geometry" , Dover, reprint (1988) pp. 10ff |
How to Cite This Entry:
Osculating plane. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Osculating_plane&oldid=12865
Osculating plane. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Osculating_plane&oldid=12865
This article was adapted from an original article by BSE-3 (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article