Orlicz-Lorentz space
Lorentz–Orlicz space
There are several definitions of Orlicz–Lorentz spaces known in the literature ([a6], [a11], [a10], [a2]). All of them are generalizations of both Orlicz and Lorentz spaces (cf. Orlicz space; for Lorentz space, see Marcinkiewicz space). The Orlicz–Lorentz space presented below arises naturally as an intermediate space between ordinary Lorentz space and the space of bounded functions in the Calderón–Lozanovskii method of interpolation ([a4]; cf. also Interpolation of operators).
Given a Young function 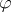 , i.e. a convex function (cf. also Convex function (of a real variable))
, i.e. a convex function (cf. also Convex function (of a real variable)) 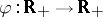 such that
such that 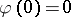 , the Orlicz–Lorentz space
, the Orlicz–Lorentz space 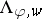 ([a6], [a11]) is the collection of all real-valued Lebesgue-measurable functions
([a6], [a11]) is the collection of all real-valued Lebesgue-measurable functions 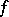 on
on  (cf. also Lebesgue integral) such that
(cf. also Lebesgue integral) such that
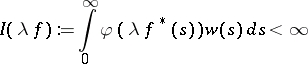 |
for some  , where the so-called weight function
, where the so-called weight function 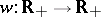 is positive, non-increasing and such that
is positive, non-increasing and such that
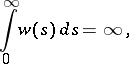 |
and for all 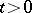 ,
,
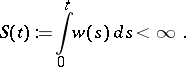 |
Here,  denotes the non-increasing rearrangement of
denotes the non-increasing rearrangement of 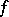 , that is,
, that is,
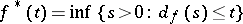 |
for  , where
, where 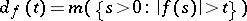 and
and 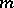 is the Lebesgue measure on
is the Lebesgue measure on  . The Orlicz–Lorentz space, equipped with the norm
. The Orlicz–Lorentz space, equipped with the norm
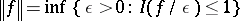 |
is a Banach function lattice (cf. also Banach lattice; Banach space) with ordering: 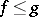 whenever
whenever 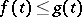 almost everywhere. If
almost everywhere. If 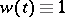 , then the Orlicz–Lorentz space becomes an Orlicz space, and if
, then the Orlicz–Lorentz space becomes an Orlicz space, and if 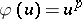 ,
,  , then it becomes a Lorentz space [a9].
, then it becomes a Lorentz space [a9].
Many properties of 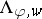 have been described in terms of growth conditions imposed on
have been described in terms of growth conditions imposed on 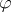 and
and  . The most common growth conditions are regularity of the weight and condition
. The most common growth conditions are regularity of the weight and condition 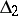 of a Young function. It is said that
of a Young function. It is said that  is regular if
is regular if 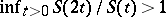 and a Young function
and a Young function 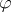 satisfies condition
satisfies condition 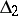 whenever
whenever 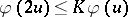 for all
for all  and some
and some 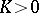 . The methods applied in the theory of Orlicz–Lorentz spaces are derived from those for both Orlicz and Lorentz spaces.
. The methods applied in the theory of Orlicz–Lorentz spaces are derived from those for both Orlicz and Lorentz spaces.
Some results on isomorphic or isometric properties of 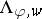 are as follows.
are as follows.
1) Condition 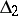 on
on  is equivalent to the following properties of
is equivalent to the following properties of  [a6].
[a6].
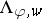 does not contain an isometric copy of
does not contain an isometric copy of 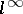 .
.
 does not contain an isomorphic copy of
does not contain an isomorphic copy of 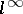 .
.
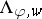 does not contain an isomorphic copy of
does not contain an isomorphic copy of  .
.
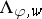 is separable (cf. also Separable space).
is separable (cf. also Separable space).
The norm in 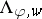 is absolutely continuous (cf. also Absolute continuity).
is absolutely continuous (cf. also Absolute continuity).
2) The function 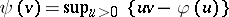 on
on 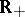 is called the Young conjugate to
is called the Young conjugate to 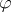 (cf. also Conjugate function). The functions
(cf. also Conjugate function). The functions 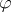 and
and 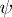 are conjugate to each other and this duality is analogous to the duality between power functions
are conjugate to each other and this duality is analogous to the duality between power functions 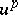 and
and 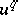 with
with 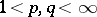 and
and 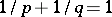 . The dual space
. The dual space  can be described in terms of
can be described in terms of 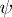 . In fact, if both
. In fact, if both 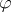 and
and 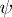 satisfy condition
satisfy condition  and
and 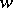 is regular, then
is regular, then 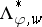 is the family of all Lebesgue-measurable functions
is the family of all Lebesgue-measurable functions 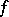 such that
such that
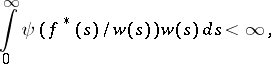 |
and the dual norm is equivalent to the quasi-norm  . The space
. The space 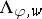 is reflexive (cf. also Reflexive space) if and only if both
is reflexive (cf. also Reflexive space) if and only if both  and
and 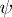 satisfy condition
satisfy condition 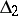 . Superreflexivity (cf. also Reflexive space) requires, in addition, the assumption of regularity of
. Superreflexivity (cf. also Reflexive space) requires, in addition, the assumption of regularity of  [a4].
[a4].
3) A number of geometric properties, like uniform convexity, rotundity, extreme points, local uniform convexity, and normal and uniform normal structure in Orlicz–Lorentz spaces have been characterized in terms of 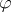 and
and 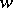 ([a3], [a4], [a6], [a7], [a8], [a5]). For instance, necessary and sufficient conditions for
([a3], [a4], [a6], [a7], [a8], [a5]). For instance, necessary and sufficient conditions for 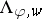 to be uniformly convex are that both
to be uniformly convex are that both 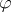 and
and 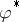 satisfy
satisfy 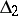 , that
, that 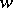 is regular and that
is regular and that 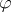 is uniformly convex, i.e.
is uniformly convex, i.e.  for every
for every 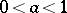 , where
, where 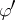 is the right derivative of
is the right derivative of 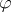 (cf. also Differentiation).
(cf. also Differentiation).
Order convexity and concavity as well as Boyd indices in Orlicz–Lorentz spaces have been studied in, e.g., [a10], [a1].
In the definition of Orlicz–Lorentz space one can replace Lebesgue-measurable functions by measurable functions with respect to a 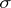 -finite measure space. All results stated above remain the same in the case of a non-atomic infinite measure. For other measure spaces, different versions of condition
-finite measure space. All results stated above remain the same in the case of a non-atomic infinite measure. For other measure spaces, different versions of condition 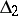 are applied ([a3], [a6], [a8]).
are applied ([a3], [a6], [a8]).
References
| [a1] | Y. Raynaud, "On Lorentz–Sharpley spaces" , Proc. Workshop on Interpolation Spaces and Related Topics , Israel Math. Conf. Proc. , 5 (1992) pp. 207–228 |
| [a2] | A. Torchinsky, "Interplation of operators and Orlicz classes" Studia Math. , 59 (1976) pp. 177–207 |
| [a3] | J. Cerda, H. Hudzik, A. Kamińska, M. Mastyło, "Geometric properties of symmetric spaces with applications to Orlicz–Lorentz spaces" Positivity , 2 (1998) pp. 311–337 |
| [a4] | H. Hudzik, A. Kamińska, M. Mastyło, "Geometric properties of some Calderón–Lozanovskii and Orlicz–Lorentz spaces" Houston J. Math. , 22 (1996) pp. 639–663 |
| [a5] | A. Kamińska, P.K. Lin, H. Sun, "Uniformly normal structure of Orlicz–Lorentz spaces" , Interaction Between Functional Analysis, Harmonic Analysis, and Probability (Columbia, Missouri, 1994) , Lecture Notes Pure Appl. Math. , 175 , M. Dekker (1996) pp. 229–238 |
| [a6] | A. Kamińska, "Some remarks on Orlicz–Lorentz spaces" Math. Nachr. , 147 (1990) pp. 29–38 |
| [a7] | A. Kamińska, "Extreme points in Orlicz–Lorentz spaces" Arch. Math. , 55 (1990) pp. 173–180 |
| [a8] | A. Kamińska, "Uniform convexity of generalized Lorentz spaces" Arch. Math. , 56 (1991) pp. 181–188 |
| [a9] | J. Lindenstrauss, L. Tzafriri, "Classical Banach spaces I–II" , Springer (1977–1979) |
| [a10] | St. Montgomery-Smith, "Boyd indices of Orlicz–Lorentz spaces" , Function Spaces (Edwardsville, IL, 1994) , Lecture Notes Pure Appl. Math. , 172 , M. Dekker (1995) pp. 321–334 |
| [a11] | L. Maligranda, "Indices and interpolation" Dissert. Math. , 234 (1985) |
Orlicz-Lorentz space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Orlicz-Lorentz_space&oldid=16855