Multi-valued mapping
point-to-set mapping
A mapping 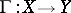 associating with each element
associating with each element  of a set
of a set 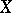 a subset
a subset 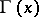 of a set
of a set 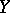 . If for each
. If for each 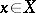 the set
the set 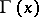 consists of one element, then the mapping
consists of one element, then the mapping 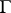 is called single-valued. A multi-valued mapping
is called single-valued. A multi-valued mapping 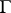 can be treated as a single-valued mapping of
can be treated as a single-valued mapping of 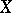 into
into 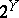 , that is, into the set of all subsets of
, that is, into the set of all subsets of 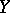 .
.
For two multi-valued mappings 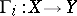 ,
, 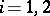 , their inclusion is naturally defined:
, their inclusion is naturally defined:  if
if 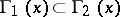 for all
for all 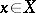 . For any family of multi-valued mappings
. For any family of multi-valued mappings  ,
, 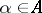 , the union and intersection are defined:
, the union and intersection are defined:  if
if 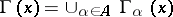 for all
for all 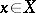 and
and 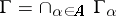 if
if 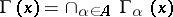 for all
for all  . For any family of multi-valued mappings
. For any family of multi-valued mappings 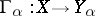 ,
, 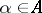 , the multi-valued mapping
, the multi-valued mapping 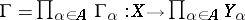 is called the Cartesian product of the multi-valued mappings
is called the Cartesian product of the multi-valued mappings 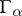 if
if 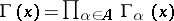 . A section of a multi-valued mapping
. A section of a multi-valued mapping 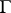 is a single-valued mapping
is a single-valued mapping 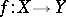 such that
such that 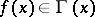 for all
for all 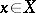 . The graph of a multi-valued mapping
. The graph of a multi-valued mapping 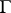 is the set
is the set 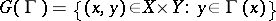 .
.
A multi-valued mapping 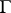 of a topological space
of a topological space 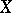 into a topological space
into a topological space 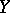 is called upper semi-continuous if for every open set
is called upper semi-continuous if for every open set 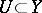 the set
the set 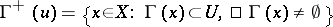 is open in
is open in  , or equivalently: For any
, or equivalently: For any 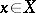 and any neighbourhood
and any neighbourhood 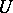 of
of 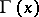 there is a neighbourhood
there is a neighbourhood 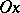 of
of  such that
such that 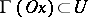 , where
, where  . A multi-valued mapping from a topological space
. A multi-valued mapping from a topological space  to a topological space
to a topological space 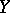 is called lower semi-continuous if for any open set
is called lower semi-continuous if for any open set 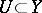 the set
the set  is open in
is open in  . If a multi-valued mapping satisfies both properties simultaneously, then it is called a continuous multi-valued mapping.
. If a multi-valued mapping satisfies both properties simultaneously, then it is called a continuous multi-valued mapping.
Let 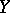 be a topological vector space. A multi-valued mapping
be a topological vector space. A multi-valued mapping 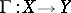 is called convex-compact valued if
is called convex-compact valued if  is a convex compact set for all
is a convex compact set for all 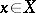 . For a finite set of multi-valued mappings
. For a finite set of multi-valued mappings 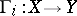 ,
, 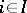 , the algebraic sum
, the algebraic sum  is defined by
is defined by 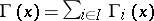 . The intersection of any (finite) family of upper semi-continuous (respectively, continuous) multi-valued mappings is upper semi-continuous (respectively, continuous). The Cartesian product of a finite family of upper semi-continuous multi-valued mappings is upper semi-continuous. The algebraic sum of a finite family of upper semi-continuous (convex-compact valued) mappings is upper semi-continuous (convex-compact valued). The intersection and Cartesian product of any family of convex-compact valued mappings is convex-compact valued.
. The intersection of any (finite) family of upper semi-continuous (respectively, continuous) multi-valued mappings is upper semi-continuous (respectively, continuous). The Cartesian product of a finite family of upper semi-continuous multi-valued mappings is upper semi-continuous. The algebraic sum of a finite family of upper semi-continuous (convex-compact valued) mappings is upper semi-continuous (convex-compact valued). The intersection and Cartesian product of any family of convex-compact valued mappings is convex-compact valued.
Let  be a paracompact space and
be a paracompact space and 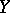 a locally convex metric linear space (cf. Locally convex space; Linear space; Metric space). Let
a locally convex metric linear space (cf. Locally convex space; Linear space; Metric space). Let 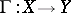 be a multi-valued mapping which is upper semi-continuous and is such that
be a multi-valued mapping which is upper semi-continuous and is such that 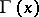 is closed in
is closed in 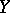 for every
for every  . Then the multi-valued mapping
. Then the multi-valued mapping 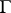 admits continuous sections. Let
admits continuous sections. Let  and
and 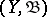 be spaces with given
be spaces with given  -algebras
-algebras  and
and  ; a multi-valued mapping
; a multi-valued mapping 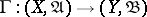 is called measurable if the graph
is called measurable if the graph  belongs to the smallest
belongs to the smallest 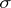 -algebra
-algebra  of
of 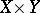 containing all sets of the form
containing all sets of the form  , where
, where 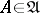 and
and 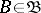 . If
. If 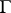 is a measurable multi-valued mapping from
is a measurable multi-valued mapping from 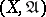 to a complete separable metric space
to a complete separable metric space 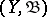 , where
, where 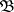 is the Borel
is the Borel  -algebra of
-algebra of 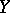 , then
, then 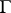 has measurable sections
has measurable sections 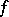 .
.
References
| [1] | K. Kuratowski, "Topology" , 1–2 , Acad. Press (1966–1968) (Translated from French) |
Comments
A multi-valued mapping is also called a set-valued or many-valued mapping. Sections are also called selections.
Theorems which prove that certain kinds of multi-valued mappings admit selections are called selection theorems. The measurable selection theorem stated in the last sentence of the main article above is known as von Neumann's measurable choice theorem. A number of selection theorems and some applications are discussed in [a4].
References
| [a1] | E. Michael, "Continuous selections" Ann. of Math. , 63 (1956) pp. 361–382 |
| [a2] | E.A. Michael, "A survey of continuous selections" W.M. Fleischmann (ed.) , Set valued mappings, selections and topological properties of  (Proc. Conf. SUNY Buffalo, 1969) , Lect. notes in math. , 171 , Springer (1970) pp. 54–58 (Proc. Conf. SUNY Buffalo, 1969) , Lect. notes in math. , 171 , Springer (1970) pp. 54–58 |
| [a3] | K. Przeslawski, D. Yost, "Continuity properties of selectors and Michael's Theorem" Mich. Math. J. , 36 (1989) pp. 113–134 |
| [a4] | T. Parthasarathy, "Selection theorems and their applications" , Lect. notes in math. , 263 , Springer (1972) |
Multi-valued mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Multi-valued_mapping&oldid=15886