Moonshine conjectures
In 1978, J. McKay observed that 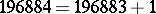 . The number on the left is the first non-trivial coefficient of the
. The number on the left is the first non-trivial coefficient of the  -function, and the numbers on the right are the dimensions of the smallest irreducible representations of the Fischer–Griess Monster
-function, and the numbers on the right are the dimensions of the smallest irreducible representations of the Fischer–Griess Monster  [a10] (cf. also Sporadic simple group). On the one side stands a modular function; on the other, a finite sporadic simple group. Moonshine is the explanation and generalization of this unlikely connection.
[a10] (cf. also Sporadic simple group). On the one side stands a modular function; on the other, a finite sporadic simple group. Moonshine is the explanation and generalization of this unlikely connection.
Monstrous moonshine [a6] conjectured that there is an infinite-dimensional graded vector space  , with the following properties. Each
, with the following properties. Each  carries a finite-dimensional representation of
carries a finite-dimensional representation of  ; write
; write  for its character. For each
for its character. For each 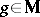 , define the Thompson–McKay series
, define the Thompson–McKay series  , where
, where  . Then
. Then  is a generator ( "Hauptmodul" ) of the field of modular functions for some genus-
is a generator ( "Hauptmodul" ) of the field of modular functions for some genus- group
group  . The group
. The group  contains
contains  as a normal subgroup, where
as a normal subgroup, where 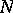 divides
divides  (
( is the order of
is the order of 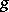 ).
).
There are  distinct Thompson series (
distinct Thompson series ( has only
has only  conjugacy classes). For example,
conjugacy classes). For example, 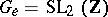 and
and 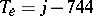 , where
, where  is the identity.
is the identity. 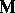 has two order-
has two order- conjugacy classes, corresponding to the modular groups
conjugacy classes, corresponding to the modular groups 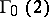 and
and
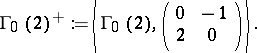 |
Let  denote the
denote the  -dimensional irreducible representation of
-dimensional irreducible representation of 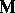 ; then
; then  ,
, 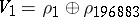 , and
, and 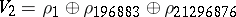 .
.
Central to these conjectures is the moonshine module 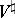 , constructed in [a9]. It is an important example of a vertex operator algebra (VOA) [a2], [a9], and as such possesses infinitely many heavily constrained bilinear products. One of these products makes
, constructed in [a9]. It is an important example of a vertex operator algebra (VOA) [a2], [a9], and as such possesses infinitely many heavily constrained bilinear products. One of these products makes  into the Griess algebra [a10] extended by an identity element. The automorphism group of
into the Griess algebra [a10] extended by an identity element. The automorphism group of  is
is  .
.
The monstrous moonshine conjectures, and, in particular, the identification of  with
with  , were proved in [a4], which also defined vertex operator algebras and a generalization of Kac–Moody algebras called Borcherds or generalized Kac–Moody algebras [a3] (cf. also Kac–Moody algebra; Borcherds Lie algebra).
, were proved in [a4], which also defined vertex operator algebras and a generalization of Kac–Moody algebras called Borcherds or generalized Kac–Moody algebras [a3] (cf. also Kac–Moody algebra; Borcherds Lie algebra).
The series  were generalized by S.P. Norton to commuting pairs
were generalized by S.P. Norton to commuting pairs 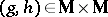 [a12]. In particular, to each such pair there is a modular function
[a12]. In particular, to each such pair there is a modular function  , invariant under a genus-
, invariant under a genus- group, such that
group, such that
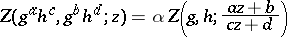 |
for some root of unity 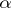 , for any
, for any
 |
This action of  is related to its natural action on the fundamental group
is related to its natural action on the fundamental group  of the torus. The coefficients of the
of the torus. The coefficients of the  -expansion of
-expansion of  are characters of the centralizer
are characters of the centralizer 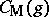 evaluated at
evaluated at  . Simultaneous conjugation of
. Simultaneous conjugation of  ,
,  leaves
leaves 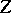 unchanged:
unchanged:  . The Thompson–McKay series are recovered by the specialization
. The Thompson–McKay series are recovered by the specialization  :
:  . Only special cases of these generalized moonshine conjectures have been proven.
. Only special cases of these generalized moonshine conjectures have been proven.
There are several other conjectures. For example, the series 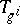 were conjecturally related by the replication formulas [a6], [a1]:
were conjecturally related by the replication formulas [a6], [a1]:
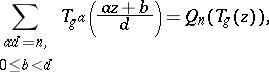 | (a1) |
where  is the unique polynomial for which
is the unique polynomial for which  is a power series with only strictly positive powers of
is a power series with only strictly positive powers of 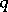 . The resemblance of (a1) with Hecke operators is not accidental. These formulas are related to identities such as
. The resemblance of (a1) with Hecke operators is not accidental. These formulas are related to identities such as
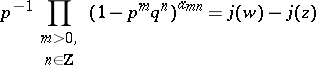 | (a2) |
for  , where
, where  , as well as to the existence of several modular equations obeyed by the
, as well as to the existence of several modular equations obeyed by the  . These identities played a large role in the proof of moonshine. R. Borcherds proved that the
. These identities played a large role in the proof of moonshine. R. Borcherds proved that the  obey (a1) by using the denominator identities of Borcherds algebras; the resulting modular equations can be used to prove the Hauptmodul property [a7]. It would be very nice to see other direct connections between the group operation in
obey (a1) by using the denominator identities of Borcherds algebras; the resulting modular equations can be used to prove the Hauptmodul property [a7]. It would be very nice to see other direct connections between the group operation in 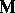 and the series
and the series  : for instance, are there other relations among the
: for instance, are there other relations among the  which correspond to more general products of conjugacy classes in
which correspond to more general products of conjugacy classes in 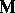 ?
?
The Hirzebruch prize question [a11] asks for a compact, differentiable,  -dimensional manifold on which
-dimensional manifold on which  acts by diffeomorphisms and whose twisted Witten genus is
acts by diffeomorphisms and whose twisted Witten genus is  . It is still open (as of 2000).
. It is still open (as of 2000).
Vertex operator algebras are a key component in moonshine. There will be some form of moonshine for finite subgroups of the automorphism group of any rational vertex operator algebra obeying a technical (and probably redundant) "C2 condition" (see e.g. [a18]). The resulting functions, however, will not be Hauptmoduls in general. There is no complete understanding yet (2000) of why Hauptmoduls are associated to  , although it has been conjectured to do with the "6-transposition property" of
, although it has been conjectured to do with the "6-transposition property" of  [a12], or the uniqueness of
[a12], or the uniqueness of  [a17].
[a17].
Moonshine is related to conformal field theory (e.g.  is the chiral algebra of a holomorphic
is the chiral algebra of a holomorphic 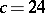 theory), in which context many of moonshine's special features appear natural [a8], [a17], and this has motivated some of the developments (most significantly the definition of vertex operator algebras).
theory), in which context many of moonshine's special features appear natural [a8], [a17], and this has motivated some of the developments (most significantly the definition of vertex operator algebras).
It still (as of 2000) is unclear why certain Hauptmoduls appear in moonshine and others do not. What form does moonshine (e.g. the replication formulas) take in genus  (see e.g. [a16])? How about moonshine for other groups (see e.g. [a13])? In [a14], a variant called modular moonshine was established, where
(see e.g. [a16])? How about moonshine for other groups (see e.g. [a13])? In [a14], a variant called modular moonshine was established, where  is replaced with vertex algebras over characteristic
is replaced with vertex algebras over characteristic 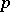 , and where the role of
, and where the role of 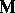 is taken by centralizers in
is taken by centralizers in  of order
of order  elements.
elements.
For his work in monstrous moonshine and related topics, Borcherds was awarded a Fields Medal in 1998 [a5].
References
| [a1] | D. Alexander, C. Cummins, J. McKay, C. Simons, "Completely replicable functions" , Groups, Combinatorics and Geometry , Cambridge Univ. Press (1992) pp. 87–98 |
| [a2] | R.E. Borcherds, "Vertex algebras, Kac–Moody algebras, and the Monster" Proc. Nat. Acad. Sci. USA , 83 (1986) pp. 3068–3071 |
| [a3] | R.E. Borcherds, "Generalized Kac–Moody algebras" J. Algebra , 115 (1988) pp. 501–512 |
| [a4] | R.E. Borcherds, "Monstrous moonshine and monstrous Lie superalgebras" Invent. Math. , 109 (1992) pp. 405–444 |
| [a5] | R.E. Borcherds, "What is Moonshine?" , Proc. ICM, Berlin , DMV (1998) pp. 607–615 |
| [a6] | J.H. Conway, S.P. Norton, "Monstrous moonshine" Bull. London Math. Soc. , 11 (1979) pp. 308–339 |
| [a7] | C. Cummins, T. Gannon, "Modular equations and the genus  property of Moonshine functions" Invent. Math. , 129 (1997) pp. 413–443 property of Moonshine functions" Invent. Math. , 129 (1997) pp. 413–443 |
| [a8] | L. Dixon, P. Ginsparg, J. Harvey, "Beauty and the Beast: superconformal symmetry in a Monster module" Commun. Math. Phys. , 119 (1988) pp. 221–241 |
| [a9] | I.B. Frenkel, J. Lepowsky, A. Meurman, "Vertex operators and the monster" , Acad. Press (1988) |
| [a10] | R.L. Griess, "The friendly giant" Invent. Math. , 69 (1982) pp. 1–102 |
| [a11] | F. Hirzebruch, T. Berger, R. Jung, "Manifolds and modular forms" , Aspects of Math. , Vieweg (1994) (Edition: Second) |
| [a12] | S.P. Norton, "Generalized moonshine" Proc. Symp. Pure Math. , 47 (1987) pp. 208–209 |
| [a13] | L. Queen, "Modular functions arising from some finite groups" Math. Comput. , 37 (1981) pp. 547–580 |
| [a14] | A.J. E. Ryba, "Modular moonshine?" , Moonshine, the Monster, and Related Topics , Contemp. Math. , 193 , Amer. Math. Soc. (1996) pp. 307–336 |
| [a15] | R.E. Borcherds, "Modular moonshine III" Duke Math. J. , 93 (1998) pp. 129–154 |
| [a16] | G.W. Smith, "Replicant powers for higher genera" , Moonshine, the Monster, and Related Topics , Contemp. Math. , 193 , Amer. Math. Soc. (1996) pp. 337–352 |
| [a17] | M.P. Tuite, "On the relationship between Monstrous moonshine and the uniqueness of the Moonshine module" Commun. Math. Phys. , 166 (1995) pp. 495–532 |
| [a18] | Y. Zhu, "Modular invariance of characters of vertex operator algebras" J. Amer. Math. Soc. , 9 (1996) pp. 237–302 |
| [a19] | C. Dong, H. Li, G. Mason, "Modular invariance of trace functions in orbifold theory" preprint q-alg/9703016 (1997) |
Moonshine conjectures. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Moonshine_conjectures&oldid=18566