Luzin theorem
Luzin's theorem in the theory of functions of a complex variable (the local principle of finite area) is a result of N.N. Luzin that reveals a connection between the boundary properties of an analytic function in the unit disc and the metric of the Riemann surface onto which it maps the disc (see [1], [2]).
Let 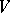 be any domain inside the unit disc
be any domain inside the unit disc  of the complex
of the complex  -plane adjoining an arc
-plane adjoining an arc 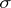 of the unit circle
of the unit circle 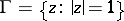 , and let
, and let
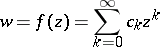 |
be a regular analytic function in 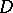 . If the area of the Riemann surface that is the image of
. If the area of the Riemann surface that is the image of 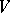 under the mapping
under the mapping 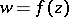 is finite, then the series
is finite, then the series
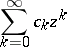 |
converges almost-everywhere on  .
.
In connection with this theorem Luzin made a conjecture, also known as Luzin's problem. A point 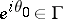 is called a Luzin point of the function
is called a Luzin point of the function 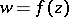 if
if 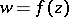 maps every disc touching
maps every disc touching 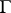 from the inside at
from the inside at  onto a domain of infinite area on the Riemann surface of
onto a domain of infinite area on the Riemann surface of  . The Luzin conjecture is that there are bounded analytic functions in
. The Luzin conjecture is that there are bounded analytic functions in 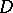 such that every point of
such that every point of 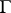 is a Luzin point for them. The Luzin conjecture was first confirmed completely in 1955 (see [3]).
is a Luzin point for them. The Luzin conjecture was first confirmed completely in 1955 (see [3]).
References
| [1] | N.N. Luzin, "On localization of the finite area principle" Dokl. Akad. Nauk SSSR , 56 (1947) pp. 447–450 (In Russian) |
| [2] | N.N. Luzin, "Collected works" , 1 , Moscow (1953) pp. 318–330 (In Russian) |
| [3] | A. Lohwater, "The boundary behaviour of analytic functions" Itogi Nauki i Tekhn. Mat. Anal. , 10 (1973) pp. 99–259 (In Russian) |
Comments
The reference for the solution of Luzin's problem is [a1].
References
| [a1] | A.J. Lohwater, G. Piranian, "On a conjecture of Luzin" Michigan Math. J. , 3 (1955) pp. 63–68 |
Luzin's theorems in descriptive set theory are, by convention, split into three parts. The first and main part is directed towards the study of effective sets (analytic, Borel, Luzin (projective) sets). Here one is concerned with the Luzin separability principles and the theorem on the existence of Luzin sets of arbitrary class (cf. Luzin set). The second part is the study of problems lying on the path to the solution of the continuum hypothesis and the problem of the cardinality of  -sets (cf.
-sets (cf. 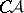 -set). Here one distinguishes the Luzin–Sierpiński theorem on partitioning an interval into
-set). Here one distinguishes the Luzin–Sierpiński theorem on partitioning an interval into  Borel sets, determined by the corresponding Luzin sieve, and also Luzin's covering theorem: Let
Borel sets, determined by the corresponding Luzin sieve, and also Luzin's covering theorem: Let 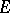 and
and 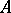 be disjoint analytic sets (cf.
be disjoint analytic sets (cf. 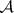 -set; Analytic set) and let
-set; Analytic set) and let
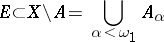 |
be a decomposition of 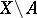 into constituents; then there is an index
into constituents; then there is an index  such that
such that
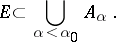 |
The third part contains results obtained by the use of the axiom of choice. Here one borders on philosophical work in set theory. One distinguishes Luzin's theorem on the existence of an uncountable set of the first category (cf. Category of a set) in any perfect set, and on partitioning an interval into an uncountable number of non-measurable sets. To complete this part there is Luzin's theorem on subsets of the set of natural numbers, which reflects some properties of the remainder 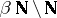 of the Stone–Čech compactification
of the Stone–Čech compactification 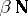 of the natural number series
of the natural number series  .
.
References
| [1] | N.N. Luzin, "Collected works" , 2 , Moscow (1958) (In Russian) |
B.A. Efimov
Comments
See Luzin sieve for the definition of constituents.
"Luzin's theorem on subsets of the set of natural numbers" states that there is a family  of infinite subsets of
of infinite subsets of 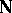 such that
such that 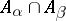 is finite for
is finite for 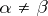 and such that for any two uncountable disjoint subsets
and such that for any two uncountable disjoint subsets  and
and  of
of 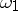 there is no subset
there is no subset  of
of 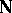 such that for all
such that for all 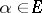 :
: 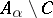 is finite and for all
is finite and for all 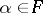 :
: 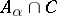 is finite. A family like this usually called a Luzin family. See [a2].
is finite. A family like this usually called a Luzin family. See [a2].
In the West, the name "Luzin theorem" refers almost always to a result in measure theory; see Luzin criterion. It may also refer to the following result of Luzin in descriptive set theory: If 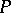 is a Polish space,
is a Polish space, 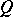 a separable metrizable space and
a separable metrizable space and 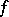 is an injective Borel mapping from
is an injective Borel mapping from 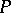 into
into 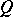 , then the direct image
, then the direct image  of any Borel subset
of any Borel subset 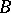 of
of  is a Borel subset of
is a Borel subset of 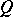 . Luzin's covering theorem in descriptive set theory is usually called the (classical) boundness theorem in the West; it gave rise, together with the Luzin–Sierpiński theorem, Luzin sieves, etc., to the modern use of countable ordinals in this theory. See also Descriptive set theory.
. Luzin's covering theorem in descriptive set theory is usually called the (classical) boundness theorem in the West; it gave rise, together with the Luzin–Sierpiński theorem, Luzin sieves, etc., to the modern use of countable ordinals in this theory. See also Descriptive set theory.
References
| [a1] | K. Kuratowski, "Topology" , 1 , PWN & Acad. Press (1966) (Translated from French) |
| [a2] | E.K. van Douwen, "The integers and topology" K. Kunen (ed.) J.E. Vaughan (ed.) , Handbook of Set-Theoretic Topology , North-Holland (1984) pp. 111–167 |
| [a3] | R. Engelking, "Hausdorff's gaps and limits and compactifications" , Theory of Sets and Topology (in honour of F. Hausdorff) , Deutsch. Verlag Wissenschaft. (1972) pp. 89–94 |
Luzin theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Luzin_theorem&oldid=14126