Linear hyperbolic partial differential equation and system
A partial differential equation (or system) of the form
 | (1) |
for which at any point  of its domain of definition
of its domain of definition  one can distinguish among the real variables
one can distinguish among the real variables  (if necessary, after a suitable affine transformation of the independent variables) one variable, say
(if necessary, after a suitable affine transformation of the independent variables) one variable, say 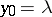 , in a such a way that for all points
, in a such a way that for all points  of the
of the  -dimensional Euclidean space
-dimensional Euclidean space  the characteristic equation (in
the characteristic equation (in 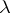 )
)
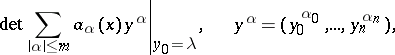 | (2) |
has exactly  real roots. Here
real roots. Here  is a vector with non-negative integer coordinates,
is a vector with non-negative integer coordinates, 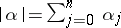 is the order of the differential operator
is the order of the differential operator 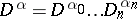 ,
, 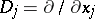 ,
,  ,
, 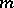 is the order of the system (1),
is the order of the system (1),  is a real square matrix of order
is a real square matrix of order 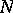 , defined in
, defined in  ,
, 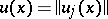 ,
, 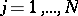 , is an unknown column vector, and
, is an unknown column vector, and  is a vector with
is a vector with  components, defined in
components, defined in  .
.
A typical example is the wave equation
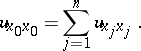 | (3) |
Many problems in mathematical physics reduce to linear hyperbolic partial differential equations or systems of equations.
A subset 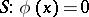 is said to be characteristic at a point
is said to be characteristic at a point  if
if 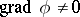 and
and 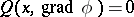 , where
, where
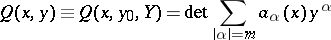 |
is the characteristic form of the system (1). If 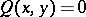 , then one says that the vector
, then one says that the vector 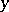 defines a characteristic direction or characteristic normal at the point
defines a characteristic direction or characteristic normal at the point  . The surface
. The surface 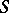 is called a characteristic surface (or characteristic) of the system (1) if
is called a characteristic surface (or characteristic) of the system (1) if
 |
A surface which does not have characteristic normals at any point is called a free surface. On a free surface the rank of the characteristic matrix
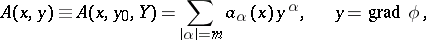 |
is equal to 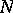 , while on a characteristic surface
, while on a characteristic surface 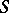 it is less than
it is less than  . A characteristic
. A characteristic  is said to be simple if for some
is said to be simple if for some  and all
and all 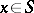 ,
,
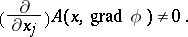 |
Otherwise the characteristic is said to be multiple. A characteristic is sometimes said to be simple if the rank of the matrix 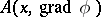 is
is 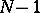 .
.
The system (1) is said to be hyperbolic at the point  with respect to the hyperplane
with respect to the hyperplane 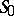 :
:  if the matrix
if the matrix 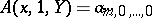 is non-singular (that is, the surface
is non-singular (that is, the surface 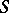 is free) and if all roots
is free) and if all roots  ,
,  , of the characteristic equation
, of the characteristic equation 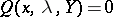 are real for all points
are real for all points 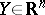 . The system (1) is said to be hyperbolic in the domain
. The system (1) is said to be hyperbolic in the domain  with respect to
with respect to 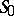 if it is hyperbolic with respect to
if it is hyperbolic with respect to  at every point
at every point  .
.
An important class of hyperbolic equations and systems consists of strictly hyperbolic equations and systems, which are sometimes called fully hyperbolic systems, or systems, hyperbolic in the narrow sense. A system (1) is called a strictly hyperbolic system if all roots  of the characteristic equation are distinct for any non-zero vector
of the characteristic equation are distinct for any non-zero vector 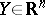 . The characteristics of a strictly hyperbolic equation (or system) are simple. Strictly hyperbolic (with respect to
. The characteristics of a strictly hyperbolic equation (or system) are simple. Strictly hyperbolic (with respect to  ) systems are notable for the fact that the Cauchy problem
) systems are notable for the fact that the Cauchy problem
 | (4) |
for them is well-posed under the single assumption of sufficient smoothness of the coefficients 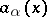 ,
,  of the system (1) and of the initial data (initial functions)
of the system (1) and of the initial data (initial functions) 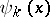 ,
, 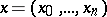 . There are examples of hyperbolic but not strictly hyperbolic equations of the form (1) (even with constant coefficients in front of the derivatives of order
. There are examples of hyperbolic but not strictly hyperbolic equations of the form (1) (even with constant coefficients in front of the derivatives of order  ) for which the Cauchy problem is ill-posed.
) for which the Cauchy problem is ill-posed.
The solution 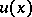 of the Cauchy problem (4) for the wave equation (3) can be written out explicitly, and when
of the Cauchy problem (4) for the wave equation (3) can be written out explicitly, and when 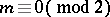 , and only then, it has the property that the value of
, and only then, it has the property that the value of 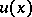 at the vertex
at the vertex 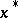 of the characteristic cone
of the characteristic cone 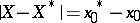 ,
, 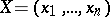 , depends only on the value of the initial data
, depends only on the value of the initial data  and
and 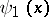 and their derivatives on the base
and their derivatives on the base 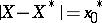 ,
,  , of this cone (the so-called Huygens principle).
, of this cone (the so-called Huygens principle).
For strictly hyperbolic (with respect to  ) equations and systems the question of the diffusion of waves and the related question of gaps have been investigated (cf. Lacuna). An exhaustive answer has been given in the case of an equation with constant coefficients of the form
) equations and systems the question of the diffusion of waves and the related question of gaps have been investigated (cf. Lacuna). An exhaustive answer has been given in the case of an equation with constant coefficients of the form
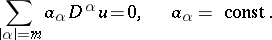 |
For the case of one (scalar) equation with constant coefficients 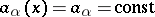 the definition of strict hyperbolicity has been generalized as follows. Equation (1) is said to be hyperbolic with respect to a non-zero vector
the definition of strict hyperbolicity has been generalized as follows. Equation (1) is said to be hyperbolic with respect to a non-zero vector 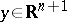 if
if
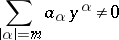 |
and if there is a real number 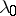 such that for all
such that for all 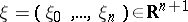 and
and 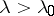 ,
,
 |
Of all linear equations with constant coefficients, only for equations that are hyperbolic in this sense the Cauchy problem is well-posed for arbitrary sufficiently smooth initial functions defined on the hyperplane
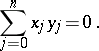 |
In particular, the wave equation (3) is hyperbolic in this sense with respect to any vector for which
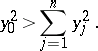 |
There are various generalizations of the definition of strict hyperbolicity of equations and systems. These are mainly equations and systems that are completely characterized by the fact that the Cauchy problem with data on a free surface is uniquely solvable for them for any sufficiently smooth initial functions, without any restrictions on the growth at infinity.
Another important class of linear hyperbolic systems of the first order is the class of symmetric hyperbolic systems. The system
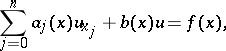 | (5) |
where  ,
, 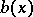 are square matrices of order
are square matrices of order 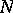 , defined in
, defined in  , and
, and  is an unknown vector of
is an unknown vector of 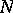 components, is called a symmetric hyperbolic system in
components, is called a symmetric hyperbolic system in  if the matrices
if the matrices 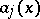 are symmetric (or are symmetrizable simultaneously by the same transformation) and if at every point there is a spatially-oriented hyperplane (or, space-like hyperplane), that is, a hyperplane whose normal
are symmetric (or are symmetrizable simultaneously by the same transformation) and if at every point there is a spatially-oriented hyperplane (or, space-like hyperplane), that is, a hyperplane whose normal 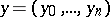 has the property that the matrix
has the property that the matrix 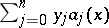 is positive definite. If for a symmetric hyperbolic system (5) with sufficiently smooth coefficients the given initial functions and the right-hand side have square-integrable generalized partial derivatives of order
is positive definite. If for a symmetric hyperbolic system (5) with sufficiently smooth coefficients the given initial functions and the right-hand side have square-integrable generalized partial derivatives of order 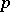 , then there is a unique generalized solution of the Cauchy problem with the same number of square-integrable partial derivatives. Any strictly hyperbolic partial differential equation of the second order reduces to a symmetric hyperbolic system.
, then there is a unique generalized solution of the Cauchy problem with the same number of square-integrable partial derivatives. Any strictly hyperbolic partial differential equation of the second order reduces to a symmetric hyperbolic system.
An equation (1) of the second order in the class of solutions regular in a domain  can be written in the form
can be written in the form
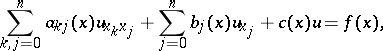 | (6) |
where 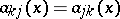 ,
,  ,
, 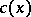 , and
, and 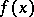 are functions defined in
are functions defined in  . Equation (6) is hyperbolic in
. Equation (6) is hyperbolic in  if at every point of
if at every point of  all eigen values of the matrix of leading coefficients
all eigen values of the matrix of leading coefficients  ,
,  , are non-zero, and one of these eigen values differs in sign from all others. With respect to (6), along with the characteristic surface one can distinguish two types of smooth surfaces: spatially-oriented surfaces and time-oriented surfaces (also called space-like and time-like surfaces). If the surfaces are given by an equation of the form
, are non-zero, and one of these eigen values differs in sign from all others. With respect to (6), along with the characteristic surface one can distinguish two types of smooth surfaces: spatially-oriented surfaces and time-oriented surfaces (also called space-like and time-like surfaces). If the surfaces are given by an equation of the form  , then on a surface of the first type
, then on a surface of the first type 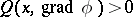 , while on a surface of the second type
, while on a surface of the second type 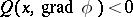 , where
, where
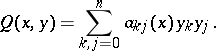 |
The Cauchy problem for hyperbolic partial differential equations with initial data on a time-like surface is generally not well-posed.
A hyperbolic partial differential equation
 |
is said to be uniformly (or regularly) hyperbolic in a domain  if there is a positive number
if there is a positive number  such that
such that
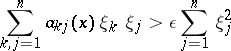 |
for all  in
in  and for any non-zero vector
and for any non-zero vector  . For
. For 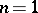 the inequality
the inequality
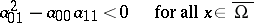 |
is a necessary and sufficient condition for (6) to be uniformly hyperbolic in  . The equation for the vibration of a string,
. The equation for the vibration of a string,
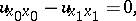 |
is a typical representative of a linear uniformly hyperbolic partial differential equation of the second order with two independent variables. The general solution of this equation in any convex domain  of the plane
of the plane 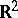 is given by the d'Alembert formula:
is given by the d'Alembert formula:
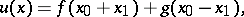 |
where 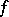 and
and 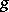 are arbitrary functions.
are arbitrary functions.
After a non-singular real change of variables  and
and 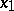 , the hyperbolic partial differential equation (6) with
, the hyperbolic partial differential equation (6) with 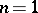 reduces to the normal (canonical) form
reduces to the normal (canonical) form
 | (7) |
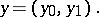 |
For hyperbolic systems written in the form (7), where  ,
, 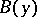 and
and 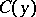 are given real square matrices of order
are given real square matrices of order 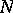 ,
, 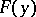 is a given vector and
is a given vector and  an unknown vector, both with
an unknown vector, both with 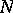 components, the question of the well-posedness of the Cauchy problem with initial data on a non-characteristic (free) curve and the Goursat problem with data on two intersecting characteristics have been completely investigated by the Riemann method.
components, the question of the well-posedness of the Cauchy problem with initial data on a non-characteristic (free) curve and the Goursat problem with data on two intersecting characteristics have been completely investigated by the Riemann method.
The main problems about hyperbolic equations are the following: the Cauchy problem, the Cauchy characteristic problem and the mixed problem (see also Mixed and boundary value problems for hyperbolic equations and systems).
In the investigation of the main problems an important role is played by fundamental solutions, which make it possible to obtain explicit (integral) representations of regular and generalized solutions and to establish their structural and qualitative properties, in particular to study the question of wave fronts and the propagation of discontinuities.
Equation (6) is called an ultra-hyperbolic equation in a domain  if at every point
if at every point 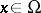 all eigen values of the matrix
all eigen values of the matrix 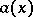 are non-zero and at least two of them differ in sign from all the others, of which there are at least two. An example of an ultra-hyperbolic equation is an equation of the form
are non-zero and at least two of them differ in sign from all the others, of which there are at least two. An example of an ultra-hyperbolic equation is an equation of the form
 | (8) |
which has the following property: If 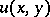 is a regular solution of (8) in a domain
is a regular solution of (8) in a domain  of the Euclidean space of the variables
of the Euclidean space of the variables 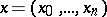 ,
, 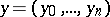 and if
and if 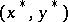 is an arbitrary point of
is an arbitrary point of  , then the mean value of the function
, then the mean value of the function 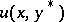 calculated on the sphere
calculated on the sphere  with centre at the point
with centre at the point 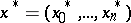 and radius
and radius  , is equal to the mean value of the function
, is equal to the mean value of the function  calculated on the sphere
calculated on the sphere 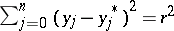 with centre at the point
with centre at the point  and the same radius
and the same radius  . This theorem is extensively used in the theory of linear hyperbolic partial differential equations of the second order with constant coefficients.
. This theorem is extensively used in the theory of linear hyperbolic partial differential equations of the second order with constant coefficients.
References
| [1] | J. Hadamard, "Lectures on Cauchy's problem in linear partial differential equations" , Dover, reprint (1952) |
| [2] | L. Bers, F. John, M. Schechter, "Partial differential equations" , Interscience (1964) |
| [3] | A.V. Bitsadse, "Equations of mixed type" , Pergamon (1964) (Translated from Russian) |
| [4] | A.V. Bitsadze, "Equations of mathematical physics" , MIR (1980) (Translated from Russian) |
| [5] | V.S. Vladimirov, "Equations of mathematical physics" , MIR (1984) (Translated from Russian) |
| [6] | I.M. Gel'fand, G.E. Shilov, "Generalized functions" , Acad. Press (1964) (Translated from Russian) |
| [7] | L. Gårding, "Cauchy's problem for hyperbolic equations" , Third Congress Scand. Math. Helsinki, 1957 , Mercators Tryckeri (1958) pp. 104–109 |
| [8] | A.A. Dezin, "Boundary problems for certain symmetric linear first-order systems" Mat. Sb. , 49 : 4 (1959) pp. 459–484 (In Russian) |
| [9] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) |
| [10] | O.A. Ladyzhenskaya, "A mixed problem for a hyperbolic equation" , Moscow (1953) (In Russian) |
| [11] | J. Leray, L. Gårding, T. Kotake, "Uniformisation et developpement asymptotique de la solution du problème de Cauchy linéaire à données holomorphes; analogie avec la théorie des ondes asymptotiques et approchées" Bull. Soc. Math. France , 92 (1964) pp. 236–361 |
| [12] | I.G. Petrovskii, "Partial differential equations" , Saunders (1967) (Translated from Russian) |
| [13] | V.I. Smirnov, "A course of higher mathematics" , 2 , Addison-Wesley (1964) (Translated from Russian) |
| [14] | A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian) |
| [15] | L. Hörmander, "Linear partial differential operators" , Springer (1976) |
Comments
A fully hyperbolic system, or system hyperbolic in the narrow sense, is also called a totally hyperbolic system or a system hyperbolic in the sense of Petrovskii.
For the definition of hyperbolicity in the case of scalar equations with constant coefficients, see [a1], Vol. II, Chapt. 12.
For fundamental solutions and propagation of singularities, see also [a1], Vol. III, Chapts. 13, 14.
The theorem mentioned in the last line of the main article above is called Asgeirsson's theorem, cf. [9], Chapt. 6.
References
| [a1] | L.V. Hörmander, "The analysis of linear partial differential operators" , 1–4 , Springer (1983–1985) |
| [a2] | P.R. Garabedian, "Partial differential equations" , Wiley (1964) |
| [a3] | F. John, "Partial differential equations" , Springer (1974) |
Linear hyperbolic partial differential equation and system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Linear_hyperbolic_partial_differential_equation_and_system&oldid=11206