Linear group

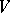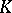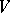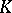A group of linear transformations of a vector space of finite dimension over some skew-field . The choice of a basis in realizes a linear group as a group of non-singular square -matrices over . In this way an isomorphism is established between linear and matrix groups.

The group of all automorphisms of a free -module 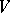is also called the general linear group (full linear group) and is denoted by 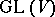, and the group of all invertible 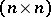-matrices (also called the general linear group) is denoted by 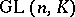or 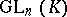. A subgroup of 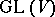is called a linear group of -matrices or linear group of order . The theory of linear groups is most developed when 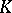is commutative, that is, 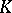is a field. Therefore henceforth (unless stated otherwise) only linear groups over a field will be considered.

The theory of linear groups arose in the middle of the 19th century and was developed in close connection with the theory of Lie groups and Galois theory. The beginning of a systematic investigation of linear groups was made in the work of C. Jordan (see [1]). The connection with Galois theory first led to the study of solvable and classical linear groups (see Classical group), over a prime field. Some general facts were established about the reducibility or irreducibility of a linear group 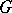, that is, concerning properties of the 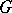-module 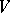. For every linear group 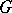there is a composition series of 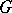-submodules

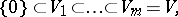such that all quotient modules are irreducible. In other words, every matrix group is conjugate in 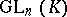to a group of quasi-triangular form with irreducible diagonal blocks. Let 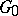be the subgroup of 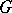consisting of all elements that act trivially on the quotients 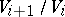, 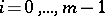. Then 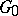is a normal nilpotent subgroup whose elements satisfy (in the 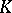-algebra of all linear transformations of 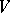) the equation 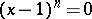; such linear groups are said to be unipotent. Every unipotent group, regarded as a matrix group, is conjugate in 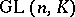to some subgroup of the group of upper triangular matrices with unit diagonal. To a substantial extent the structure of the quotient 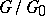is determined by the structure of the irreducible linear groups 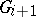induced by in the quotients . 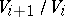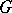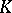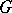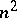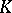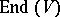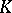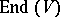If a linear group is irreducible over an algebraically closed field , then contains linearly independent (over ) elements of the -algebra , that is, the -linear hull of coincides with ( "Burnside theoremBurnside's theorem" ). Every normal subgroup of a completely reducible linear group is completely reducible.

Infinite linear groups.

Although the theory of linear groups has quite a long history, general methods were created comparatively recently. Only the solvable and classical linear groups constitute an exception. In 1870, Jordan investigated the structure of solvable linear groups over finite fields and obtained a number of classification results concerning these groups. These investigations received a further development (see [13]): A detailed study was made of the structure, and the maximal solvable and locally nilpotent subgroups of 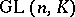over an algebraically closed field 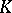were classified. The main structure theorem on solvable linear groups was obtained by A.I. Mal'tsev in 1951 (see [8b]): A solvable linear group of 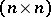-matrices over an algebraically closed field has a normal subgroup 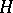of finite index such that 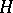is conjugate to a subgroup of the triangular group, and the index is bounded by an explicit function of (see also Lie–Kolchin theorem); in particular, the commutator subgroup of 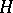is a unipotent group and, from the abstract point of view, 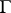is a finite extension of 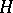with nilpotent commutator subgroup.

An important and much studied branch of the theory of linear groups is the theory of classical groups (see [4] and [7], for example).

A new stage of development of the theory of linear groups began in the 1960-s, when a general method of investigation was created, based on the technique of algebraic groups (see Linear algebraic group and also [9], [18]). This method made it possible to solve a number of problems in the theory of linear groups. For example, by means of it the theorem on free subgroups of a linear group was proved (see [14]): Every linear group over a field of characteristic zero either contains a non-Abelian free subgroup or has a solvable subgroup of finite index. A theory of periodic linear groups was constructed (see [9]) (it turned out that the main structure results of the theory of finite groups (cf. Finite group) are preserved in the more general case of periodic linear groups).

Another important method in the theory of linear groups, the so-called method of approximation, was first used by Mal'tsev in 1940 (see ). It is suitable for the investigation of linear groups over integral domains of finite type, in particular for linear groups with finitely many generators. The essence of the method is the following: Let 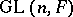be a general linear group over a finitely-generated subring 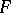of the field 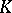; then 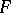can be approximated modulo maximal ideals by finite fields 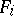, which implies an approximation of the group by finite matrix groups 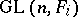. For every subgroup 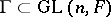one obtains an induced approximation by finite linear groups 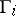. It turns out that in many cases the properties of the group 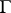are determined to a large extent by properties of the groups 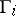. This method was perfected later (see [18]), which led to a proof of a general approximation theorem from which most of the results about infinite linear groups with finitely many generators can be derived.

Finite linear groups.

The most outstanding structure result about finite linear groups up to now is Jordan's theorem (1878): There is an integral-valued function 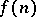such that every finite linear group of 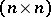-matrices over a field of characteristic zero has an Abelian normal subgroup of index less than 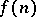. For fields of positive characteristic there are infinite series of simple finite groups for a fixed , and so Jordan's theorem does not carry over directly to this case. Nevertheless, by using modular representations of finite groups it has been proved that there is an integral-valued function 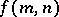such that a finite linear group of 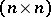-matrices over a field of characteristic 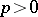for which the order of the Sylow 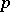-subgroup (cf. Sylow subgroup) does not exceed 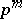has an Abelian normal subgroup of index less than 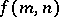(see [16]).

One of the main problems in the theory of finite linear groups is that of classifying simple linear groups. Since L. Dickson in 1901 presented [2] the main facts about the classical simple finite linear groups, many new results have been obtained. Among these a central place is taken by the results of C. Chevalley (see [15]), who used methods of the theory of Lie algebras to construct simple finite linear groups; this led to the discovery of new types of simple finite linear groups and made it possible to obtain almost-all known simple finite linear groups by a uniform method (for more details see [11], [12]).

Linear groups over skew-fields and rings.

A systematic investigation of linear groups over a non-commutative skew-field 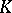began after the work of J. Dieudonné in 1943 (see [5]), in which he described the construction of a determinant over a skew-field (see Determinant). The subgroup of 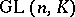of transformations with determinant 1 is called the special linear group and is denoted by 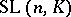. 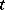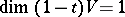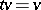It is generated by transvections (transformations such that and for 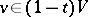), and every invariant subgroup of 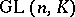is either scalar or contains , except for the cases , , 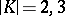when is solvable. 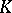If is finite dimensional over its centre , then there is a unique determinant with values in 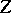, called the reduced norm (see [5]), and 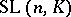is contained in the group 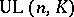of elements with reduced norm . The question, posed in 1943, of whether these groups coincide (the Tannaka–Artin problem, cf. Kneser–Tits hypothesis) was solved negatively in [10]. The group 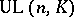and the quotient group 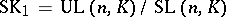, called the reduced Whitehead group, play an important role in the theory of linear algebraic groups and in algebraic 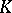-theory [5].

The main questions in the theory of linear groups over rings are connected with the description of normal subgroups of general linear groups and other classical groups. Progress in this area is very closely connected with the development of algebraic 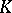-theory (see [5]). Thus, the problem of describing the normal subgroups of 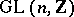, where is the ring of integers, is actually equivalent to the congruence problem for the group 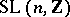when 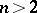. Namely, every non-scalar normal subgroup of 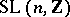, , has finite index and is a congruence subgroup, while 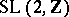is a finite extension of a free group and therefore has several normal subgroups of infinite index.

Automorphisms of the classical linear groups have also been studied over fields and over rings (see [19]).

References

[1]	C. Jordan, "Traité des substitutions et des équations algébriques" , Paris (1870) pp. 114–125
[2]	L.E. Dickson, "Linear groups" , Teubner (1901)
[3]	J.D. Dixon, "The structure of linear groups" , v. Nostrand-Reinhold (1971)
[4]	E. Artin, "Geometric algebra" , Interscience (1957)
[5]	H. Bass, "Algebraic 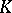-theory" , Benjamin (1968)
[6]	A. Borel, "Linear algebraic groups" , Benjamin (1969)
[7]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1955)
[8a]	A.I. [A.I. Mal'tsev] Mal'cev, "On the faithful representation of infinite groups by matrices" Transl. Amer. Math. Soc. (2) , 45 (1965) pp. 1–18
[8b]	A.I. [A.I. Mal'tsev] Mal'cev, "On some classes of infinite solvable groups" Transl. Amer. Math. Soc. , 2 (1956) pp. 1–22
[9]	V.P. Platonov, "The theory of algebraic linear groups and periodic groups" Transl. Amer. Math. Soc. (2) , 69 (1968) pp. 61–110 Izv. Akad. Nauk SSSR Ser. Mat. , 30 : 3 (1966) pp. 573–620
[10]	V.P. Platonov, "The Tannaka–Artin problem and reduced -theory" Math. USSR Izv. , 10 (1976) pp. 211–243 Izv. Akad. Nauk SSSR Ser. Mat. , 40 : 2 (1976) pp. 227–261
[11]	A. Borel (ed.) R. Carter (ed.) C.W. Curtis (ed.) N. Iwahori (ed.) T.A. Springer (ed.) R. Steinberg (ed.) , Seminar on algebraic groups and related finite groups , Lect. notes in math. , 131 , Springer (1970)
[12]	R.G. Steinberg, "Lectures on Chevalley groups" , Yale Univ. Press (1968)
[13]	D.A. Suprunenko, "Matrix groups" , Amer. Math. Soc. (1976) (In Russian)
[14]	J. Tits, "Free subgroups in linear groups" J. of Algebra , 20 (1972) pp. 250–270
[15]	C. Chevalley, "Sur certains groupes simples" Tôhoku Math. J. , 7 (1955) pp. 14–66
[16]	R. Brauer, W. Feit, "An analogue of Jordan's theorem in characteristic 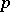" Ann. of Math. (2) , 84 : 1 (1966) pp. 119–131
[17]	P. Draxl, M. Kneser, "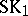 von Schiefkörpen" , Springer (1980)
[18]	B. Wehfritz, "Infinite linear groups" , Springer (1973)
[19]	Yu.I. Merzlyakov, "Linear groups" J. Soviet Math. , 1 : 5 (1973) pp. 571–593 Itogi Nauk. Algebra Topol. Geom. 1970 (1971) pp. 75–110

Comments

For a treatment of Chevalley groups (especially finite Chevalley groups) see [a1].

References

[a1]	R.W. Carter, "Simple groups of Lie type" , Wiley (Interscience) (1972)
[a2]	O.T. O'Meara, "A survey of the isomorphism theory of the classical groups" , Ring theory and algebra , 3 , M. Dekker (1980) pp. 225–242