Lie theorem
Lie's theorem is one of the three classical theorems in the theory of Lie groups that describe the connection between a local Lie group (cf. Lie group, local) and its Lie algebra. Lie's theorems are the foundations of the theory developed in the 19th century by S. Lie and his school (see ).
Let 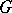 be an
be an  -dimensional real effective local Lie transformation group of a domain
-dimensional real effective local Lie transformation group of a domain 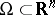 , let
, let  be the identity of
be the identity of 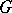 and suppose that in local coordinates in a neighbourhood of the set
and suppose that in local coordinates in a neighbourhood of the set 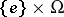 in
in 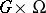 the action of
the action of 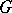 on
on  is given by a system of analytic functions
is given by a system of analytic functions
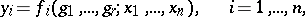 | (1) |
where 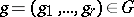 ,
, 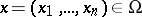 and
and 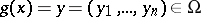 . This action defines
. This action defines  analytic vector fields on
analytic vector fields on  ,
,
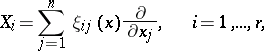 | (2) |
where 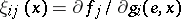 .
.
Lie's first theorem establishes that the functions 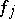 ,
, 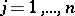 , which define the action of
, which define the action of 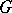 are themselves defined by some auxiliary system of analytic functions
are themselves defined by some auxiliary system of analytic functions  ,
, 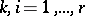 , on
, on  which satisfy the condition
which satisfy the condition
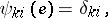 | (3) |
where 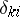 is the Kronecker symbol. More precisely,
is the Kronecker symbol. More precisely, 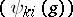 is the matrix of the differential of the right translation of
is the matrix of the differential of the right translation of 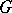 by the element
by the element 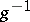 at the point
at the point 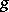 , and the system of functions (1) is precisely the solution of the system of equations
, and the system of functions (1) is precisely the solution of the system of equations
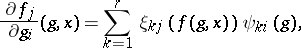 | (4) |
 |
that satisfies the initial conditions 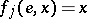 ,
,  .
.
Lie's second theorem describes the properties of the functions 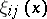 and
and 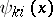 . Namely, the
. Namely, the 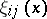 satisfy the system of equations
satisfy the system of equations
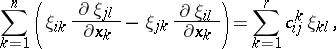 | (5) |
 |
(this system is the condition that the system (4) is integrable), and the functions 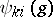 satisfy the system of equations
satisfy the system of equations
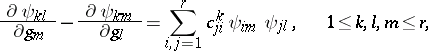 |
where the 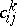 are certain constants. The relations (5) imply that the commutator (Lie bracket)
are certain constants. The relations (5) imply that the commutator (Lie bracket) 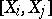 of two vector fields
of two vector fields  and
and  is a linear combination of the fields
is a linear combination of the fields 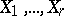 with constant coefficients:
with constant coefficients:
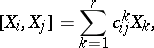 | (6) |
that is, the linear hull 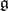 of the fields
of the fields 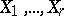 is an algebra with respect to the Lie bracket.
is an algebra with respect to the Lie bracket.
The converse of Lie's first and second theorems is the following: If the functions 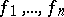 give a solution of (4) in which the matrix
give a solution of (4) in which the matrix 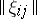 has maximal rank and if (3) and (5) are satisfied, then (1) determines a local effective Lie transformation group. This local group is generated by the one-parameter transformation groups given by (2).
has maximal rank and if (3) and (5) are satisfied, then (1) determines a local effective Lie transformation group. This local group is generated by the one-parameter transformation groups given by (2).
Lie's third theorem asserts that the constants  satisfy the following relations:
satisfy the following relations:
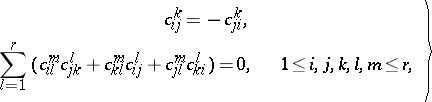 | (7) |
that is, 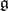 is a Lie algebra. The converse of the third theorem is important: If the
is a Lie algebra. The converse of the third theorem is important: If the 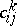 are any constants satisfying (7), then there is a system of vector fields
are any constants satisfying (7), then there is a system of vector fields 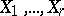 satisfying (6), and these vector fields arise by means of the construction described above from some local Lie transformation group (in other words, every finite-dimensional Lie algebra is the Lie algebra of some local Lie transformation group). Lie's third theorem is sometimes (see , for example) taken to be the assertion about the existence, for every finite-dimensional Lie algebra
satisfying (6), and these vector fields arise by means of the construction described above from some local Lie transformation group (in other words, every finite-dimensional Lie algebra is the Lie algebra of some local Lie transformation group). Lie's third theorem is sometimes (see , for example) taken to be the assertion about the existence, for every finite-dimensional Lie algebra 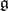 over
over 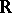 or
or 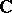 , of a global Lie group with Lie algebra
, of a global Lie group with Lie algebra 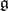 (see Lie algebra of an analytic group).
(see Lie algebra of an analytic group).
Lie's theorem on solvable Lie algebras: Let 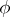 be a linear representation of a finite-dimensional solvable Lie algebra (cf. Lie algebra, solvable)
be a linear representation of a finite-dimensional solvable Lie algebra (cf. Lie algebra, solvable) 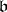 in a vector space
in a vector space 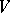 over an algebraically closed field of characteristic 0; then there is a basis of
over an algebraically closed field of characteristic 0; then there is a basis of 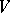 in which all the operators
in which all the operators 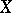 of
of 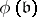 are written as upper triangular matrices. A similar assertion is true for a linear continuous representation of a connected topological solvable group in a finite-dimensional complex vector space (the group-theoretic analogue of Lie's theorem); the assumption that the group is connected is essential. A version of the group-theoretic analogue of Lie's theorem is known as the Lie–Kolchin theorem.
are written as upper triangular matrices. A similar assertion is true for a linear continuous representation of a connected topological solvable group in a finite-dimensional complex vector space (the group-theoretic analogue of Lie's theorem); the assumption that the group is connected is essential. A version of the group-theoretic analogue of Lie's theorem is known as the Lie–Kolchin theorem.
References
| [1] | S. Lie, F. Engel, "Theorie der Transformationsgruppen" , 1–3 , Leipzig (1888–1893) |
| [2] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) |
| [3] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
| [4] | J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) |
| [5] | C. Chevalley, "Théorie des groupes de Lie" , 3 , Hermann (1955) |
| [6] | N.G. Chebotarev, "The theory of Lie groups" , Moscow-Leningrad (1940) (In Russian) |
Comments
For part 1) of the main article above see also Frobenius theorem on Pfaffian systems.
References
| [a1] | A. Borel, "Linear algebraic groups" , Benjamin (1969) |
| [a2] | V.S. Varadarajan, "Lie groups, Lie algebras, and their representations" , Prentice-Hall (1974) |
| [a3] | S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) pp. 121 |
Lie theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_theorem&oldid=15957