Lie group, compact
A compact group that is a finite-dimensional real Lie group. Compact Lie groups can be characterized as finite-dimensional locally connected compact topological groups.
If 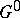 is the connected component of the identity of a compact Lie group
is the connected component of the identity of a compact Lie group 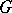 , then the group of connected components
, then the group of connected components 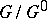 is finite. The study of the structure of connected compact Lie groups is a basic topic in the theory of Lie groups.
is finite. The study of the structure of connected compact Lie groups is a basic topic in the theory of Lie groups.
The following examples of connected compact Lie groups play an important role in the general structure theory of compact Lie groups.
1) The multiplicative group  of all complex numbers of modulus 1.
of all complex numbers of modulus 1.
2) The group  of all complex unitary matrices of order
of all complex unitary matrices of order  with determinant 1.
with determinant 1.
3) The group 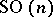 of all real orthogonal matrices of order
of all real orthogonal matrices of order  with determinant 1.
with determinant 1.
4) The group 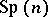 of all matrices
of all matrices  for which
for which  , where
, where
 |
 is the transposition sign and
is the transposition sign and 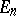 is the unit matrix of order
is the unit matrix of order  .
.
A complete classification of connected compact Lie groups was obtained in the works of E. Cartan [1] and H. Weyl [2]. It is as follows.
There are two basic types of connected compact Lie groups.
1) Connected commutative compact Lie groups. These are precisely the tori, that is, groups of the form 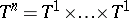 (
( factors) (cf. also Torus).
factors) (cf. also Torus).
2) Connected semi-simple compact Lie groups (see Lie group, semi-simple). If 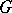 is a connected semi-simple compact Lie group, then the universal covering group
is a connected semi-simple compact Lie group, then the universal covering group 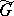 of
of 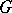 is also a compact Lie group (Weyl's theorem). The centre
is also a compact Lie group (Weyl's theorem). The centre 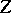 of
of 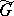 is finite, and all connected Lie groups locally isomorphic to
is finite, and all connected Lie groups locally isomorphic to  are compact and are, up to isomorphism, the groups of the form
are compact and are, up to isomorphism, the groups of the form 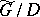 , where
, where 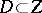 . The Lie algebras of semi-simple compact Lie groups can be intrinsically characterized among all finite-dimensional real Lie algebras as algebras with negative-definite Killing form.
. The Lie algebras of semi-simple compact Lie groups can be intrinsically characterized among all finite-dimensional real Lie algebras as algebras with negative-definite Killing form.
The two basic types of connected Lie groups indicated above determine the structure of arbitrary compact Lie groups. Namely, the latter are, up to isomorphism, all groups of the form 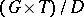 , where
, where 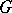 is a connected simply-connected compact Lie group with centre
is a connected simply-connected compact Lie group with centre  ,
, 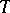 is a torus and
is a torus and 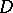 is a finite subgroup of the group
is a finite subgroup of the group 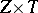 which intersects
which intersects 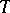 only in the identity. The Lie algebras of arbitrary compact Lie groups can also be characterized intrinsically among all finite-dimensional real Lie algebras: they are precisely the Lie algebras
only in the identity. The Lie algebras of arbitrary compact Lie groups can also be characterized intrinsically among all finite-dimensional real Lie algebras: they are precisely the Lie algebras 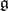 that have a positive-definite scalar product
that have a positive-definite scalar product  such that
such that 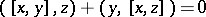 for any
for any 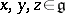 . They are called compact Lie algebras.
. They are called compact Lie algebras.
Thus, the classification of connected compact Lie groups reduces to the classification of connected simply-connected semi-simple compact Lie groups (or, equivalently, to the classification of semi-simple compact Lie algebras) and a description of their centres. It turns out that semi-simple compact Lie algebras are in one-to-one correspondence with semi-simple complex Lie algebras (and therefore with reduced root systems, cf. Root system). Namely, if  is a semi-simple compact Lie algebra, then its complexification
is a semi-simple compact Lie algebra, then its complexification 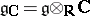 is semi-simple. Conversely, in any semi-simple Lie algebra over
is semi-simple. Conversely, in any semi-simple Lie algebra over 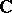 there is a unique (up to conjugacy) compact real form (cf. Form of an (algebraic) structure). In particular, the final result on the classification of simple compact Lie algebras and the connected simply-connected compact Lie groups corresponding to them is the following. There are four infinite series of so-called classical simple compact Lie algebras that correspond to the following series of irreducible reduced root systems:
there is a unique (up to conjugacy) compact real form (cf. Form of an (algebraic) structure). In particular, the final result on the classification of simple compact Lie algebras and the connected simply-connected compact Lie groups corresponding to them is the following. There are four infinite series of so-called classical simple compact Lie algebras that correspond to the following series of irreducible reduced root systems: 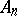 ,
, 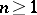 ,
, 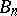 ,
, 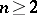 ,
,  ,
, 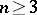 , and
, and 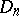 ,
,  . They are, respectively, the Lie algebras of the groups
. They are, respectively, the Lie algebras of the groups 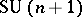 ,
, 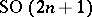 ,
, 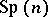 , and
, and 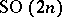 . Apart from these there are the five so-called exceptional simple compact Lie algebras corresponding to the root systems of the types
. Apart from these there are the five so-called exceptional simple compact Lie algebras corresponding to the root systems of the types 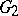 ,
, 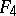 ,
, 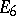 ,
,  , and
, and  . Any compact simple Lie algebra is isomorphic to one of these Lie algebras, and they themselves are not pairwise isomorphic to one another. The compact Lie groups
. Any compact simple Lie algebra is isomorphic to one of these Lie algebras, and they themselves are not pairwise isomorphic to one another. The compact Lie groups 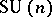 and
and 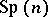 ,
, 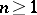 , are connected and simply connected. The group
, are connected and simply connected. The group 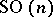 ,
, 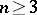 , is connected but not simply connected. Its universal covering is called the spinor compact Lie group and is denoted by
, is connected but not simply connected. Its universal covering is called the spinor compact Lie group and is denoted by 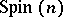 . The centre of a connected simply-connected semi-simple compact Lie group coincides with the centre of the corresponding simply-connected complex Lie group (see Lie group, semi-simple).
. The centre of a connected simply-connected semi-simple compact Lie group coincides with the centre of the corresponding simply-connected complex Lie group (see Lie group, semi-simple).
Any compact Lie group has a faithful linear representation; the image of such a representation is a real algebraic group. Any compact Lie group 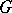 has a complexification
has a complexification 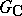 (see Complexification of a Lie group). Moreover,
(see Complexification of a Lie group). Moreover, 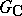 is a complex reductive algebraic group (cf. Reductive group) whose affine algebra
is a complex reductive algebraic group (cf. Reductive group) whose affine algebra 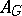 can be described as the algebra of all representation functions on
can be described as the algebra of all representation functions on 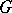 , that is, continuous complex-valued functions
, that is, continuous complex-valued functions 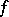 such that the linear envelope of the translates of
such that the linear envelope of the translates of 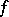 by elements of
by elements of 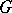 is finite-dimensional. The algebra
is finite-dimensional. The algebra 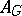 has a natural real structure and so it determines an algebraic group over
has a natural real structure and so it determines an algebraic group over 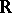 . The real points of this group form
. The real points of this group form 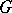 , and the complex points form
, and the complex points form 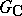 . The group
. The group 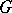 is a maximal compact subgroup in
is a maximal compact subgroup in 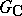 . As a result one obtains a one-to-one correspondence between the classes of isomorphic compact Lie groups and reductive algebraic groups over
. As a result one obtains a one-to-one correspondence between the classes of isomorphic compact Lie groups and reductive algebraic groups over 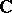 .
.
Any compact Lie group is a real-analytic group. Compact complex-analytic groups are also called complex compact Lie groups. Any connected complex compact Lie group (as a complex Lie group) is isomorphic to a complex torus 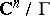 , where
, where 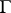 is a discrete subgroup of rank
is a discrete subgroup of rank 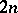 in
in 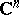 , and (as a real Lie group) it is isomorphic to
, and (as a real Lie group) it is isomorphic to 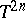 . Two complex tori
. Two complex tori 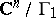 and
and 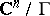 are isomorphic (as complex Lie groups) if and only if
are isomorphic (as complex Lie groups) if and only if 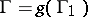 for some
for some 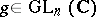 .
.
References
| [1] | E. Cartan, "La topologie des groupes de Lie" , Hermann (1936) |
| [2] | H. Weyl, Uspekhi Mat. Nauk , 4 (1938) pp. 201–246 |
| [3] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
| [4] | , Theórie des algèbres de Lie. Topologie des groupes de Lie , Sem. S. Lie , Ie année 1954–1955 , Ecole Norm. Sup. (1955) |
| [5] | D.P. Zhelobenko, "Compact Lie groups and their representations" , Amer. Math. Soc. (1973) (Translated from Russian) |
| [6] | M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) |
| [7] | A.L. Onishchik, E.B. Vinberg, "Seminar on algebraic groups and Lie groups, 1967/68" , Moscow (1969) (In Russian) |
| [8] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) |
| [9] | J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) |
| [10] | J.F. Adams, "Lectures on Lie groups" , Benjamin (1969) |
Comments
References
| [a1] | Th. Bröcker, T. Tom Dieck, "Representations of compact Lie groups" , Springer (1985) |
Lie group, compact. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_group,_compact&oldid=14523