Lagrangian
Lagrange function, Lagrange integrand
The integrand  in the problem of an extremum for the functional
in the problem of an extremum for the functional
 | (*) |
the extremum problem is solved under the possible imposition of constraints and boundary conditions; here  ,
, 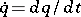 and
and  is an arbitrary differentiable mapping
is an arbitrary differentiable mapping  .
.
The term "Lagrangian" arises in classical mechanics, where in the simplest case the Lagrangian is the difference between the kinetic and the potential energy of the system, and the motions of the system coincide with the extremals of the corresponding integral functional (the principle of stationary action). In the general case in classical mechanics, the Lagrangian is an arbitrary differentiable mapping  , where
, where  is the tangent bundle of a differentiable manifold
is the tangent bundle of a differentiable manifold  (the configuration manifold of the system).
(the configuration manifold of the system).
A necessary condition for a weak extremum of the functional (*) in the absence of constraints is given by the Euler–Lagrange equation
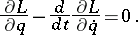 |
The presence of constraints of equality type is taken into account by means of Lagrange multipliers. In the presence of "non-classical" constraints of inequality type, in the theory of optimal control, a necessary condition for a strong extremum of the functional (*) is given by the Pontryagin maximum principle.
Using the concept of the Lagrangian it is convenient to consider the various symmetries of the system, since to any one-parameter group of diffeomorphisms of the configuration manifold that preserve the Lagrangian there corresponds a first integral of the equations of motion (Noether's theorem).
In many problems it is useful to rewrite the Euler–Lagrange equations in the form of the Hamilton equations, which makes it possible, in particular, to use the method of canonical transformations and Hamilton–Jacobi theory. Transition to the Hamiltonian form is also useful in the quantization of the original classical system. If the Lagrangian is not degenerate, then transition to the Hamiltonian formulation is carried out by means of the Legendre transform; in the case of degeneracy a more complicated procedure for reduction is used (see [1], [6]).
In the mechanics of continuous media and in quantum field theory, in which fields can be regarded as mechanical systems with infinitely many degrees of freedom, one considers extremal problems for functionals of the form
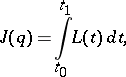 |
where
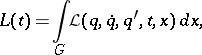 |
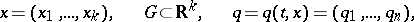 |
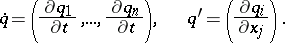 |
In this case the functional 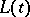 is called the Lagrangian, and the function
is called the Lagrangian, and the function 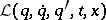 is called the density of the Lagrangian. The basic concepts for such systems (the Hamiltonian, etc.) are introduced by analogy with classical mechanics.
is called the density of the Lagrangian. The basic concepts for such systems (the Hamiltonian, etc.) are introduced by analogy with classical mechanics.
References
| [1] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
| [2] | N.N. Bogolyubov, D.V. Shirkov, "Introduction to the theory of quantized fields" , Interscience (1959) (Translated from Russian) |
| [3] | A.D. Ioffe, V.M. Tikhomirov, "Theory of extremal problems" , North-Holland (1979) (Translated from Russian) |
| [4] | L.S. Pontryagin, V.G. Boltayanskii, R.V. Gamkrelidze, E.F. Mishchenko, "The mathematical theory of optimal processes" , Wiley (1962) (Translated from Russian) |
| [5] | S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964) |
| [6] | L.D. Faddeev, "The Feynman integral for singular Lagrangians" Teoret. i Mat. Fiz. , 1 : 1 (1969) pp. 1–8 (In Russian) (English abstract) |
Comments
References
| [a1] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
| [a2] | P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) pp. Chapt. 2, Sects. 10–13 (Translated from French) |
Lagrangian. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lagrangian&oldid=13584