Integral exponential function

The special function defined for real 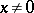by the equation

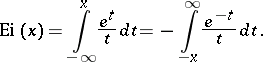The graph of the integral exponential function is illustrated in Fig..

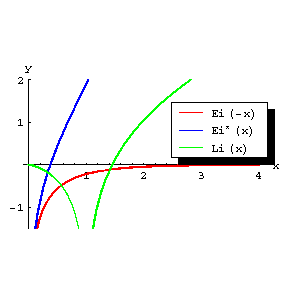
Figure: i051440a

Graphs of the functions 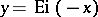, 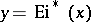and .

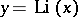For 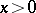, the function 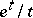has an infinite discontinuity at 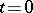, and the integral exponential function is understood in the sense of the principal value of this integral:

The integral exponential function can be represented by the series

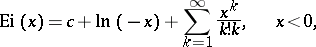(1)

and

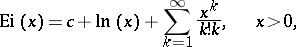(2)

where is the Euler constant.

There is an asymptotic representation:

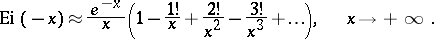As a function of the complex variable , the integral exponential function

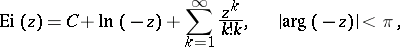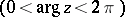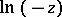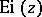is a single-valued analytic function in the -plane slit along the positive real semi-axis ; here the value of is chosen such that . The behaviour of close to the slit is described by the limiting relations:

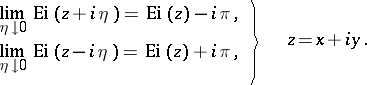The asymptotic representation in the region 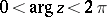is:

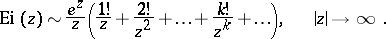The integral exponential function is related to the integral logarithm 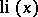by the formulas

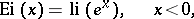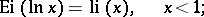and to the integral sine 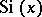and the integral cosine 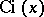by the formulas:

The differentiation formula is:

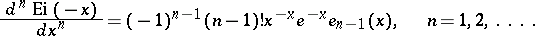The following notations are sometimes used:

References

[1]	H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , Higher transcendental functions , 2. Bessel functions, parabolic cylinder functions, orthogonal polynomials , McGraw-Hill (1953)
[2]	E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German)
[3]	A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960)
[4]	N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian)

Comments

The function 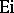is usually called the exponential integral.

Instead of by the series representation, for complex values of ( not positive real) the function 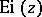can be defined by the integal (as for real ); since the integrand is analytic, the integral is path-independent in .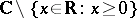

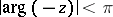Formula (1) with replaced by then holds for , and the function defined by (2) (for 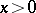) is also known as the modified exponential integral.