Gauss sum
A trigonometric sum of the form
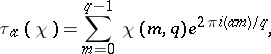 |
where 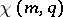 is a numerical character modulo
is a numerical character modulo 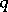 . A Gauss sum is fully defined by specifying the character
. A Gauss sum is fully defined by specifying the character 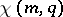 and the number
and the number  . Such sums were studied by C.F. Gauss (1811) for an odd prime number
. Such sums were studied by C.F. Gauss (1811) for an odd prime number  and the character
and the character 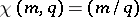 , where
, where 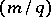 is the Legendre symbol. In such a case
is the Legendre symbol. In such a case
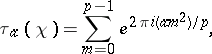 | (*) |
where 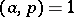 . By studying the properties of the sum (*), Gauss found the exact expression for the modulus of this sum:
. By studying the properties of the sum (*), Gauss found the exact expression for the modulus of this sum:
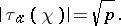 |
He also solved the more difficult problem of determining the sign of 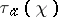 , and showed that
, and showed that
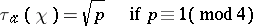 |
and
 |
Gauss utilized the properties of the sum (*) to solve certain problems in number theory; a particular case is one of the proofs of the quadratic reciprocity law.
The significance of Gauss sums in number theory only became evident in the 1920s. At that time H. Weyl used general trigonometric sums (cf. Weyl sum) in his study on uniform distribution. These sums were used at the same time by I.M. Vinogradov to obtain an estimate from above of the smallest quadratic non-residue modulo 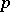 .
.
With the aid of Gauss sums it is possible to establish a relation between two important objects in number theory — viz. between the multiplicative characters  and the additive characters
and the additive characters
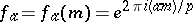 |
(only the case of a prime module  is considered, for the sake of simplicity). The set
is considered, for the sake of simplicity). The set 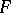 of all complex-valued functions
of all complex-valued functions  with period
with period 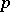 forms a
forms a 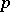 -dimensional vector space over the field of complex numbers. If one defines the scalar product in
-dimensional vector space over the field of complex numbers. If one defines the scalar product in 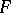 by putting
by putting
 |
then the functions 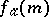 ,
, 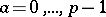 , form an orthonormal basis of
, form an orthonormal basis of 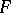 ; here
; here
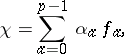 |
where 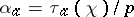 . Thus, the Gauss sums
. Thus, the Gauss sums 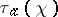 (up to the factor
(up to the factor 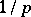 ) are coordinates in the expansion of the multiplicative character
) are coordinates in the expansion of the multiplicative character 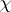 by the additive characters
by the additive characters 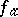 . The possibility of a linear representation of any character
. The possibility of a linear representation of any character 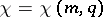 as a linear combination of exponents
as a linear combination of exponents 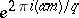 , which follows from the properties of Gauss sums of general type, forms the basis of the proof of the functional equation for the
, which follows from the properties of Gauss sums of general type, forms the basis of the proof of the functional equation for the 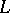 -function.
-function.
These ideas are effectively applied in the method of the large sieve, and in passing from an estimate of sums of additive characters to an estimate of sums of multiplicative characters. Gauss sums are also employed to represent  -functions by finite sums. Such a representation is employed in the problem of the number of divisor classes of a cyclotomic field.
-functions by finite sums. Such a representation is employed in the problem of the number of divisor classes of a cyclotomic field.
The problem of the sign of the Gauss sum 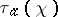 belonging to a quadratic character may be stated in a more general manner for a Gauss sum belonging to a character
belonging to a quadratic character may be stated in a more general manner for a Gauss sum belonging to a character 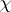 of order
of order 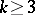 . The Kummer hypothesis on cubic Gauss sums with a prime module
. The Kummer hypothesis on cubic Gauss sums with a prime module  (
(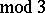 ), as well as its generalization to
), as well as its generalization to 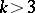 , arises.
, arises.
References
| [1] | C.F. Gauss, "Disquisitiones Arithmeticae" , Yale Univ. Press (1966) (Translated from Latin) |
| [2] | I.M. Vinogradov, "The method of trigonometical sums in the theory of numbers" , Interscience (1954) (Translated from Russian) |
| [3] | H. Davenport, "Multiplicative number theory" , Springer (1980) |
| [4] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
| [5] | H. Hasse, "Vorlesungen über Zahlentheorie" , Springer (1950) |
Comments
A numerical character modulo  is basically a character of the group of units
is basically a character of the group of units 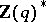 of the residue ring
of the residue ring 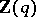 , i.e. a homomorphism of Abelian groups
, i.e. a homomorphism of Abelian groups 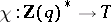 , where
, where 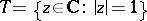 . Given such a
. Given such a 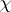 it is extended to a function on
it is extended to a function on 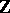 by setting
by setting
 |
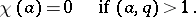 |
Here 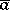 denotes the residue class of
denotes the residue class of  modulo
modulo 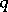 . This function
. This function 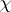 on
on 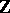 then satisfies
then satisfies 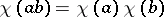 ,
, 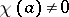 if and only if
if and only if 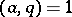 ,
, 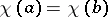 if
if 
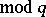 , and, conversely, any such function is a numerical character modulo
, and, conversely, any such function is a numerical character modulo 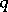 . There are
. There are 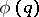 of them, where
of them, where 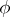 is the Euler function. Instead of "numerical character modulo q" one also sees the phrase residue character modulo
is the Euler function. Instead of "numerical character modulo q" one also sees the phrase residue character modulo 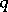 or Dirichlet character modulo
or Dirichlet character modulo 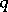 .
.
Gauss sum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gauss_sum&oldid=18033