Frobenius group
Suppose a finite group  contains a subgroup satisfying specific properties. Using that information, what can be said about the structure of
contains a subgroup satisfying specific properties. Using that information, what can be said about the structure of 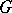 itself? One way to tackle such a problem is via character theory (cf. also Character of a group), another is by viewing
itself? One way to tackle such a problem is via character theory (cf. also Character of a group), another is by viewing  as a permutation group. A classical and beautiful application of character theory is provided in elucidating the structure of Frobenius groups. Namely, let
as a permutation group. A classical and beautiful application of character theory is provided in elucidating the structure of Frobenius groups. Namely, let  . Assume that
. Assume that 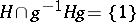 whenever
whenever  . Then
. Then 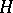 is a so-called Frobenius complement in
is a so-called Frobenius complement in  ; the group
; the group 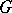 is then a Frobenius group by definition. It was proved by G. Frobenius in 1901, see [a3], that the set
is then a Frobenius group by definition. It was proved by G. Frobenius in 1901, see [a3], that the set
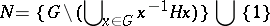 |
is in fact a normal subgroup of  . Almost a century later, Frobenius' proof that
. Almost a century later, Frobenius' proof that 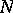 is a subgroup of
is a subgroup of 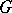 is still the only existing proof; it uses character theory! The normal subgroup
is still the only existing proof; it uses character theory! The normal subgroup 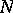 is called the Frobenius kernel of
is called the Frobenius kernel of 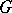 .
.
It can be shown that 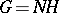 , that
, that 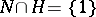 and that the orders of
and that the orders of 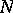 and
and 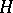 are relatively prime. Therefore, by the Schur–Zassenhaus theorem, all Frobenius complements in
are relatively prime. Therefore, by the Schur–Zassenhaus theorem, all Frobenius complements in 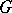 are conjugate to each other. Below, let
are conjugate to each other. Below, let 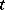 be an element of a group and let
be an element of a group and let  be a subset of that group; let
be a subset of that group; let 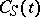 denote the set
denote the set 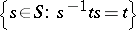 .
.
A finite Frobenius group 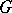 with Frobenius complement
with Frobenius complement 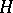 and corresponding Frobenius kernel
and corresponding Frobenius kernel 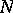 satisfies:
satisfies:
1) 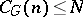 for all
for all  ;
;
2) 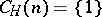 for all
for all 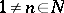 ;
;
3)  for all
for all 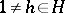 ;
;
4) every  is conjugate to an element of
is conjugate to an element of 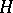 ;
;
5) if  , then
, then  is conjugate to every element of the coset
is conjugate to every element of the coset 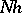 ;
;
6) each non-principal complex irreducible character of 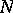 induces irreducibly to
induces irreducibly to 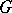 .
.
As a converse, assume that some finite group  contains a normal subgroup
contains a normal subgroup 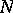 and some subgroup
and some subgroup 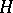 satisfying
satisfying 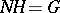 and
and 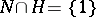 . Then the statements 1)–6) are all equivalent to each other, and if one of them is true, then
. Then the statements 1)–6) are all equivalent to each other, and if one of them is true, then 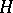 is a Frobenius complement of
is a Frobenius complement of 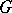 , turning
, turning 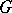 into a Frobenius group with
into a Frobenius group with 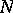 as corresponding Frobenius kernel. Even more general, if some finite group
as corresponding Frobenius kernel. Even more general, if some finite group 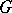 with proper normal subgroup
with proper normal subgroup 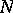 satisfies 1), then, applying one of the Sylow theorems, it is not hard to see that all orders of
satisfies 1), then, applying one of the Sylow theorems, it is not hard to see that all orders of 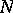 and
and 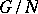 are relatively prime. Whence there exists a subgroup
are relatively prime. Whence there exists a subgroup 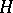 of
of  satisfying
satisfying 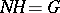 and
and  (by the Schur–Zassenhaus theorem). Thus, again
(by the Schur–Zassenhaus theorem). Thus, again 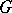 is a Frobenius group with Frobenius complement
is a Frobenius group with Frobenius complement 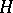 and Frobenius kernel
and Frobenius kernel 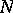 .
.
Viewed another way, suppose a finite group 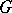 , containing a non-trivial proper subgroup
, containing a non-trivial proper subgroup 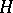 , acts transitively on a finite set
, acts transitively on a finite set  with
with  , such that
, such that 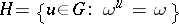 for some prescribed element
for some prescribed element 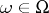 and such that only the identity of
and such that only the identity of  leaves invariant more than one element of
leaves invariant more than one element of  . Then
. Then 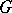 is a Frobenius group with Frobenius complement
is a Frobenius group with Frobenius complement 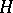 . Any element
. Any element 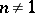 of the Frobenius kernel
of the Frobenius kernel 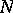 acts fixed-point freely on
acts fixed-point freely on  , i.e.
, i.e. 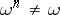 for each
for each  .
.
There is a characterization of finite Frobenius groups in terms of group characters only. Namely, let 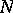 be a subgroup of a finite group
be a subgroup of a finite group 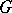 satisfying
satisfying 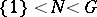 . Then the following assertions are equivalent:
. Then the following assertions are equivalent:
a) statement 6) above;
b) 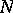 is a normal subgroup of
is a normal subgroup of  and
and 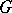 is a Frobenius group with Frobenius kernel
is a Frobenius group with Frobenius kernel 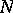 . The step from b) to a) was known to Frobenius; the converse step with, in addition,
. The step from b) to a) was known to Frobenius; the converse step with, in addition,  normal in
normal in 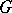 is surely due to Frobenius; however, the step from a) to b) with
is surely due to Frobenius; however, the step from a) to b) with  not necessarily normal in
not necessarily normal in 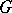 is due to E.B. Kuisch (see [a7]).
is due to E.B. Kuisch (see [a7]).
This characterization led Kuisch, and later R.W. van der Waall, to the study of so-called 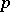 -modular Frobenius groups; see [a8]. Namely, let
-modular Frobenius groups; see [a8]. Namely, let 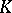 be a field of positive characteristic
be a field of positive characteristic 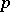 . Then
. Then 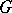 is a
is a 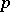 -modular Frobenius group if it contains a non-trivial normal subgroup
-modular Frobenius group if it contains a non-trivial normal subgroup 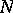 such that
such that 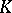 is a splitting field for the group algebra
is a splitting field for the group algebra 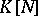 and if one of the following (equivalent) statements holds:
and if one of the following (equivalent) statements holds:
A) every non-principal irreducible 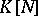 -module
-module 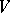 has the property that the induced
has the property that the induced  -module
-module  is irreducible;
is irreducible;
B) 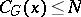 for every
for every 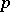 -regular non-trivial element
-regular non-trivial element 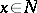 . Any
. Any 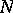 featuring in A)–B) is a
featuring in A)–B) is a 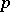 -modular Frobenius kernel.
-modular Frobenius kernel.
In 1959, J.G. Thompson [a9] showed that for a "classical" Frobenius group 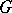 , the Frobenius kernel
, the Frobenius kernel 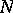 is nilpotent (cf. also Nilpotent group), thereby solving a long-standing conjecture of W.S. Burnside. It was proved by H. Zassenhaus in 1939, [a10], that a Sylow
is nilpotent (cf. also Nilpotent group), thereby solving a long-standing conjecture of W.S. Burnside. It was proved by H. Zassenhaus in 1939, [a10], that a Sylow 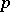 -subgroup (cf. also Sylow subgroup) of a Frobenius complement
-subgroup (cf. also Sylow subgroup) of a Frobenius complement  of
of 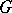 is cyclic (cf. also Cyclic group) when
is cyclic (cf. also Cyclic group) when 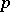 is odd, and cyclic or generalized quaternion if
is odd, and cyclic or generalized quaternion if 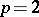 . He also proved that if
. He also proved that if 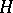 is not solvable (cf. also Solvable group), then it admits precisely one non-Abelian composition factor, namely the alternating group on five symbols.
is not solvable (cf. also Solvable group), then it admits precisely one non-Abelian composition factor, namely the alternating group on five symbols.
The situation is more involved for 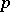 -modular Frobenius groups. Namely, a
-modular Frobenius groups. Namely, a 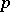 -modular Frobenius kernel
-modular Frobenius kernel 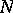 is either solvable (cf. also Solvable group) or else
is either solvable (cf. also Solvable group) or else 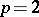 and any non–Abelian composition factor of
and any non–Abelian composition factor of 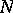 is isomorphic to
is isomorphic to 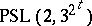 for some integer
for some integer 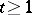 .
.
Furthermore, assume that 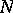 is not a
is not a 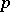 -group. Then:
-group. Then:
any Sylow 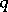 -subgroup of
-subgroup of  is cyclic whenever
is cyclic whenever 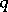 is relatively prime to
is relatively prime to 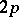 ;
;
any Sylow  -subgroup of
-subgroup of 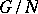 is cyclic or generalized quaternion if
is cyclic or generalized quaternion if  is odd. On the other hand, any non-trivial finite
is odd. On the other hand, any non-trivial finite 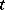 -group (
-group (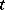 a prime number) is isomorphic to some quotient group
a prime number) is isomorphic to some quotient group 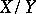 , where
, where 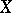 is a suitable
is a suitable 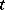 -modular Frobenius group with
-modular Frobenius group with 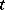 -modular Frobenius kernel
-modular Frobenius kernel 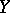 . See also [a8].
. See also [a8].
Historically, finite Frobenius groups have played a major role in many areas of group theory, notably in the analysis of  -transitive groups and finite simple groups (cf. also Transitive group; Simple finite group).
-transitive groups and finite simple groups (cf. also Transitive group; Simple finite group).
Frobenius groups can be defined for infinite groups as well. Those groups are the non-regular transitive permutation groups in which only the identity has more than one fixed point. Again, let  consist of the identity and those elements of the Frobenius group
consist of the identity and those elements of the Frobenius group  not occurring in any point stabilizer (cf. also Stabilizer). Contrary to the finite case, it is now not always true that
not occurring in any point stabilizer (cf. also Stabilizer). Contrary to the finite case, it is now not always true that 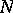 is a subgroup of
is a subgroup of 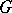 . See [a2] for examples.
. See [a2] for examples.
References
| [a1] | Yu.G. Berkovich, E.M. Zhmud, "Characters of finite groups" , Amer. Math. Soc. (1998/9) |
| [a2] | J.D. Dixon, B. Mortimer, "Permutation groups" , GTM , 163 , Springer (1996) |
| [a3] | G. Frobenius, "Ueber auflösbare Gruppen IV" Sitzungsber. Preuss. Akad. Wissenschaft. (1901) pp. 1216–1230 |
| [a4] | B. Huppert, "Endliche Gruppen" , I , Springer (1967) |
| [a5] | B. Huppert, "Character theory of finite groups" , Experim. Math. , 25 , de Gruyter (1998) |
| [a6] | I.M. Isaacs, "Character theory of finite groups" , Acad. Press (1976) |
| [a7] | E.B. Kuisch, R.W. van der Waall, "Homogeneous character induction" J. Algebra , 156 (1993) pp. 395–406 |
| [a8] | E.B. Kuisch, R.W. van der Waall, "Modular Frobenius groups" Manuscripta Math. , 90 (1996) pp. 403–427 |
| [a9] | J.G. Thompson, "Finite groups with fixed point free automorphisms of prime order" Proc. Nat. Acad. Sci. USA , 45 (1959) pp. 578–581 |
| [a10] | H. Zassenhaus, "Ueber endliche Fastkörper" Abh. Math. Sem. Univ. Hamburg , 11 (1936) pp. 187–220 |
Frobenius group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Frobenius_group&oldid=19132