Free module
A free object (a free algebra) in the variety of modules over a fixed ring 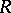 . If
. If  is associative and has a unit, then a free module is a module with a basis, that is, a linearly independent system of generators. The cardinality of a basis of a free module is called its rank. The rank is not always defined uniquely, that is, there are rings over which a free module can have two bases consisting of a different number of elements. This is equivalent to the existence over
is associative and has a unit, then a free module is a module with a basis, that is, a linearly independent system of generators. The cardinality of a basis of a free module is called its rank. The rank is not always defined uniquely, that is, there are rings over which a free module can have two bases consisting of a different number of elements. This is equivalent to the existence over 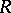 of two rectangular matrices
of two rectangular matrices  and
and 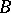 for which
for which
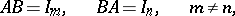 |
where 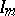 and
and  denote the unit matrices of orders
denote the unit matrices of orders 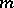 and
and  , respectively. However, non-uniqueness holds only for finite bases; if the rank of a free module is infinite, then all bases have the same cardinality. In addition, over rings that admit a homomorphism into a skew-field (in particular, over commutative rings), the rank of a free module is always uniquely defined.
, respectively. However, non-uniqueness holds only for finite bases; if the rank of a free module is infinite, then all bases have the same cardinality. In addition, over rings that admit a homomorphism into a skew-field (in particular, over commutative rings), the rank of a free module is always uniquely defined.
A ring  , considered as a left module over itself, is a free module of rank 1. Every left free module is a direct sum of free modules of rank 1. Every module
, considered as a left module over itself, is a free module of rank 1. Every left free module is a direct sum of free modules of rank 1. Every module  is representable as a quotient module
is representable as a quotient module 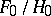 of a free module
of a free module 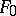 . The submodule
. The submodule 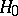 is, in turn, representable as a quotient module
is, in turn, representable as a quotient module  of a free module
of a free module 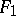 . By continuing this process one obtains the exact sequence
. By continuing this process one obtains the exact sequence
 |
which is called the free resolution of 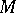 . Skew-fields can be characterized as rings over which all modules are free. Over a principal ideal domain a submodule of a free module is free. Near to free modules are projective modules and flat modules (cf. Projective module; Flat module).
. Skew-fields can be characterized as rings over which all modules are free. Over a principal ideal domain a submodule of a free module is free. Near to free modules are projective modules and flat modules (cf. Projective module; Flat module).
References
| [1] | P.M. Cohn, "Free rings and their relations" , Acad. Press (1971) |
| [2] | S. MacLane, "Homology" , Springer (1963) |
Free module. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Free_module&oldid=13029