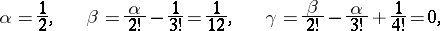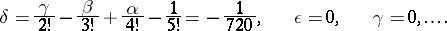A summation formula that connects the partial sums of a series with the integral and derivatives of its general term:
where  are the Bernoulli numbers and
are the Bernoulli numbers and  is the remainder. Using the Bernoulli polynomials
is the remainder. Using the Bernoulli polynomials  ,
,  , the remainder can be rewritten in the form
, the remainder can be rewritten in the form
For  the remainder
the remainder  can be expressed by means of the Bernoulli numbers:
can be expressed by means of the Bernoulli numbers:
If the derivatives 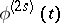 and
and 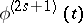 have the same sign and do not change sign on
have the same sign and do not change sign on 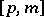 , then
, then
If, furthermore,
then the Euler–MacLaurin formula becomes
This version is used, for example, to derive the Stirling formula, in which case 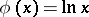 and
and  is the Euler constant. The formula has also been generalized to multiple sums.
is the Euler constant. The formula has also been generalized to multiple sums.
The Euler–MacLaurin formula finds application in the approximate calculation of definite integrals, the study of convergence of series, the computation of sums, and the expansion of functions in Taylor series. For example, for  ,
,  ,
, 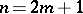 , and
, and 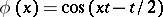 , it yields the expression
, it yields the expression
The Euler–MacLaurin formula plays an important role in the study of asymptotic expansions, number-theoretic estimates and finite-difference calculus.
Sometimes the Euler–MacLaurin formula is applied in the form
The formula was first obtained by L. Euler [1] as
where 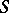 is the sum of the first terms of the series with general term
is the sum of the first terms of the series with general term 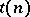 ,
, 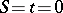 for
for 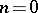 , and the coefficients are determined from the recurrence relations
, and the coefficients are determined from the recurrence relations
The formula was later discovered independently by C. MacLaurin [2].
References
| [1] | L. Euler, Comment. Acad. Sci. Imp. Petrop. , 6 (1738) pp. 68–97 |
| [2] | C. MacLaurin, "A treatise of fluxions" , 1–2 , Edinburgh (1742) |
| [3] | G.H. Hardy, "Divergent series" , Clarendon Press (1949) |
| [4] | N.E. Nörlund, "Volesungen über Differenzenrechnung" , Springer (1924) |
| [5] | A.O. [A.O. Gel'fond] Gelfond, "Differenzenrechnung" , Deutsch. Verlag Wissenschaft. (1958) (Translated from Russian) |
The use of the Euler–MacLaurin sum formula in numerical quadrature is discussed in [a1] and [a2]. By replacing the various derivatives by finite differences the quadrature rules of Bessel, Gauss and Gregory are obtained.
References
| [a1] | F.B. Hildebrand, "Introduction to numerical analysis" , McGraw-Hill (1974) |
| [a2] | J.F. Steffensen, "Interpolation" , Chelsea, reprint (1950) |
How to Cite This Entry:
Euler-MacLaurin formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Euler-MacLaurin_formula&oldid=11457
This article was adapted from an original article by Yu.N. Subbotin (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article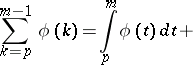
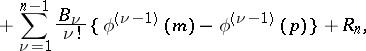
 are the Bernoulli numbers and
are the Bernoulli numbers and  is the remainder. Using the Bernoulli polynomials
is the remainder. Using the Bernoulli polynomials  ,
,  , the remainder can be rewritten in the form
, the remainder can be rewritten in the form
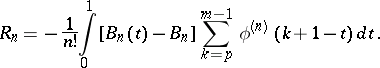
 the remainder
the remainder  can be expressed by means of the Bernoulli numbers:
can be expressed by means of the Bernoulli numbers:
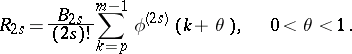
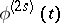 and
and 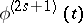 have the same sign and do not change sign on
have the same sign and do not change sign on 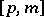 , then
, then
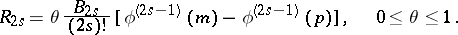
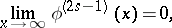

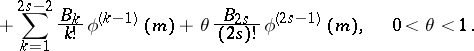
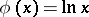 and
and  is the Euler constant. The formula has also been generalized to multiple sums.
is the Euler constant. The formula has also been generalized to multiple sums.
 ,
,  ,
, 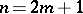 , and
, and 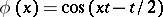 , it yields the expression
, it yields the expression
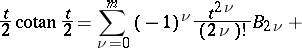
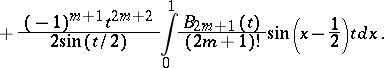
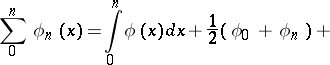

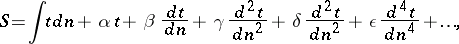
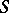 is the sum of the first terms of the series with general term
is the sum of the first terms of the series with general term 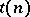 ,
, 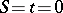 for
for 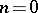 , and the coefficients are determined from the recurrence relations
, and the coefficients are determined from the recurrence relations