Dispersion method
in number theory
A method for solving certain binary equations (binary additive problems) of the form
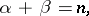 | (1) |
where 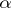 and
and  belong to sequences of natural numbers which are sufficiently dense and sufficiently well distributed in the sequence of natural numbers.
belong to sequences of natural numbers which are sufficiently dense and sufficiently well distributed in the sequence of natural numbers.
The method, which was developed by Yu.V. Linnik in 1958–1961 and is therefore also known as Linnik's dispersion method, combines elementary concepts of probability theory (in particular, the concept of dispersion and Chebyshev-type inequalities) with the analytic and algebraic ideas of I.M. Vinogradov and A. Weil. It may be described as follows (see also Additive number theory).
Equation (1) may be reduced to equations of the form
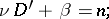 | (2) |
where  and
and 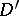 run, independently of each other, through certain values from a rectangular domain
run, independently of each other, through certain values from a rectangular domain  ,
,  , where
, where 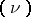 and
and 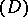 are certain intervals; moreover, the numbers
are certain intervals; moreover, the numbers  are prime, while various additional conditions may be imposed on
are prime, while various additional conditions may be imposed on 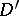 . Let
. Let 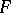 be the number of solutions of this equation.
be the number of solutions of this equation.
Now consider the equation
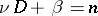 |
for an arbitrary 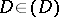 , and let
, and let 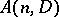 be the number of its solutions found by some heuristic means. The (hypothetical) number of expected solutions of equation (2) is then written in the form
be the number of its solutions found by some heuristic means. The (hypothetical) number of expected solutions of equation (2) is then written in the form
 |
An estimate of the difference 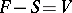 has the form
has the form
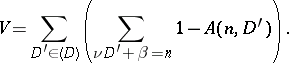 | (3) |
An application of the Cauchy inequality yields
 | (4) |
where 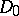 is the length of the interval
is the length of the interval 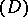 and
and
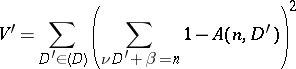 | (5) |
is the dispersion of the number of solutions of equation (2).
If the summation in (5) is extended over all 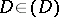 , all additional conditions imposed on
, all additional conditions imposed on 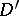 in (2) are dropped. At the same time the value of the dispersion can only increase; accordingly,
in (2) are dropped. At the same time the value of the dispersion can only increase; accordingly,
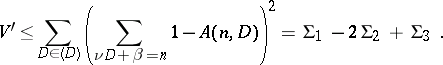 |
The sums 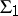 ,
, 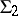 and
and  can be computed asymptotically in certain cases. The computation of
can be computed asymptotically in certain cases. The computation of 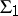 , which is the basic sum in the dispersion method, is the most difficult. The asymptotic computation of
, which is the basic sum in the dispersion method, is the most difficult. The asymptotic computation of 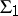 is effected using the Vinogradov method by computing, for certain functions, the number of fractional parts comprised in the given segment, and also by using the most recent estimates for trigonometric sums obtained by methods of algebraic geometry. Asymptotics for the sums
is effected using the Vinogradov method by computing, for certain functions, the number of fractional parts comprised in the given segment, and also by using the most recent estimates for trigonometric sums obtained by methods of algebraic geometry. Asymptotics for the sums 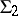 and
and 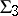 are found by elementary summations. If, as a result, the dispersion is found to be not too large, (3) and (4) yield an asymptotic expansion for the number of solutions of equation (2).
are found by elementary summations. If, as a result, the dispersion is found to be not too large, (3) and (4) yield an asymptotic expansion for the number of solutions of equation (2).
Combining the numbers of solutions of all equations of the type (2) yields an asymptotic formula for the number of solutions of equation (1).
The above scheme of the dispersion method may be used also to solve equations of the type
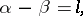 |
where 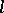 is a given non-zero integer.
is a given non-zero integer.
Linnik, et al. (see [3]) employed this method to solve a number of classical additive problems which, prior to the genesis of the dispersion method, could only be solved on the strength of heuristic or hypothetical conceptions. Such problems include the additive divisor problem (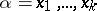 ,
, 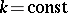 ,
,  ); the Titchmarsh problem on divisors (
); the Titchmarsh problem on divisors (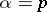 is a prime,
is a prime, 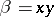 ); and the Hardy–Littlewood problem (
); and the Hardy–Littlewood problem (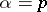 is a prime,
is a prime,  ).
).
The dispersion method also yielded solutions of certain analogues and generalizations of these problems; this includes, in particular, an asymptotic expansion for the number of solutions of the general Hardy–Littlewood equation
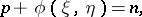 |
where 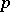 is a prime number and
is a prime number and 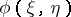 is a given primitive positive-definite quadratic form. The existence of an infinite set of prime numbers of the type
is a given primitive positive-definite quadratic form. The existence of an infinite set of prime numbers of the type
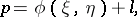 |
where 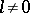 is an arbitrary fixed integer, was proved.
is an arbitrary fixed integer, was proved.
The domain of application of the dispersion method intersects with that of Linnik's large sieve method.
References
| [1] | Yu.V. Linnik, "The dispersion method in binary additive problems" , Amer. Math. Soc. (1963) (Translated from Russian) |
| [2] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
| [3] | B.M. Bredekhin, "The dispersion method and definite binary additive problems" Russian Math. Surveys , 20 : 2 (1965) pp. 85–125 Uspekhi Mat. Nauk , 20 : 2 (1965) pp. 89–130 |
| [4] | B.M. Bredekhin, Yu.V. Linnik, "A new method in analytic number theory" , Current problems in analytic number theory , Minsk (1974) pp. 5–22 (In Russian) |
Dispersion method. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dispersion_method&oldid=15408