Cosmological constant
A physical constant characterizing the properties of vacuum, sometimes introduced in the general theory of relativity. Einstein's equations (cf. Einstein equations) including the cosmological constant are
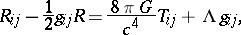 |
where  is the cosmological constant,
is the cosmological constant,  is the metric tensor,
is the metric tensor,  is the Ricci tensor,
is the Ricci tensor,  is the curvature of space,
is the curvature of space,  is the energy-momentum tensor,
is the energy-momentum tensor,  is the speed of light, and
is the speed of light, and  is the gravitational constant. These equations are the Lagrange equations for the action
is the gravitational constant. These equations are the Lagrange equations for the action
 |
where  is the action for matter and
is the action for matter and  denotes four-dimensional volume. A. Einstein introduced the cosmological constant in the general theory of relativity [1] in order to ensure that the equations of the gravitational field admit a spatially homogeneous static solution (the so-called Einstein cosmological model). However, since the advent of Friedmann's evolutionary cosmological model and its experimental verification, the fact that the original Einstein equations have no such solution is not considered a defect of the theory. There are no reliable indications that the cosmological constant is distinct from zero. However, the existence of a sufficiently small cosmological constant
denotes four-dimensional volume. A. Einstein introduced the cosmological constant in the general theory of relativity [1] in order to ensure that the equations of the gravitational field admit a spatially homogeneous static solution (the so-called Einstein cosmological model). However, since the advent of Friedmann's evolutionary cosmological model and its experimental verification, the fact that the original Einstein equations have no such solution is not considered a defect of the theory. There are no reliable indications that the cosmological constant is distinct from zero. However, the existence of a sufficiently small cosmological constant 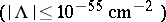 does not contradict the observed data or general physical principles.
does not contradict the observed data or general physical principles.
The existence of a cosmological constant may essentially modify certain steps in the evolution of the most widespread cosmological models (see [2], Chapt. 4). In this connection, it has been proposed that cosmological models with a cosmological constant should be utilized to explain certain properties of the distribution of quasars (see [3], [4], [5]).
The term  in the gravitational field equations may be incorporated in the energy-momentum tensor of vacuum (see [2]). In this case, vacuum has energy density
in the gravitational field equations may be incorporated in the energy-momentum tensor of vacuum (see [2]). In this case, vacuum has energy density  and pressure
and pressure  , corresponding to the state equation
, corresponding to the state equation  . In a theory with a cosmological constant the properties of vacuum already appear in the non-relativistic approximation. Thus, the gravitational potential of a point mass in a theory with a cosmological constant is (see [6], Chapt. 16)
. In a theory with a cosmological constant the properties of vacuum already appear in the non-relativistic approximation. Thus, the gravitational potential of a point mass in a theory with a cosmological constant is (see [6], Chapt. 16)
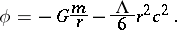 |
The term  is invariant under transformations of the local Lorentz group, corresponding to the principle of Lorentz-invariance of vacuum in quantum field theory. The concept of the cosmological constant as an index of the energy density and pressure of vacuum makes it possible, in principle, to relate the concept of a cosmological constant with the concepts of quantum field theory. There are various formulas that link the value of the cosmological constant to the fundamental physical constants and the age of the Universe (see [2], Chapt. 24).
is invariant under transformations of the local Lorentz group, corresponding to the principle of Lorentz-invariance of vacuum in quantum field theory. The concept of the cosmological constant as an index of the energy density and pressure of vacuum makes it possible, in principle, to relate the concept of a cosmological constant with the concepts of quantum field theory. There are various formulas that link the value of the cosmological constant to the fundamental physical constants and the age of the Universe (see [2], Chapt. 24).
References
| [1] | A. Einstein, Sitzungsber. K. Preuss. Akad. Wissenschaft. (1917) pp. 142–152 |
| [2] | Ya.B. Zel'dovich, I.D. Novikov, "Relativistic astrophysics" , 2. Relativistic astrophysics , Chicago (1983) (Translated from Russian) |
| [3] | V. Petrosian, E. Salpeter, P. Szekeres, Astrophys. J. , 147 (1967) pp. 1222–1226 |
| [4] | I.S. Shklovskii, Astron. Tsirkulyar , 429 (1967) |
| [5] | N.S. Kardashev, Astron. Tsirkulyar , 430 (1967) |
| [6] | R.C. Tolman, "Relativity, thermodynamics and cosmology" , Clarendon Press (1934) |
Comments
References
| [a1] | S. Weinberg, "Gravitation and cosmology" , Wiley (1972) |
Cosmological constant. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cosmological_constant&oldid=12365