Constructive quantum field theory
A branch of mathematical physics studying the properties of models of quantum field theory. One of the problems of constructive quantum field theory consists in the investigation of interacting quantum fields in real four-dimensional space-time. Mathematically, the existence of these fields has not yet been established (1987). Therefore the main efforts have been directed towards the study of the less singular models of quantum field theory in two- and three-dimensional space-time. Constructive quantum field theory is a synthesis of ideas and methods of axiomatic field theory and renormalization theory with modern mathematical methods. The concept of a relativistic quantum field itself admits various equivalent mathematical interpretations, enabling one to use methods from different areas of mathematics.
A quantum field can be treated either in terms of the theory of non-linear hyperbolic equations for operator-valued generalized functions, or in terms of the theory of generalized random fields (establishing a closed contact with statistical mechanics), or as a system of analytic functions of several complex variables (in the study of analytic properties of the  -matrix), or it can be considered from the point of view of
-matrix), or it can be considered from the point of view of 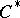 -algebras and representation theory.
-algebras and representation theory.
In the first works on constructive quantum field theory, mainly methods from functional analysis were used. A relativistic quantum field in two-dimensional space-time satisfying the Wightman axioms was first successfully constructed [8] using the Euclidean formulation [9] of quantum field theory, enabling one to invoke methods from probability theory and statistical mechanics.
A relativistic quantum field is completely characterized by its Wightman functions 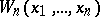 . The equivalent Euclidean formulation of quantum field theory is given in terms of Schwinger functions
. The equivalent Euclidean formulation of quantum field theory is given in terms of Schwinger functions 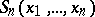 (these are obtained from
(these are obtained from 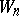 by analytic continuation to the Euclidean points
by analytic continuation to the Euclidean points 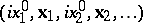 ), satisfying the Osterwalder–Schrader axioms. Under certain additional hypotheses it can be proved that the
), satisfying the Osterwalder–Schrader axioms. Under certain additional hypotheses it can be proved that the 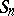 are moments of a probability measure with special properties. The method of constructing models of quantum field theory in which one begins by constructing a probability measure and then verifies the Osterwalder–Schrader axioms for its moments has been proved most convenient and is most widespread.
are moments of a probability measure with special properties. The method of constructing models of quantum field theory in which one begins by constructing a probability measure and then verifies the Osterwalder–Schrader axioms for its moments has been proved most convenient and is most widespread.
In the simplest case of a single scalar field one considers a measurable space 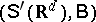 , where
, where 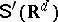 is the space of (real-valued) tempered distributions, and
is the space of (real-valued) tempered distributions, and 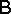 is the
is the  -algebra generated by the cylinder sets, and a class of probability measures
-algebra generated by the cylinder sets, and a class of probability measures 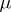 on
on 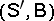 possessing the following special properties.
possessing the following special properties.
1) On 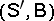 there is defined a natural representation of the connected component
there is defined a natural representation of the connected component  of the identity of the Euclidean group of motions of
of the identity of the Euclidean group of motions of 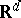 by automorphisms of the
by automorphisms of the 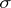 -algebra
-algebra 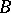 . It is required that the measure
. It is required that the measure 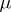 be
be  -invariant. This condition is the Euclidean expression of relativistic invariance.
-invariant. This condition is the Euclidean expression of relativistic invariance.
2) Let  be the generalized random field on
be the generalized random field on  defined by
defined by 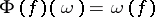 ,
, 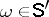 ,
, 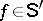 . For any function
. For any function 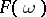 on
on  one defines
one defines 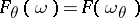 , where
, where  ,
, 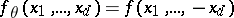 . Let
. Let 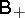 be the
be the  -algebra generated by the functions
-algebra generated by the functions 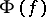 with
with 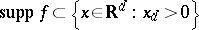 . It is required that the positivity condition of Osterwalder–Schrader holds:
. It is required that the positivity condition of Osterwalder–Schrader holds:
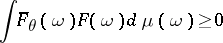 |
for any 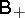 -measurable
-measurable  on
on  . This condition expresses the positive definiteness of the scalar product in relativistic Hilbert space. For two-dimensional models the somewhat stronger Markov condition for the field
. This condition expresses the positive definiteness of the scalar product in relativistic Hilbert space. For two-dimensional models the somewhat stronger Markov condition for the field 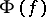 is widely used.
is widely used.
3) It is required that there exists a norm  on
on 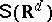 such that
such that
 |
is uniformly bounded and continuous with respect to  on
on
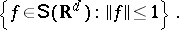 |
4) The subgroup of translations of the group 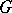 must act ergodically. This expresses the uniqueness of vacuum in relativistic theory.
must act ergodically. This expresses the uniqueness of vacuum in relativistic theory.
If the measure 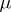 satisfies the conditions 1)–4), then it is called a quantum measure, and the corresponding generalized random field
satisfies the conditions 1)–4), then it is called a quantum measure, and the corresponding generalized random field 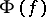 is called a Euclidean field. The moments of the quantum measure,
is called a Euclidean field. The moments of the quantum measure,
 |
are the Schwinger functions, which satisfy the Osterwalder–Schrader axioms. There exists a unique relativistic quantum field satisfying all Wightman axioms and such the analytic continuations of its Wightman functions to the Euclidean points are the same as the Schwinger functions of the given quantum measure 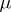 . If a measure
. If a measure 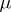 satisfies conditions 1)–3) only, then conditions 1)–4) hold for all its ergodic components.
satisfies conditions 1)–3) only, then conditions 1)–4) hold for all its ergodic components.
One class of quantum measures is easily constructed, namely the Gaussian measures (depending on a parameter 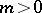 ) with characteristic functional
) with characteristic functional
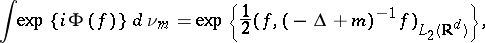 |
where  is the Laplace operator. The corresponding Euclidean field is called the free (scalar) Euclidean field of mass
is the Laplace operator. The corresponding Euclidean field is called the free (scalar) Euclidean field of mass 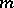 .
.
The construction of non-Gaussian measures presents great difficulties, and the results depend essentially on the dimension 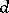 . The usual procedure is as follows. A function
. The usual procedure is as follows. A function 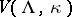 is constructed on
is constructed on 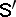 (the interaction potential), depending on the parameters
(the interaction potential), depending on the parameters  , called the volume cut-off, and
, called the volume cut-off, and 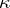 , called the ultraviolet cut-off. Heuristically
, called the ultraviolet cut-off. Heuristically 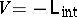 (see Quantum field theory).
(see Quantum field theory). 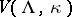 is additive in
is additive in 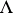 , but not in
, but not in 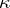 . The measure
. The measure
 |
is then constructed (the definition of 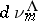 is given below) and one studies the limits of the sequence of measures
is given below) and one studies the limits of the sequence of measures 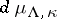 as
as 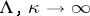 . For certain potentials
. For certain potentials 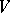 , the limit measure
, the limit measure 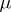 satisfies conditions 1)–4). Convergence of the measures is usually understood in the sense of convergence of all moments and characteristic functionals.
satisfies conditions 1)–4). Convergence of the measures is usually understood in the sense of convergence of all moments and characteristic functionals.
For example, for models with interaction  with
with 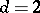 , this procedure is made concrete in the following way. Let
, this procedure is made concrete in the following way. Let 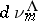 be a Gaussian measure on
be a Gaussian measure on 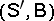 with characteristic functional
with characteristic functional
 |
where  is a self-adjoint extension of the Laplace operator with certain boundary conditions on the boundary
is a self-adjoint extension of the Laplace operator with certain boundary conditions on the boundary  of the domain
of the domain 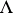 in the plane
in the plane 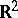 (usually
(usually 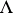 is a rectangle); the kernel
is a rectangle); the kernel  can, for example, be the Green function for the Dirichlet problem. Suppose further that
can, for example, be the Green function for the Dirichlet problem. Suppose further that 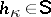 and
and  as
as  . The random variable
. The random variable 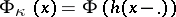 is, for
is, for 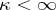 , a smooth function of the parameter
, a smooth function of the parameter 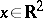 and
and
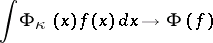 |
as 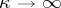 . Set
. Set
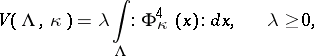 |
where 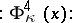 is a Wick power of
is a Wick power of 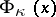 (see Fock space). Then
(see Fock space). Then
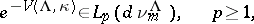 |
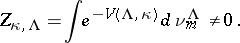 |
Finally, let
 |
As 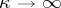 ,
, 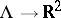 , all moments and characteristic functionals of the measures
, all moments and characteristic functionals of the measures 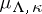 converge to the moments and characteristic functionals of a certain quantum measure
converge to the moments and characteristic functionals of a certain quantum measure 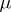 . It turns out that for sufficiently large
. It turns out that for sufficiently large  the measures
the measures 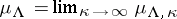 with different boundary conditions on
with different boundary conditions on 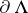 have, in general, different limits as
have, in general, different limits as  . In this case it is said that there is a phase transition.
. In this case it is said that there is a phase transition.
The central problem of constructive quantum field theory consists of describing all the quantum measures (phases) corresponding to a given interaction potential, and in studying the properties of the corresponding relativistic quantum fields. In the first instance one is interested in the spectral properties of the mass operator of the Poincaré group (the study of which reduces to examining the behaviour of the Schwinger functions at large distances) and the properties of the  -matrix such as its analyticity, unitarity, etc. (the
-matrix such as its analyticity, unitarity, etc. (the 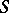 -matrix is studied by analytic continuation of the Schwinger functions).
-matrix is studied by analytic continuation of the Schwinger functions).
The existence of quantum measures for 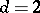 was proved (1978) for interaction potentials
was proved (1978) for interaction potentials 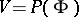 , where
, where 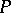 is any polynomial that is bounded from below,
is any polynomial that is bounded from below,  (the sine-Gordon equation) and certain other non-polynomial interactions, as well as for some multi-components fields
(the sine-Gordon equation) and certain other non-polynomial interactions, as well as for some multi-components fields  . For a sufficiently weak polynomial interaction a study has been undertaken of the dependence of the spectrum of the mass operator on the form of the polynomial, and the existence of the
. For a sufficiently weak polynomial interaction a study has been undertaken of the dependence of the spectrum of the mass operator on the form of the polynomial, and the existence of the  -matrix has been established. Fermion and scalar fields with Yukawa interaction have also been investigated. The Euclidean fermion field is not a generalized random field and takes values in a Grassmann algebra. However, one can "integrate out" the fermion variables and the problem then reduces to estimating certain path integrals with respect to ordinary Gaussian measures. All these models have phase transitions for certain values of the parameters.
-matrix has been established. Fermion and scalar fields with Yukawa interaction have also been investigated. The Euclidean fermion field is not a generalized random field and takes values in a Grassmann algebra. However, one can "integrate out" the fermion variables and the problem then reduces to estimating certain path integrals with respect to ordinary Gaussian measures. All these models have phase transitions for certain values of the parameters.
The constructions of relativistic quantum fields described above lead only to the so-called vacuum sectors, that is, to quantum fields satisfying the Wightman axioms, supplemented by the axiom of existence of vacuum. These fields are solutions of non-linear equations with obvious initial conditions. For a number of two-dimensional models (sine-Gordon, etc.) soliton sectors have been constructed in which vacuum is absent but which have a discrete spectrum for the mass operator; from the physical point of view this is of great interest. These new sectors are constructed by means of special automorphisms of the  -algebra of observables in the vacuum sector.
-algebra of observables in the vacuum sector.
For 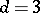 , the existence has been proved of quantum measures in a model with
, the existence has been proved of quantum measures in a model with 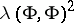 interaction, where
interaction, where
 |
Here the interaction potential has the form
 |
 |
where 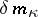 and
and 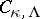 are certain definite functions of
are certain definite functions of 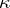 and
and 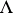 ; that is, counter terms are added. Also, in this model a phase transition occurs for sufficiently large
; that is, counter terms are added. Also, in this model a phase transition occurs for sufficiently large 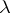 , and it is accompanied by
, and it is accompanied by 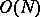 -symmetry breakdown.
-symmetry breakdown.
References
| [1] | N.N. Bogolyubov, A.A. Logunov, I.T. Todorov, "Introduction to axiomatic quantum field theory" , Benjamin (1975) (Translated from Russian) |
| [2] | , Constructive quantum field theory , Lect. notes in physics , 25 , Springer (1973) |
| [3] | M. Reed, B. Simon, "Methods of modern mathematical physics" , 1–4 , Acad. Press (1972–1978) |
| [4] | B. Simon, "The  model of Euclidean (quantum) field theory" , Princeton Univ. Press (1974) model of Euclidean (quantum) field theory" , Princeton Univ. Press (1974) |
| [5] | C. Hepp, "Théorie de la renormalisation" , Lect. notes in physics , 2 , Springer (1969) |
| [6] | , Euclidean quantum field theory. The Markov approach , Moscow (1978) (In Russian; translated from English) |
| [7] | R.L. Dobrushin, R.A. Minlos, "Polynomials of linear random functions" Uspekhi Mat. Nauk , 32 : 2 (1977) pp. 67–122 (In Russian) |
| [8] | J. Glimm, A. Jaffe, T. Spencer, "The Wightman axioms and particle structure in the 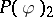 quantum field model" Ann. of Math. , 100 : 3 (1974) pp. 585–632 quantum field model" Ann. of Math. , 100 : 3 (1974) pp. 585–632 |
| [9] | E. Nelson, "Construction of quantum fields from Markoff fields" J. Funct. Anal. , 12 : 1 (1973) pp. 97–112 |
| [10] | J. Fröhlich, "Schwinger functions and their generating functionals II. Markovian and generalized path space measures on 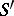 " Adv. Math. , 23 : 2 (1977) pp. 119–181 " Adv. Math. , 23 : 2 (1977) pp. 119–181 |
Comments
References
| [a1] | J. Glimm, A. Jaffe, "Quantum physics, a functional integral point of view" , Springer (1981) |
Constructive quantum field theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Constructive_quantum_field_theory&oldid=12337