Character of a representation of a group
In the case of a finite-dimensional representation 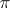 this is the function
this is the function 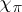 on the group
on the group 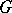 defined by the formula
defined by the formula
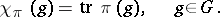 |
For arbitrary continuous representations of a topological group 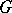 over
over 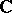 this definition is generalized as follows:
this definition is generalized as follows:
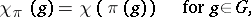 |
where 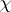 is a linear functional defined on some ideal
is a linear functional defined on some ideal 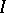 of the algebra
of the algebra 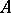 generated by the family of operators
generated by the family of operators  ,
, 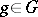 , that is invariant under inner automorphisms of
, that is invariant under inner automorphisms of 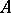 . In certain cases the character of a representation
. In certain cases the character of a representation 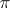 is defined as that of the representation of a certain group algebra of
is defined as that of the representation of a certain group algebra of  determined by
determined by 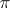 (see Character of a representation of an associative algebra).
(see Character of a representation of an associative algebra).
The character of a direct sum (of a tensor product) of finite-dimensional representations is equal to the sum (the product) of the characters of these representations. The character of a finite-dimensional representation of a group is a function that is constant on classes of conjugate elements; the character of a continuous finite-dimensional unitary representation of a group is a continuous positive-definite function on the group.
In many cases the character of a representation of a group determines the representation uniquely up to equivalence; for example, the character of an irreducible finite-dimensional representation over a field of characteristic 0 determines the representation uniquely up to spatial equivalence; the character of a finite-dimensional continuous unitary representation of a compact group is determining up to unitary equivalence.
The character of a representation of a locally compact group 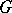 admitting an extension to a representation of the algebra of continuous functions of compact support on
admitting an extension to a representation of the algebra of continuous functions of compact support on 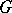 can be defined by a measure on
can be defined by a measure on 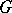 ; in particular, the character of the regular representation of a unimodular group is given by a probability point measure concentrated at the unit element of
; in particular, the character of the regular representation of a unimodular group is given by a probability point measure concentrated at the unit element of  . The character of a representation
. The character of a representation 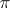 of a Lie group
of a Lie group 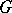 admitting an extension to a representation of the algebra
admitting an extension to a representation of the algebra 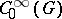 of infinitely-differentiable functions of compact support on
of infinitely-differentiable functions of compact support on  can be defined as a generalized function on
can be defined as a generalized function on 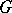 . If
. If 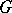 is a nilpotent or a linear semi-simple Lie group, then the characters of irreducible unitary representations
is a nilpotent or a linear semi-simple Lie group, then the characters of irreducible unitary representations 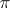 of
of 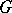 are defined by locally integrable functions
are defined by locally integrable functions 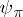 according to the formula
according to the formula
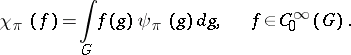 |
These characters determine the representation  uniquely up to unitary equivalence.
uniquely up to unitary equivalence.
If the group 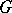 is compact, every continuous positive-definite function on
is compact, every continuous positive-definite function on  that is constant on classes of conjugate elements can be expanded into a series with respect to the characters of the irreducible representations
that is constant on classes of conjugate elements can be expanded into a series with respect to the characters of the irreducible representations 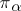 of
of  . The series converges uniformly on
. The series converges uniformly on 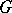 and the characters
and the characters 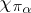 form an orthonormal system in the space
form an orthonormal system in the space 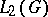 that is complete in the subspace of functions in
that is complete in the subspace of functions in 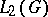 that are constant on classes of conjugate elements in
that are constant on classes of conjugate elements in 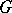 . If
. If 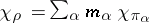 is the expansion of the character of a continuous finite-dimensional representation
is the expansion of the character of a continuous finite-dimensional representation 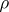 of the group
of the group 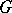 with respect to the characters
with respect to the characters  , then the
, then the 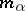 are integers, namely, the multiplicities with which the
are integers, namely, the multiplicities with which the 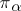 occur in
occur in 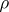 . If
. If  is a continuous representation of
is a continuous representation of 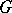 in a quasi-complete, barrelled, locally convex topological space
in a quasi-complete, barrelled, locally convex topological space 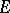 , then there exists a maximal subspace
, then there exists a maximal subspace  of
of 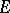 such that the restriction of
such that the restriction of 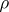 to
to 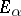 is a multiple of
is a multiple of 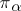 , and there is a continuous projection
, and there is a continuous projection 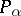 of
of  onto
onto 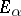 , defined by
, defined by
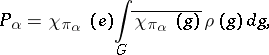 |
where 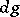 is the Haar measure on
is the Haar measure on 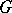 for which
for which 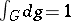 .
.
References
| [1] | A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) |
| [2] | C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962) |
| [3] | J. Dixmier, "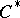 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
| [4] | G.F. Frobenius, J.-P. Serre (ed.) , Gesammelte Abhandlungen , Springer (1968) |
| [5] | M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) |
| [6] | D.E. Littlewood, "The theory of group characters and matrix representations of groups" , Clarendon Press (1950) |
Character of a representation of a group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Character_of_a_representation_of_a_group&oldid=15563