Best approximation
of a function  by functions
by functions  from a fixed set
from a fixed set 
The quantity
 |
where 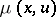 is the error of approximation (see Approximation of functions, measure of). The concept of a best approximation is meaningful in an arbitrary metric space
is the error of approximation (see Approximation of functions, measure of). The concept of a best approximation is meaningful in an arbitrary metric space  when
when  is defined by the distance between
is defined by the distance between  and
and  ; in this case
; in this case 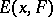 is the distance from
is the distance from  to the set
to the set 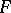 . If
. If 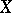 is a normed linear space, then for a fixed
is a normed linear space, then for a fixed  the best approximation
the best approximation
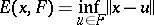 | (1) |
may be regarded as a functional defined on 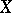 (the functional of best approximation).
(the functional of best approximation).
The functional of best approximation is continuous, whatever the set 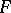 . If
. If 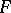 is a subspace, the functional of best approximation is a semi-norm, i.e.
is a subspace, the functional of best approximation is a semi-norm, i.e.
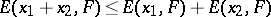 |
and
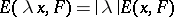 |
for any  . If
. If  is a finite-dimensional subspace, then for any
is a finite-dimensional subspace, then for any 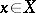 there exists an element
there exists an element 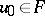 (an element of best approximation) at which the infimum in (1) is attained:
(an element of best approximation) at which the infimum in (1) is attained:
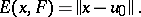 |
In a space 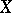 with a strictly convex norm, the element of best approximation is unique.
with a strictly convex norm, the element of best approximation is unique.
Through the use of duality theorems, the best approximation in a normed linear space 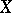 can be expressed in terms of the supremum of the values of certain functionals from the adjoint space
can be expressed in terms of the supremum of the values of certain functionals from the adjoint space 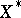 (see, e.g. [5], [8]). If
(see, e.g. [5], [8]). If 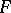 is a closed convex subset of
is a closed convex subset of 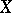 , then for any
, then for any 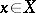
 | (2) |
in particular, if 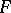 is a subspace, then
is a subspace, then
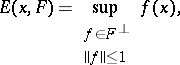 | (3) |
where 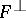 is the set of functionals
is the set of functionals  in
in 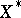 such that
such that 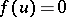 for any
for any 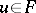 . In the function spaces
. In the function spaces 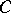 or
or  , the right-hand sides of (2) and (3) take explicit forms depending on the form of the linear functional. In a Hilbert space
, the right-hand sides of (2) and (3) take explicit forms depending on the form of the linear functional. In a Hilbert space  , the best approximation of an element
, the best approximation of an element  by an
by an  -dimensional subspace
-dimensional subspace  is obtained by orthogonal projection on
is obtained by orthogonal projection on 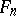 and can be calculated; one has:
and can be calculated; one has:
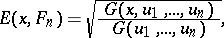 |
where  form a basis of
form a basis of 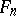 and
and 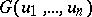 is the Gram determinant, the elements of which are the scalar products
is the Gram determinant, the elements of which are the scalar products  ,
,  . If
. If 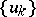 is an orthonormal basis, then
is an orthonormal basis, then
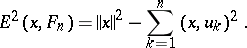 |
In the space 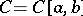 one has the following estimate for the best uniform approximation of a function
one has the following estimate for the best uniform approximation of a function  by an
by an  -dimensional Chebyshev subspace
-dimensional Chebyshev subspace 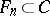 (the de la Vallée-Poussin theorem): If for some function
(the de la Vallée-Poussin theorem): If for some function 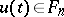 there exist
there exist  points
points 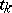 ,
, 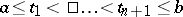 , for which the difference
, for which the difference
 |
takes values with alternating signs, then
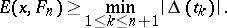 |
For best approximations in 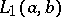 see Markov criterion. In several important cases, the best approximations of functions by finite-dimensional subspaces can be bounded from above in terms of differential-difference characteristics (e.g. the modulus of continuity) of the approximated function or its derivatives.
see Markov criterion. In several important cases, the best approximations of functions by finite-dimensional subspaces can be bounded from above in terms of differential-difference characteristics (e.g. the modulus of continuity) of the approximated function or its derivatives.
The concept of a best uniform approximation of continuous functions by polynomials is due to P.L. Chebyshev (1854), who developed the theoretical foundations of the concept and established a criterion for polynomials of best approximation in the metric space 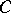 (see Polynomial of best approximation).
(see Polynomial of best approximation).
The best approximation of a class of functions is the supremum of the best approximations of the functions 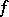 in the given class
in the given class 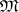 by a fixed set of functions
by a fixed set of functions 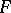 , i.e. the quantity
, i.e. the quantity
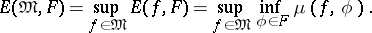 |
The number  characterizes the maximum deviation (in the specific metric chosen) of the class
characterizes the maximum deviation (in the specific metric chosen) of the class  from the approximating set
from the approximating set 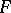 and indicates the minimal possible error to be expected when approximating an arbitrary function
and indicates the minimal possible error to be expected when approximating an arbitrary function  by functions of
by functions of 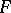 .
.
Let  be a subset of a normed linear function space
be a subset of a normed linear function space  , let
, let 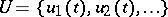 be a linearly independent system of functions in
be a linearly independent system of functions in  and let
and let 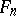 ,
,  be the subspaces generated by the first
be the subspaces generated by the first  elements of this system. By investigating the sequence
elements of this system. By investigating the sequence  ,
, 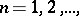 one can draw conclusions regarding both the structural and smoothness properties of the functions in
one can draw conclusions regarding both the structural and smoothness properties of the functions in  and the approximation properties of the system
and the approximation properties of the system  relative to
relative to 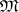 . If
. If  is a Banach function space and
is a Banach function space and 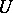 is closed in
is closed in 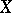 , i.e.
, i.e.  , then
, then  as
as 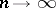 if and only if
if and only if  is a compact subset of
is a compact subset of  .
.
In various important cases, e.g. when the  are subspaces of trigonometric polynomials or periodic splines, and the class
are subspaces of trigonometric polynomials or periodic splines, and the class 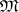 is defined by conditions imposed on the norm or on the modulus of continuity of some derivative
is defined by conditions imposed on the norm or on the modulus of continuity of some derivative  , the numbers
, the numbers 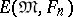 can be calculated explicitly [5]. In the non-periodic case, results are available concerning the asymptotic behaviour of
can be calculated explicitly [5]. In the non-periodic case, results are available concerning the asymptotic behaviour of 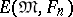 as
as  .
.
References
| [1] | P.L. Chebyshev, "Complete collected works" , 2 , Moscow (1947) (In Russian) |
| [2] | N.I. [N.I. Akhiezer] Achiezer, "Theory of approximation" , F. Ungar (1956) (Translated from Russian) |
| [3] | V.K. Dzyadyk, "Introduction to the theory of uniform approximation of functions by polynomials" , Moscow (1977) (In Russian) |
| [4] | V.L. Goncharov, "The theory of interpolation and approximation of functions" , Moscow (1954) (In Russian) |
| [5] | N.P. Korneichuk, "Extremal problems in approximation theory" , Moscow (1976) (In Russian) |
| [6] | S.M. Nikol'skii, "Approximation of functions of several variables and imbedding theorems" , Springer (1975) (Translated from Russian) |
| [7] | A.F. Timan, "Theory of approximation of functions of a real variable" , Pergamon (1963) (Translated from Russian) |
| [8] | V.M. Tikhomirov, "Some problems in approximation theory" , Moscow (1976) (In Russian) |
| [9] | P.J. Laurent, "Approximation et optimisation" , Hermann (1972) |
Comments
In Western literature an element, a functional or a polynomial of best approximation is also called a best approximation.
References
| [a1] | G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966) |
| [a2] | E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff |
| [a3] | J.R. Rice, "The approximation of functions" , 1. Linear theory , Addison-Wesley (1964) |
| [a4] | A. Pinkus, " -widths in approximation theory" , Springer (1985) (Translated from Russian) -widths in approximation theory" , Springer (1985) (Translated from Russian) |
Best approximation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Best_approximation&oldid=16361